7.4 Determining Empirical and Molecular Formulas
Learning Objectives
By the end of this section, you will be able to:
- Determine the empirical formula of a compound
- Determine the molecular formula of a compound
Determination of Empirical Formulas
As previously mentioned, the most common approach to determining a compound’s chemical formula is to first measure the masses of its constituent elements. However, we must keep in mind that chemical formulas represent the relative numbers, not masses, of atoms in the substance. Therefore, any experimentally derived data involving mass must be used to derive the corresponding numbers of atoms in the compound. To accomplish this, we can use molar masses to convert the mass of each element to a number of moles. We then consider the moles of each element relative to each other, converting these numbers into a whole-number ratio that can be used to derive the empirical formula of the substance. Consider a sample of compound determined to contain 1.71 g C and 0.287 g H. The corresponding numbers of atoms (in moles) are:
[latex]1.17 \;\text{g C} \times \frac{1 \;\text{mol C}}{12.01 \;\text{g C}} = 0.142 \;\text{mol C}[/latex]
[latex]0.287 \;\text{g H} \times \frac{1 \;\text{mol H}}{1.008 \;\text{g H}} = 0.284 \;\text{mol H}[/latex]
Thus, we can accurately represent this compound with the formula C0.142H0.248. Of course, per accepted convention, formulas contain whole-number subscripts, which can be achieved by dividing each subscript by the smaller subscript:
(Recall that subscripts of “1” are not written but rather assumed if no other number is present.)
The empirical formula for this compound is thus CH2. This may or not be the compound’s molecular formula as well; however, we would need additional information to make that determination (as discussed later in this section).
A molecular formula is the true formula for a compound. It lists how many atoms of each element are in the compound. The empirical formula is the simplest or most reduced ratio of elements in a compound. If a compound’s chemical formula cannot be reduced any further, then the empirical formula is the same as the molecular formula.
Consider as another example a sample of compound determined to contain 5.31 g Cl and 8.40 g O. Following the same approach yields a tentative empirical formula of:
[latex]\text{Cl}_{0.150}\text{O}_{0.525} \; = \; \text{Cl}_{\frac{0.150}{0.150}} \; \text{O}_{\frac{0.525}{0.150}} = \text{ClO}_{3.5}[/latex]
In this case, dividing by the smallest subscript still leaves us with a decimal subscript in the empirical formula. To convert this into a whole number, we must multiply each of the subscripts by two, retaining the same atom ratio and yielding Cl2O7 as the final empirical formula.
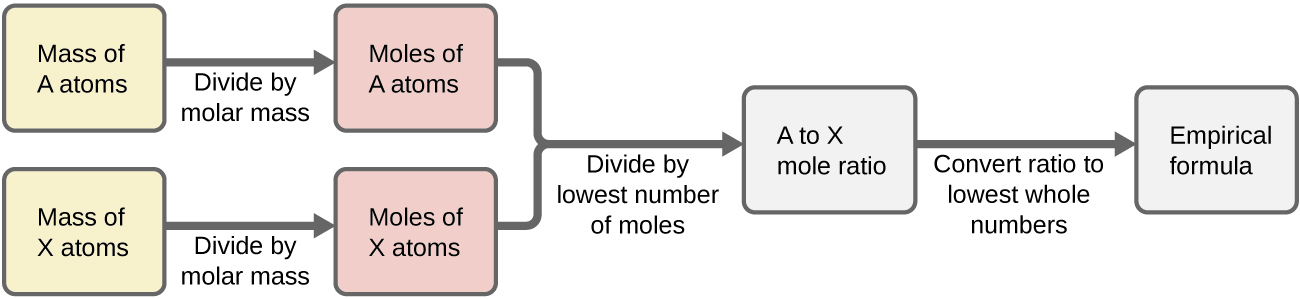
In summary, empirical formulas are derived from experimentally measured element masses by:
- Deriving the number of moles of each element from its mass
- Dividing each element’s molar amount by the smallest molar amount to yield subscripts for a tentative empirical formula
- Multiplying all coefficients by an integer, if necessary, to ensure that the smallest whole-number ratio of subscripts is obtained
Figure 7.4a outlines this procedure in flow chart fashion for a substance containing elements A and X.
Example 7.4a
Determining a Compound’s Empirical Formula from the Masses of Its Elements
A sample of the black mineral hematite (Figure 7.3b), an oxide of iron found in many iron ores, contains 34.97 g of iron and 15.03 g of oxygen. What is the empirical formula of hematite?

Solution
For this problem, we are given the mass in grams of each element. Begin by finding the moles of each:
Next, derive the iron-to-oxygen molar ratio by dividing by the lesser number of moles:
The ratio is 1.000 mol of iron to 1.500 mol of oxygen (Fe1O1.5). Finally, multiply the ratio by two to get the smallest possible whole number subscripts while still maintaining the correct iron-to-oxygen ratio:
The empirical formula is Fe2O3.
Exercise 7.4a
What is the empirical formula of a compound if a sample contains 0.130 g of nitrogen and 0.370 g of oxygen?
Check Your Answer[1]
Watch Calculating Percent Composition and Empirical Formulas
Video source: Mr. Causey. (2013, March 22). Calculating percent composition and empirical formulas [Video]. YouTube.
Deriving Empirical Formulas from Percent Composition
Finally, with regard to deriving empirical formulas, consider instances in which a compound’s percent composition is available rather than the absolute masses of the compound’s constituent elements. In such cases, the percent composition can be used to calculate the masses of elements present in any convenient mass of compound; these masses can then be used to derive the empirical formula in the usual fashion.
Example 7.4b
Determining an Empirical Formula from Percent Composition
The bacterial fermentation of grain to produce ethanol forms a gas with a percent composition of 27.29% C and 72.71% O (Figure 7.3c). What is the empirical formula for this gas?

Solution
Since the scale for percentages is 100, it is most convenient to calculate the mass of elements present in a sample weighing 100 g. The calculation is “most convenient” because, per the definition for percent composition, the mass of a given element in grams is numerically equivalent to the element’s mass percentage. This numerical equivalence results from the definition of the “percentage” unit, whose name is derived from the Latin phrase per centum meaning “by the hundred.” Considering this definition, the mass percentages provided may be more conveniently expressed as fractions:
The molar amounts of carbon and hydrogen in a 100-g sample are calculated by dividing each element’s mass by its molar mass:
Coefficients for the tentative empirical formula are derived by dividing each molar amount by the lesser of the two:
Since the resulting ratio is one carbon to two oxygen atoms, the empirical formula is CO2.
Exercise 7.4b
What is the empirical formula of a compound containing 40.0% C, 6.71% H, and 53.28% O?
Check Your Answer[2]
Derivation of Molecular Formulas
Recall that empirical formulas are symbols representing the relative numbers of a compound’s elements. Determining the absolute numbers of atoms that compose a single molecule of a covalent compound requires knowledge of both its empirical formula and its molecular mass or molar mass. These quantities may be determined experimentally by various measurement techniques. Molecular mass, for example, is often derived from the mass spectrum of the compound (see discussion of this technique in the previous chapter on atoms and molecules). Molar mass can be measured by a number of experimental methods, many of which will be introduced in later chapters of this text.
Molecular formulas are derived by comparing the compound’s molecular or molar mass to its empirical formula mass. As the name suggests, an empirical formula mass is the sum of the average atomic masses of all the atoms represented in an empirical formula. If we know the molecular (or molar) mass of the substance, we can divide this by the empirical formula mass in order to identify the number of empirical formula units per molecule, which we designate as n:
The molecular formula is then obtained by multiplying each subscript in the empirical formula by n, as shown by the generic empirical formula AxBy:
For example, consider a covalent compound whose empirical formula is determined to be CH2O. The empirical formula mass for this compound is approximately 30 amu (the sum of 12 amu for one C atom, 2 amu for two H atoms, and 16 amu for one O atom). If the compound’s molecular mass is determined to be 180 amu, this indicates that molecules of this compound contain six times the number of atoms represented in the empirical formula:
Molecules of this compound are then represented by molecular formulas whose subscripts are six times greater than those in the empirical formula:
Note that this same approach may be used when the molar mass (g/mol) instead of the molecular mass (amu) is used. In this case, we are merely considering one mole of empirical formula units and molecules, as opposed to single units and molecules.
Example 7.4c
Determination of the Molecular Formula for Nicotine
Nicotine, an alkaloid in the nightshade family of plants that is mainly responsible for the addictive nature of cigarettes, contains 74.02% C, 8.710% H, and 17.27% N. If 40.57 g of nicotine contains 0.2500 mol nicotine, what is the molecular formula?
Solution
Determining the molecular formula from the provided data will require comparison of the compound’s empirical formula mass to its molar mass. As the first step, use the percent composition to derive the compound’s empirical formula. Assuming a convenient, a 100-g sample of nicotine yields the following molar amounts of its elements:
Next, we calculate the molar ratios of these elements relative to the least abundant element, N.
The C-to-N and H-to-N molar ratios are adequately close to whole numbers, and so the empirical formula is C5H7N. The empirical formula mass for this compound is therefore 81.13 amu/formula unit, or 81.13 g/mol formula unit.
We calculate the molar mass for nicotine from the given mass and molar amount of compound:
Comparing the molar mass and empirical formula mass indicates that each nicotine molecule contains two formula units:
Thus, we can derive the molecular formula for nicotine from the empirical formula by multiplying each subscript by two:
Exercise 7.4c
What is the molecular formula of a compound with a percent composition of 49.47% C, 5.201% H, 28.84% N, and 16.48% O, and a molecular mass of 194.2 amu?
Check Your Answer[3]
Glucose, an example of empirical vs molecular formulas
Consider glucose, the sugar that circulates in our blood to provide fuel for the body and brain. Results from combustion analysis of glucose report that glucose contains 39.68% carbon and 6.58% hydrogen. Because combustion occurs in the presence of oxygen, it is impossible to directly determine the percentage of oxygen in a compound by using combustion analysis; other more complex methods are necessary. Assuming that the remaining percentage is due to oxygen, then glucose would contain 53.79% oxygen. A 100.0 g sample of glucose would therefore contain 39.68 g of carbon, 6.58 g of hydrogen, and 53.79 g of oxygen. To calculate the number of moles of each element in the 100.0 g sample, divide the mass of each element by its molar mass:
[latex]moles \, C = 39.68 \, g \, C \times {1 \, mol \, C \over 12.011 \, g \, C } = 3.304 \, mol \, C \label{3.3.4a}[/latex]
[latex]moles \, H = 6.58 \, g \, H \times {1 \, mol \, H \over 1.0079 \, g \, H } = 6.53 \, mol \, H \label{3.3.4b}[/latex]
[latex]moles \, O = 53.79 \, g \, O \times {1 \, mol \, O \over 15.9994 \, g \, O } = 3.362 \, mol \, O \label{3.3.4c}[/latex]
Once again, the subscripts of the elements in the empirical formula are found by dividing the number of moles of each element by the number of moles of the element present in the smallest amount:
[latex]C: {3.304 \over 3.304} = 1.000 \, \, \, \, H: {6.53 \over 3.304} = 1.98 \, \, \, \, O: {3.362 \over 3.304} = 1.018[/latex]
The oxygen:carbon ratio is 1.018, or approximately 1, and the hydrogen:carbon ratio is approximately 2. The empirical formula of glucose is therefore CH2O, but what is its molecular formula?
Many known compounds have the empirical formula CH2O, including formaldehyde, which is used to preserve biological specimens and has properties that are very different from the sugar circulating in the blood. At this point, it cannot be known whether glucose is CH2O, C2H4O2, or any other (CH2O)n. However, the experimentally determined molar mass of glucose (180 g/mol) can be used to resolve this dilemma.
First, calculate the formula mass, the molar mass of the formula unit, which is the sum of the atomic masses of the elements in the empirical formula multiplied by their respective subscripts. For glucose,
[latex]\text {formula mass of} CH_2O = \left [ 1 \, mol C \left ( {12.011 \, g \over 1 \, mol \, C} \right ) \right ] + \left [ 2 \, mol \, H \left ({1.0079 \, g \over 1 \, mol \, H }\right )\right ] + \left [ 1 \, mole \, O \left ( {15.5994 \, mol \, O \over 1 \, mol \, O} \right ) \right ] = 30.026 g \label{3.3.5}[/latex]
This is much smaller than the observed molar mass of 180 g/mol.
Second, determine the number of formula units per mole. For glucose, calculate the number of (CH2O) units—that is, the n in (CH2O)n—by dividing the molar mass of glucose by the formula mass of CH2O:
[latex]n={180 \, g \over 30.026\, g/CH_2O} = 5.99 \approx 6 CH_2O \, \text {formula units} \label{3.3.6}[/latex]
Each glucose contains six CH2O formula units, which gives a molecular formula for glucose of (CH2O)6, which is more commonly written as C6H12O6. The molecular structures of formaldehyde and glucose, both of which have the empirical formula CH2O, are shown in Figure 7.4d:

Source: “Glucose, an example of empirical vs molecular formulas” is adapted from “Empirical and Molecular Formulas” In Chemistry 101A (LibreTexts), licensed under CC BY-NC-SA 4.0.
Scientists in Action: Ellen Henrietta Swallow Richards, PhD.

Ellen Henrietta Swallow Richards spent much of her career studying the quantity of substances within compounds whether it was in water, metals or food. She was able to determine the amount of nickel in various ores and the relative quantities of substances in food and water. Her work led to educating the population on nutrition from foods, clean water standards and how to chemically analyze ores.
Read more about Ellen’s history and contributions to chemistry provided by the American Society of Civil Engineers [New Tab].
Key Equations
- [latex]\%\text{X} = \frac{\text{mass X}}{\text{mass commpound}} \times 100\%[/latex]
- [latex]\frac{\text{molecular or molar mass ( amu or} \;\frac{\text{g}}{\text{mol}})}{\text{empirical formula mass ( amu or} \;\frac{\text{g}}{\text{mol}})} = n \;\text{formula units/molecule}[/latex]
- (AxBy)n = AnxBny
Attribution & References
Except where otherwise noted, this page is adapted by Adrienne Richards and Samantha Sullivan Sauer from “6.2 Determining Empirical and Molecular Formulas” In General Chemistry 1 & 2 by Rice University, a derivative of Chemistry (Open Stax) by Paul Flowers, Klaus Theopold, Richard Langley & William R. Robinson and is licensed under CC BY 4.0. Access for free at Chemistry (OpenStax)
- The Section: “Glucose, an example of empirical vs molecular formulas” is adapted from “Empirical and Molecular Formulas” In Chemistry 101A (LibreTexts), licensed under CC BY-NC-SA 4.0.
simplest or most reduced ratio of elements in a compound
true formula for a compound; lists how many atoms of each element are in the compound
sum of average atomic masses for all atoms represented in an empirical formula

