7.7 – Reaction Mechanisms

Unimolecular Elementary Reactions

The molecularity of an elementary reaction is the number of reactant species (atoms, molecules, or ions). For example, a unimolecular reaction involves the rearrangement of a single reactant species to produce one or more molecules of product:

A → products

The rate equation for a unimolecular reaction is:

rate = k[A]

A unimolecular reaction may be one of several elementary reactions in a complex mechanism. For example, the reaction:

O₃ → O₂ + O

illustrates a unimolecular elementary reaction that occurs as one part of a two-step reaction mechanism. However, some unimolecular reactions may have only a single reaction in the reaction mechanism. (In other words, an elementary reaction can also be an overall reaction in some cases.) For example, the gas-phase decomposition of cyclobutane, C₄H₈, to ethylene, C₂H₄, occurs via a unimolecular, single-step mechanism:

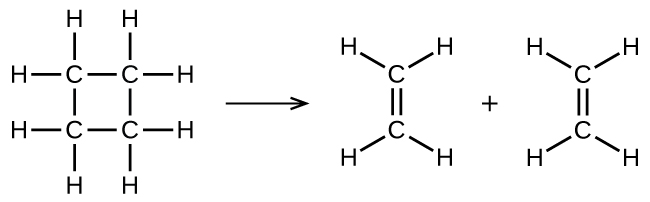
For these unimolecular reactions to occur, all that is required is the separation of parts of single reactant molecules into products.

Chemical bonds do not simply fall apart during chemical reactions. Energy is required to break chemical bonds. The activation energy for the decomposition of C₄H₈, for example, is 261 kJ per mole. This means that it requires 261 kilojoules to distort one mole of these molecules into activated complexes that decompose into products:

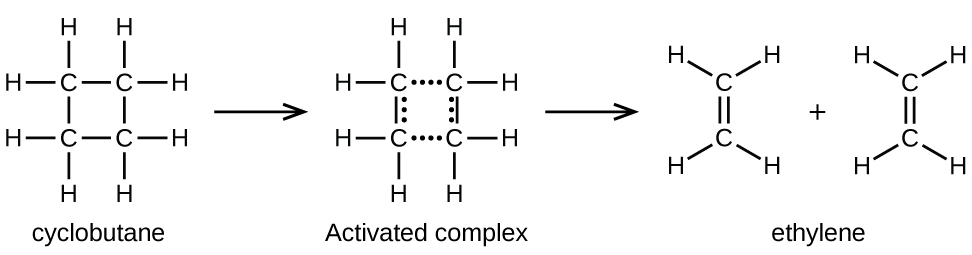
In a sample of C₄H₈, a few of the rapidly moving C₄H₈ molecules collide with other rapidly moving molecules and pick up additional energy. When the C₄H₈ molecules gain enough energy, they can transform into an activated complex, and the formation of ethylene molecules can occur. In effect, a particularly energetic collision knocks a C₄H₈ molecule into the geometry of the activated complex. However, only a small fraction of gas molecules travel at sufficiently high speeds with large enough kinetic energies to accomplish this. Hence, at any given moment, only a few molecules pick up enough energy from collisions to react.

The rate of decomposition of C₄H₈ is directly proportional to its concentration. Doubling the concentration of C₄H₈ in a sample gives twice as many molecules per liter. Although the fraction of molecules with enough energy to react remains the same, the total number of such molecules is twice as great. Consequently, there is twice as much C₄H₈ per liter, and the reaction rate is twice as fast:

rate = -Δ[C₄H₈]Δt = k[C₄H₈]

A similar relationship applies to any unimolecular elementary reaction; the reaction rate is directly proportional to the concentration of the reactant, and the reaction exhibits first-order behavior. The proportionality constant is the rate constant for the particular unimolecular reaction.

Bimolecular Elementary Reactions

The collision and combination of two molecules or atoms to form an activated complex in an elementary reaction is called a bimolecular reaction. There are two types of bimolecular elementary reactions:

A + B  →  products

and

2A  →  products

For the first type, in which the two reactant molecules are different, the rate law is first-order in A and first order in B:

rate = k[A][B]

For the second type, in which two identical molecules collide and react, the rate law is second order in A:

rate = k[A][A] = k[A]²

Some chemical reactions have mechanisms that consist of a single bimolecular elementary reaction. One example is the reaction of nitrogen dioxide with carbon monoxide:

NO₂ (g) + CO (g) → NO (g) + CO₂ (g)

Another is the decomposition of two hydrogen iodide molecules to produce hydrogen, H₂, and iodine, I₂ Figure 7.7.1.:

2HI (g) → H₂ (g) + I₂ (g)

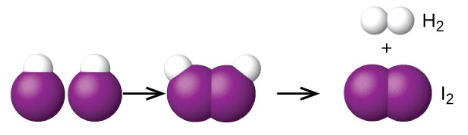
Two HI molecules – Transition state – Hydrogen iodide molecules decompose to produce hydrogen H₂ and iodine I₂.

Figure 7.7.1. The probable mechanism for the dissociation of two HI molecules to produce one molecule of H₂ and one molecule of I₂.

Bimolecular elementary reactions may also be involved as steps in a multistep reaction mechanism. The reaction of atomic oxygen with ozone is one example:

O (g) + O₃ (g)  →  2O₂ (g)

Termolecular Elementary Reactions

An elementary termolecular reaction involves the simultaneous collision of three atoms, molecules, or ions. Termolecular elementary reactions are uncommon because the probability of three particles colliding simultaneously is less than one one-thousandth of the probability of two particles colliding. There are, however, a few established termolecular elementary reactions. The reaction of nitric oxide with oxygen appears to involve termolecular steps:

2NO + O₂  →  2NO₂

rate = k[NO]² [O₂]

Likewise, the reaction of nitric oxide with chlorine appears to involve termolecular steps:

2NO + Cl₂  →  2NOCl

rate = k[NO]² [Cl₂]

Relating Reaction Mechanisms to Rate Laws

It’s often the case that one step in a multistep reaction mechanism is significantly slower than the others. Because a reaction cannot proceed faster than its slowest step, this step will limit the rate at which the overall reaction occurs. The slowest step is therefore called the rate-limiting step (or rate-determining step) of the reaction Figure 7.7.2.

Figure 7.7.2. A cattle chute is a non chemical example of a rate-determining step. Cattle can only be moved from one holding pen to another as quickly as one animal can make its way through the chute. (credit: Loren Kerns)

As described earlier, rate laws may be derived directly from the chemical equations for elementary reactions. This is not the case, however, for ordinary chemical reactions. The balanced equations most often encountered represent the overall change for some chemical system, and very often this is the result of some multistep reaction mechanisms. In every case, we must determine the overall rate law from experimental data and deduce the mechanism from the rate law (and sometimes from other data). The reaction of NO₂ and CO provides an illustrative example:

NO₂ (g) + CO (g) → CO₂ (g) + NO (g)

For temperatures above 225 °C, the rate law has been found to be:

rate = k[NO₂][CO]

The reaction is first order with respect to NO₂ and first-order with respect to CO. This is consistent with a single-step bimolecular mechanism and it is possible that this is the mechanism for this reaction at high temperatures.

At temperatures below 225 °C, the reaction is described by a rate law that is second order with respect to NO₂:

rate = k[NO₂]²

This is consistent with a mechanism that involves the following two elementary reactions, the first of which is slower and is therefore the rate-determining step:

NO₂ (g) + NO₂ (g)  →  NO₃ (g) + NO (g) (slow)

NO₃ (g) + CO (g)  →  NO₂ (g) + CO₂ (g) (fast)

The rate-determining step gives a rate law showing second-order dependence on the NO₂ concentration, and the sum of the two equations gives the net overall reaction.

In general, when the rate-determining (slower) step is the first step in a mechanism, the rate law for the overall reaction is the same as the rate law for this step. However, when the rate-determining step is preceded by a step involving an equilibrium reaction, the rate law for the overall reaction may be more difficult to derive. In order to solve this type of rate equation, the method used is called pre-equilibrium.

An elementary reaction is at equilibrium when it proceeds in both the forward and reverse directions at equal rates. Consider the dimerization of NO to N₂O₂, with k₁ used to represent the rate constant of the forward reaction and k_-1 used to represent the rate constant of the reverse reaction:

NO + NO  ⇌  N₂O₂

rate_forward = rate_reverse

k₁[NO]² = k_-1 [N₂O₂]

If N₂O₂ was an intermediate in a mechanism, this expression could be rearranged to represent the concentration of N₂O₂ in the overall rate law expression using algebraic manipulation:

However, once again, intermediates cannot be listed as part of the overall rate law expression, though they can be included in an individual elementary reaction of a mechanism. The following example will illustrate how to derive overall rate laws from mechanisms involving equilibrium steps preceding the rate-determining step.

Example 7.7.2 (using pre-equilibrium) – Deriving the Overall Rate Law Expression for a Multistep Reaction Mechanism

Nitryl chloride (NO₂Cl) decomposes to nitrogen dioxide (NO₂) and chlorine gas (Cl₂) according to the following mechanism:

2NO₂Cl (g)  ⇌  ClO₂ (g) + N₂O (g) + ClO (g) (fast, k₁ represents the rate constant for the forward reaction and k₋₁ the rate constant for the reverse reaction)

N₂O (g) + ClO₂ (g)  ⇌  NO₂ (g) + NOCl (g)(fast, k₂ for the forward reaction, k₋₂ for the reverse reaction)

NOCl + ClO  →  NO₂ + Cl₂ (slow, k₃ the rate constant for the forward reaction)

Determine the overall reaction, write the rate law expression for each elementary reaction, identify any intermediates, and determine the overall rate law expression.

Solution

For the overall reaction, simply sum the three steps, cancel intermediates, and combine like formulas:

2NO₂Cl (g) → 2NO₂ (g) + Cl₂ (g)

Next, write the rate law expression for each elementary reaction. Remember that for elementary reactions that are part of a mechanism, the rate law expression can be derived directly from the stoichiometry:

k₁[NO₂Cl]₂ = k_-1 [ClO₂][N₂O][ClO]

k₂[N₂O][ClO₂] = k_-2[NO₂][NOCl]

Rate = k₃ [NOCl][ClO]

The third step, which is the slow step, is the rate-determining step. Therefore, the overall rate law expression could be written as Rate = k₃ [NOCl][ClO]. However, both NOCl and ClO are intermediates. Algebraic expressions must be used to represent [NOCl] and [ClO] such that no intermediates remain in the overall rate law expression.

Using elementary reaction 1,

[ClO] = k₁[NO₂Cl]² k_-1[ClO₂][N₂O]

Using elementary reaction 2,

[NOCl] = k₂[N₂O][ClO₂]k_-2[NO₂]

Now substitute these algebraic expressions into the overall rate law expression and simplify:

rate = k₃ (k₂[N₂O][ClO₂]k_-2[NO₂]) (k₁[NO₂Cl]²k_-1[ClO₂][N₂O])

rate = k₁k₂k₃[NO₂Cl]2k = 2k_-1[NO₂]

Notice that this rate law shows an inverse dependence on the concentration of one of the product species, consistent with the presence of an equilibrium step in the reaction mechanism.

Check Your Learning 7.7.1 (using the steady-state approximation) – Deriving the Overall Rate Law Expression for a Multistep Reaction Mechanism

Atomic chlorine in the atmosphere reacts with ozone in the following pair of elementary reactions:

Cl + O₃ (g)  →  ClO (g) + O₂ (g) (rate constant k₁)

ClO (g) + O  →  Cl (g) + O₂ (g) (rate constant k₂)

Determine the overall reaction, write the rate law expression for each elementary reaction, identify any intermediates, and determine the overall rate law expression.

Answer

overall reaction: O₃ (g) + O → 2O₂ (g)

rate₁ = k₁[O₃][Cl]; rate₂ = k₂[ClO][O]

intermediate: ClO (g)

overall rate = k₂k₁[O₃][Cl][O]

Questions

★★ Questions

In an alternative mechanism for the reaction of NO₂ with CO, N₂O₄ appears as an intermediate. Write the rate law for each elementary reaction. Is this mechanism consistent with the experimentally determined rate law (rate = k[NO₂]²)?

Iodine monochloride (ICl) reacts with H2 as follows. 2ICl (l) + H₂ (g)  →  2HCl (g) + I₂ (s). The experimentally determined rate law is rate = k[ICl][H₂]. Write a two-step mechanism for this reaction using only bimolecular elementary reactions and show that it is consistent with the experimental rate law. (Hint: HI is an intermediate.)

Assume the reaction between NO and H₂ occurs via a three-step process. Write the rate law for each elementary reaction, write the balanced chemical equation for the overall reaction, and identify the rate-determining step. Is the rate law for the rate-determining step consistent with the experimentally derived rate law for the overall reaction: rate = k[NO]²[H₂]?

Answers

The rate law for step 1 is rate = k₁[NO₂]²; for step 2, it is rate = k₂[N₂O₄][CO]. If step 1 is slow (and therefore the rate-determining step), then the overall rate law for the reaction will be the same: rate = k₁[NO₂]². This is the same as the experimentally determined rate law. Hence this mechanism, with N₂O₄ as an intermediate, and the one described previously, with NO₃ as an intermediate, are kinetically indistinguishable. In this case, further experiments are needed to distinguish between them. For example, the researcher could try to detect the proposed intermediates, NO₃ and N₂O₄, directly.

This mechanism is consistent with the experimental rate law if the first step is the rate-determining step.

Rate determining step = #2. Yes, because the rate of formation of [N₂O₂] =k₁[NO]². Substituting k₁[NO]² for [N₂O₂] in the rate law for step 2 gives the experimentally derived rate law for the overall chemical reaction, where k = k₁k₂.

•Step 1: rate = k₁[NO]²

•Step 2: rate = k₂[N₂O₂][H₂]

•Step 3: rate = k₂[N₂O₂][H₂]

The overall reaction is then

2NO (g) + 2H₂ (g) → N₂ (g) + 2H₂O (g)