1.5 – Redox Reactions

Earth’s atmosphere contains about 20% molecular oxygen, O₂, a chemically reactive gas that plays an essential role in the metabolism of aerobic organisms and in many environmental processes that shape the world. The term oxidation was originally used to describe chemical reactions involving O₂, a term which you may be familiar with within the context of real-life scenarios and applications like the browning of some fruits and the implication of antioxidants. However, in the sciences, its meaning has evolved to refer to a broad and important reaction class known as oxidation-reduction (redox) reactions. A few examples of such reactions will be used to develop a clear picture of this classification, and we’ll use the stoichiometry skills you’ve learned throughout this chapter to balance redox reactions and solve amounts/concentrations of reactants/products.

Oxidation-Reduction (Redox) Reactions

Some redox reactions involve the transfer of electrons between reactant species to yield ionic products, such as the reaction between sodium and chlorine to yield sodium chloride:

2 Na (s) + Cl₂ (g)  →  2 NaCl (s)

It is helpful to view the process with regard to each individual reactant, that is, to represent the fate of each reactant in the form of an equation called a half-reaction:

2 Na (s)  →  2 Na⁺ (s) + 2 e^–

Cl₂ (g) + 2 e^–  →  2 Cl^– (s)

These equations show that Na atoms lose electrons while Cl atoms (in the Cl₂ molecule) gain electrons, recall the “s”  indicates that the resulting ions are present in the form of a solid ionic compound. For redox reactions of this sort, the loss and gain of electrons define the complementary processes that occur:

Oxidation = loss of electrons

Reduction = gain of electrons

Table 1.5.1. Tips & tricks – Oxidation & reduction memory aids.

Here’s are two acronyms you can easily use to remember the difference between oxidation and reduction: 1) OIL RIG OIL = Oxidation Is Loss (of electrons) RIG = Reduction Is Gain (of electrons) 2) LEO says GER LEO (zodiac sign for the lion) = Lose Electrons – Oxidation GER (like a lion growl – *grrr*) = Gain Electrons – Reduction

In this reaction, then, sodium undergoes oxidation and chlorine undergoes reduction. Viewed from a more active perspective, sodium functions as a reducing agent (reductant), since it provides electrons to (or reduces) chlorine. Likewise, chlorine functions as an oxidizing agent (oxidant), as it effectively removes electrons from (oxidizes) sodium.

Reducing agent = species that is oxidized

Oxidizing agent = species that is reduced

Hence, given that the electrons are transferred from one reactant to another, it’s important to remember that if something has been oxidized (it lost electrons), then something else has been reduced (gained those electrons).

Some redox processes, however, do not involve the transfer of electrons. Consider, for example, a reaction similar to the one yielding HCl:

H₂ (g) + Cl₂ (g)  →  2 HCl (g)

The product of this reaction is a covalent compound, so the transfer of electrons in the explicit sense is not involved. To clarify the similarity of this reaction to the previous one and permit an unambiguous definition of redox reactions, a property called oxidation number has been defined. The oxidation number (or oxidation state) of an element in a compound is the charge its atoms would possess if the compound was ionic. The following guidelines are used to assign oxidation numbers to each element in a molecule or ion:

i) The oxidation number of an atom in its elemental form is zero (e.g. O₂, Cl₂, Na).

ii) The oxidation number of a monatomic ion is equal to the ion’s charge (e.g. +1 for Na⁺, -2 for O^2-).

iii) The sum of oxidation numbers for all atoms in a molecule or polyatomic ion equals the charge on the molecule or ion.

Oxidation numbers for common nonmetals are usually assigned as follows:

A) Hydrogen: +1 when combined with nonmetals (e.g. H₂O), −1 when combined with metals and boron

B) Oxygen: −2 in most compounds (e.g. H₂O), sometimes −1 (so-called peroxides, O₂²⁻), very rarely −1/2 (so-called superoxides, O₂⁻), might be +2 or -1 when coupled to a more electronegative centre (such as F) or a group 1 or group 2 metal

C) Halogens: −1 for F always (e.g. HF), −1 for other halogens when combined with metals, nonmetals (except O), and other halogens lower in the group

Note: The proper convention for reporting charge is to write the number first, followed by the sign (e.g., 2+), while oxidation number is written with the reversed sequence, sign followed by number (e.g., +2). This convention aims to emphasize the distinction between these two related properties.

A few tips to keep in mind as you solve problems involving determining oxidation numbers in compounds: 1) if two rules appear to contradict each other, follow the rule that appears higher on the list; 2) for a multi-atom species, figure out the easy oxidation states first, then solve for the other unknown atoms.

 

Example 1.5.1 – Assigning Oxidation Numbers

Follow the guidelines in this section of the text to assign oxidation numbers to all the elements in the following species:

(a) H₂S

(b) SO₃^2-

(c) Na₂SO₄

Solution

(a) According to guideline A above, the oxidation number for H is +1.

Using this oxidation number and the compound’s formula, guideline iii may then be used to calculate the oxidation number for sulfur, represented by x:

Charge on H₂S = 0 = (2 + 1) + (1 × x)

x = 0 – (2 × (+1)) = -2

(b) Guideline B suggests the oxidation number for oxygen is −2.

Using this oxidation number and the ion’s formula, guideline iii may then be used to calculate the oxidation number for sulfur:

Charge on SO₃^2- = -2 = (3 × (-2)) + (1 × x)

x = -2 – (3 × (-2)) = +4

(c) For ionic compounds, it’s convenient to assign oxidation numbers for the cation and anion separately.

According to guideline ii, the oxidation number for sodium is +1.

Assuming the usual oxidation number for oxygen (−2 per guideline B), the oxidation number for sulfur is calculated as directed by guideline iii:

charge on SO₄^2- = -2 = (4 × (-2)) + (1 × x)

x = -2 – (4 × (-2)) = +6

Check Your Learning 1.5.1 – Assigning Oxidation Numbers

Assign oxidation states to the elements whose atoms are underlined in each of the following compounds or ions:

(a) KNO₃

(b) AlH₃

(c) NH₄⁺

(d) H₂PO₄⁻

Answer

(a) N, +5; (b) Al, +3; (c) N, −3; (d) P, +5

Using the oxidation number concept, an all-inclusive definition of redox reaction has been established. Oxidation-reduction (redox) reactions are those in which one or more elements involved undergo a change in oxidation number. (While the vast majority of redox reactions involve changes in oxidation number for two or more elements, a few interesting exceptions to this rule do exist – see Example 1.5.2 “Describing Redox Reactions”.) Definitions for the complementary processes of this reaction class are correspondingly revised as shown here:

Oxidation = increase in oxidation number

Reduction = decrease in oxidation number

Returning to the reactions used to introduce this topic, they may now both be identified as redox processes. In the reaction between sodium and chlorine to yield sodium chloride, sodium is oxidized (its oxidation number increases from 0 in Na to +1 in NaCl) and chlorine is reduced (its oxidation number decreases from 0 in Cl₂ to −1 in NaCl). In the reaction between molecular hydrogen and chlorine, hydrogen is oxidized (its oxidation number increases from 0 in H₂ to +1 in HCl) and chlorine is reduced (its oxidation number decreases from 0 in Cl₂ to −1 in HCl).

Types of Redox Reactions – Combustion

Several subclasses of redox reactions are recognized, including combustion reactions in which the reductant (also called a fuel) and oxidant (often, but not necessarily, molecular oxygen) react vigorously and produce significant amounts of heat, and often light, in the form of a flame. Solid rocket-fuel reactions (Figure 1.5.1) are combustion processes. A typical propellant reaction in which solid aluminum is oxidized by ammonium perchlorate is represented by this equation:

10 Al (s) + 6 NH₄ClO₄ (s)  →  4 Al₂O₃ (s) + 2 AlCl₃ (s) + 12 H₂O (g) + 3 N₂ (g)

Figure 1.5.1. Many modern rocket fuels are solid mixtures of substances combined in carefully measured amounts and ignited to yield a thrust-generating chemical reaction. (credit: modification of work by NASA)

Check out this brief video showing the test-firing of a small-scale, prototype, hybrid rocket engine planned for use in the new Space Launch System being developed by NASA. The first engines firing at 3 s (green flame) use a liquid fuel/oxidant mixture, and the second, more powerful engines firing at 4 s (yellow flame) use a solid mixture.

Types of Redox Reactions – Single-displacement (Replacement)

Single-displacement (replacement) reactions are redox reactions in which an ion in solution is displaced (or replaced) via the oxidation of a metallic element. One common example of this type of reaction is the acid oxidation of certain metals:

Zn (s) + 2 HCl (aq)  →  ZnCl₂ (aq) + H₂ (g)

Metallic elements may also be oxidized by solutions of other metal salts; for example:

Cu (s) + 2 AgNO₃ (aq)  →  Cu(NO₃)₂ (aq) + 2 Ag (s)

This reaction may be observed by placing copper wire in a solution containing a dissolved silver salt. Silver ions in solution are reduced to elemental silver at the surface of the copper wire, and the resulting Cu²⁺ ions dissolve in the solution to yield a characteristic blue colour (Figure 1.5.2).

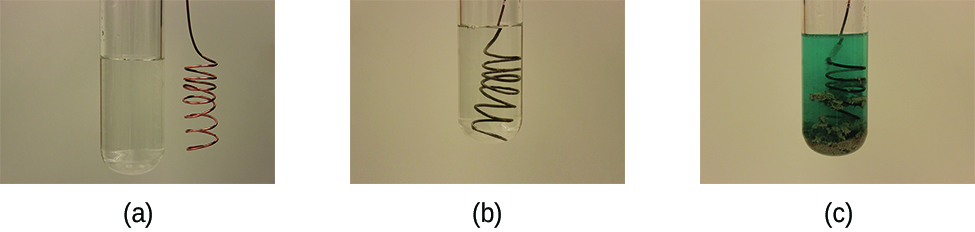
Figure 1.5.2. (a) A copper wire is shown next to a solution containing silver(I) ions. (b) Displacement of dissolved silver ions by copper ions results in (c) accumulation of gray-coloured silver metal on the wire and development of a blue colour in the solution, due to dissolved copper ions. (credit: modification of work by Mark Ott)

Example 1.5.2 – Describing Redox Reactions

Identify which equations represent redox reactions, providing a name for the reaction if appropriate. For those reactions identified as redox, name the oxidant and reductant.

(a) ZnCO₃ (s)  →  ZnO (s) + CO₂ (g)

(b) 2 Ga (l) + 3 Br₂ (l)  →  2 GaBr₃ (s)

(c) 2 H₂O₂ (aq)  →  2 H₂O (l) + O₂ (g)

(d) BaCl₂ (aq) + K₂SO₄ (aq)  →  BaSO₄ (s) + 2 KCl (aq)

(e) C₂H₄ (g) + 3 O₂ (g)  →  2 CO₂ (g) + 2 H₂O (l) 

Solution

Redox reactions are identified per definition if one or more elements undergo a change in oxidation number.

(a) This is not a redox reaction, since oxidation numbers remain unchanged for all elements.

(b) This is a redox reaction. Gallium is oxidized, its oxidation number increasing from 0 in Ga (l) to +3 in GaBr₃ (s). The reducing agent is Ga (l). Bromine is reduced, its oxidation number decreasing from 0 in Br₂ (l) to −1 in GaBr₃ (s). The oxidizing agent is Br₂ (l).

(c) This is a redox reaction. It is a particularly interesting process, as it involves the same element, oxygen, undergoing both oxidation and reduction (a so-called disproportionation reaction). Oxygen is oxidized, its oxidation number increasing from −1 in H₂O₂ (aq) to 0 in O₂ (g). Oxygen is also reduced, its oxidation number decreasing from −1 in H₂O₂ (aq) to −2 in H₂O (l). For disproportionation reactions, the same substance functions as an oxidant and a reductant.

(d) This is not a redox reaction since oxidation numbers remain unchanged for all elements.

(e) This is a redox reaction (combustion). Carbon is oxidized, its oxidation number increasing from −2 in C₂H₄ (g) to +4 in CO₂ (g). The reducing agent (fuel) is C₂H₄ (g). Oxygen is reduced, its oxidation number decreasing from 0 in O₂ (g) to −2 in H₂O (l). The oxidizing agent is O₂ (g).

Check Your Learning 1.5.2 – Describing Redox Reactions

This equation describes the production of tin (II) chloride:

Sn (s) + 2 HCl (g)  →  SnCl₂ (s) + H₂ (g)

Is this a redox reaction? If so, provide a more specific name for the reaction if appropriate, and identify the oxidant and reductant.

Answer

Yes, a single-replacement reaction. Sn (s) is the reductant, HCl (g) is the

oxidant.

Balancing Redox Reactions – The Half-Reaction Method

The redox reactions discussed so far tended to be rather simple, and conservation of mass (atom counting by type) and deriving a correctly balanced chemical equation was relatively simple. Balancing other reactions becomes more complicated when they take place in aqueous media that often involves water, hydronium ions, and hydroxide ions as reactants or products. Although these species are not oxidized or reduced, they do participate in chemical change in other ways (e.g., by providing the elements required to form oxyanions). Equations representing these reactions are sometimes very difficult to balance by inspection, so systematic approaches have been developed to assist in the process. One very useful approach is to use the half-reaction method, which splits oxidation-reduction reactions into their oxidation “half” and reduction “half” to make finding the overall equation easier. This method involves the following general steps:

1. Write out the net ionic form of the reaction (see the preceding section “Solution Stoichiometry” to refresh your memory on expressing net ionic equations).

2. Separate this equation into its two half-reactions representing the redox process.

3. Balance all elements except oxygen and hydrogen.

4. Balance oxygen atoms by adding H₂O molecules.

5. Balance hydrogen atoms by adding H⁺ ions.

6. Balance the net charge on each side by adding electrons.

7. If necessary, multiply each half-reaction’s coefficients by the smallest possible integers to yield equal numbers of electrons in each.

8. Add the balanced half-reactions together and simplify by removing species that appear on both sides of the equation.

9. Verify your final reaction (check that the number of atoms and the total charges are balanced).

Redox reactions frequently occur in solutions, which could be acidic, basic, or neutral. When balancing oxidation-reduction reactions, the nature of the solution may be important:

Acidic solution: follow all steps 1-9 as normal.

Basic solution: follow all steps 1-9 as normal, add two additional “steps” to step 5 :

• Add OH⁻ ions to both sides of the equation in numbers equal to the number of H⁺ ions. A common mistake students make is that they add OH^– to only one side of the equation, but just like in math equations, if you add one element to one side, you must add it to the other side as well (hence, add the same number of OH^– ions to both sides!).
• On the side of the equation containing both H⁺ and OH⁻ ions, combine these ions to yield water molecules.

Example 1.5.3 – Balancing Redox Reactions in Acidic Solution 

Write a balanced equation for the reaction between dichromate ion and iron (II) to yield iron(III) and chromium(III) in an acidic solution.

Cr₂O₇^2- + Fe²⁺  →  Cr³⁺ + Fe³⁺

Solution

1. Write the two half-reactions. Each half-reaction will contain one reactant

and one product with one element in common.

Fe²⁺  →  Fe³⁺

Cr₂O₇^2–  →  Cr³⁺

2. Balance all elements except oxygen and hydrogen. The iron half-reaction is already balanced, but the chromium half-reaction shows two Cr atoms on the left and one Cr atom on the right. Changing the coefficient on the right side of the equation to 2 achieves balance with regard to Cr atoms.

Fe²⁺  →  Fe³⁺

Cr₂O₇^2–  →  2 Cr³⁺

3. Balance oxygen atoms by adding H₂O molecules. The iron half-reaction does not contain O atoms. The chromium half-reaction shows seven O atoms on the left and none on the right, so seven water molecules are added to the right side.

Fe²⁺  →  Fe³⁺

Cr₂O₇^2–  →  2 Cr³⁺ + 7 H₂O

4. Balance hydrogen atoms by adding H⁺ ions. The iron half-reaction does not contain H atoms. The chromium half-reaction shows 14 H atoms on the right and none on the left, so 14 hydrogen ions are added to the left side.

Fe²⁺  →  Fe³⁺

Cr₂O₇^2- + 14 H⁺  →  2 Cr³⁺ + 7 H₂O

5. Balance charge by adding electrons. The iron half-reaction shows a total charge of 2+ on the left side (1 Fe²⁺ ion) and 3+ on the right side (1 Fe³⁺ ion). Adding one electron to the right side brings that side’s total charge to (3+) + (1−) = 2+, and charge balance is achieved. The chromium half-reaction shows a total charge of (1 × 2−) + (14 × 1+) = 12+ on the left side (1 Cr₂O₇²⁻ ion and 14 H⁺ ions). The total charge on the right side is (2 × 3+) = 6 + (2 Cr³⁺ ions). Adding six electrons to the left side will bring that side’s total charge to ((12+) + (6−)) = 6+, and charge balance is achieved.

Fe²⁺  →  Fe³⁺ + e^–

Cr₂O₇^2- + 14 H⁺ + 6 e^–  →  2 Cr³⁺ + 7 H₂O

Note: At this point, make sure you always check that one of your equations has the electron(s) on the reactants side, while the other equation has the electron(s) on the products side. If this isn’t the case, it’s a clear sign that you’ve done something wrong – go back and check your work to find the error(s).

6. Multiply the two half-reactions so the number of electrons in one reaction equals the number of electrons in the other reaction. To be consistent with mass conservation, and the idea that redox reactions involve the transfer (not creation or destruction) of electrons, the iron half-reaction’s coefficient must be multiplied by 6.

6 Fe²⁺  →  6 Fe³⁺ + 6 e^–

Cr₂O₇^2- + 14 H⁺ + 6 e^–  →  2 Cr³⁺ + 7 H₂O

A good way to qualitatively check your work when balancing both half-reactions is by recalling your understanding of oxidation and reduction. For the first reaction, 6 Fe²⁺ → 6 Fe³⁺ + 6 e^–, the oxidation state of iron goes up from +2 and +3, and there are electrons being lost in the products side, so it’s the oxidation half-reaction. For the second reaction, the oxidation state of chromium goes down from +6 to +3, and electrons are gained in the reactants side, so it’s the reduction half-reaction.

7. Add the balanced half-reactions and cancel species that appear on both sides of the equation.

6 Fe²⁺ + Cr₂O₇^2- + 14 H⁺ + 6 e^–  →  6 Fe³⁺ + 6 e^– + 2 Cr³⁺ + 7 H₂O

Only the six electrons are redundant species. Removing them from each side of the equation yields the simplified, balanced equation here:

6 Fe²⁺ + Cr₂O₇^2- + 14 H⁺  →  6 Fe³⁺ + 2 Cr³⁺ + 7 H₂O

A final check of atom and charge balance confirms the equation is balanced.

Element	Reactants	Products
Fe	6	6
Cr	2	2
O	7	7
H	14	14
Charge	+24	+24

Check Your Learning 1.5.3 – Balancing Redox Reactions in Acidic Solution 

In acidic solution, hydrogen peroxide reacts with Fe²⁺ to produce Fe³⁺ and H₂O. Write a balanced equation for this reaction.

Answer

H₂O₂ (aq) + 2 H⁺ (aq) + 2 Fe²⁺  →  2 H₂O (l) + 2 Fe³⁺

Example 1.5.4 – Balancing Redox Reactions in Basic Solution

Balance the following reaction equation in basic solution:

MnO₄^– (aq) + Cr(OH)₃ (s)  →  MnO₂ (s) + CrO₄^2- (aq)

Solution

This is an oxidation-reduction reaction, so start by collecting the species given into an unbalanced oxidation half-reaction and an unbalanced reduction half-reaction:

Oxidation (unbalanced): Cr(OH)₃ (s)  →  CrO₄^2- (aq)

Reduction (unbalanced): MnO₄^– (aq)  →  MnO₂ (s)

Starting with the oxidation half-reaction, we can balance the chromium:

Oxidation (unbalanced): Cr(OH)₃ (s)  →  CrO₄^2- (aq)

In acidic solution, we can use or generate hydrogen ions (H⁺). Adding one water molecule to the left side provides the necessary oxygen; the “leftover” hydrogen appears as five H⁺ on the right side:

Oxidation (unbalanced): Cr(OH)₃ (s) + H₂O (l)  →  CrO₄^2- (aq) + 5 H⁺ (aq)

The left side of the equation has a total charge of [0], and the right side a total charge of [−2 + 5 × (+1) = +3]. The difference is three, adding three electrons to the right side produces a mass- and charge-balanced oxidation half-reaction (in acidic solution):

Oxidation (balanced): Cr(OH)₃ (s) + H₂O (l)  →  CrO₄^2- (aq) + 5 H⁺ (aq) + 3 e^–

Checking the half-reaction:

  Cr: Does (1 x 1) = (1 x 1)? Yes.

  H: Does (1 x 3 + 1 x 2) = (5 x 1)? Yes.

  O: Does (1 x 3 + 1 x 1) = (4 x 1)? Yes.

  Charge: Does [0] = [1 x (-2) +5 x (+1) + 3 x (-1)]? Yes.

Now work on the reduction. It is necessary to convert the four O atoms in the MnO₄⁻ minus the two O atoms in MnO₂ into two water molecules. To do this, add four H⁺ to convert the oxygen into two water molecules:

Reduction (unbalanced): MnO₄^– (aq) + 4 H⁺ (aq)  →  MnO₂ (s) + 2 H₂O (l)

Then add three electrons to the left side to balance the charge:

Reduction (balanced): MnO₄^– (aq) + 4 H⁺ (aq) + 3 e^–  →  MnO₂ (s) + 2 H₂O (l)

Make sure to check the half-reaction:

Mn: Does (1 x 1) = (1 x 1)? Yes.

H: Does (4 x 1) = (2 x 2)? Yes.

O: Does (1 x 4) = (1 x 2 + 2 x 1)? Yes.

Charge: Does [1 x (-1) + 4 x (+1) + 3 x (-1)] = [0]? Yes.

Collecting what we have so far:

Oxidation (balanced): Cr(OH)₃ (s) + H₂O (l)  →  CrO₄^2- (aq) + 5 H⁺ (aq) + 3 e^–

Reduction (balanced): MnO₄^– (aq) + 4 H⁺ (aq) + 3 e^–  →  MnO₂ (s) + 2 H₂O (l)

In this case, both half-reactions involve the same number of electrons; therefore, simply add the two half-reactions together.

Cr(OH)₃ (s) + H₂O (l) + MnO₄^– (aq) + 4 H⁺ (aq) + 3 e^–  →  MnO₂ (s) + 2 H₂O (l) + CrO₄^2- (aq) + 5 H⁺ (aq) + 3 e^–

Cr(OH)₃ (s) + MnO₄^– (aq)  →  MnO₂ (s) + H₂O (l) + CrO₄^2- (aq) + H⁺ (aq)

Checking each side of the equation:

Mn: Does (1 x 1) = (1 x 1)? Yes.

Cr: Does (1 x 1) = (1 x 1)? Yes.

H: Does (1 x 3) = (2 x 1 + 1 x 1)? Yes.

O: Does (1 x 4 + 1 x 3) = (1 x 4 + 1 x 2 + 1 x 1)? Yes.

Charge: Does [1 x (-1)] = [1 x (-2) + 1 x (+1)]? Yes.

This is the balanced equation in acidic solution. For a basic solution, add one hydroxide ion to each side and simplify:

OH^– (aq) + Cr(OH)₃ (s) + H₂O (l) + MnO₄^– (aq)  →  MnO₂ (s) + H₂O (l) + CrO₄^2- (aq) + (H⁺ + OH^–) (aq)

OH^– (aq) + Cr(OH)₃(s) + H₂O (l) + MnO₄^– (aq)  →  MnO₂ (s) + 2H₂O (l) + CrO₄^2- (aq)

Checking each side of the equation:

Mn: Does (1 x 1) = (1 x 1)? Yes.

Cr: Does (1 x 1) = (1 x 1)? Yes.

H: Does (1 x 1 + 1 x 3) = (2 x 2)? Yes.

O: Does (1 x 1 +1 x 4 + 1 x 3) = (1 x 4 + 1 x 2 + 2 x 1)? Yes.

Charge: Does [1 x (-1) + 1 x (-1)] = [1 x (-2)]? Yes.

This is the balanced equation in basic solution.

Check Your Learning 1.5.4 – Balancing Redox Reactions in Basic Solution

Balance the following in the type of solution indicated.

(a) H₂ + Cu⁺  ⟶  Cu (acidic solution)

(b) H₂ + Cu(OH)₂  ⟶  Cu (basic solution)

(c) Fe + Ag⁺  ⟶  Fe²⁺ + Ag

(d) Identify the oxidizing agents in reactions (a), (b), and (c).

(e) Identify the reducing agents in reactions (a), (b), and (c).

Answer

(a) H₂ (g) + Cu²⁺ (aq)  ⟶  2 H⁺ (aq) + Cu (s);

(b) H₂ (g) + Cu(OH)₂ (s)  ⟶  2 H₂O (l) + Cu (s);

(c) Fe (s) + 2 Ag⁺ (aq)  ⟶  Fe²⁺ (aq) + 2 Ag (s);

(d) Recall oxidizing agent = species reduced: (a) Cu²⁺; (b) Cu(OH)₂; (c) Ag⁺

(e) Recall reducing agent = species oxidized: (a) H₂; (b) H₂; (c) Fe

★ Questions

1. Determine the oxidation states of the elements in the following compounds:

(a) NaI

(b) GdCl₃

(c) LiNO₃

(d) H₂Se

(e) Mg₂Si

(f) RbO₂, rubidium superoxide

(g) HF

2. Determine the oxidation states of the elements in the compounds listed. None of the oxygen-containing compounds are peroxides or superoxides.

(a)H₃PO₄

(b) Al(OH)₃

(c) SeO₂

(d) KNO₂

(e) In₂S₃

(f.) P₄O₆

3. Identify the atoms that are oxidized and reduced, the change in oxidation state for each, and the oxidizing and reducing agents in each of the following equations:

(a) Mg (s) + NiCl₂ (aq)  →  MgCl₂ (aq) + Ni (s)

(b) PCl₃ (l) + Cl₂ (g)  →  PCl₅ (s)

(c) C₂H₄ (g) + 3 O₂ (g)  →  2 CO₂ (g) + 2 H₂O (g)

(d) Zn (s) + H₂SO₄ (aq)  →  ZnSO₄ (aq) + H₂ (g)

(e) 2 K₂S₂O₃ (s) + I₂ (s)  →  K₂S₄O₆ (s) + 2 KI (s)

(f) 3 Cu (s) + 8 HNO₃ (aq)  →  3 Cu(NO₃)₂ (aq) + 2 NO (g) + 4 H₂O (l)

4. Complete and balance the following oxidation-reduction reactions, which give the highest possible oxidation state for the oxidized atoms.

(a) Al (s) + F₂ (g)  →

(b) Al (s) + CuBr₂ (aq)  → (single displacement)

(c) P₄ (s) + O₂ (g)  →

(d) Ca (s) + H₂O (l)  →  (products are a strong base and a diatomic gas)

5. When heated to 700–800 °C, diamonds, which are pure carbon, are oxidized by atmospheric oxygen. (They burn!) Write the balanced equation for this reaction.

6. The military has experimented with lasers that produce very intense light when fluorine combines explosively with hydrogen. What is the balanced equation for this reaction?

★★ Questions

7. Complete and balance each of the following half-reactions (steps 2–5 in half-reaction method):

(a) Sn⁴⁺ (aq)  →  Sn²⁺ (aq)

(b) [Ag(NH₃)₂] (aq)  →  Ag⁺ (s) + NH₃ (aq)

(c) Hg₂Cl₂ (s)  →  Hg (l) + Cl^– (aq)

(d) H₂O (l)  →  O₂ (g) (in acidic solution)

(e) IO₃^– (aq)  →  I₂ (s)

(f) SO₃^2- (aq)  →  SO₄^2- (aq) (in acidic solution)

(g) MnO₄^– (aq)  →  Mn²⁺ (aq) (in acidic solution)

(h) Cl^– (aq)  →  ClO₃^– (aq) (in basic solution)

8. Balance each of the following equations according to the half-reaction method:

(a) Sn²⁺ (aq) + Cu²⁺ (aq)  →  Sn⁴⁺ (aq) + Cu⁺ (aq)

(b) H₂S (g) + Hg₂²⁺ (aq)  →  Hg (l) + S (s)  (in acid)

(c) CN^– (aq) + ClO₂ (aq)  →  CNO^– (aq) + Cl^– (aq)  (in acid)

(d) Fe²⁺ (aq) + Ce⁴⁺ (aq)  →  Fe³⁺ (aq) + Ce³⁺ (aq)

(e) HBrO (aq)  →  Br^– (aq) + O₂ (g)  (in acid)

9. Balance each of the following equations according to the half-reaction method:

(a) MnO₄^– (aq) + NO₂^– (aq)  →  MnO₂ (s) + NO₃^– (aq)  (in base)

(b) MnO₄^2- (aq)  →  MnO₄^– (aq) + MnO₂ (s)  (in base)

(c) Br₂ (l) + SO₂ (g)  →  Br^– (aq) + SO₄^2- (aq)  (in acid)

10. Balance the following in acidic solution:

(a) H₂O₂ + Sn²⁺  →  H₂O + Sn⁴⁺

(b) PbO₂ + Hg  →  Hg₂²⁺ + Pb²⁺

(c) Al + Cr₂O₇^2–  →  Al³⁺ + Cr³⁺

11. Balance the following in basic solution:

(a) SO₃^2- (aq) + Cu(OH)₂ (s)  →  SO₄^2- (aq) + Cu(OH)

(b) O₂ (g) + Mn(OH)₂ (s)  →  MnO₂ (s)

(c) NO₃^– (aq) + H₂ (g)  →  NO (g)

(d) Al (s) + CrO₄^2- (aq)  →  Al(OH)₃ (s) + Cr(OH)₄^– (aq)

Answers

1. (a) Na +1, I -1; (b) Gd +3, Cl -1; (c) Li +1, N +5, O -3; (d) H +1, Se -2; (e) Mg +2, Si -4; (f) Rb -1, O +0.5; (g) H +1, F -1

2. (a) H +1, P +5, O −2; (b) Al +3, H +1, O −2; (c) Se +4, O −2; (d) K +1, N +3, O −2; (e) In +3, S −2; (f) P +3, O −2

3. (a) Mg: oxidized, 0 → +2, reducing agent, Ni: reduced, +2 → 0, oxidizing agent;

(b) P: oxidized, +3 → 5, reducing agent, Cl:  reduced, 0 → -1, oxidizing agent;

(c) C: oxidized, -2 → +4, reducing agent, O: reduced, 0 → -2, oxidizing agent;

(d) Zn: oxidized, 0 → +2, reducing agent, H: reduced, +1 → 0, oxidizing agent;

(e) S: oxidized, +2 → +5/2, reducing agent, I: reduced, 0 → -1, oxidizing agent;

(f) Cu: oxidized, 0 → +2, reducing agent, N: reduced, +5 → +2, oxidizing agent

4. (a) 2 Al (s) + 3 F₂ (g)  →  2 AlF₃ (s)

(b) Al (s) + CuBr₂ (aq)  →  3 Cu (s) + 2 AlBr₃ (aq)

(c) P₄ (s) + O₂ (g)  →  P₄O₁₀ (s)

(d) Ca (s) + H₂O (l)  →  Ca(OH)₂ (aq) + H₂ (g)

5. C_diamond (s) + O₂ (g)  →  CO₂ (g)

6. H₂ (g) + F₂ (g)  →  CO₂ (g)

7. (a) Sn⁴⁺ (aq) + 2e^–  →  Sn²⁺ (aq)

(b) [Ag(NH₃)₂] (aq) + e^–  →  Ag⁺ (s) + NH₃ (aq)

(c) Hg₂Cl₂ (s) + 2 e^–  →  Hg (l) + Cl^– (aq)

(d) 2 H₂O (l)  →  O₂ (g) + 4 H⁺ (aq) + 4 e^–

(e) 6 H₂O (l) + IO₃^– (aq) + 10 e^–  →  I₂ (s) + 12 OH^– (aq)

(f) H₂O (l) + SO₃^2- (aq)  →  SO₄^2- (aq) + 2 H⁺ (aq) + 2 e^–

(g) 8 H⁺ (aq) + MnO₄^– (aq) + 5 e^–  →  Mn²⁺ (aq) + 4 H₂O (l)

(h) Cl^– (aq) + 6 OH^– (aq)  →  ClO₃^– (aq) + 3 H₂O (l) + 6 e^–

8. (a) Sn²⁺ (aq) + 2 Cu²⁺ (aq)  →  Sn⁴⁺ (aq) + 2 Cu⁺ (aq)

(b) H₂S (g) + Hg₂²⁺ (aq) + 2 H₂O (l)  →  2 Hg (l) + S (s) + 2 H₃O⁺ (aq)

(c) 5 CN^– (aq) + 2 ClO₂ (aq) + 3 H₂O (l) → 5 CNO^– (aq) + 2 Cl^– (aq) + 2 H₃O⁺ (aq)

(d) Fe²⁺ (aq) + Ce⁴⁺ (aq)  →  Fe³⁺ (aq) + Ce³⁺ (aq)

(e) 2 HBrO (aq) + 2 H₂O (l)  →  2 H₃O⁺ (aq) + 2 Br^– (aq) + O₂ (g) 

9. (a) 2 MnO₄^– (aq) + 3 NO₂^– (aq) + H₂O (l)  →  2 MnO₂ (s) + 3 NO₃^– (aq) + 2 OH^– (aq)

(b) 3 MnO₄^2- (aq) + 2 H₂O (l)  →  2 MnO₄^– (aq) + MnO₂ (s) + 4 OH^– (aq)

(c) Br₂ (l) + SO₂ (g) + 2 H₂O (l)  →  2 Br^– (aq) + SO₄^2- (aq)  + 4 H⁺ (aq)

10. (a) 2 H⁺ + H₂O₂ + Sn²⁺  →  H₂O + Sn⁴⁺

(b) 4 H⁺ + PbO₂ + 2 Hg  →  Hg₂²⁺ + Pb²⁺ + 2 H₂O

(c) 2 Al + Cr₂O₇^2- + 14 H⁺  →  2 Al³⁺ + 2 Cr³⁺ + 7 H₂O

11. (a) SO₃^2- (aq) + 2 Cu(OH)₂ (s)  →  SO₄^2- (aq) + 2 Cu(OH) + H₂O

(b) O₂ (g) + 2 Mn(OH)₂ (s)  →  2 MnO₂ (s) + 2 H₂O

(c) 2 NO₃^– (aq) + 3 H₂ (g) + 2 H₂O  →  NO (g) + 2 OH^– (aq)

(d) Al (s) + CrO₄^2- (aq) + 4 H₂O  →  Al(OH)₃ (s) + Cr(OH)₄^– (aq) + OH^– (aq)