4 Gas Laws
Michael Mombourquette
4.1: Introduction
In this section, we will be exploring gas behavior. The results of experimental observation and work are expressed here as ‘laws’ and are useful models to help us predict gas behavior in the right circumstances. We will develop the Kinetic molecular theory, which gives us insight into the molecular level reasons that explain gas behaviors. Later in the unit, we will discover conditions under which the first gas laws do not predict the observed behavior. More advanced models are proposed to better describe gas behaviors under these conditions.
You will be expected to know each of the gas laws presented here, the conditions under which they work and do not work. You will also be expected to be able to use these ideas and the equations within to solve problems both here in the Gas units but also later in other units of the course.
4.2: Avogadro’s Law
According to Avogadro, equal volumes of different (ideal) gases at the same temperature and pressure contain equal numbers of molecules (moles) of the different gases. This law explained Gay-Lussac Law that noted that volumes of gases reacting are related by small whole number ratios.
For example, in the reaction B
2H2(g) + O2(g) → 2H2O(g)
2L 1L → 2L
Volume Ratio of Reactants:Products :: 3L:2L
Avogadro’s law assumes all atoms (molecules) are the same size. This assumption is only true at extremely small pressures. It’s not bad for every-day atmospheric pressures.
Standard Temperatures and Pressures STP are defined to be
0 ℃ and 100 kPa (=0.98692 atm) [1]
One mole of Gas at STP occupies 22.7 L ([2])
There are slight variances in real gases at normal conditions but not much.
Example: What is the Molar mass of a gas of which 2.641 g occupies 705.6 mL @STP?
![]()
![]()
4.3: Pressures of Gases
Pressure = force/area
Consider the following inverted tube filled with mercury such that its open end is immersed in a pool of mercury.
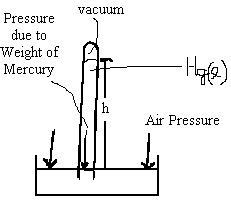 |
Diagram of inverted test tube in mercury. The column of mercury of height, h, is supported by the air pressure. |
A standard rule in fluid dynamics states that the pressure of a liquid is constant at any given height. So the pressure inside and outside the tube at the height of the surface must be equal and opposite that of the air pressure. Thus, the pressure due to the weight of the column of mercury must be equal to the pressure of the air. By measuring the height of the column we have a direct measure of the air pressure.
Note that a gas at STP (T= 0 ℃., P = 100 kPa) will support a column of mercury of height 750.0 mm. Hence, we some times take the short-cut way of expressing pressure as a height of a column of mercury that would be supported by the gas pressure being measured. At STP, the pressure of an ideal gas is 750 mmHg. [3]
Example: What is the height of a column of water which can be supported by a pressure of exactly 1 bar (=100 kPa = 750.0 mmHg) at 25 ℃?
The density of water at that temperature is 0.997 g cm-3.
![]()
![]()
where ![]() is the height of the column of liquid,
is the height of the column of liquid, ![]() is its density,
is its density, ![]() is the area of the column and
is the area of the column and ![]() is the acceleration due to gravity. We could look up the value for acceleration due to gravity and calculate
is the acceleration due to gravity. We could look up the value for acceleration due to gravity and calculate ![]() that way. However, we happen to know the height of a column of mercury that the same air pressure would support (750 mm) therefore, let’s just equate the pressure of water and the pressure of mercury as follows.
that way. However, we happen to know the height of a column of mercury that the same air pressure would support (750 mm) therefore, let’s just equate the pressure of water and the pressure of mercury as follows.
Note that 13.6 g/mL is the density of Mercury. https://www.periodic-table.org/mercury-density/.
4.4: Boyle’s Law
Boyle measured the relationship between the pressure and the volume of a given sample of gas at fixed temperature. He found that a sample of gas compresses if the external pressure applied to it increases and that the product PV is constant.
Boyle’s law, stated in mathematical terms for a gas whose pressure and volume is measured at two different pressure/volume states at a constant temperature is then,
![]()
Note: This law is only truly valid at infinitely low pressures. Standard pressures, however lead to a reasonable approximation for most gases.
Example: A sample of air occupies a volume of 450.0 mL at 20 ℃ and 1.00 bar (100 kPa). What will be the pressure of this gas if it is transferred to a 2.000 L bulb at the same temperature?
![]()
![]()
A gas that obeys both Boyle’s Law and Avogadro’s law is an Ideal gas. The atoms (molecules) of an ideal gas are infinitely small such that they never collide with each other. They never even interact with each other in any way. Thus, the atoms/molecules of an ideal gas each behaves as if they were the only atom in the container. Simple experience tells us that this is not exactly true, even at room temperature (gas-phase reactions occur). It is a reasonably good assumption for most gases at or near STP. The assumption of ideality breaks down as the pressure is increased or as the temperature is lowered. It also breaks down sooner for particularly large or massive or polar gas molecules.
4.5: Charles’ Law
The volume of any gas increases linearly with increasing temperature at constant pressure.
If we plot a graph of the volume of a sample of gas versus the temperature we get something that looks like the following:
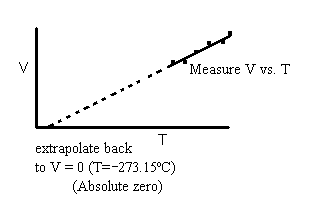 |
From the extrapolated line, we can determine the temperature at which an ideal gas would have a zero volume. Since ideal gases have infinitely small atoms the only contribution to the volume of a gas is the pressure exerted by the moving atoms bumping against the walls of the container. If no volume then there must be no kinetic energy left. Thus, absolute zero is the temperature at which all kinetic energy (motion) has been removed.
NOTE: This does not mean all energy has been removed, merely all kinetic energy. |
To make the V versus T function simpler, we should use the absolute temperature scale (Kelvin): TK = TC + 273.15.
To avoid the need to know k, we use ratios. The ratio of V to T of an ideal gas at constant pressure is constant over all temperatures. Or…
![]()
Example: A sample of gas occupies 400.0 mL at 25.00℃ and 1 bar pressure. What volume will it occupy at 200.00℃ at the same P?
![]()
![]()
4.6: Ideal Gas Law
*Note that in the video, I misstated the moles of butane as just 6.414 rather than 6.414×10-2, which is written correctly in the example worked out below.
If we take the three gas laws we’ve studied so far, we can combine them into a single law called the Ideal Gas law. This law covers the relationship between temperature, pressure, volume and number of moles of an Ideal gas.
| Avogadro’s Law | V = k1n |T,P |
| Boyle’s Law | V = k2/P |T,n |
| Charles’ Law | V = k3T |n,P |
After some consideration and algebra, we arrive at
V = koverall nT/P
where koverall turns out to be the Ideal gas constant (or universal gas constant)
R = 8.314 462 J mol-1K-1.
We’re more familiar with the equation written as:
PV = nRT
This is the Ideal Gas Law.
Example: A sample of butane (C4H10) of mass 3.728 g is placed in an evacuated bulb of volume 489 mL at 25.℃. What is its pressure?
PV = nRT
![]()
![]()
![]()
![]()
NOTE: in this case, we needed to be sure that all units were SI units. Then the pressure simply worked out to be the SI unit of pressure.
There are many kinds of calculations that involve the Ideal Gas Law. Many of them involve far more than simply substituting values into the equation and getting an answer. Let’s try one example where we use the Ideal gas law to set up the problem but we don’t actually complete a calculation using the Ideal Gas Equation.
Example: A bulb is filled with H2 gas at a temperature T. The pressure is 756 mmHg. A portion of the gas is transferred to a flask and at 100 kPa, (NOTE: 100 kPA = 750.0 mmHg) occupies 40.0 mL at the same temperature T. The pressure in the original bulb drops to 625 mmHg at temp. T. What is the volume of the bulb?
This is a many step problem and there is no way you can expect to be able to ‘know’ how to solve it at first glance. You do, however have enough knowledge to solve it now. You just have to put down what you know and see how it all fits.
PV = nRT
let:
n = total # moles of H2(g)
V = Volume of bulb
| n = n1 + n2 | (1) |
where n1 is the number of moles removed to the flask and n2 is the number of moles remaining behind.
| Initial: | 756 mmHg × V = nRT | (2) |
| Transferred: | 750 mmHg × 40.0 mL = n1RT | (3) |
| Remaining: | 625 mmHg × V = n2RT | (4) |
At this point, we have 4 equations in what seems to be 5 unknowns, n, n1, n2, V and T. However, T is a constant in this process (as well as R) and we will be able to eliminate both R and T through some simple math. If we actually needed to determine T then we would need a 5th equation. but since we are not interested in it’s value, we only need 4 equations, so long as we can eliminate it some way, which we can.
Now substitute (1) into (2)
756mmHg × V = (n1 + n2)RT = n1 RT + n2 RT
Now sub in (3) and (4) (thus eliminating RT from the picture)
756mmHg × V = 750mmHg × 40.0mL + 625mmHg × V
Cancel out the units mmHg[4] and gather the known terms to the right-hand side.
![]()
4.7: Dalton’s Law of Partial Pressures
* I wrote .938 instead of .983 in the KClO2 example but the math is all correct.
If more than one gas occupy a single container then the number of moles of each gas is proportional to the pressure of each gas (the gas’ partial pressure) and the total pressure is equal to the sum of all the partial pressures. In like manner, the total number of moles of gas is equal to the sum of the numbers of moles of the individual gases.
This actually follows from the Ideal Gas law PV = nRT .
For Dalton’s Law to work all gases in the mixture must behave like ideal gases under the conditions studied.
![]()
and
![]()
Dalton’s Law says the total pressure is simply the sum of the individual gas pressures (a.k.a. partial pressures) so we substitute for P1 and P2.
![]()
This allows us to make a few quick calculations in our head. Useful in certain gas-law type problems. Let’s take, for example, the atmosphere.
The air is made up of
78.08 % N2 (mole %)
20.95 % O2
0.934% Ar + …
This quickly translates into (for a 100 mole sample of air)
78.08 mol N2
20.95 mol O2
0.934 mol Ar + …
or, if we have 100 mmHg total pressure, then the partial pressures are
78.08 mmHg of N2
20.95 mmHg of O2
0.934 mmHg of Ar + …
Consider an experiment where we’re collecting a certain gas by bubbling it into an inverted glass jar initially filled with water.
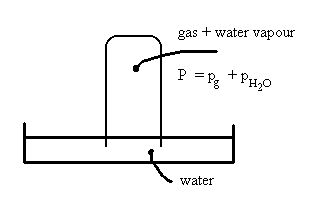
The partial pressure of water, pH2O, in this case is actually the vapour pressure, P*H2O, and can be found in any one of several tables of thermodynamic data.
There are many other situations in which we must consider the pressure of more than one gas at a time. Consider, for example:
A closed bulb contains 0.0100 mol of He(g) and a sample of solid white ammonium chloride (NH4Cl). The pressure of the Helium at 27℃ is 114 mmHg.
The bulb is then heated to 327℃ and the ammonium chloride decomposes into ammonia gas and hydrogen chloride gas. The final pressure is 908 mmHg.
- What is pHCl @ 327℃ ?
- How many grams of ammonium chloride were in the bulb initially?
To solve this:
a. We sum up the partial pressures.
PT = pHe + pNH3 + pHCl = 908 mmHg (after the reaction @ 327℃)
According to the ideal gas law,
![]()
Since, for the He, n and V are constant then we can use the P/T constant ratio to determine He pressure at 327℃.
![]()
![]()
The balanced chemical reaction for the decomposition of ammonium chloride is
NH4Cl(s) → NH3(g) + HCl(g) 1:1 ratio. therefore:
pNH3 = pHCl = X
PT = 228 mm Hg + 2X = 908 mHg
X = 340 mmHg = pHCl
b. We start by writing out the number of moles of HCl and of He since we can use this in Dalton’s Law.
![]()
(We don’t know V or T so we need at least one more equation)
![]()
Now divide these two equations one into the other to eliminate the V/RT .
![]()
![]()
Now, we convert the moles of HCl into g of NH4Cl using an extended fraction. To do this, we need two conversion factors. The first is the mole ratio from the balanced chemical equation. The second is the molar mass of ammonium chloride.
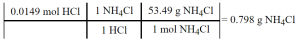
4.8: The Kinetic Molecular Theory
To understand a gas and its properties, we need to lay out a few concepts. In the kinetic molecular theory, there are several postulates upon which we base our explanations.
- a gas is composed of molecules that are far apart from each other in comparison to their own dimensions (most volume occupied by a gas is empty space).
- gas molecules are in constant random motion, each molecule continues to move in a straight line unless it collides with another molecule or with a wall of the container
- the molecules exert no force on each other or on the wall unless they collide. These collisions are elastic (the total translational kinetic remains unchanged).
- the average kinetic energy of the molecules is proportional to the absolute temperature.
The kinetic energy of a single molecule is
![]() where
where ![]() is the mass of the molecule and
is the mass of the molecule and ![]() is the speed of the molecule.
is the speed of the molecule.
Let’s consider a single molecule of mass m traveling with speed ![]() in a box where all sides (
in a box where all sides (![]() ,
, ![]() , and
, and ![]() ) have length
) have length ![]() . The average time it takes for the molecule to strike a wall, bounce around and return
. The average time it takes for the molecule to strike a wall, bounce around and return 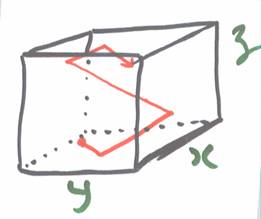 to a wall to strike it again depends on the component of speed perpendicular to the wall and on
to a wall to strike it again depends on the component of speed perpendicular to the wall and on ![]() .
.
Thus, for the wall perpendicular to the ![]() direction, the number of times per second a molecule strikes one wall is
direction, the number of times per second a molecule strikes one wall is ![]() . (it must travel length
. (it must travel length ![]() twice between each collision with a given wall).
twice between each collision with a given wall).
Since the collisions are elastic, the change in momentum is simply the difference from the momentum before collision ![]() and the momentum after collision
and the momentum after collision ![]() .
.
![]()
Thus, the change in momentum per second of one wall is
![]()
Since force equals the rate of change of momentum, we’ve just calculated the force exerted on the wall. We know that Pressure is measured as Force divided by area so we can now calculate the pressure exerted by a single molecule in the ![]() direction.
direction.
![]()
since L x A = Volume (V). Similarly, we can measure force and pressure in ![]() and
and ![]() directions. The overall pressure caused by one molecule will be the average of the three component pressures
directions. The overall pressure caused by one molecule will be the average of the three component pressures
![]()
where
![]()
We can extrapolate this idea to a gas sample with ![]() molecules in it by using, instead of the speed
molecules in it by using, instead of the speed ![]() , the average of the square of the speeds. This is done to allow greater influence of the outlier speeds in determining the average value. This is the mean-squared-speed.
, the average of the square of the speeds. This is done to allow greater influence of the outlier speeds in determining the average value. This is the mean-squared-speed.
![]()
Thus,
![]()
The average translational kinetic energy
![]()
can now be incorporated into this equation.
![]()
Thus, for one mole (n=1) of an ideal gas we can write
Total translational kinetic energy per mole
![]()
Now let’s look at the effects these have on our relationships we’ve seen earlier:
Boyle’s Law says that, for a sample of gas, ![]() is constant at any given temperature.
is constant at any given temperature.
We can see that the Kinetic molecular theory predicts that ![]() is dependent only on the number of molecules and on the temperature. If we reduce the volume of a container of gas by 1/2 (at constant
is dependent only on the number of molecules and on the temperature. If we reduce the volume of a container of gas by 1/2 (at constant ![]() ) then the pressure should rise by 2.
) then the pressure should rise by 2.
We have seen that P comes from collisions with the walls. If the volume is reduced then the length of time it takes a molecule to travel back and forth between the walls is reduced and the number of collisions goes up, thus the pressure goes up.
Charles’ Law says that the volume to temperature ratio of a gas is constant. Again, we can see that this is exactly what theory predicts.
Notice that I did not specify the molar mass of the molecules when I stated that kinetic energy was proportional to ![]() . Thus whether the molecules are heavy or light or if there is a mixture of molecules they all have the same (average) kinetic energy at a given
. Thus whether the molecules are heavy or light or if there is a mixture of molecules they all have the same (average) kinetic energy at a given ![]() .
.
This is called the Principle of Equipartition of Energy.
We can look at how molecular motion varies with molar mass using a few simple relationships. Let’s consider one mole of an ideal gas. There are ![]() molecules in one mole.
molecules in one mole.
![]()
with some rearranging, we get
![]()
This leads us to the Root Mean Squared Speed
![]()
We can measure this effect quite handily. Heavy molecules move slower than lighter molecules. Thus, if we were to release two different scents into the air at one time, the one coming from the lighter molecule would be smelled (assuming no wind currents) first by an observer as some distance from the two samples. This effect is also seen when we have helium balloons The helium is a light molecule and quickly escapes through the pores in the latex rubber of the balloon. Balloons inflated by breath (with heavier air molecules) stay inflated much longer.
One other consequence of the Kinetic Molecular Theory is the fact that we’ve not actually been able to measure the speed of any given molecule, just their average speeds. There is an equation called the Maxwell-Boltzman distribution, which predicts the proportion of molecules in a gas sample to have any given speed (or kinetic energy). We can see this in action from the following diagram plotting the fraction of molecules versus the speed of the molecules for a sample of molecules at several temperatures. An Excel spreadsheet has been kindly supplied by Prof. Horton that allows you to experiment with these settings. You can download it from here. Try inserting different molar masses or temperatures into the data page and see what happens to the graph.
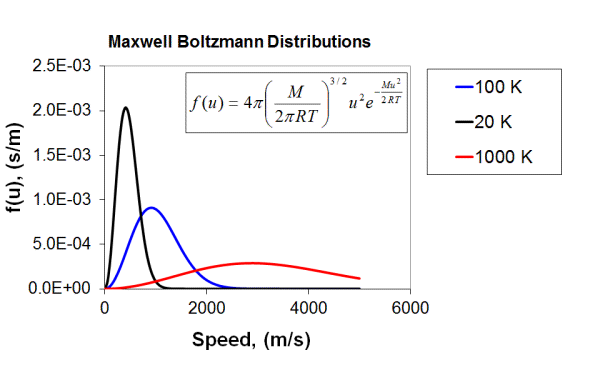 |
| Figure: The Maxwell-Boltzman distribution for three different gas samples (red, blue and black). In this case, the only difference between the three gas samples is the temperature, as indicated in the key on the right. |
4.9: The Van der Waals Equation
We’ve already discussed the postulates behind the ideal gas law but let’s recap here.
Ideal gases have molecules:
- that are infinitely small in size,
- that don’t interact with each other.
Is there a way to take into account the fact that these two assumptions are not always valid? To account for these two properties of the material which the Ideal gas law ignores, we need two new parameters. In 1873, J. H. van der Waals proposed a new equation which attempted to account for the size of the atoms and their interactions. The parameterized phenomenological equation contained two adjustable parameters a and b. These two parameters are varied to fit the equation results to the experimental properties of the gas in question.
The van der Waals equation is
![]()
or by dividing both sides by n, we get an expression that uses molar volume,
![]()
where Vm = V/n = molar volume of the gas. For most gases, the values of a and b are quite small (See table of values).
To try to understand the meaning of the two parameters, we can rearrange the vdW equation to have pressure on the left hand side and then compare this equation with the ideal gas law.
![]()
The vdW equation rearranges to be:
![]()
Recall that Vm is the molar volume. As a gas is compressed, the molar volume is reduced, which makes the relative size of the parameters a and b larger. Consider the situation where the molar volume is large (say, near standard temp and at or below standard pressure) then the second term ![]() will be quite small (because a is quite small compared to Vm). Similarly, the first term reduces the ideal gas law, since Vm is much larger than b, the denominator of the first term can be assumed to be identical to Vm with a high degree of accuracy. In other words, if the molar volume is large, the assumptions made in the ideal gas law are valid. As the molar volume becomes smaller, then the corrections made in the vdW equation become important.
will be quite small (because a is quite small compared to Vm). Similarly, the first term reduces the ideal gas law, since Vm is much larger than b, the denominator of the first term can be assumed to be identical to Vm with a high degree of accuracy. In other words, if the molar volume is large, the assumptions made in the ideal gas law are valid. As the molar volume becomes smaller, then the corrections made in the vdW equation become important.
The parameter a serves as a correction to the pressure of the gas as a result of intermolecular attractive forces. As the molar volume is reduced, the molecules are forced closer together and the interactions become significant.
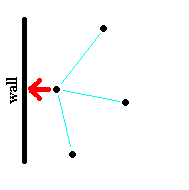 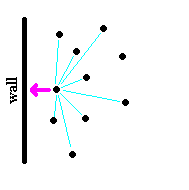 |
| Figure: The diagram on the left shows a low density (high molar volume) case where the atom, which is about to strike the wall (apply pressure) has relatively few attractive forces with other gas molecules because of large distances between them. On the right, the molar volume has been reduced and the resulting larger number of intermolecular forces acting on the molecules as they collide with the wall effectively reduce the force of the collision (pressure is lower than predicted by Ideal Gas law). |
The parameter b serves to correct the molar volume of the gas. An ideal gas has molecules that have no size. We need to correct for this assumption. If the molecules have a non-zero finite size then they will have, in effect less volume in which to travel since some of the volume of the container is now taken up by the molecules themselves. At low densities (high molar volumes) this is not very significant since the gas molecules are so far apart that they rarely interact anyway. As the gas is compressed, the molar volume of the gas is reduced to the point where the volume of the molecules becomes an important factor to consider.
  |
| Figure: The two cylinders contain the same amount of gas molecules (black dots) On the left, the molar volume is larger and the amount of free space for the molecules to move around in is larger than the volume of the molecules themselves. On the right, the cylinder has been compressed (smaller molar volume) and the gas molecules have much less free space to move about in. Now, the volume of the molecules themselves take up a significant portion of the volume of the cylinder, effectively reducing the volume available to any one molecule. |
For now, it is important to note that the parameters a and b are related to intrinsic properties of the gas itself and are therefore different for each gas. The following figure shows isotherms for three different gases as calculated using the van der Waals equation shown with T = 586 K. Curve 2 (black) is an ideal gas with parameters a = b = 0. Curve 1 (blue) is a gas with a vary small value for a but a moderate value for b. Note that at this temperature, there is no region where the gas pressure is smaller than a similarly conditioned ideal gas. Curve 3 (red) is a gas with a fairly large value of a and a moderate value for b. Note that for this gas, there are some conditions where the calculated pressure is lower than that of a similarly conditioned ideal gas. Parameter a is a measure of the attractive forces. Hence, the blue has very little attractive forces but some molecular volume (non-zero b) so the pressure only goes up as the molar volume goes down. The red gas has a slightly larger molecular volume than the blue gas and much larger attractive forces so as the molar volume goes down, you see both effects. As the molar volume is decreased, the attractive forces begin to dominate. Eventually at very small molar volume, the repulsive forces (molecular volume) takes over and you see a rapid rise in the pressure for that gas.
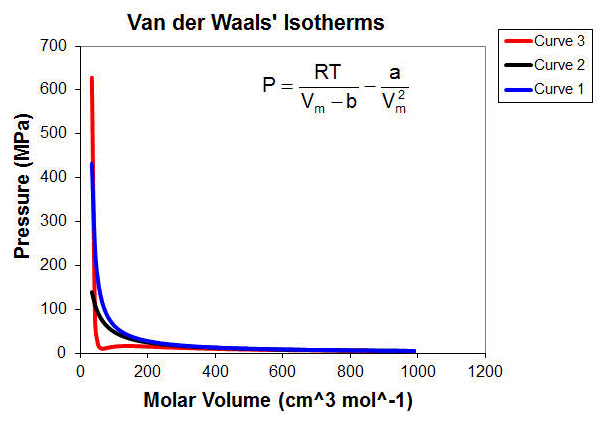
Through the use of this sheet, you can see that as parameter a is increased, the measured pressure is decreased, compared to an ideal gas (a = 0) pressure at the same conditions. Similarly, you can see that as b is increased, the pressure deviates positively from the ideal gas pressure.
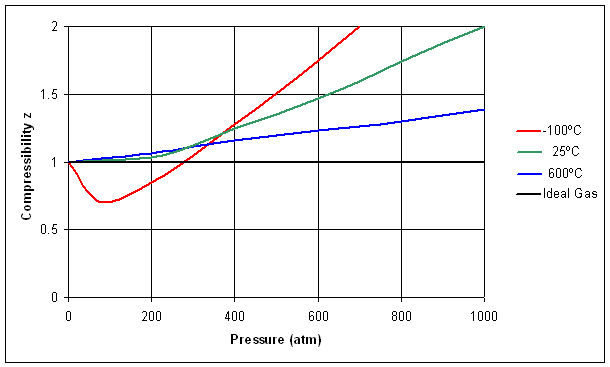
4.10: Compressibility Factor
We can also see the non-ideality of gasses by calculating the unit less compressibility factor, z. Parameter z is defined as the ratio of the measured molar volume of a gas to the molar volume of an ideal gas under the same T and P conditions.
![]()
for an Ideal gas, z always has a value of 1. For real gases, the value may deviate positively or negatively, depending on the effect of either the size of the molecules (repulsive forces), modeled by parameter b, or the attractive forces, modeled by parameter a.
|
|
| Figure: Compressibility factor for nitrogen gas as a function of pressure at three different temperatures. The Ideal gas value (z=1) is included for comparison. Notice that at lower temperatures and moderately high pressures, the attractive intermolecular forces cause a negative deviation from the Ideal Gas value for z, while at the higher temperatures, these do not contribute as significantly. At all temperatures, if the pressure is high enough, there will be a positive deviation because of the size of the molecules. |
-
Try not to get confused (as I did) between the Standard Temperature and Pressure for the Gas Laws
and the Thermodynamic Standard State conditions
STP (ref) Temperature = 0℃ Before 1990: Pressure = 101.325 kPa 1 atm 760 mmHg Since 1990: Pressure = 100 kPa (1 bar) 0.98692 atm 750 mmHg An unofficial standard you may have heard about is the Standard Ambient Temperature and Pressure. This NOT the same as the thermodynamic standard state and is not used as a standard in this chemistry course.Thermodynamic Standard State Temperature = (for tabulated data only) 25℃ (298.15 K) Pressure P = 100 kPa (1 bar) 0.98692 atm 750 mmHg
It's been some time now (since 1983) that the thermodynamic standard pressure has been 100 kPa (alias 1 bar). An older non-SI unit of pressure used to measure pressure and a different value for standard pressure was defined in that system. The old Standard pressure was 1 atmosphere (1 atm) where one atmosphere is taken to be the average atmospheric pressure at sea-level at about 45° longitude on the surface of the earth. While this notion of how to determine the standard pressure has some endearing charm to it, it does not result in a number that is easy to use calculationally. It is far easier to stick with the SI units of Pa. It just turns out that 100 kPa is very nearly the same pressure as 1 atmosphere. 100 kPa = 0.98692 atm = 1 bar. This is the new standard pressure for both Standard state calculations (thermodynamics) and STP (gas law calculations) ↵SATP Temperature = 25℃ (298.15 K) Pressure P= 100 kPa (1 bar) 0.98692 atm 750 mmHg
- The correct molar volume of an ideal gas using the modern definition of STP is 22.7 L/mol. Some of you may have memorized a value of 22.4 L/mol. This volume is only correct using the old definition of STP (0℃, 1 atm). ↵
- The height of a column of mercury that is supported by an ideal gas at STP is 750 mm. This is close to but not the same as the height of a mercury column supported by an ideal gas at the old value for STP (0℃, 1 atm) which is 760 mm. ↵
- It is useful to note that since the units of pressure (mmHg) used here have all cancelled it was not necessary to convert to SI units (Pa). This would have resulted in a wasted effort and exactly the same result in the end. Before converting to SI units always be sure you need to. If you can find a way to cancel out the units, simply do so and ignore them. ↵
- NOTE: Angle brackets
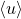 means average and may also be written as
means average and may also be written as 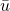 with a bar over it. Since that particular typesetting is difficult to do in a web page, I'll stick with the equally valid alternate means (pun) of indicating average. ↵
with a bar over it. Since that particular typesetting is difficult to do in a web page, I'll stick with the equally valid alternate means (pun) of indicating average. ↵ - Remember that
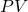 has units of energy:
has units of energy: 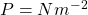 and
and 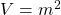 , so
, so 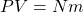 ==> Joules ↵
==> Joules ↵