Stoichiometry

Michael Mombourquette

3 Stoichiometry

Michael Mombourquette

3.1: Introduction

Stoichiometry refers to all quantitative aspects of chemical composition and reactions. The word comes from the Greek words for “element” and “measure”.

In this module, students will be learning about various basic calculation techniques that will be instrumental to the rest of their work in this course. Students will be called upon to use these techniques repeatedly throughout the course as part of answering later problems. Students should Learn the techniques and concepts described herein better than they know the back of their own hands.

3.2: Atomic Structure

Let’s explore first the general structure of an atom. We will go into much more detail in a later section but for now, we should recall a few basic concepts from our high school courses. The atom is made up of three types of elementary particles: the proton (p), the neutron (n) and the electron (e-). Each has a unique mass, although the mass of the proton, mp and the mass of the neutron is very close, whereas the mass of the electron is quite different. The proton contains a positive charge and the electron contains a negative charge while the neutron is neutral. The premise we want to explore here is that in chemical processes, these three particles will never be lost or created.

Consider Hydrogen. A hydrogen atom can be thought of as containing two particles:

Proton p	m_p =	1.6726 × 10^-27 kg
	Charge =	+1.6022 × 10^-19 C

Electron e^–	m_e =	9.1096 × 10^-31 kg
	Charge =	-1.6022 × 10^-19 C

One model of the hydrogen atom has the two types of particle orbit each other, much like the moon and earth do. Using Newtonian physics to describe their paths, we would expect to see that the larger mass object moves very little while the smaller mass object moves much more. Since the mass of the proton is about 1836 times that of an electron, it will move 1836 times less than the electron (since F = ma) for a given amount of kinetic energy per particle.

Thus, we can picture the atom as a central “fixed” nucleus with an electron orbiting around it. This is in line with the Rutherford model of the atom, which was later developed further by Bohr.

Unfortunately, this model doesn’t explain why the electrons don’t simply give up their energy and decay their orbit until they contact the nucleus and stay there. Newtonian physics predicts that the moon and earth (and all orbiting bodies) will eventually suffer this same fate.

A more modern treatment of the atom using quantum mechanics predicts that the atom can be thought of more like a fixed nucleus with electron position being undeterminable, but a “likelihood” of locating the electron around the nucleus would look like a probability cloud (e^– cloud).

The e^– density depends on distance r (more later) and on angular position.

The energy of such a system can be determined once we know the forces acting on the particles. While we are generally not going to go in to much detail about how to calculate certain energies from their given forces, we can at least recognize that if the forces are large, then the dynamic system containing such forces will likely have higher energies, etc.

There are 3 types of forces in nature:

gravitational
electrostatic important in Chemistry
nuclear

We are generally only interested in electrostatic forces in chemistry and from these, we can derive all the energies involved in chemical reactions.

The force of interaction between two point charges (very small charged partcles) can be described using Coulomb’s Law:

$F = K \frac{Q_1 Q_2}{r^2}$

where $F$ is the force of attraction (or repulsion) between two charged particles, and are the charges of the two particles, respectively, $r$ is the distance separating the two particles and $k$ is a constant derived from other universal constants and has a value of approximately 9×10⁹ N m²C^-2.

Charges are generally measured in Coulombs (C) and a value of -1 C = charge of 6.241×10¹⁸ electrons. This is not a generally convenient value of charge for atomic level discussions so we define ‘e’ as equal to the charge of one proton

e = 1.602 × 10^-19 C

So therefore,

-e = charge of one electron

We often use e as a unit of charge (more convenient).

e.g. H⁺ => proton => charge = +1e

e^– => electron => charge = -1e

He²⁺ => Helium Nucleus => charge = +2e

3.3: Mass of Atoms

If we measure the mass of hydrogen and helium, we get the approximate relationship

m_He = 4 m_H

Hydrogen has only a single proton in the nucleus so there must be 4 particles with the same mass as a proton in the helium nucleus. Ther are only two protons in Helium (+2 charge) so there must be two other particles that are almost the same mass as protons but with zero charge in the He nucleus. These are neutrons.

so He =>

2 protons + 2 neutrons

$\underbrace{+e\;charge\;\;\;\;\;neutral} }$

Nucleus

As a first approximation, we can add up the mass of the individual elementary particles within an atom to calculate its mass. This method yields calculated masses which are not very accurate for various reasons but we can still use the concept.

When we write atomic symbols, we often include the mass number and atomic number as superscripted and subscripted prefixed numbers, respectively, where

Mass Number = Total # of Nucleons (protons and neutrons)

Atomic number Z = Number of Protons in the nucleus.

Actually, the subscript atomic number is a bit redundant as the chemical symbol already dictates the number of protons. Hydrogen has one proton, helium has two protons etc. However, we sometimes include the value of Z in the chemical name for completeness.

subscript 1 is Not needed as symbol H implies Z = 1 etc.	→	$_{1}^{1}\mathrm{H}$	Z = 1 mass # = 1 (Hydrogen; one proton)
		$_{1}^{2}\mathrm{H}$	Z = 1 mass # = 2 (Deuterium, D; one proton and one neutron) This is an isotope of H.
		$_{2}^{4}\mathrm{He}$	Z = 2 mass # = 4 (Helium)
		$\begin{array}{lcl}_{8}^{16}\mathrm{O}\\ _{8}^{17}\mathrm{O}\\ _{8}^{18}\mathrm{O}\end{array}$	all have eight protons. so same element but 8, 9, 10 neutrons, respectively. So they are different isotopes.

In chemical reactions, all nuclei remain unchanged – only the electrons are redistributed. In nuclear reactions, the nuclei are changed (high energy bombardment by proton, He²⁺, etc. ).

Chemicals with different isotopes have very similar chemical & physical properties:

	Freezing	Boiling
H₂O	O ℃	100 ℃
(heavy water) D₂O	3.82 ℃	101.42 ℃

In a naturally occurring sample of hydrogen, we find mostly H, but some D:

relative abundance	0.99985 H
	0.00015 D

We can use the relative abundances along with the atomic mass of each isotope to determine an average mass for a sample of hydrogen.

Example: Calculate the mass of an “average” atom of hydrogen found in nature if the isotope masses of H = 1.00783 u and of D = 2.01410 u.

Mass of H (= 1.00783 u) × 0.99985	= 1.00768 u
Mass of D (= 2.01410 u) × 0.00015	= 0.00030 u
Average Mass of Hydrogen	= 1.00798 u

NOTE: Symbol u stands for atomic mass unit and represents 1/12 of the mass of an atom of $^{12}_{\;6}\mathrm{C}$

We can use average measured mass of an element to determine the relative abundances of the isotopes that comprise it.

Example: $.$ Chlorine has two isotopes $_{17}^{35}\mathrm{Cl}$ and $_{17}^{37}\mathrm{Cl}$ , with masses of 34.96885 u and 36.96590 u respectively.

The average atomic mass of Chlorine is 35.453 u. What are the relative abundances (in percent) of the two isotopes?
Let fractional abundance (mass fraction) of $^{35}\mathrm{Cl}$ be X.
Therefore, the fractional abundance of $^{37}\mathrm{Cl}$ is (1-X) , since the sum of the two isotope abundances must be 1.

We thus have

(34.96885 u) (X) + (36.96590 u) (1-X) = 35.453 u

after some algebra: X = 0.7576 1-X = 0.2424
so, the percent abundance of $^{35}\mathrm{Cl}$ = 75.76%
and the percent abundance of $^{37}\mathrm{Cl}$ = 24.24%

Note that the two percent abundance values are quoted here to only 4 sig figs while the two masses are given to 7 or 8 sig figs. This apparent loss of sig figs results from the fact that during the algebra there is a subtraction of one mass from the other. In other words, it’s the difference in the two masses that is significant, not their absolute value in determining the accuracy of this result.

We often use atomic mass or Relative Atomic Mass as a short form for average atomic mass. The word relative means, relative to carbon-12, which has a defined mass of exactly 12u. Note that the relative atomic mass of carbon in the table is not exactly 12. That is because there is a small amount of carbon-13 and carbon-14 that is found in naturally occurring samples.

Click here for a table of relative atomic masses.

The accuracy to which the atomic masses are known vary, depending on stability of isotopes and on constancy of isotopic ratios. We see that the number of figures of accuracy differs for each element. Atomic masses given in parentheses are those of radio-active elements whose mass is known with very poor accuracy.

3.4: Conservation of Mass and Energy

Much of the work of measuring and calculating amounts in chemistry depends on the law of conservation of mass, which can be stated as
“Mass can neither be created, not destroyed”.

Let’s try a simple test of this law.

If we add the masses of the subatomic particles that make up ⁴He, we get

2 protons	= 2 × 1.00728 u =	2.01456 u
2 neutrons	= 2 × 1.00866 u =	2.01732 u
2 electrons	= 2 × 0.00055 u =	0.00110 u
		4.03298 u

but experiment shows that the measured mass of ⁴He is 4.00260 u

difference is 0.03038 u

This is because of energy released as the particles combine to form the atom.

E = mc²

So we see that mass is not conserved. Energy and mass can be inter-converted.

Thus: original Law

Mass can neither be created, nor destroyed, is false.

We now have Two Laws:

In a chemical reaction, atoms (and hence mass) are neither created nor destroyed.
Energy can be neither created nor destroyed but can be changed from one form to another.

3.5: The Mole

Working with individual atoms and individual molecules doesn’t happen easily in chemistry. Generally, we work with large amounts of atoms and molecules so it is convenient to define a measure that will allow us to work with large amounts of these subatomic particles.

We are familiar with going to a bakery and asking for 2 dozen buns. We know that means 24 buns. It’s a bit more convenient to use the number “dozen = 12” to simplify the counting of our baked items. We do the same in chemistry with the number called mole. Additionally, we want to be able to use those same masses we saw in the table of relative atomic masses, but in more macroscopic units.

We define the mole to the number of ¹²C atoms necessary to make up a mass of exactly 12 g. Since we had also defined the mass of one atom of ¹²C to be exactly 12 u. Now, we can use the numbers in the “relative atomic mass” table quite readily in units of u (per atom) or g/mole, depending on our needs for the problem at hand. We call this number N_A => Avagadro’s constant (or number):

N_A ~ 6.022045 × 10²³

This number is conveniently used as a unit of measuring the amount (number) of elementary particles (atom, molecule, ion, etc…) in a sample. The number is called the Mole.

Mole => the amount of a substance which contains as many elementary entities as there are atoms in exactly 0.012 kg (12g) of ¹²C.

When the unit mole is used, the elementary entities must be stated, e.g., Atoms, Molecules, Ions, etc…

Species	Average Species Mass	Mass of one mole of the species
Atoms
C atom	12.01 u	12.01 g
O atom	16.00 u	16.00 g
Al atom	26.98 u	26.98 g

Molecules
CO	12.01 u + 16.00 u = 28.01 u	28.01 g
CH₄	12.01 u + 4 × 1.008 u) = 16.04 u	16.04 g

Example: How many moles of Al are in 50.00 g of aluminum?

50 g Al = ? mol Al atoms

We know the atomic relative mass of aluminum is M_Al = 26.98 u, so 1 mol Al = 26.98 g Al We use this equality as a conversion factor:

$\begin{array}{|c|} 1 mol\; Al\; atoms\\ \hline 26.98 g\; Al\end{array}$

Note that the equality written here may seem confusing as it is somewhat abbreviated. Earlier, I said both sides of a true equation must have the same dimensions. This ‘equation’ has the missing words “the amount of aluminum in” missing from both sides, for brevity. Thus, the dimension is “amount” and the units of measure of that amount could be either grams or moles.

$\begin{array}{|c|c|c} 50.00\; g\; Al & 1\; mol\; Al\; atoms \\ \hline & 26.98\; g\; Al\end{array} = 1.853\; mol\; Al atoms$

The mass of 1 mole of a substance is its molar mass (M).

For example, the mass of 1 mole of H = 1.008 g, so,

$\begin{array}{rcl} \textrm{Molar Mass } (M_H) &=& 1.008\textrm{ g/mol}\\ &=& 1.008\textrm{ g mol}^{-1} \end{array}$

We’ll use this molar mass as our starting point for the next question.

What is the mass of 1 mole of H₂?

By counting the atoms, we can make the following equality: 1 H₂ = 2 H. We turn this equality into a conversion factor to use in the calculation. Some people like to put the unit mole into this relationship but it is redundant since the ratio is a ratio of atoms and molecules just as validly as it is a ratio of moles of those same atoms and molecules. It’s a ratio. The units cancel.

$\frac{\mathrm{2 H}}{\mathrm{H}_2}$

We’ll be very explicit in the units, including the species to make the units very clear here. This may seem redundant but you’ll find it is a very good way to avoid the mistake of, say dividing by 2 rather than multiplying by 2. This may seem silly but it is a very common mistake made by students who do not do the thorough math. Using the extended fraction formalism discussed earlier, we write:

$\begin{array}{|c|c|} \textrm{1.008 g} & \textrm{2 H} \\ \hline \textrm{1 mol H} & \textrm{1 H}_2\end{array} = 2.016 \textrm{ g/mol H}_2$

We know we have the correct math done here since the units “H” cancel properly.

We must be careful when talking of moles. moles is not a unit without identifying the species being counted using these moles. If we say: “what is the mass of one mole of hydrogen?” we have not distinguished between moles of H atoms and moles of H₂ molecules.

Always specify the elementary particle of interest when discussing moles.

The mass of one mole of any atom in the table is simply the relative mass number in the table, but with units of g/mol.

The mass of one mole is more succinctly called the “Molar Mass”. Thus, we can look up the molar mass of any element, say Beryllium, from the table. M_Be = 9.012182 g/mol, where the variable M (capital M) means molar mass and the units normally associated with it are written as g/mol.

Formula Mass & Molecular Mass

Consider Hydrogen peroxide:

Hydrogen peroxide is a molecular compound. Experiment shows that it has a ratio of H atoms to O atoms of 1:1. Thus, we could write a formula for this compound to be HO. Since we do not know if this formula represents the actual number of atoms in the molecule, we must label it the Empirical Formula. The empirical formula gives the lowest whole-number ratio of the atoms in compound.

We can calculate the empirical formula molar mass as the molar mass of such a molecule if it existed.

Example: The empirical formula molar mass of hydrogen peroxide is

HO ⇒ (1.00794 g/mol) + (15.9994 g/mol) = 17.00734 g/mol.

In a certain experiment, we measured the molar mass of hydrogen peroxide to be about 34 g/mol. Clearly, the empirical formula molar mass is only about half the measured molar mass. Thus, there are likely two empirical formula units in every molecule. Hence, it’s molecular formula is H₂O₂. And we can calculate a molecular formula molar mass more accurately using the table of relative masses to be

H₂O₂ ⇒ 2(1.00794 g/mol) + 2(15.9994 g/mol) = 34.01468 g/mol.

Molecular Formula Molar Mass of H₂O₂ is 34.01468 g/mol. Since this is very close to the measured molar mass, we are confident that the correct molecular formula is H₂O₂.

Now consider sodium chloride. It also has a 1:1 ratio of atoms Na:Cl. thus, the empirical formula is NaCl and we can calculate the empirical formula molar mass to be

NaCl ⇒ 22.989768 g/mol + 35.4527 g/mol = 58.44247 g/mol.

NaCl does not form Molecules so there is no molecular formula for sodium chloride, we must use the Empirical formula when referring to this compound.

Empirical Formula Molar Mass of NaCl is 58.44247 g/mol.

We often use a simplified term molar mass when referring to chemical compounds. If that compound forms molecules, then our simplified term refers to the molecular formula molar mass but if the compound does not form molecules (salts and metals) then the term refers to the empirical formula molar mass.

Thus, the molar mass of hydrogen peroxide is 34.01468 g/mol and the molar mass of sodium chloride is 58.44247 g/mol.

Distinction between the empirical formula and molecular formula:

$\begin{array}{cccc} \textrm{Substance} & \textrm{Emperical Formula} & \textrm{Molecular Formula} & \textrm{Molar Mass}\\ \hline \textrm{water}& \mathrm{H_2O} &\mathrm{H_2O} & 33.0674 \textrm{g/mol *}\\ \\ \textrm{hydrogen peroxide}& \mathrm{HO} &\mathrm{H_2O_2} & 34.01468 \textrm{g/mol}\\ \\ \textrm{sodium chloride}& \mathrm{NaCl} &\oslash & 58.44247 \textrm{g/mol *}\\ \textrm{(table salt)}\\ \textrm{ethane}& \mathrm{CH_3} &\mathrm{C_2H_6} & 30.06904 \textrm{g/mol}\\ \\ \textrm{benzene}& \mathrm{CH} &\mathrm{C_6H_6} & 78.11184 \textrm{g/mol}\\ \\ \textrm{Iron (metal)}& \mathrm{Fe} &\oslash & 55.845 \textrm{g/mol *}\\ \end{array}$

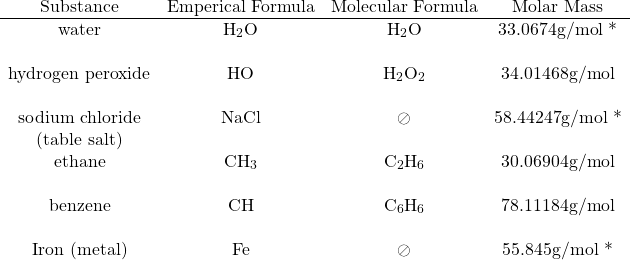
* These molar masses are based on the empirical formula mass for different reasons:

water’s molecular formula is the same as its empirical formula,
sodium chloride is a salt, which doesn’t form molecules and finally,
iron is a metal, which doesn’t form molecules.

3.6: Chemical Calculations – Composition & Formulas

We are often interested in understanding the composition of a substance. One way of expressing this is in % composition. We can express the percentage of a substance found within a given sample using several measurement techniques. For example, we often use volumes to compare liquids mixed together, c.f. the amount alcohol in beer is about 5%v/v. In other words, 5% of the volume of a bottle of beer is alcohol.

Another very common measurement technique is measure the mass of the material. In a given chemical compound, we can use mass measurements to determine the percent by mass of each element found within the compound.

% by mass of an element in a compound	=	molar mass of element in 1 formula unit	×100%
		molar mass of the compound
or
% by mass of an element in a compound	=	mass of element in 1 mole of compound	×100%
		mass of one mole of the compound

The difference in these two formulae is not quite trivial. In the former, the units in the fraction are g/mol whereas in the later formula, the units are just g. In either case, the units cancel, leaving only a % symbol.

For example:

Molar Mass of Fe₂O₃ ⇒ (55.85 g/mol) (2) + (16.00 g/mol) (3) = 159.70 g/mol

∴ Molar Mass = 159.70 g mol^-1

One mole of Fe₂O₃ contains 2 mol Fe atoms

Total molar mass Fe in the formula = 55.85g/mol ×2 = 111.70 g/mol

$\mathrm{ \% Fe = \frac{111.70}{159.7 g/mol}\;\times\;100\%\!=\!69.94\;\%}$

Similarly, for Oxygen: [using the second formalism above of the mass in one mole rather than the molar mass. (same number, different units)]

total mass of oxygen in one mole of compound is 3×16.00 g

$\mathrm{ \% O = \frac{3\times (16.00 g) }{159.7 g/mol} \times 100\%\!=\!30.06\;\%}$

Alternatively, since this compound contains only Fe and O, we can calculate the % O by subtracting the % Fe from 100% because, by definition,
%Fe + %O = 100%, so 100% – 69.94% = 30.06%

Now that we know this concept of percent by mass, we can use it to calcuate other values.

Example: How much Nitrogen is needed to make 10.00 kg of ammonia?

Ammonia ⇒ NH₃ → 1 atom N for every Molecule NH₃

$\mathrm{ \% N = \frac{ Mass\; of\; 1\; mole\; of\; N}{Mass\; of\; 1\; mole\; of\; NH_3} \! \times 100 \%}$

$\mathrm{ \% N = \frac{14.01\;g}{14.01\;g + 3(1.008)\;g} \times 100 \% \! = \! 82.25 \% }$

We can use the percent by mass as a conversion factor if we write it out in it’s full form. Recall that percent means “per 100”. So, we can write:

$\mathrm{\% N\;=\;82.25\%\;=\; \frac{82.25\; g\; N}{ 100\; g\; NH_3}}$

We can use a percent by mass as a conversion factor to convert from mass of compound to mass of element or vice versa. So we can now use this conversion factor to determine the mass of N in 10.00 g of NH₃. Using the conversion factor, we can easily see that by cancelling the units “kg NH₃” we get the desired units of “kg N” and hence we know that we have done the correct math calculation.

$\begin{array}{|c|c|} \mathrm{10.00\; kg\ NH_3}} & \mathrm{82.25\; g\; N} \\ \hline & \mathrm{100\; g\; NH_3} & \end{array}\mathrm{\;=\;8.225\; kg\; N}$

We need 8.225 kg of N to produce 10.00 kg of NH₃.

3.6.1: Empirical Formula Determination

Recall that the empirical formula gives us the lowest whole number ratio of the moles of elements in a compound. Generally, we cannot measure moles directly (too many molecules to count). We measure the mass of a compound and through experiment, we determine the composition by mass of the elements in the compound. The simplest calculation we might perform to find an empirical formula would start with the percent by mass of the elements.

Example: The percent by mass of hydrogen in water is determined to be 11.19%. What is the empirical formula of water?

Since there are only two elements in water, we can easily calculate the percent by mass of oxygen as

% O = 100% – % H.

% O = 100% – 11.19% = 88.81%

now, we need to determine the actual number of grams of each element so we can easily do this if we assume we have 100g of water.

So, we can calculate $m_H = 11.19 \% \times 100$ g water = 11.19 g H … remember that, in this case, % means $\frac{\textrm{g H}}{100\textrm{ g water}}$ so the 100 g water cancels out. It’s more obvious why we choose 100 g H2O if we expand the 11.19 % and use the extended fraction formalism.

$m_\textrm{H} = \begin{array}{|c|c|} \mathrm{11.19\; g\; H} & \mathrm{100\; g\; H_2O} \\ \hline \mathrm{100\; g\; H_2O} & \end{array} \mathrm{\; =\; 11.19\; g\; H_2O}$

Similarly, we can easily calculate the mass of oxygen.

$m_\textrm{O} = 88.81\; g$

Now, we use molar mass of the elements as conversion factors to change from grams of the element to moles of the element.

$n_\textrm{H} = \begin{array}{|c|c|} \mathrm{11.19\; g\; H} &\mathrm{1\; mol\; H }\\ \hline & \mathrm{1.008\; g\;H}\end{array}\mathrm{ \; =\; 11.01\; mol\; H\;atoms}$

$n_\textrm{O} = \begin{array}{|c|c|} \mathrm{88.81\; g\; O }& \mathrm{1\; mol\; O }\\ \hline & \mathrm{16.00\; g\;O}\end{array} \mathrm{\; =\; 5.55\; mol\; O\;atoms}$

Once we have the moles of each element, we look for the lowest whole number ratio of moles. To do this, divide by the lowest number of moles (in this case divide moles of H and moles of O by 5.55) to get the mole ratio.

Ratio:

$\textrm{# mol H atoms} = \frac{11.10\textrm{ mol}}{5.55\textrm{ mol}} = 2 \rightarrow \textrm{H}$

$\textrm{# mol O atoms} = \frac{5.55\textrm{ mol}}{5.55\textrm{ mol}} = 1 \rightarrow \textrm{O}$

This is the lowest possible ratio of H and O so we have the emperical formula for water, H₂O.

It turns out that this is also the molecular formula. But we cannot know this unless we perform a separate experiment in which we measure the molar mass of water. According to the emperical formula, the unit mass is $2\times 1.008 + 16.00$ g/mol = 18.02 g/mol. This is also the experimentally determined molar mass of water so we can be confident that the molecular formula for water is the same as the emperical formula, H₂O.

Example: This example gives us a ratio that is not the lowest whole number mole ration. We need to take do some extra steps to find the lowest whole number mole ratios.

A compound containing only Phosphorous and oxygen was found to have the following composition:

43.7% P 56.3% O by mass .

What is the empirical formula?

Assume 100 g of compound →	43.7 g P
	56.3 g O

Using the Extended fraction formalism, we use the molar mass as a conversion factor to convert mass to moles:

$\begin{array}{|c|c|} \mathrm{43.7\; g\; P} & \mathrm{1\; mol\; P} \\ \hline & \textrm{30.97 g P}\end{array}\textrm{ = 1.41 mol P}$

$\begin{array}{|c|c|} \textrm{56.3 g O} &\textrm{ 1 mol O }\\ \hline & \textrm{16.00 g O}\end{array}\textrm{ = 3.52 mol O}$

Example:

In this example, we show an actual experiment, whereby mass measurements are made to determine the amount of each element in an unknown compound. This is an example of a general class of experiments whereby we perform chemical reactions of some sort or other to separate the elements of a compound so they can be weighed separately. For example, in the combustion of a compound which contains carbon and hydrogen and oxygen like ascorbic acid, we can use the device pictured below to measure the amount of hydrogen (measured as a mass of water collected) and the amount of carbon (measured as a mass of carbon dioxide collected) and with some work, we can calculate the amount of oxygen and hence determine the empirical formula.

Here is a diagram of an experimental apparatus. In this experiment, the compound is burned with excess oxygen gas. The exhaust gas contains fully oxidized products (we assume fully oxidized products because we use a large excess of oxygen; this may not always be a valid assumption in some reactions where the reaction is slow or complex). The exhaust gases are passed through a chamber containing a chemical that absorbs water and carbon dioxide, in turn. By measuring the increase in the mass of these components, we can proceed to get the empirical formula as described below.

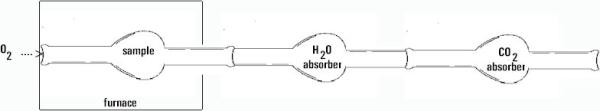
Example: A sample of 6.49 mg of ascorbic acid is burned in excess oxygen. Ascorbic acid contains only C, H and O. The mass of the H₂O absorber increased by 2.64 mg while that of the CO₂ absorber increased by 9.74 mg. What is the empirical formula for ascorbic acid?

Our goal here is to determine the amount of each element in ascorbic acid. Since the experiment only measures water and carbon dioxide, we will have to use these to figure out the hydrogen and carbon content respectively. We first assume that the only source of hydrogen to make water and carbon to make carbon dioxide was from our sample (true if the apparatus was clean). We assume the two absorbers absorbed only (and all) the particular compound they were designed for. Thus, if we can figure out the number of moles of each element in these compounds, we’ll have the number of moles in our original sample.

Molar mass H₂O = 2(1.008) + 16.00 = 18.02 g mol^-1

Molar mass CO₂ = 12.01 + 2(16.00) = 44.01 g mol^-1

First, we’ll use these molar masses to determine the moles of the each compound (H₂O and CO₂) collected and then the mass /mole of the element H and C, respectively in each compound to get the final mass of the element.

Mass of H collected =

$\begin{array}{|c|c|c|c|} \mathrm{0.00264 \; g\; H_2O} & \mathrm{1\; mol\; H_2O} &\mathrm{ 2\; H} &\mathrm{ 1.008\; g\; H} \\ \hline & \mathrm{18.02\; g\; H_2O} &\mathrm{ 1\; H_2O} &\mathrm{ 1\; mol\;H}\end{array} \mathrm{\;= 0.295 \times 10^{-3} \;g\; H}$

Mass of C collected =

$\begin{array}{|c|c|c|c|} \mathrm{0.00974\; g\; CO_2} &\mathrm{ 1\; mol\; CO_2} &\textrm{1 C}& \textrm{12.01 g C} \\ \hline & \mathrm{44.01\; g\; CO_2} &\mathrm{1\;CO_2}&\textrm{1 mol C}\end{array} \mathrm{\;= 2.66 \times 10^{-3}\; g\; C}$

Now that we know the mass of C and of H, we can determine the mass of O by subtracting these two amounts from the total mass of the ascorbic acid, since it contains only C, H and O.

Mass O in ascorbic acid = 6.49 mg – (2.66 mg + 0.295 mg) = 3.53 x 10^-3 g O
(Note, I replaced the “× 10^-3” by the prefix milli as in 1×10^-3 g = 1 mg)

Now that we have the mass of each element, we can calculate the moles of each element and from there, the mole ratio of each element.

Set up as a table to show data:
$\begin{array}{lccc} \hline\\ &\textrm{C}&\textrm{H}&\textrm{O}\\ \textrm{mass} & 2.66\times10^{-3}\textrm{ g} & 0.295\times10^{-3}\textrm{ g} & 3.53\times10^{-3}\textrm{ g}\\ \\ \hline\\ \textrm{moles} & \frac{2.66\times10^{-3}g}{12.01\;g/mol}& \frac{0.295\times10^{-3}g}{1.008\;g/mol} & \frac{3.53\times10^{-3}g}{16.00\;g/mol}\\ {\small \textrm{(of atoms)}}& 2.21\times10^{-4} mol & 2.92\times10^{-4} mol & 2.21\times10^{-4} mol \\ \\ \hline\\ \textrm{mole ratio} & \frac{2.21\times10^{-4} }{2.21\times10^{-4}} & \frac{2.92\times10^{-4}}{2.21\times10^{-4}} & \frac{2.21\times10^{-4}}{2.21\times10^{-4}}\\ {\small \textrm{(divide by smallest)}}&1.00 & 1.32 & 1.00\\ \\ \hline\\ \textrm{whole \# ratio} & 3 & 3.96\approx 4 & 3\\ \end{array}$

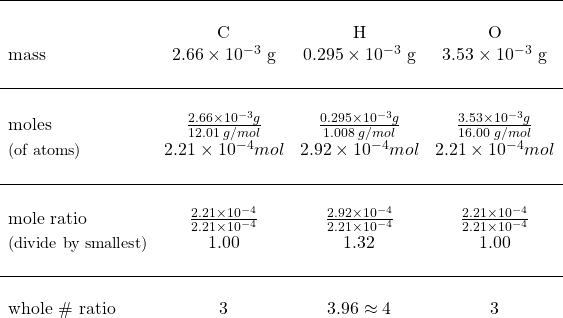
$\therefore$ the empirical formula = C₃H₄O₃

3.6.2: Chemical Equations

Many chemical reactions can be represented, at least in summary, by listing the reactants and products, separated by an arrow to represent the chemical process involved. Although it is not common that reactions would occur in a single step as might be implied by such a construct, this method is still very useful. This method represents, essentially, the initial and final states of the chemical system involved, not the actual chemical process involved.

Consider the reaction of carbon and oxygen to produce carbon dioxide. We can represent this reaction easily as described above with the following construction:

C + O₂ → CO₂

In most reactions, we must do a bit more work to properly identify the proper initial and final state of the chemical system. For thermodynamics, it is very important to exactly identify the state of each chemical.

C(s, graphite) + O₂(g) → CO₂(g)

Here, we can easily see that carbon is in the solid state (in particular, graphite) while oxygen and carbon dioxide are gases. Here, we have a completed chemical reaction. It properly identifies the states of the chemical system before and after the reaction takes place. Yet, this reaction is still far from completely characterized. We know, for example, that carbon exists as graphite, buckyballs or as other aggregate particles, but rarely as individual atoms as this formula seems to imply.

Other reactions lead to further complications. We need to be concerned with the law of conservation of matter: “Matter can neither be created, nor destroyed”. Thus, there must be the same number of atoms of each element on the reactant side of a chemical reaction as on the product side. We’ll use this idea to help balance reactions where simply listing the compounds is not sufficient as it was above.

3.6.3: Balancing Chemical Reactions

Let’s consider the reaction between water and oxygen to produce water. We can write this reaction as a gas-phase reaction and eliminate complications like phase changes, etc.

H₂(g) + O₂(g) → H₂O(g)

Simply listing the compounds as we have done here does not satisfy the law of conservation of matter. We could easily decide what to do in the above case and rewrite this equation so that is does. By inspection, we have:

H₂(g) + 1/2 O₂(g) → H₂O(g)

This equation now follows the law of conservation of matter. There are exactly the same numbers of atoms of each element on both sides of the equation.

Algebraic Method

Let’s redo this balancing process using a more rigorous method called the algebraic method. This method will be useful to you to balance even the hardest of chemical reactions for the rest of your chemistry career. Here are the steps involved:

1) Write the unbalanced reaction by simply listing the reactants and products. draw a vertical line to divide the reactants from the products.

H₂(g) + O₂(g) | → H₂O(g)

2) Use the chemicals as column headers and add a column for totals for both reactants and products. Add a column called elements in front of the table.

Elements	Totals	H₂	O₂	H₂O	Totals
H
O

3) Assign letter variables (red) to indicate the amount of each compound, starting with the second one. (we assign an arbitrary value of 1 to the first coefficient.)

Elements	Totals	H₂	aO₂	bH₂O	Totals
H
O

4) Identify and count each element on the reactants and on the products side of the table and put the totals in the respective columns.

Elements	Totals	H₂	aO₂	bH₂O	Totals
H	2	2	0	2b	2b
O	2a	0	2a	b	b

5) The total on the left MUST equal the total on the right so you can set up equations and solve for the variables as a set of simultaneous linear equations. This part is just high-school algebra. You can solve for the variables any way you wish.

H: 2 = 2b so, b = 1

O: 2a = b so, a = 1/2

6) Finish balancing the chemical equation by putting the proper coefficients in place. If a coefficient turns out to be negative, move that chemical to the other side of the reaction.

H₂(g) + 1/2 O₂(g) → H₂O(g)

That seemed fairly simple. Obviously, we didn’t really need to use the algebraic method to solve this.

This next example is more complicated and will not easily be solved by inspection. We encounter some chemicals that have an electric charge. We must be able to account for the fact that we can’t produce or destroy electrons and therefore, the overall charge of the reactants should be the same as that of the products.

Whenever there are charges in the chemical equation, we simply add a row to our table to tally the charges just as we do for each element and continue as normal.

Example: Copper metal is dissolved in nitric acid to form a blue solution containing aqueous copper(II) and NO. Balance the reaction.

In this example, the reaction involves the transfer of electrons (oxidation and reduction) and we will see this in more details later in the term (see the section on REDOX). For now, know that since this reaction is in acidic water (aqueous) there will likely be H⁺ and H₂O in the final balanced equation. Let’s guess that one (H₂O) will be a reactant and the other (H⁺) a product. This guess is random. We could have put them both as reactants or products. The results will tell us if we guessed correctly and how to fix the guess.

The other reactants will be the copper metal and the nitrate ions, as stated in the problem wording. The products must be the copper(II) ion and aqueous nitrous oxide (NO).

So we get a proposed (unbalanced equation) as follows:

NO₃^–(aq) + Cu(s) + H₂O( $\ell$ ) → Cu²⁺(aq) + NO(aq) + H⁺(aq)

We now use the algebraic method to balance this chemical equation:

Elements	Totals	NO₃^–	aCu	bH₂O	cCu²⁺	dNO	eH⁺	Totals
N	1	1	0	0	0	1	0	d
O	3+b	3	o	b	0	d	0	d
Cu	a	0	a	0	c	0	0	c
H	2b	0	0	2b	0	0	e	e
charges	-1	-1	0	0	2c	0	e	2c+e

Now, according to the law of conservation of matter, we now have the following equations.

i. 1 = d, ii. 3+b = d, iii. a = c, iv. 2b = e, v. –1 = 2c+e

These 5 simultaneous equations in 5 unknowns are solvable by whatever method you choose. The following is but one way to work through to the solution.

By substituting equation i into ii, we get b = -2 (b is the coefficient for water and all coefficients must be positive. So, water must be on the product side, not the reactant side as we have it here.)

Now we can solve for e using equation iv. 2b = e → 2× (-2) = e = -4. The negative value means the H⁺ is on the wrong side in the table above too. It is a reactant.

Now we can solve for c using equation iv; and by equation iii we also get a.

-1 = 2c+e = 2c – 4 so 2c = 3 or c = 3/2 (= a)

We’ve now solved for all the coefficients and we can write the balanced chemical equation.

NO₃^– + 3/2 Cu + 4 H⁺ → 3/2 Cu²⁺ + NO + 2 H₂O

The coefficients we determined here are called stoichiometric coefficients and they describe the stoichiometry of the reaction. In other words, they describe the amounts or measure of each compound involved in the reaction in terms of molecules (or moles of molecules). Note that we have fractional coefficients. That is OK as they represent ratios of moles of atoms. If you prefer to use whole number ratios, you can do that but there is no imperative to do so without some other wording of the question.

3.6.4: Calculations Using Balanced Chemical Equations

Now that we know how to balance chemical equations, we can do calculations to determine various chemical amounts involved in reactions. Now, we need to recognize that we can use ratios of the coefficients as conversion factors to convert from one compound to another.

Example: How many grams of chlorine are needed to react with 0.245 g of hydrogen to give HCl? How much HCl is formed?

1) Write the balanced chemical reaction:

H₂ + Cl₂ → 2 HCl

2) Use what we know to calculate stepwise towards our answer. Never try to figure out a single equation of a single method to solve these equations. Always take it a step at a time and look for common methodologies in the steps.

We need to use the given mass of hydrogen to find out the mass of chloride we need. Since the coefficients in the balanced chemical equation are really ratios of moles, we will need to convert mass H₂ to moles of H₂, then we will convert to moles of Cl₂ and finally convert moles of Cl₂ to grams of Cl₂. We see two types of conversion factors we will need. We can use the molar mass of the two compounds to convert from g to moles and vice versa. Then, we can use the stoichiometric coefficients as a conversion factor to change moles of H₂ to moles of Cl₂. We will do this in a single extended fraction as we have seen before.

$\begin{array}{|c|c|c|c|} 0.245g\;H_2 & 1 mol\; H_2 & 1 Cl_2 & 70.90g\;Cl_2 \\ \hline & 2.016g\;H_2 & 1 H_2 & 1 mol\; Cl_2 \end{array} = 8.65g\;Cl_2$

Some of you may wish to do this a step at a time so a more complex (and easier to mess up) method is given here.

First convert the grams of H₂ to moles of H₂ using the molar mass of H₂.

$\begin{array}{|c|c|} 0.245g\;H_2 & 1 mol\; H_2 \\ \hline & 2.016g\;H_2 \end{array} = 0.122 mol \; H_2$

Now find the moles of Cl₂ according to the stoichiometry:

#mol Cl₂ = #mol H₂ = 0.122 mol.

Now convert moles of Cl₂ to grams of Cl₂ using the molar mass of Cl₂.

$\begin{array}{|c|c|} 0.122 mol\;Cl_2 & 70.90g\;Cl_2 \\ \hline & 1 mol\;Cl_2 \end{array} = 8.65g \;Cl_2$

Finally, we can find the mass of HCl produced using the same technique, starting from the H₂ since it is all used up, according to the question.

$\begin{array}{|c|c|c|c|} 0.245g\;H_2 & 1 mol\;H_2 & 2 HCl & 36.46g\HCl \\ \hline & 2.016g\;H_2 & H_2 & 1 mol\;HCl \end{array} = 8.90g \;HCl$

In this particular problem, we could have just added the mass of H₂ to the mass of Cl₂ since we know that the amounts itemized here are both all used up.

0.245 g + 8.65 g = 8.90 g.

As you can see, there may be more than one way to solve any particular problem we encounter in chemistry. It’s important that you learn to solve by understanding the steps involved rather than by memorizing a sequence of operations to perform. By working out the solution, rather than memorizing, you will be better able to solve new problems that are not quite the same as ones you might have seen before.

3.6.5: Limiting Reagent Calculations

In the previous example, we were only given a single chemical amount and the question told us that it was all used up. So the calculations were simplified somewhat from what we may encounter in general. A more general question might give you an amount for more than one particular chemical and it will be up to you to determine which of the reactants will be all used up. This type of calculation is called a limiting reagent calculation.

The limiting reagent is the reagent that is used up first in a reaction. Obviously, once one of the reagents (a.k.a., reactant) is used up, the reaction will stop and any other reactants not yet reacted will remain left over. We can determine from the stoichiometry whether a given initial amount of a chemical is in sufficient quantity to react or whether it will be used up before the other chemicals are finished reacting. If all the chemicals are completely used up at exactly the same time the initial reaction mixture was said to be composed of a stoichiometric mixture of the reactants. This rarely occurs unless carefully planned. It is not even a desirable situation in many cases.

Example: Consider 3 moles of SO₂ reacting with 2 moles of O₂ to give SO₃

What is the limiting reagent?
What is the maximum amount of SO₃ that can form?
How much of the remaining reactant is left after the reaction is completed?

1) Balance the reaction: 2 SO₂ + O₂ → 2 SO₃.

The stoichiometric coefficients tell us the ratio of moles of each reactant needed. In this case for every two moles of SO₂, we need 1 mole of O₂. Our initial conditions are given as 3 moles of SO₂ and 2 moles of O₂

Trial and error is often the best way in this type of situation.

a) Let us try this. Assume that O₂ is the limiting reagent.

If we are to use up all of 2 moles of O₂ , we need to determine the moles of SO₂ we will need.

$\begin{array}{|c|c|} 2 mol\;O_2 & 2 mol\;SO_2\\ \hline & 1 mol\;O_2\end{array} = 4mol \; SO_2\;needed$

But we only have 3 moles of SO₂ so O₂ can not all be used up. It is not the limiting reagent. The only other choice is the SO₂.

So, if SO₂ is limiting, We can calculate how much O₂ is needed:

$\begin{array}{|c|c|} 3 mol\;SO_2 & 1 mol\;O_2\\ \hline & 2 mol\;SO_2\end{array} = 1.5mol \; O_2\;needed$

b) Now, we can calculate the maximum amount of product if all 3 moles of SO₂ is used up:

$\begin{array}{|c|c|} 3 mol\;SO_2 & 2 mol\;SO_3\\ \hline & 2 mol\;SO_2\end{array} = 3mol \; SO_3$

c) Since, from a) we see that 1.5 mol of O₂ is use up, we can easily determine that 0.5 mol of O₂ is remaining after the reaction is complete.

The example given here is simplified in that all measurements are given in moles. We always must use moles when working with the stoichiometric coefficients. If our question gives us grams or volumes and concentrations, we must convert to moles first before proceeding. We will see later that Dalton’s Law says that we can use partial pressures in place of moles for these ratio type calculations since pressure is proportional to moles (for an ideal gas).

3.6.6: Other Calculations

Here is another example of the type of calculations you may need to do in your chemistry problems. In this case, we are not interested in a reaction but in calculating concentrations in solutions. This turns out to be more an exercise in algebra than in chemistry. Note below that the units of concentration are requested in moles/dm³. We can determine easily that 1 dm³ = 1 L, so we are actually looking for the commonly used concentration term Molarity (mol L^-1) but expressed as SI units.

20.36 g of NaCl is dissolved in sufficient water to form 250. mL of solution. What is the concentration in moles per dm³?

Molar Mass of NaCl = 58.44 g mol^-1

$\begin{array}{c} \mathrm{20.36 g NaCl} \\ \hline \mathrm{58.44 g/mol}\end{array} = 0.3484 \mathrm{ mol NaCl}$

This is dissolved to form 250 mL of solution so:

$\begin{array}{c} \mathrm{0.3484 mol NaCl} \\ \hline \mathrm{0.250 L solution}\end{array} \mathrm{ = 1.39 M NaCl}$

where M represents the units mol/L or mol dm^-3.

Example: What volume of Conc. H₂SO₄ (98% by mass, density=1.84 g cm-3) is required to make 10.0 L of 0.200 M H₂SO₄ solution?

A common confusion here is the word concentrated (conc.). This does not mean pure, it merely means that there is a high concentration of H₂SO₄ in the solution. In this case, for every 100g of conc. H₂SO₄, only 98 g is actually H₂SO₄, the rest is probably water.

We can get the moles of the chemical H₂SO₄ that we need using the volume and concentration of the final solution.

$n(H_2SO_4) = \begin{array}{|c|c|} 10 L (final solution) & 0.200 mol H_2SO_4 \\ \hline & 1 L (final solution) \end{array} = 2.00 mol H_2SO_4$

Now we can determine the mass of H₂SO₄ using the molar mass.

$m(H_2SO_4) = \begin{array}{|c|c|} 2.00 mol H_2SO_4 & 98.08 g H_2SO_4 \\ \hline & 1 mol H_2SO_4 \end{array} = 196 g H_2SO_4$

(NOTE: 98.08 is not the 98% mentioned in the question. It’s the molar mass of pure H₂SO₄, not conc. H₂SO₄.)

Now, we can get the mass of conc H₂SO₄ that we need to measure out in order to get the necessary amount of the sulfuric acid using the mass percent as a conversion factor.

$m(conc\; H_2SO_4) = \begin{array}{|c|c|} 196 g\; pure\;H_2SO_4 &100 g\; conc\; H_2SO_4 \\ \hline & 98 g\;pure\; H_2SO_4 \end{array}\\ \;\;\;\;\;\;\; = 2.0\times10^2 g\;conc\; H_2SO_4$

Finally, we can use the density of the conc. sulfuric acid solution to determine the volume we will need to measure out.

$V(conc\; H_2SO_4) = \begin{array}{|c|c|} 2.0\times10^2 g\;conc\; H_2SO_4 &1 mL\; conc\;H_2SO_4 \\ \hline & 1.84 g\;conc\; H_2SO_4\end{array}\\ \;\;\;\;\;\;\; =1.1\times10^2 mL\;conc\; H_2SO_4$

This whole process could have been done more efficiently using the extended fraction formalism, as follows:

$\begin{array}{|c|c|c|c|c|} 10 L (final solution)&2.00 mol H_2SO_4 & 98.08 g H_2SO_4&100 g\; conc\; H_2SO_4 &1 mL\; conc\;H_2SO_4 \\ \hline & 1 L (final solution)&1.00 mol H_2SO_4 & 98 g\;pure\; H_2SO_4& 1.84 g\;conc\; H_2SO_4\end{array}\\ \;\;\;\;\;\;\; =1.1\times10^2 mL\;conc\; H_2SO_4$

You need to get into the habit of writing out the units completely as I have done here to ensure that they cancel properly. Notice for instance that simply writing g for grams is not sufficient as we have grams of H₂SO₄ and also grams of “conc H₂SO₄“, which is not the same thing. We have litres of the final solution as well as litres of the initial concentration of H₂SO₄to consider. Always write out the full units (at least at first) to be really sure you are doing the math correctly. Eventually, you may find that taking shortcuts with units is OK but beware; unit conversion errors have a nasty habit of coming back to bite you when you least expect it.

End of chapter questions.

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License