10 Solids

10.1: Classification of Solids

There are several categories of classification used to group different kinds of solids. Some focus on the way the solids are bonded together, others focus on the repeatability of the solid structure. We will try to clarify these different categorizations with examples so that the student will be able to identify the category (ies) where any given solid fits.

10.1.1: Classification Based on the Range of Atom Repeatability

The repeatability of a solid refers to the ability to use atom arrangements in one location to predict atom arrangements in other locations.  In a perfectly repeatable structure, there exists a small set of atoms (called the unit cell), whose positions can be used to predict atom positions in the whole solid structure by means of repeating the unit cell positions according to the particular symmetry of the crystal itself.  In solids that have long-range repeatability, the atom positions can be accurately described over an extended distance, using just the unit cell structure.  In solids that do not have long-range repeatability, the unit cell structure may at best give us an idea of the kind of bonding involved in the solid but irregularity in the arrangements of the atoms will quickly frustrate any attempt to predict atom positions any distance away from the “unit cell”.

Crystalline Solids have long-range repeatability.  They contain atoms or molecules bonded together in a regular pattern.   A good example of these is quartz crystals (emperical formula: SiO₂) where each silicon is bonded to four oxygens, which in turn are bonded to two silicons in a continuous covalent network extending in three dimensions.  You can find these crystals in your quartz watch.  The crystal vibrates at a fixed frequency when electric charge is placed across certain directions.  Your watch uses the vibrations to keep time.

Amorphous solids (or glasses) have at best, short-range repeatability.  They are made up of atoms or molecules with little or no regular arrangement.  Quartz that has been melted into liquid and cooled (moderately) rapidly will form glass.  Quartz glass is used for applications like windows on lasers, and fine optics like Zeiss lenses.  Many different solids can exist in both crystalline and amorphous forms.
For a particular solid (e.g., quartz), the glass and the crystal will have the same type of bonding, the same empirical formula and some very similar physical properties.  The glass and crystalline variances can also have some properties that are quite different.  For example,  quartz crystals do not transmit light equally in all directions because of the crystallinity whereas the quartz glass will transmit light equally in all directions.  Quartz glass will not fracture the same way as quartz crystals.

Semi-crystalline solids have medium-range repeatability, not true long-range repeatability but some repeatability over the short range (i.e., not totally amorphous).  Such semi-crystalline materials have different properties from both glasses and crystals.  Liquid crystals, for example, have medium range-repeatability.

10.1.2: Classification Based on Bond Type

Solids that are crystalline, semi-crystalline and amorphous can be made from all different types of atoms that are bonded in different ways.  Thus, another way to classify solids is to look at the type of bonds holding the solid together.  These different types of bond possibilities are listed here.

Molecular solids consist of molecules that are held together by week intermolecular forces.  A prime example of this is sulfur.  Molecules of sulfur (S₈) are held together by intermolecular forces far weaker than the covalent bonds that keep the atoms within each molecule.  This type of solid may not have a high melting point; none are higher than 400°C.  Molecular solids may be fairly soft, i.e., they can be easily distorted or warn away by physical force of some kind because of the relative weakness of the intermolecular forces that are holding the solid together.

Covalent (network) solids are made of atoms that are covalently bonded together to form one continuous network of covalently bonded atoms.  One could almost think of this type of solids as macroscopic molecules (big enough to see).  Diamonds are a prime example of such solids.  This type of solid tends to have a high melting point and are normally quite hard.  For example, diamond melts at 3600℃.  Network solids are not all crystalline.  Quartz, mentioned above, is a network solid in crystalline and in amorphous forms.

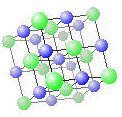Ionic solids contain ions of opposite charge which hold together with electrostatic (Columbic) interactions.  A good example of this is sodium chloride (table salt).  The crystal structure of NaCl is shown on the right. The atoms of Na⁺ alternate with atoms of Cl^– such that each positive ion has neighboring negative ions and vice versa.  Ionic solids tend to have a melting point that ranges from quite low  to moderately, depending on the strength of the ionic bond.
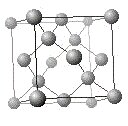
Metallic solids are made up of metal atoms, whose loosely held outer electrons are somewhat free of their positive cores and form a continuous dissociated sea of negative charge binding the positive cores together.   Metallic bonds are generally non-directional, which means the solid will hold together even if the material is distorted significantly.  Metals can be reshaped by striking (malleable) or drawn through small openings (ductile) the way copper is formed into wires.   Metals can have low melting points and also tend to be soft.   The crystal structure of copper is shown in the model on the right.

10.1.3: Classification Based on the Dimensionality of the Solid

In Network solids, the atoms are all held together with covalent bonds such that there are no small identifiable units (molecules or clusters) within the structure.  The array of atoms extends continuously throughout the whole solid.  Network solids can form in different dimensionalities.

One dimensional networks (plastic),  These tend to form very soft plastic or even waxy/tar-like solids.  One-dimensional solids generally do not form crystals by virtue of the easy entanglement of the long “molecules”, which makes long-range repeatability improbable.  Some such molecules are long enough theoretically to be measurable macroscopically individually by mass or size.

Two dimensional networks (graphite).  These have planes of atoms that can easily slide over each.  For example, graphite is used as lubricant.

Three dimensional networks (diamond).  These tend to be very strong and hard, and can have a very high melting point.  Ceramics used to line smelters and as a heat shield on spacecraft are three-dimensional network solids.

10.2: Lattices

For the rest of this section, we will restrict our discussion to crystalline solids.  These have atoms or groups of atoms arranged in a regular array or lattice in three dimensions.  We should look at the term lattice and define it closely.

A lattice is a mathematical abstraction that describes the way the atoms or groups of atoms are repeated in space. We must not mistake the diagrams that follow for arrangements of atoms.  The following details only the mathematical points that make up the lattice.  Let’s first look at simple lattices in two dimensions.  We will then extend the discussion into three dimensions.

A unit cell is any subset of the lattice that contains enough information that the whole lattice can be rebuilt by starting with just the unit cell and translating or rotating according to the symmetry of the lattice.  The simplest unit cell is one that contains the least number of points and needs only translations along the cell edges to repeat the pattern.  Unit cells contain the “stuff” we want to repeat.  The famous Dutch artist Escher regularly created paintings that were made up of repeating units.

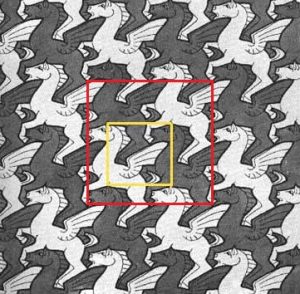
Can you find the unit cell here?  The red box shows a unit cell that can be used to replicate the rest of the painting by using it as a virtual stamp to make the rest of the painting by stamping each time some unit multiples of with and height away from the original.  The yellow box here shows the smallest unit cell but not necessarily the easiest to use.  This primitive unit cell would require an additional symmetry operation, i.e., that each motion by one cell length horizontally or vertically be accompanied by an inversion of the light gray and the dark gray shading.

10.2.1: 2-D Lattices

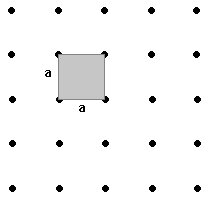
The simplest of the 2d lattices is the square lattice.  We can choose a unit cell (the repeating unit) such that it has equal length sides (a) and an angle of 90º.  We can locate the cell wherever we please.  One choice puts one point of our lattice at each corner of the cell.  We could have alternatively, placed the unit cell such that one point was at the centre.  Less conveniently, we could have placed the cell with one point anywhere within its boundaries.  In all cases, there is 1 point for each unit cell (the first choice saw only 1/4 of each vertex actually inside the boundaries of the unit cell.

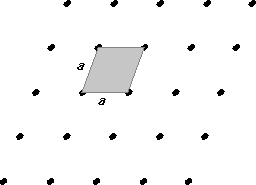
A hexagonal lattice contains points arranged such that a unit cell can be drawn with angles of 60º and all sides of length a.  A complete hexagon is drawn for visual effect.

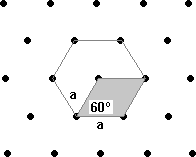
Another type of lattice is a rectangular lattice.  The unit cell in this lattice would have angles of 90º like the square lattice but would have different length sides a and b.

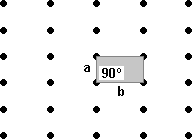
The Rhombic lattice has a unit cell with equal length sides but an angle that is neither 60º nor 90º.

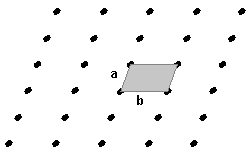
Finally, the least symmetric of all two dimensional lattices is the fully rhombic (parallelogram) lattice.  Here, the angles are neither 90º, nor 60º and the sides of the unit cell are not all the same length.

10.2.2: 3-D Lattices

In two dimensions, there are only these 5 lattices.  In three dimensions, the lattices are called point-group lattices or Bravais lattices.  There are 14 Bravais lattices.  For our purposes, we will look only at two basic types of three dimensional lattices, cubic lattices and hexagonal lattices.

Cubic Lattices

There are several cubic lattices.  We will focus on three of them here.  These include the simple cubic lattice, the body-centered cubic lattice and the face-centered cubic lattice.

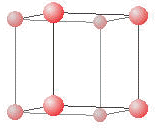
The simple cubic lattice has only one 1 lattice point within each unit cell.   Notice that there seem to be eight spheres (points) associated with this unit cell (the box).  It is important to recall that only the part of the point that is inside the box is truly in the unit cell.  Since only 1/8 of each corner point is actually inside the unit cell,  there is really only 8 × 1/8 = 1 point for each unit cell.  Each lattice point has 6 nearest neighbors (four are shown here).  We define this as the coordination number.

A body centered cubic (bcc) unit cell has two points associated with it.  The 8×1/8=1 corner points and the one point in the centre of the cell.  Monatomic bcc lattices obviously have a coordination number of 8

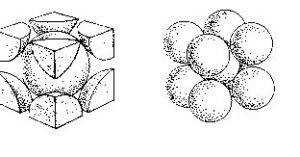
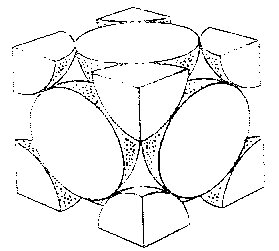
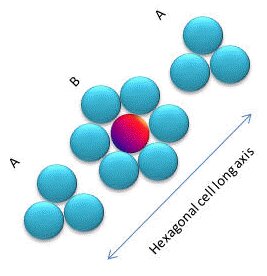
The face centered cubic (fcc) lattice, has 4 points associated with its unit cell.  The 8×1/8=1 corner points and 6×1/2=3 face-centered points.  These points are only half inside the box and so we only get to claim half of the point for the unit cell.  An alternate name for the face centered cubic lattice is the cubic closest packing (ccp).  This is one of two closest-packing arrays where the points have packed in the most efficient (least volume) way possible.   The 3d model on the left shows the lattice points.  the diagrams on the left show two different views of monatomic structures that use fcc packing.  The far left one shows stacking layers abc and allows us to count the nearest neighbors to a particular atom (red).  The layers in CCP are actually not along the cube sides, they are perpendicular to the cube body diagonal as indicated in the right-most image, where the colors correspond to the colors in the left-most image.  The colored atoms in the image all touch the red atom in the centre of the B layer, so we see that the coordination number for this type of crystal is 12.  The other diagram shows the fraction of each atom that is actually inside the unit cell.

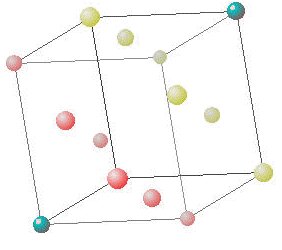
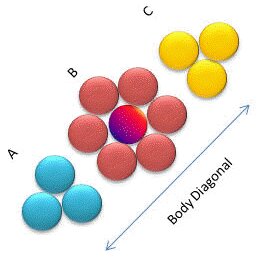
The other major class of three dimensional crystal lattice is one that gives a hexagonal unit cell.  Like the hexagonal 2d array, the hexagonal 3d array has some angles of 60º with 90º directions perpendicular to that.

The unit cell of the hexagonal closest packing (hcp) array has two sides of length a, separated by angles of 60º and one side of length b at an angle of 90º to the two others.  This is the other closest packing array where the points are packed in their most efficient (least volume) way possible.  You can see that this unit cell has one point fully inside and 8 apex points 4 have 8.33% inside and 4 have 16.66% inside the unit cell which between them add up to one point inside.

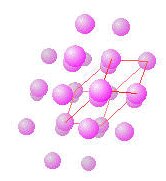
hcp lattice with unit cell highlighted.

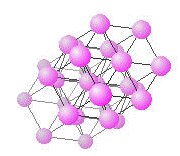
hcp lattice with closest neighbouring points highlighted.

10.3: Metals

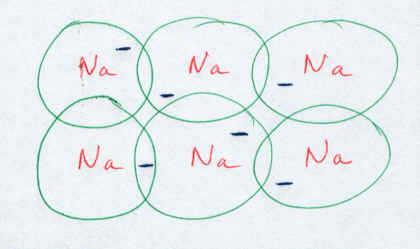
In metals, the valence electrons tend to be quite loosely held.  These outer electrons are easily lost or shared with little effort.  Let’s consider a solid piece (crystal) of metal (for example, Sodium) where the outer orbital (s) of the neighbouring metal atoms all overlap each other.

4

We see here that the loosely held electrons can freely roam from atom to atom with no hindrance.  In effect, the sodium cores (+1 charge) are floating in a sea of electrons (negative charges).  This allows for several properties characteristic of metals.

1. malleable: if you strike the metal, the atoms can slide over each other without breaking any actual bonds.  Gold can be hammered into gold-leaf (10 atoms thick) without fracturing the metal.
2. ductile: metals can be drawn through a small opening as in copper is pulled through a hole in a steel plate to form wire.
3. lustrous: Because of the large number of overlapping orbitals, the energy levels are very closely spaced such that photons of a large range of frequencies are absorbed and instantly re-emitted (alias, reflected).
4. electrically conductive: since the electrons are quite mobile, metals easily conduct electricity.

Because of the non-directional bonding that occurs in metals, atoms of metal crystals tend to pack in a very efficient manor.  The two most efficient packing methods are ones that follow the hcp and ccp lattices, which have identical packing efficiencies (about 74% of the crystal volume is actually atoms).  A third common packing method of metals is bcc, which is not as efficient as hcp or ccp.

Most metals tend to have monatomic crystal structures.  They have only one atom associated with each crystal lattice point.  This makes it easy to compare atomic positions with crystal lattice positions.  We can choose to exactly overlap the atomic positions with the lattice points.  This (very common) choice leads to confusion by some who think that the atom is the lattice point.  This is merely a coincidental choice made in monatomic crystals for the sake of simplicity.

Iron tends to pack in a monatomic bcc crystal structure.  “Monatomic” means that there is only one atom associated with each lattice point in the crystal lattice.  For convenience, we tend to put the atoms exactly on the lattice points but this is not necessary as long as all lattice points share exactly the same spatial arrangement with one and only one atom per point.

Iron’s atoms have 8 nearest neighbors and thus have a coordination number of 8.

Copper tends to pack in a monatomic ccp arrangement, where each atom is associated with its own lattice point in the ccp lattice.  Recall that this is the face centered cubic lattice array.  Each atom has a coordination number of 12, i.e., there are 12 nearest neighbors for each atom of copper.

Zinc atoms tends to arrange  themselves in a monatomic hcp crystal structure where one and only one atom of Zn is associate with each lattice point in the hcp crystal lattice.  Each Zn atom has 12 nearest neighbours, i.e., the coordination number is 12.

10.4: Ionic Solids

Ionic solids do not form monatomic crystal structures but many still form closest packing arrangements since the ionic bonds are non-directional.  To envision the crystal structure of many ionic crystals, we need to look at many factors, including the relative size of the positive and negative ions and the relative number of them.   Lets look at the packing arrangement of the atoms in a close-packing structure.  We see that there are two types of spaces between the atoms.

1. Tetrahedral spaces exist where four atoms come together (atom from layer b on top of triangular hole from layer a).
   
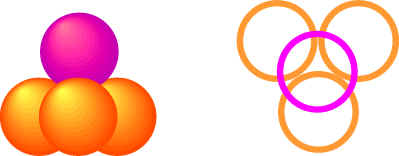
2. Octahedral spaces occur where six atoms come together (three atoms from layer b surround a hole from layer a)
   

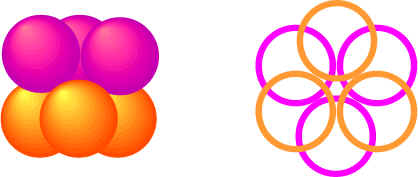
Since the octahedral holes are larger than the tetrahedral ones, they can accommodate larger cations, relative to the size of the anions.

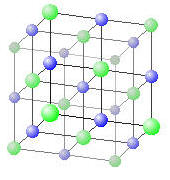
Take Sodium chloride.  The chloride ions (green in the model below) are significantly larger than the sodium ions (blue) and we can consider the sodium chloride to be a closest packing fcc array of chloride ions with sodium ions filling the octahedral holes between them.  NaCl  has a diatomic fcc crystal structure, since there are two “atoms” associated with each lattice point.

.

The calcium fluorite (CaF₂) structure has Calcium cations in the tetrahedral spaces between fluoride ions. (The Ca²⁺ is blue and the F- is green)

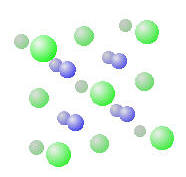
Note that there are different ratios of holes to atoms for tetrahedral (2 holes : 1 atom) versus octahedral (1:1) holes.

10.5: Cell Calculations

Once we understand the structure of crystals, we can do many different types of calculations using this information.  For the sake of simplicity, we will restrict ourselves to calculations involving monatomic (metallic) cubic crystal structures.

Let’s first look at the geometry of a cube.

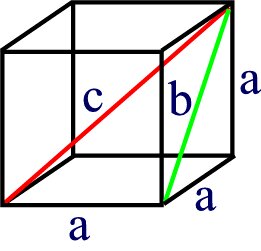
The cube has all sides of length a.  Each face has a face diagonal of length b and the body diagonal has a length c.  Using Standard trigonometric relationships, we can easily derive the following relationships.

b² = 2 a²   or    b = (2 a²)^1/2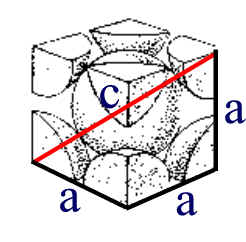

and

c² = 3 a²   or    c = (3 a²)^1/2.

Thus, if we are dealing with a Body centered cubic structure, the body diagonal is the only cell direction that is a simple multiple of atom radii.

Here, we see that c is equal to four atomic radii.  c = 4 r    or    r = c/4

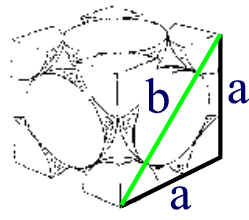
By the same logic, in a face centred cubic structure, the face diagonal would be equal to 4 times the atomic radius.

b = 4 r      or      r = b/4

Example:

The length of a unit cell of iron (monatomic bcc) is measured to be 286 pm using x-ray diffraction.  What is the size (radius) of the iron atom?

Since Fe is monatomic bcc, we have

Example:
Vanadium has a unit cell structure like iron.  x-ray diffraction shows the unit cell dimension to be 305 pm.  What is the density of Vanadium?

The 2 atoms comes from 2 atoms in a bcc unit cell.

Of course, this kind of example can be carried out on any unit cell; all you need to know is the cell dimension and the number of atoms inside the cell.  We discussed a few simple cubic cells for close-packed structures but there are other structures that also fit in a cubic cell structure

For example,

Diamond has 8 atoms inside a cubic unit cell.

NaCl has a FCC structure (of 4 Cl ions) with the Na⁺ ions in the octahedral holes (1:1) ratio so since FCC has 4 Na⁺ ions in each unit cell too.

Other structures can be handled in similar ways.