18. Kinetics Problems
Kinetics Problems
1. The rate of disappearance of nitrogen at a point in the synthesis reaction ![]() is
is
![]()
Calculate the rate of change of ![]() and
and ![]() at that same point.
at that same point.
[-1.5×10¯5 mol/L.s, +3.4×10¯5 mol/L.s]
2. n-propyl bromide reacts with thiosulfate to form n-propyl thiosulfate according to the following reaction. The reaction takes place in a solvent made of a mixture of water and ethanol.
![]()
The initial rates of the reaction under a variety of conditions is listed below. What is the order of reaction with respect to each reactant? What is the overall order of reaction? What is the rate constant for the reaction?
![Rendered by QuickLaTeX.com \[ \begin{array}{ccc} \hline \mathrm{[C_3H_7Br]/mol\;L^{-1}}&\mathrm{[S_2O_3^{2-}]/mol\;L^{-1}}&\mathrm{Initial\;rate/mol L^{-1} s^{-1}}\\ \hline 0.060&0.100&10.0\times10^{-6}\\ 0.060&0.050&5.0\times10^{-6}\\ 0.040&0.030&2.0\times10^{-6}\\ 0.040&0.050&3.3\times10^{-6}\\ 0.050&0.030&2.5\times10^{-6}\\ \hline \end{array} \]](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-a3a5db428f79015be703b50e7f1de2fa_l3.png)
[1, 1, 2, 1.7×10-3,mol-1L s-1]
3. The decomposition of sulfuryl chloride ![]() is a first order reaction:
is a first order reaction:
![]()
The rate constant at 593 K was found to be 2.2×10-5 s-1. If the initial concentratio of the ![]() was 0.020 mol/L, calculate
was 0.020 mol/L, calculate
- the initial rate of the reaction
![Rendered by QuickLaTeX.com \mathrm{[SO_2Cl_2]}](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-ff3e9572b6aadc67f54fbfa33845fd1c_l3.png) after
after 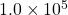 seconds
seconds- the rate of the reaction after
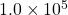 seconds.
seconds.
[4.4×10-7 mol /L.s, 2.2×10-3 mol/L, 4.9×10-8 mol/L s]
4. What is the half-life for the decomposition of sulfuryl chloride under the conditions described in problem 2?
[3.2×104 s]
5. Dinitrogen pentoxide decomposes into nitrogen dioxide and oxygen gas in a first order reaction:
N2O5(g) → 2 NO(g) + ½ O2(g)
Suppose the initial concentration of the dinitrogen pentoxide is 0.010 mol/L. Sketch a graph showing how the concentrations of all three species vary with time as the reaction proceeds to completion.
6. At a temperature of 45℃, the decomposition of dinitrogen pentoxide described in question 5 is 2.5×10-4 s-1. If the initial concentration of N2O5 is 0.100 mol/L, calculate the concentrations of N2O5, NO2 and O2 after 6.0×103s.
[2.2×10-3 mol/L, 1.6×10-2 mol/L, 3.9×10-3 mol/L]
7. The radioactive decay of 14C is a first order process with a half-life of 5700 years. What is the rate constant for the decay expressed in units of s-1?
[3.9×10-12 s-1]
8. Gaseous tetrafluoroethylene C2F4 dimerizes at high temperature according to the following equation:
2 C2F4(g) → C4F8(g)
The reaction is second order in C2F4 and at 600 K, the rate constant is 0.052 L mol-1 s-1 . If the initial concentration is [C2F4] = 0.0100 mol/L, calculate [C2F4] and [C4F8]: a, after 400s, and b, after 2000 s.
[a, 8.28×10-3 mol/L, 8.6×10-4 mol/L. b, 4.9×10-3 mol/L, 2.55×10-3 mol/L ]
9. For the dimerization of gaseous tetrafluorethylene, C2F4, under the conditions described in the previous question, how long will it take for 95% of the C2F4 to be used up?
[3.6×104 s]
10. The rate constant, at a temperature of 400 ℃ for the second order reaction H2(g) + I2(g) → 2HI(g) is 2.34×10-2 mol-1 L s-1. The activation energy is 150. kJ/mol. Calculate the pre-exponential factor and the rate constant for the reaction at 450℃.
[1.0×1010 mol-1 L s-1, 0.148 mol-1 L s-1]
11. Ethyl bromide, C2H5Br, when heated in the gas phase, decomposes in a first order reaction to produce ethylene, C2H4, and hydrogen bromide, HBr.
a. write a balanced chemical reaction for this process.
b. the activation energy for this reaction is 92.3 kJ/mol and the pre-exponential factor is 6.8×1011 s-1 . What is the value of the rate constant at 450. K?
c. If 0.0100 mol/L of ethyl bromide is introduced into a flask at 450. K, how long will it be before 75% of it has decomposed?
[b, 13 s-1, c, 0.11 s]
12. The conversion of ethanol to acetaldehyde in the body is a zero order reaction, with a typical rate constant of 4×10-3 mol L_1hour-1. If the volume of fluids in the body is about 40 L, estimate the initial molar concentration and mass fraction of ethanol in the body fluids caused by rapidly ingesting 10 g of pure ethanol, if the ethanol is dissolved uniformly. Calculate the time required for the concentration of ethanol to become zero (within 1 sig fig, of course).
13. A reaction has an activation energy of 40 kJ/mol and the enthalpy change in the reaction is -100. kJ/mol. Draw a sketch of energy as a function of reaction coordinate, showing the relationship between the energy of the reactant and the products. Estimate the activation for the reverse reaction.
[140 kJ/mol]
14 The half-life was measured for a reaction A → Products for various initial concentrations of A at a certain temperature, with the results shown in the table below. what is the order of this reaction?. write the rate equation and calculate the value for the rate constant.
![Rendered by QuickLaTeX.com \[\begin{array}{|c|c|}\hline \mathrm{[A]\; /\; mol\;L^{-1}}& \mathrm{t_{1/2} \;/\; minutes} \\ \hline 0.50 & 25.\\ 1.00 & 50.\\ 2.00 & 100.\\ \hline \end{array}\]](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-2daf0ad674e4c41703301b4e6c77fbd0_l3.png)
[0 order, 0.010 mol L-1 min-1]
15. The oxidation of I¯by peroxodisulfate S2O82¯is a slow reaction described by
2 I¯(aq) + S2O82¯(aq) → I2(aq) + 2 SO42¯(aq)
Thiosulfate ion, S2O32¯(aq), and some starch are added to the solution. as fast as it is produced, the iodine reacts with the thiosulfate ion:
I2(aq) + 2 S2O32¯(aq) → 2 I¯(aq) + S2O62¯(aq)
When the thiosulfate ion is completely used up, the iodine concentration builds up and forms a blue coloured complex wit the starch. In a particular experiment, the solution at the beginning of the reaction is prepared by mixing the following:
20.0 mL of 0.125 M (NH4)2S2O8 solution
20.0 mL of 0.22 M KI solution
1.0 mL of 0.060 M Na2S2O3 solution
Starch indicator.
The solution turned blue 65 seconds after mixing. Estimate the initial value of ![]() in the first reaction by calculating the value of
in the first reaction by calculating the value of ![]() in the first 65 seconds.
in the first 65 seconds.
[1.1×10-5 mol L-1 s-1]
16. For the isomerization of cyclopropane to propene, discussed in section 18.4.1 Arrhenius Equation, the activation energy is 275 kJ/mol. Use the Thermodynamic data table to calculate ![]() and the equilibrium constant at 25 ℃ for the reaction. Draw an energy diagram to scale indicating the relationship between the activation energy and the enthalpy change for the reaction
and the equilibrium constant at 25 ℃ for the reaction. Draw an energy diagram to scale indicating the relationship between the activation energy and the enthalpy change for the reaction
[-32.8 kJ/mol, 1.8×107]

