13.2 Notes Payable
Recognition and Measurement of Notes Payable
A note payable is an unconditional written promise to pay a specific sum of money to the creditor, on demand or on a defined future date. It is supported by a formal written promissory note. These notes are negotiable instruments in the same way as cheques and bank drafts.
Notes payable are initially recognized at the fair value on the date that the note is legally executed (usually upon signing). Subsequent valuation is measured at amortized cost using the effective interest rate.
Characteristics
A typical note payable requires payment of a specified face amount, also called principal, and interest, that is paid as a single lump sum at maturity, as a series of payments, or as a combination of both. (This topic will be discussed later in this chapter.)
Secured notes payable identify collateral security in the form of assets belonging to the borrower that the creditor can seize if the note is not paid at the maturity date.
Notes may be referred to as interest bearing or non-interest bearing:
- Interest-bearing notes have a stated rate of interest that is payable in addition to the face value of the note.
- Notes that are zero-bearing or non-interest bearing do not have a stated rate of interest. Although, while they may appear at first glance not to have any interest, there is always an interest component embedded in the note. The interest component will be equal to the difference between the borrowed and repaid amounts.
Cash payments vary and can be a single payment of principal and interest upon maturity, or payment of interest only throughout the term of the note with the principal portion payable upon maturity, or a mix of interest and principal throughout the term of the note.
Transaction Costs
It is common for notes to incur transaction costs, especially if the note payable is acquired using a broker as they will charge a commission for their services. For a company using either ASPE or IFRS, the transaction costs associated with financial liabilities, such as notes payable that are carried at amortized cost, are to be capitalized, meaning that the costs will reduce the note payable amount. If the debt is subsequently classified and measured at its fair value, the transaction costs are to be expensed. This is referred to as the fair value option and will be discussed later in this chapter.
Classification
Notes may be classified as short-term (current) or long-term payables on the SFP/BS:
- Short-term notes are current liabilities payable within the next 12 months, or within the business’s operating cycle if longer than 12 months.
- Long-term notes are notes that do not meet the definition of a current (short-term) liability. For example, notes with due dates greater than one year.
As previously discussed, the difference between a short-term note and a long-term note is the length of time to maturity. Also, the process to issue a long-term note is more formal, and involves approval by the board of directors and the creation of legal documents that outline the rights and obligations of both parties. These include the interest rate, property pledged as security, payment terms, due dates, and any restrictive covenants. Restrictive covenants are any quantifiable measures that are given minimum threshold values that the borrower must maintain. Additionally, restrictions on minimum working capital (current assets minus current liabilities), management remuneration, capital expenditures, or dividends paid to shareholders are often found in covenant conditions. Maintenance of certain ratio thresholds, such as the current ratio or debt to equity ratios, are all common measures identified in restrictive covenants.
As the length of time to maturity of the note increases, the interest component becomes increasingly more significant. As a result, any notes payable with greater than one year to maturity are to be classified as long-term notes and require the use of present values to estimate their fair value at the time of issuance. A review of the time value of money, or present value, is presented in the following to assist you with this learning concept.
13.2.1. Long-Term Notes Payable, Interest, and the Time Value of Money
Long-term notes payable are to be measured initially at their fair value, which is calculated as the present value amount. But what is present value? It is a discounted cash flow concept, which we will discuss next.
It is common knowledge that money borrowed from a bank will accrue interest that the borrower will pay to the bank, along with the principal. The present value of a note payable is equivalent to the amount of money deposited today, at a given rate of interest, which will result in the specified future amount that must be repaid upon maturity. The cash flow is discounted to a lesser sum that eliminates the interest component—hence the term discounted cash flows. The future amount can be a single payment at the date of maturity, a series of payments over future time periods, or a combination of both. Put into context for notes payables, if a creditor must wait until a future date to receive repayment for its lending, then note payable’s face value at maturity will not be an exact measure of its fair value today (transaction date) because of the embedded interest component.
For example, assume that a company purchases equipment in exchange for a two-year, $5,000 note payable, and that notes of a similar risk have a market rate of 9%. The face value of the note is therefore $5,000. The note’s present value, without the interest component, is $4,208.40, not $5,000. The $4,208.40 is the amount that, if deposited today at an interest rate of 9%, would equal exactly $5,000 at the end of two years. Using a variables string, the present value of the note can be expressed as:
| PV = (9% I/Y, 2N, 5000FV) = $4,208.40 |
| Where I/Y is interest of 9% each year for two years; |
| N is for the number of years that the interest is compounded; |
| FV is the payment at the end of two years’ time (future value) of $5,000. |
To summarize, the present value (discounted cash flow) of $4,208.40 is the fair value of the $5,000 note at the time of the purchase. The additional amount received of $791.60 ($5,000.00 – $4,208.40) is the interest component paid to the creditor over the life of the two-year note.
Note: The symbols PV, I/Y, N, FV, and PMT are intended to be a generic reference to the underlying variables string. Each brand of financial calculator will have its own owner’s manual that will identify the set of keys for inputting the variables above.
After issuance, long-term notes payable are measured at amortized cost. As illustrated next, determining present values requires an analysis of cash flows using interest rates and time lines
Present Values and Timelines
The following timelines will illustrate present value using discounted cash flows. Below are three different scenarios:
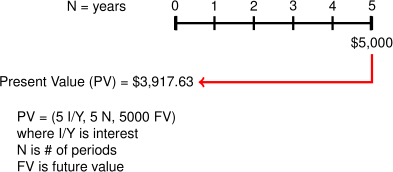
- Assume that on January 1, Maxwell lends some money to Nictonia in exchange for a $5,000, five-year note payable as a lump-sum amount at the end of five years. Notes of similar risk have a market interest rate of 5%. Additionally, Maxwell’s year-end is December 31. The first step is to identify the amount(s) and timing of all the cash flows as illustrated below on the timeline. Inputting the variables into a financial calculator, the amount of money that Maxwell would be willing to lend to the borrower using the present value calculation of the cash flows would be $3,917.63.
In this case, Maxwell would be willing to lend $3,917.63 today in exchange for a payment of $5,000 at the end of five years, at an interest rate of 5% per annum. Nictonia’s entry for the note payable at the date of issuance would be:
Notes of this nature are often referred to as zero-interest or non-interest-bearing notes. This is a misnomer, however, as all debt transactions between unrelated third parties will bear interest based on market interest rates. For example, note that Maxwell lends $3,917.63 now and collects $5,000 at the end of five years. The difference of $1,082.37 represents the interest component over the five years.
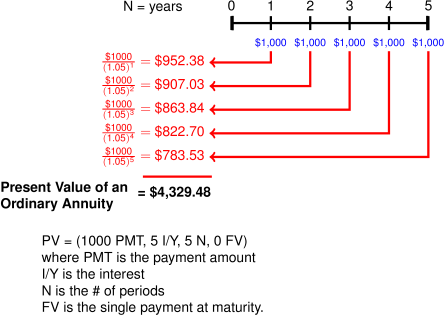
- Now assume that on January 1, Maxwell lends an amount of money in exchange for a $5,000, five-year note. The current market rate for similar notes is 5%. The repayment of the note is $1,000 at the end of each year for the next five years (present value of an ordinary annuity). The amount of money that Maxwell would be willing to lend Nictonia using the present value calculation of the cash flows would be $4,329.48, as follows:
Nictonia’s entry for the note payable at the date of issuance would be:
Note that in this example Maxwell is willing to lend more money, $4,329.48 as compared to $3,917.63, to Nictonia. Another way of looking at it would be that the interest component embedded in the note is less in this case. This makes sense as the principal amount of the note is being slowly reduced over its five-year term due to of the yearly payments of $1,000. In other words, the higher the frequency of payments, the lower the interest component will be. This is the same concept as with a mortgage owing for a house. It is common for financial advisors to say that a mortgage payment paid twice a month, instead of a single payment once a month, will result in a significant reduction in interest costs over the term of the mortgage. The bottom line is that if the principal amount owing at any time over the life of a note is reduced, there will be less interest charged overall. Another name for a note with equal payments of interest and principal is an instalment or blended payment note.
- How would the amount of the loan and the entries above differ if Maxwell received five equal payments of $1,000 at the beginning of each year (present value of an annuity due) instead of at the end of each year, as shown in example 2? The amount of money that Maxwell would be willing to lend Nictonia using the present value calculation of the cash flows paid at the beginning of the period (P/AD generic symbol) would be $4,545.95, as follows:
Nictonia’s entry for the note payable at the date of issuance would be:
Again, the interest component will be less because a payment is paid immediately upon execution of the note, which causes the principal amount to be reduced sooner than a payment made at the end of each year.
Below is a comparison of the three scenarios:
| Scenario 1 | Scenario 2 | Scenario 3 | |
|---|---|---|---|
| Single payment at maturity | Five payments of $1,000 at the end of each month | Five payments of $1,000 at the beginning of each month | |
| Face value of the note | $5,000 | $5,000 | $5,000 |
| Less: present value of the note | 3,918 | 4,329 | 4,546 |
| Interest component | $1,082 | $671 | $454 |
Note that the interest component decreases for each of the scenarios even though the total cash repaid is $5,000 in each case. This is due to the timing of the cash flows, as discussed earlier. In scenario 1, the principal is not reduced until maturity and interest would accrue for the full five years of the note. In scenario 2, the principal is being reduced at the end of each year, so the interest will decrease due to the decreasing balance owing. In scenario 3, there is an immediate reduction of principal because of the first payment of $1,000 made upon issuance of the note. The remaining four payments are made at the beginning of each year instead of at the end. This results in a faster reduction in the principal amount owing as compared with scenario 2.
Present Values With Unknown Variables
As is the case with any algebraic equation, if all variables except one are known, the final unknown variable can be derived. In the case of present value calculations, if any four of the five variables in the following equation
PV = (PMT, I/Y, N, FV)
are known, the fifth unknown variable amount can be determined using a financial calculator or an Excel net present value function. For example, if the interest rate (I/Y) is not known, it can be derived if all the other variables in the variables string are known. This will be illustrated when non-interest-bearing long-term notes payable are discussed later in this chapter.
Present Values: When Stated Interest Rates Are Different Than Effective (Market) Interest Rates
Differences between the stated interest, or face rate, and the effective, or market, rate at the time a note is issued can have accounting consequences as follows:
- If the stated interest rate of the note, (i.e., the interest rate that the note pays) is 10% at a time when the effective interest rate (also called the market rate or yield) is 10% for notes with similar characteristics and risk, the note is initially recognized as: Face value = Fair value = Present value of the note. This makes intuitive sense since the stated rate of 10% is equal to the market rate of 10%.
- If the stated interest rate is 10%, and the market rate is 11%, the stated rate is lower than the market rate and the note is trading at a discount.
- If the stated interest rate is 10%, and the market rate is 9%, the stated rate is higher than the market rate and the note is trading at a premium.
The premium or discount amount is to be amortized over the term of the note.
Below are the acceptable methods to amortize discounts or premiums:
- If a company follows IFRS, the effective interest method of amortization is required, which we will discuss in the next section.
- If a company follows ASPE, the amortization method is not specified, so either straight-line amortization or the effective interest method is appropriate as an accounting policy choice.
Here are some examples with journal entries involving various face value, or stated rates, compared to market rates.
- Notes issued at face value. Assume that on January 1, Carpe Diem Ltd. lends $10,000 to Fascination Co. in exchange for a $10,000, three-year note, bearing interest at 10% payable annually at the end of each year (ordinary annuity). The market interest rate for a note of similar risk is also 10%. The note’s present value is calculated as:
Face value of the note $10,000 Present value of the note principal and interest: Payment = 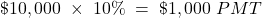
PV = (1000 PMT, 10 I/Y, 3 N, 10000 FV) 10,000 Difference $0 In this case, the note’s face value and present value, or fair value, are the same ($10,000) because the effective, or market, and stated interest rates are the same. Fascination Co.’s entry on the date of issuance is:
If Fascination Co.’s year-end was December 31, the accrued interest each year would be:
- Stated rate lower than market rate – a discount Assume that Anchor Ltd. makes a loan to Sizzle Corp. in exchange a $10,000, three-year note, bearing interest at 10% payable annually. The market rate of interest for a note of similar risk is 12%. Recall that the stated rate of 10% determines the amount of the cash paid for interest. However, the present value uses the effective (market) rate to discount all cash flows to determine the amount to record as the note’s value at the time of issuance. The note’s present value is calculated as:
Face value of the note $10,000 Present value of the note principal and interest: Payment = 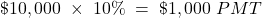
PV = (1000 PMT, 12 I/Y, 3 N, 10000 FV) 9,520 Discount amount $480 As shown above, the note’s stated rate (10%) is less than the market rate (12%), so the note is issued at a discount.
Sizzle Corp.’s entry to record the issuance of the note payable would be:
Even though the face value of the note is $10,000, the amount of money lent to Sizzle Corp. would only be $9,520. This considers the discount amount due to the difference between the stated and market interest rates discussed earlier. In return, Sizzle Corp. will pay to Anchor Ltd. an annual cash payment of $1,000 for three years, plus a lump sum payment of $10,000 at the end of the third year when the note matures. The total cash payments will be $13,000 over the term of the note, and the interest component of the note would be:
Cash paid $13,000 Present value (fair value) 9,520 Interest component 3,480 (over the three-year term) As mentioned earlier, if Sizzle Corp. follows IFRS, the $480 discount amount would be amortized using the effective interest rate method. If Sizzle Corp. follows ASPE, there would be a choice between the effective interest method and the straight-line method.
Below is a schedule that calculates the cash payments, interest expense, discount amortization, and the carrying amount (book value) of the note at the end of each year using the effective interest method:
$10,000 Note Payment and Amortization Schedule
Effective Interest Method
Stated rate of 10% and Market Rate of 12%Cash Paid Interest Expense @ 12% Amortized Discount Carrying Amount Date of issue $9,520 End of year 1 $1,000 $1,142* $142 9,662 End of year 2 1,000 1,159 159 9,821 End of year 3 1,000 1,179 179 10,000 End of year 3 final payment 10,000 - - 0 $13,000 $3,480 $480 * 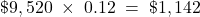
Note that the total discount amortized of $480 in the schedule is equal to the discount originally calculated as the difference between the face value of the note and the present value of the note principal and interest. Also, the amortization amount calculated each year is added to the note’s carrying value, thereby increasing its carrying amount until it reaches its maturity value of $10,000. As a result, the carrying amount at the end of each period is always equal to the present value of the note’s remaining cash flows discounted at the 12% market rate. This is consistent with the accounting standards for the subsequent measurement of long-term notes payable at the amortized cost.
Assuming that Sizzle Corp.’s year-end was the same date as the note’s interest paid, at the end of year 1, using the schedule above, Sizzle Corp.’s entry would be:

Alternatively, if Sizzle Corp. followed ASPE the straight-line method of amortizing the discount is simple to apply. The total discount of $480 is amortized over the three-year term of the note in equal amounts. The annual amortization of the discount is $160 (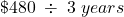 ) for each of the three years, as shown in the following entry:
) for each of the three years, as shown in the following entry:Comparing the three years’ entries for both the effective interest and the straight-line methods, the following pattern for amortization over the life of the note payable is shown below:
Effective Interest Straight-Line End of year 1 $142 $160 End of year 2 159 160 End of year 3 179 160 $480 $480 The amortization of the discount using the effective interest method results in increasing amounts of interest expense that will be recorded in the adjusting entry (decreasing amounts of interest expense for amortizing a premium) compared to the equal amounts of interest expense using the straight-line method. The straight-line method is easier to apply but its shortcoming is that the interest rate (yield) for the note is not held constant at the 12% market rate as it is with the effective interest method. This is because the amortization of the discount is in equal amounts and does not take into consideration what the carrying amount of the note was at any period of time. However, at the end of year 3, the notes payable balance is $10,000 for both methods, and so the same entry is recorded for the payment of the cash.
- Stated rate more than market rate – a premium Had the note’s stated rate of 10% been greater than a market rate of 9%, the present value of $10,253 would be greater than the face value of the note due to the premium. The same types of calculations and entries as shown in the previous illustration would be used. Note that the premium amortized each year would decrease the carrying amount of the note at the end of each year until it reaches its face value amount of $10,000.
$10,000 Note Payment and Amortization Schedule
Effective Interest Method
Stated rate of 10% and Market Rate of 9%Cash Paid Interest Expense @ 9% Amortized Premium Carrying Amount Date of issue $10,253 End of year 1 $1,000 $923* $77 10,176 End of year 2 1,000 916 84 10,092 End of year 3 1,000 908 92 10,001 End of year 3 final payment 10,000 - - 0 $13,000 $2,747 $253 * 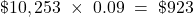
Sizzle Corp.’s entry on the note’s issuance date is for the present value amount (fair value):
If the company’s year-end was the same date as the note’s interest paid at the end of year 1, using the schedule above, the entry would be:
The entry when paid at maturity would be:
- Zero-interest-bearing notes Some companies will issue zero-interest-bearing notes as a sales incentive. While they do not state an interest rate, the term zero-interest is inaccurate as the notes do include an interest component that is equal to the difference between the cash lent and the higher amount of cash repaid at maturity. Even though the interest rate is not stated, the implied interest rate can be derived because the cash amounts lent and received are both known. In most cases, the transaction between the issuer and acquirer of the note is at arm’s length, so the implicit interest rate would be a reasonable estimate of the market rate.Assume that on January 1, Eclipse Corp. received a five-year, $10,000 zero-interest-bearing note from Galaxy Ltd. The amount of cash lent to Galaxy Ltd., which is equal to the present value, is $7,835 (rounded). Galaxy Ltd.’s year-end is December 31. Looking at the cash flows and the timeline:
Note that the sign for the $7,835 PV is preceded by the +/- symbol, meaning that the PV amount is to have the opposite symbol to the FV amount. Also, FV is the cash paid at maturity, while the PV is the amount of cash lent to the note issuer. Many financial calculators require the use of a +/- sign for one value, and no sign for the other, to correctly calculate imputed interest rates. Consult your calculator manual for further instructions regarding zero-interest note calculations.
The implied interest rate is calculated to be 5% and the note’s interest component (rounded) is $2,165 ($10,000 – $7,835), which is the difference between the cash lent and the higher amount of cash repaid at maturity. Below is the schedule for the interest and amortization calculations using the effective interest rate method:
Non-Interest-Bearing Note Payment and Amortization Schedule
Effective Interest MethodCash Paid Interest Income @ 5% Amortized Discount Carrying Amount Date of issue $0 $7,835.26 End of year 1 0 $391.76* $391.76 8,227.02 End of year 2 0 411.35 411.35 8,638.37 End of year 3 0 431.92 431.92 9,070.29 End of year 4 0 453.51 453.51 9,523.80 End of year 5 0 476.20** 476.20 10,000 End of year 5 payment $10,000 - - 0 $2,164.74 $2164.74 * 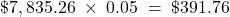
** roundingGalaxy Ltd.’s entry for the note payable when issued would be:
At Galaxy Ltd.’s year-end on December 31, the accrued interest at the end of the first year using the effective interest method would be:
At maturity when the cash payment is made, Galaxy Ltd.’s entry would be:
If Galaxy Ltd. followed ASPE instead of IFRS, the entry using the straight-line method for amortizing the discount is calculated as the total discount of $2,164.74, amortized over the 5-year period term of the note resulting in equal amounts each year. Therefore, the annual amortization is $432.95 (
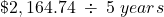 ) each year:
) each year: - Notes Payable in Exchange for Property, Goods, or Services When property, goods, or services are exchanged for a note, and the market rate and the timing and amounts of cash paid are all known, the present value of the note can be determined. For example, assume that on May 1, Hudson Inc. receives a $200,000, 5-year note from Xertoc Corp. in exchange for land that originally cost $120,000. If the market rate for a note with similar characteristics and risks is 8%, the present value is calculated as follows:
PV 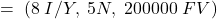
PV 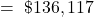
Xertoc Corp.’s entry upon issuance of the note and purchase of the land would be:
However, if the market rate is not known, either of following two approaches can be used to determine the fair value of the note:
- Determine the fair value of the property, goods, or services received. As was discussed for zero-interest-bearing notes where the interest rate was not known, the implicit interest rate can still be derived because the cash amount lent, and the timing and amount of the cash flows paid from the issuer are both known. In this case the amount lent is the fair value of the property, goods, or services given up. Once the interest is calculated, the effective interest method can be applied.[1] For example, on June 1, Mayflower Consulting Ltd. receives a $40,000, three-year note from Norfolk Ltd. in exchange for some land. The market rate cannot be accurately determined due to some credit risks regarding Norfolk Ltd. The land fair value on the transaction date is $31,750. The imputed interest rate is calculated as follows:
I/Y 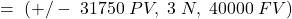
I/Y 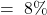
and the interest expense component is $8,250 over three years
(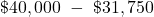 ).
).Norfolk Ltd.’s entry upon issuance of the note would be:
- Determine an imputed interest rate. An imputed interest rate is an estimated interest rate used for a note with comparable terms, conditions, and risks between an independent borrower and lender.
On June 1, Edmunds Co. receives a $30,000, three-year note from Virginia Simms Ltd. in exchange for some swamp land. The land has a historic cost of $5,000 but neither the market rate nor the fair value of the land can be determined.
In this case, a risk-adjusted rate of return must be determined and subsequently used to determine the note’s present value (fair value). For companies that follow IFRS, the fair value hierarchy identified in IFRS 13 Fair Value Measurement would be used to determine the appropriate risk adjusted rate of return and the subsequent fair value of the land. In the absence of a directly comparable market, level 2 or level 3 inputs are used. This can include present value calculations based on expected future cash flows. In this case, the future cash flow is the $30,000 note payment. The discount rate should be determined based on the risk-free rate of return, adjusted for the risk factors of the transaction. Alternately, use risk-adjusted cash flows, discounted at the risk-free rate. The calculated PV subsequently becomes the fair value used. In this case, the risk-free rate of return adjusted for the risk factors for this transaction is determined to be 7%. The present value is calculated as follows:
| PV | |
| PV |
Virginia Simms Ltd.’s entry upon issuance of the note would be:
13.2.2. Subsequent Measurements and Derecognition
As previously discussed, under ASPE and IFRS, long-term notes payable that are held by debtors are subsequently accounted for at amortized cost, which is calculated as:
- present value of the cash flows, including commissions or fees if any
- +/- reductions in principal or for any adjustments for amortization of the discount or premium
- derecognition of the debt through retirement or settlement. All premiums or discounts will be fully amortized by the maturity date. The carrying amount at maturity will be the same as the note’s face value so there will be no gain or loss at maturity unless the debt is settled early.
Impairment
If a debtor runs into financial difficulties and is unable to pay, or fully repay, the note, the estimated impaired cash flows become an important reporting disclosure for the lender. If the lender can reasonably estimate the impaired cash flows an entry is made to record the debt impairment. The impairment amount is calculated as the difference between the carrying value at amortized cost and the present value of the estimated impaired cash flows.
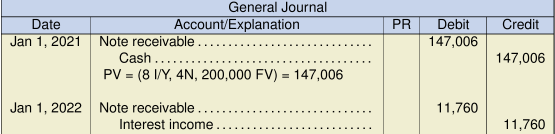
For example, on January 1, 2021, Empire Construction Ltd. signed a $200,000, four-year, non-interest-bearing note payable with Second National Bank. The required yield for the bank was 8%. During 2023, Empire Construction Ltd. experienced some serious financial difficulties. Based on the information provided by Empire Construction Ltd. management, the bank estimated that it was probable that it would receive only 75% of the 2023 balance at maturity. Additionally, the current market rate of interest in 2023 is 7%.
Below are the effective interest schedule and entries for Second National Bank:
|
Second National Bank |
|||
|---|---|---|---|
| Payment | Interest @ 8% | Carrying Value | |
| Jan 1, 2021 | $147,006 | ||
| Jan 1, 2022 | 0 | $11,760 | 158,766 |
| Jan 1, 2023 | 0 | 12,701 | 171,468 (Impairment date) |
| Jan 1, 2024 | 0 | 13,717 | 185,185 |
| Jan 1, 2025 | 0 | 14,815 | 200,000 |
For the lender, the entries for 2021 and 2022 would be:
The interest schedule and amounts entered would be the same for Empire Construction Ltd. who would record the entries to interest expense and to notes payable as a long-term liability. In 2023, the impairment would be calculated and recorded by the lender as calculated and shown below:
| Note receivable balance as at January 1, 2023 | $171,468 | |
| Present value of impaired cash flows: | ||
| (At the original required yield of 8%) | ||
| PV = (8 I/Y, ( |
110,255 | |
| Impairment loss | $61,213 | |
| * |
||
Empire Construction Ltd. (debtor) makes no entry since it still legally owes the debt amount, unless the impairment results in a troubled debt restructuring, which is discussed next.
Troubled Debt Restructurings
A troubled debt restructuring occurs if a lender grants concessions, to a debtor, such as a reduced interest rate, an extended maturity date, or a reduction in the debts’ face amount. These can take the form of a settlement of the debt or a modification of the debt’s terms.
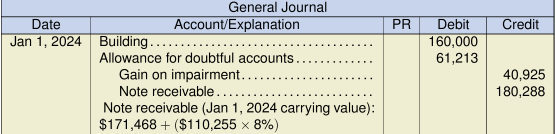
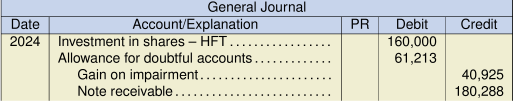
- Settlement of Debt This occurs when the debt is derecognized and all amounts relating to the debt, and any unamortized discounts or premiums, are removed from the debtor’s accounts. A gain by the debtor is usually recorded, since the creditor grants a concession when there is a troubled debt restructuring. The creditor will also remove the debt from the accounts and will record a loss. The debtor will settle the account by transferring assets such as property, plant, or equipment that may have been used to secure the note (a loan foreclosure), by issuing shares, or using the cash proceeds received for a new debt obtained from a new creditor. To illustrate, continuing with the example of Empire Construction Ltd.’s note with Second National Bank, assume that by January 1, 2024, financial troubles have continued to plague Empire Construction Ltd. to the point where it could no longer pay the loan when it matured the following year. On January 1, 2024, Second National Bank agrees to accept a building with a fair value of $160,000 from Empire Construction Ltd. in full settlement of the note. The building had an original cost of $185,000 and accumulated depreciation of $5,000. The bank’s (creditor) entry for the settlement is recorded below:
The fair value of the building is the valuation used to record the asset. The note receivable and related doubtful account is derecognized, or removed, from the accounts, and a further gain of $40,925 is recorded. If the bank had not previously used an allowance account, the loss on impairment would be $20,288 (
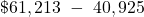 ). At this point, the bank has fully recovered the loan and made a net profit of $12,994:
). At this point, the bank has fully recovered the loan and made a net profit of $12,994:
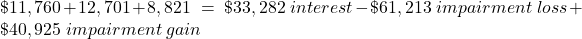
If the note had originally been secured by the building, the bank could have applied to the courts to legally seize ownership of the building to satisfy the loan obligation.The debtor’s entries are shown below:
Note that there is a separate asset loss recorded of $20,000, as well as a gain recorded of $25,185, which is required for the restructuring of the note.
Had Second National Bank agreed to accept Empire Construction Ltd.’s shares, with a market value of $160,000 in full settlement of the note, the entry would have been similar:
The entry for Empire Construction Ltd. would be:
- Modification of Terms If the creditor grants concessions such as a reduced interest rate, an extended maturity date, a reduction in the debt’s face amount, or accrued interest, there is a 10% threshold that is used to determine if the concession is minor or substantial.
- Modification of Terms Less Than 10% If the present value of the new terms, using the historic interest rate for consistency and comparability, is less than 10% different from the present value of the remaining cash flows of the old debt, then it is considered a modification of terms. The old debt amount remains but is restated using the new terms. In addition, a new effective interest rate is determined as the rate that equates the old debt with the revised cash flows resulting from the concessions made, such as the changed interest rate, due date, and face value. No gain from restructuring of the debt is recorded by the debtor.For example, on January 1, 2021, Lehry Ltd. owed $50,000, with interest payments to be made annually to Freeman Financial Trust. However, it ran into financial difficulties before any payments were made. On January 1, 2021, Freeman Financial Trust agrees to make the following concessions:
- Reduce the interest rate from 5% to 4%, with annual payments remaining at $2,500.
- Extend the due date from January 1, 2021, to January 1, 2024.
- Reduce the face value from $50,000 to $45,000.
Applying the 10% threshold to the present value calculations:
Carrying value of old debt, due January 1, 2021 (present value) $50,000 The present value, using the historic rate of 5% for the new terms: PV = (2,500 PMT, 5%, 3 N, 45,000 FV) = $45,681 Concession amount $4,319 The present value of $45,681 has a concession amount of $4,319, which is less than the 10% of the present value of the old debt of $50,000 that is now due. As a result, the concession is treated as a modification of terms. The old debt remains as the carrying value of the note but with a new effective interest rate and reduced face value of $45,000 at maturity. The new effective interest rate is calculated the same way as was done for a non-interest-bearing note where the present value, payment amount, number of years, and future value are known:
I/Y = (+/- 50,000 PV, 2,500 PMT, 3 N, 45,000 FV) = 1.72 % (rounded)
The new effective interest rate schedule is shown below:
Interest
Payment @1.72% Amortization Balance Jan 1, 2021 $50,000 Jan 1, 2022 $2,500 $860 $1,640 48,360 Jan 1, 2023 2,500 832 1,668 46,692 Jan 1, 2024 2,500 808* 1,692 45,000 *rounded Assuming a year-end date of December 31, Lehry Ltd. would make the following adjusting entry:
At maturity, Lehry Ltd. would make the following entry to settle and derecognize the note:
Freeman Financial Trust would account for the restructuring of the note as an impairment loss of $4,319 concession amount calculated above, which was discussed in the previous section of this chapter.
To recap, where debt is exchanged but the terms of the new debt are not substantially different (less than 10% – no substantial modification) from the old debt, the accounting is different. The old debt continues to exist but with new terms. Under ASPE, no gain is recorded by the debtor. Also, under ASPE, a new effective interest rate is determined by comparing the carrying amount of the original, old debt with the present value of the revised cash flows. Under IFRS, a gain is recorded by the debtor. In addition, under IFRS 9, the debt is remeasured by discounting the new cash flows at the original effective interest rate.
- Modification of Terms Greater Than 10% Modifications to a debt would be considered substantial if either of the following conditions is present:
- The present value of the new terms (using the historic interest rate) is more than 10% different than the present value of the remaining cash flows of the old debt.
- There is a change in creditor and the original debt is legally discharged (CPA Canada, 2016, Part II, Section 3856.A52 and IFRS 9/B3.3.6).
If either condition exists, the modification is substantial and will be considered a settlement of the old debt, and a new debt with the new terms is assumed.
Going back to the Lehry Ltd. example, on January 1, 2021, Lehry Ltd. owed $50,000 to Freeman Financial Trust but has run into financial difficulties. On January 1, 2021, Freeman Financial Trust agrees to make the following concessions:
- Reduce the interest rate from 5% to 3%. Payments are to remain at $2,500.
- Extend the due date from January 1, 2021, to January 1, 2023.
- Reduce the face value from $50,000 to $40,000.
Applying the 10% threshold to the present value calculations:
Carrying value of old debt, due January 1, 2021 (present value) $50,000 The present value, using the historic rate of 5% for the new terms: PV = (2,500 PMT, 5%, 2 N, 40,000 FV) = $40,930 Concession amount $9,070 The present value of $40,930 has a concession amount of $9,070, which is more than the 10% of the present value of the old debt of $50,000, which is now due. As a result, the concession is treated as a substantial modification of terms. The old debt is settled, a gain is recorded by the debtor, and a new debt with the new terms is recorded as shown in the following entry:
The present value of the new debt is calculated as follows:
PV = (2,500 PMT, 3 I/Y, 2 N, 40,000 FV) = $42,488
Freeman Financial Trust would account for the restructuring of the note as an impairment, a term previously discussed in this chapter, except that no allowance account would be used since this modification is a settlement and not simply an adjustment. If there was an outstanding allowance account balance from a previous impairment loss for this debt, the allowance account would also be closed as part of this entry.

If the new debt’s value differs by more than 10% of the old debt’s value, the change to the lending arrangement is considered to be a substantial modification The new debt would be considered a settlement and a gain would have to be recorded. The old debt is removed and the new debt recorded.
- According to IAS (2013) “IFRS 13 Fair Value Measurement applies to IFRSes that require or permit fair value measurements or disclosures and provides a single IFRS framework for measuring fair value and requires disclosures about fair value measurement. The Standard defines fair value based on an ‘exit price’ notion and uses a ‘fair value hierarchy’, which results in a market-based, rather than entity-specific, measurement. IFRS 13 was originally issued in May 2011 and applies to annual periods beginning on or after 1 January 2013” (para 1). IFRS 13 is beyond the scope of this course. For simplicity, the fair value of the property, goods, or services given up, as explained in the chapter material, assumes that IFRS 13 assumptions and hierarchy to determine fair values have been appropriately considered. ↵