Chapter 8.8: Radicals of Mixed Index
Knowing that a radical has the same properties as exponents allows conversion of radicals to exponential form and then reduce according to the various rules of exponents is possible. This is shown in the following examples.
Example 1
Simplify ![]() .
.
![Rendered by QuickLaTeX.com \begin{array}{ll} \text{First rewrite the radical as a fractional exponent}& (x^6y^2)^{\frac{1}{8}} \\ \\ \text{Multiply all exponents}& x^{6\cdot \frac{1}{8}}y^{2\cdot \frac{1}{8}} \\ \\ \text{This yields} & x^{\frac{6}{8}}y^{\frac{2}{8}}\\ \\ \text{Reducing this gives} & x^{\frac{3}{4}}y^{\frac{1}{4}}\\ \\ \text{Rewrite as} & \sqrt[4]{x^3y} \end{array}](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-d081259c94c12bd0d38b1ac0a2fed07e_l3.png)
Note: In Example 9.8.1, all exponents are reduced by the common factor 2. If there is a common factor in all exponents, reduce by dividing that common factor without having to convert to a different form.
Example 2
Simplify ![]() .
.
For this radical, notice that each exponent has the common factor 3.
The solution is to divide each exponent by 3, which yields ![]() .
.
When encountering problems where the index of the radicals do not match,convert each radical to individual exponents and use the properties of exponents to combine and then reduce the radicals.
Example 3
Simplify ![]() .
.
First, convert each radical to a complete exponential form.
![Rendered by QuickLaTeX.com \begin{array}{ll} \text{This looks like} & (4x^2y)^{\frac{1}{3}}(8xy^3)^{\frac{1}{4}} \\ \\ \text{Multiply all exponents} & 4^{\frac{1}{3}}x^{2\cdot \frac{1}{3}}y^{\frac{1}{3}}8^{\frac{1}{4}}x^{\frac{1}{4}}y^{3\cdot \frac{1}{4}} \\ \\ \text{This yields} & 4^{\frac{1}{3}}x^{\frac{2}{3}}y^{\frac{1}{3}}8^{\frac{1}{4}}x^{\frac{1}{4}}y^{\frac{3}{4}} \\ \\ \text{Combining like variables leaves} & 4^{\frac{1}{3}}8^{\frac{1}{4}}x^{\frac{2}{3}}x^{\frac{1}{4}}y^{\frac{1}{3}}y^{\frac{3}{4}} \\ \\ (\text{Note: }& 4^{\frac{1}{3}}8^{\frac{1}{4}}=2^{2\cdot \frac{1}{3}}2^{3\cdot \frac{1}{4}}=2^{\frac{2}{3}}2^{\frac{3}{4}}) \\ \\ \text{Accounting for this yields} & 2^{\frac{2}{3}}2^{\frac{3}{4}}x^{\frac{2}{3}}x^{\frac{1}{4}}y^{\frac{1}{3}}y^{\frac{3}{4}}\\ \\ \text{Reducing this yields} & 2^{\frac{2}{3}+\frac{3}{4}}x^{\frac{2}{3}+\frac{1}{4}}y^{\frac{1}{3}+\frac{3}{4}} \\ \\ \text{Which further reduces to} & 2^{\frac{17}{12}}x^{\frac{11}{12}}y^{\frac{13}{12}} \\ \\ \text{Reduce this} & 2y\cdot 2^{\frac{5}{12}}x^{\frac{11}{12}}y^{\frac{1}{12}} \\ \\ \text{Convert this back into a radical} & 2y(2^5x^{11}y)^{\frac{1}{12}} \\ \\ \text{Which leaves } & 2y \sqrt[12]{2^5x^{11}y} \end{array}](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-0f3061a0a145cb507e8b7b48ace6d6be_l3.png)
The strategy of converting all radicals to exponents works for increasingly complex radicals.
Example 4
Simplify ![]() .
.
First, convert each radical to a complete exponential form.
![Rendered by QuickLaTeX.com \begin{array}{ll} \text{This looks like} & 3^{\frac{1}{2}}x^{\frac{1}{2}}(y+z)^{\frac{1}{2}}9^{\frac{1}{3}}x^{\frac{1}{3}}(y+z)^{\frac{2}{3}} \\ \\ (\text{Note: } & 9^{\frac{1}{3}}=3^{\frac{2}{3}})\\ \\ \text{Combining like variables leaves} & 3^{\frac{1}{2}}3^{\frac{2}{3}}x^{\frac{1}{2}}x^{\frac{1}{3}} (y+z)^{\frac{1}{2}}(y+z)^{\frac{2}{3}}\\ \\ \text{Reducing this yields} & 3^{\frac{1}{2}+\frac{2}{3}}x^{\frac{1}{2}+\frac{1}{3}}(y+z)^{\frac{1}{2}+\frac{2}{3}} \\ \\ \text{Which further reduces to} & 3^{\frac{7}{6}}x^{\frac{5}{6}}(y+z)^{\frac{7}{6}} \\ \\ \text{Reduce this} & 3(y+z)3^{\frac{1}{6}}x^{\frac{5}{6}}(y+z)^{\frac{1}{6}} \\ \\ \text{Convert this back into a radical} & 3(y+z)[3x^5(y+z)]^{\frac{1}{6}} \\ \\ \text{Which leaves} & 3(y+z) \sqrt[6]{3x^5(y+z)} \end{array}](https://ecampusontario.pressbooks.pub/app/uploads/quicklatex/quicklatex.com-53b2f00af525699dd437d2518e8fbd8a_l3.png)
Questions
Reduce the following radicals. Leave as fractional exponents.
Combine the following radicals. Leave as fractional exponents.
Answers to odd questions
1. ![]()
3. ![]()
5. 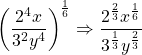
7. ![]()
9. ![]()
11. ![]()
13. ![]()
15. ![]()
17. ![]()
19. ![]()
21. ![]()
23. ![]()

