8.6: Application: Treasury Bills and Commercial Paper
Application: Treasury Bills and Commercial Paper
Treasury Bills: The Basics
Treasury bills, also known as T-bills, are short-term financial instruments that both federal and provincial governments issue with maturities no longer than one year. Approximately 27% of the national debt is borrowed through T-bills.
Here are some of the basics about T-bills:
- The Government of Canada regularly places T-bills up for auction every second Tuesday. Provincial governments issue them at irregular intervals.
- The most common terms for federal and provincial T-bills are 30 days, 60 days, 90 days, 182 days, and 364 days.
- T-bills do not earn interest. Instead, they are sold at a discount and redeemed at full value. This follows the principle of “buy low, sell high.” The percentage by which the value of the T-bill grows from sale to redemption is called the yield or rate of return. From a mathematical perspective, the yield is calculated in the exact same way as an interest rate is calculated, and therefore the yield is mathematically substituted as the discount rate in all simple interest formulas. Up-to-date yields on T-bills can be found at www.bankofcanada.ca/en/rates/monmrt.html.
- The face value of a T-bill (also called par value) is the maturity value, payable at the end of the term. It includes both the principal and yield together.
- T-bills do not have to be retained by the initial investor throughout their entire term. At any point during a T-bill’s term, an investor is able to sell it to another investor through secondary financial markets. Prevailing yields on T-bills at the time of sale are used to calculate the price.
Commercial Papers – The Basics
A commercial paper (or paper for short) is the same as a T-bill except that it is issued by a large corporation instead of a government. It is an alternative to short-term bank borrowing for large corporations. Most of these large companies have solid credit ratings, meaning that investors bear very little risk that the face value will not be repaid upon maturity.
Commercial papers carry the same properties as T-bills. The only fundamental differences lie in the term and the yield:
- The terms are usually less than 270 days but can range from 30 days to 364 days. The most typical terms are 30 days, 60 days, and 90 days.
- The yield on commercial papers tends to be slightly higher than on T-bills since corporations do carry a higher risk of default than governments.
How It Works
Mathematically, T-bills and commercial papers operate in the exact same way. The future value for both of these investment instruments is always known since it is the face value. Commonly, the two calculated variables are either the present value (price) or the yield (interest rate). The yield is explored later in this section. Follow these steps to calculate the price:
Step 1: The face value, yield, and time before maturity must be known. Draw a timeline if necessary, as illustrated below, and identify the following:
- The face value (S).
- The yield (r) on the date of the sale, which is always expressed annually. Remember that mathematically the yield is the same as the discount rate.
- The number of days (t) remaining between the date of the sale and the maturity date. Count the first day but not the last day. Express the number of days annually to match the annual yield.

Step 2: Solve for the present value. using [latex]P=\frac{S}{1+rt}[/latex], which is the price of the T-bill or commercial paper. This price is always less than the face value.
Concept Check:
Example 8.6.1: Price of a Treasury Bill
A Government of Canada 182-day issue T-bill has a face value of $100,000. Market yields on these T-bills are 1.5%. Calculate the price of the T-bill on its issue date.
Solution:
Step 1: Given variables:
S = $100,000; r = 1.5% ; t = 182/365
Step 2: Solve for the present value, P.
[latex]\begin{align} P&=\frac{S}{1+rt}\\ &=\frac{\$100,\!000}{1+(0.015)\left(\frac{182}{365}\right)}\\ &=\$99,\!257.61 \end{align}[/latex]
An investor will pay $99,257.61 for the T-bill. If the investor holds onto the T-bill until maturity, the investor realizes a yield of 1.5% and receives $100,000.
Example 8.6.2: Selling a Commercial Paper During Its Term
Pfizer Inc. issued a 90-day, $250,000 commercial paper on April 18 when the market rate of return was 3.1%. The paper was sold 49 days later when the market rate of return was 3.63%. Calculate the price of the commercial paper on its date of sale.
Solution:
Note that the historical rate of return of 3.1% is irrelevant to the price of the commercial paper today. The number of days elapsed since the date of issue is also unimportant. The number of days before maturity is the key piece of information.
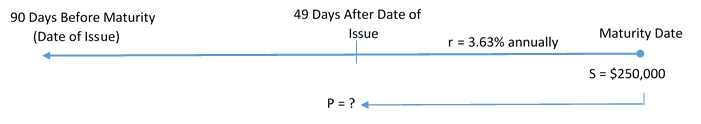
Step 1: Given variables:
S = $250,000; r = 3.63%; t = 90 – 49 = 41 days or 41/365 years
Step 2:Solve for the present value, P.
[latex]\begin{align} P&=\frac{S}{1+rt}\\ &=\frac{$250,\!000}{1+(0.0363)\left(\frac{41}{365}\right)}\\ &=\$248,\!984.76 \end{align}[/latex]
An investor pays $248,984.76 for the commercial paper on the date of sale. If the investor holds onto the commercial paper for 41 more days (until maturity), the investor realizes a yield of 3.63% and receives $250,000.
How It Works
Calculating a Rate of Return: Sometimes the unknown value when working with T-bills and commercial papers is the yield, or rate of return. In these cases, follow these steps to solve the problem:
Step 1: The face value, price, and time before maturity must be known. Draw a timeline if necessary, as illustrated below, and identify:

- The number of days (t) remaining between the date of the sale and the maturity date. Count the first day but not the last day. Express the number of days annually so that the calculated yield will be annual.
Step 2: Apply formula I = S − P, to calculate the interest earned during the investment.
Step 3: Apply simple interest formula, I=Prt, rearranging for r to solve for the interest rate (or yield or rate of return).
Example 8.6.3: Figuring Out Rates of Return for Multiple Investors
Marlie paid $489,027.04 on the date of issue for a $500,000 face value T-bill with a 364-day term. Marlie received $496,302.21 when he sold it to Josephine 217 days after the date of issue. Josephine held the T-bill until maturity. Determine the following:
a) Marlie’s actual rate of return.
b) Josephine’s actual rate of return.
c) If Marlie held onto the T-bill for the entire 364 days instead of selling it to Josephine, what would his rate of return have been?
d) Comment on the answers to (a) and (c).
Solution:
Calculate three yields or rates of return (r) involving Marlie and the sale to Josephine, Josephine herself, and Marlie without the sale to Josephine. Afterwards, comment on the rate of return for Marlie with and without the sale.
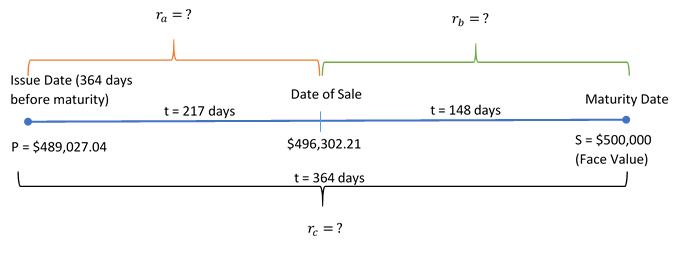
Step 1: Given information:
The present values, maturity value, and terms are known.
a) Marlie with sale:
P = $489,027.04; S = $496,302.21; t = 217/365
b) Josephine:
P = $496,302.21; S = $500,000; 364 – 217 = 147 days remaining; t = 147/365
c) Marlie without sale:
P = $489,027.04; S = $500,000; t = 364/365
Step 2: For each situation, calculate the interest amount (I).
a) Marlie with sale to Josephine:
I = $496,302.21 – $489,027.04 = $7,275.17
b) Josephine by herself:
I = $500,000 – $496,302.21 = $3,697.79
c) Marlie without sale to Josephine:
I = $500,000 – $489,027.04 = $10,972.96
Step 3: For each situation, apply simple interest formula, rearranging for r.
a) Marlie with sale to Josephine:
[latex]\begin{align} r&=\frac{\$7,\!275.17}{(\$489,\!027.04)\left(\frac{217}{365}\right)}=2.50\% \end{align}[/latex]
b) Josephine by herself:
[latex]\begin{align} r&=\frac{\$3,\!697.79}{(\$496,\!302.21)\left(\frac{147}{365}\right)}=1.85\% \end{align}[/latex]
c) Marlie without sale to Josephine:
[latex]\begin{align} r&=\frac{\$10,\!972.96}{(\$489,\!027.04)\left(\frac{364}{365}\right)}=2.25\% \end{align}[/latex]
When Marlie sold the T-bill after holding it for 217 days, he realized a 2.50% rate of return. Josephine then held the T-bill for another 148 days to maturity, realizing a 1.85% rate of return. If Marlie hadn’t sold the note to Josephine and instead held it for the entire 364 days, he would have realized a 2.25% rate of return.
Step 4: Compare the answers for (a) and (c) and comment.
The yield on the date of issue was 2.25%. Marlie realized a higher rate of return because the interest rates in the market decreased during the 217 days he held it (to 1.85%, which is what Josephine is able to obtain by holding it until maturity). This raises the selling price of the T-bill. If his investment of $489,027.04 grows by 2.25% for 217 days, he has $6,541.57 in interest. The additional $733.60 of interest (totaling $7,275.17) is due to the lower yield in the market, increasing his rate of return to 2.50% instead of 2.25%.
Exercises
In each of the exercises that follow, try them on your own. Full solutions are available should you get stuck.
- A 60-day, $90,000 face value commercial paper was issued when yields were 2.09%. What was its purchase price? (Answer: $89,691.85)
- A 90-day Province of Ontario T-bill with a $35,000 face value matures on December 11. Farrah works for Hearthplace Industries and notices that the company temporarily has some extra cash available. If she invests the money on October 28, when the yield is 4.94%, and sells the T-bill on November 25, when the yield is 4.83%, calculate how much money Farrah earned and the rate of return she realized. (Answer: Amount earned = $133.24; r = 4.99%)
- Philippe purchased a $100,000 Citicorp Financial 220-day commercial paper for $96,453.93. He sold it 110 days later to Damien for $98,414.58, who then held onto the commercial paper until its maturity date.
a) What is Philippe’s actual rate of return? (Answer: 6.74%)
b) What is Damien’s actual rate of return? (Answer: 5.35%)
c) What is the rate of return Philippe would have realized if he had held onto the note instead of selling it to Damien? (Answer: 6.10%)
Image Descriptions
Figure 8.6.0: Timeline showing on the Left, “Date of sale”, “P = ?”, with arrow moving to the end (on the Right) to “Maturity Date” and “S = Face Value”. Yield (r) on the Date of Sale. [Back to Figure 8.6.0]
Figure 8.6.2: Timeline showing “Maturity Date” on the Right with arrow back to the Left to “90 Days Before Maturity (Date of Issue)”. At “Maturity Date”, S = $250,000 moves back to “49 Days After Date of Issue” to P = ? with r = 3.63% annually. [Back to Figure 8.6.2]
Figure 8.6.Y: Timeline showing on the Left, “Date of sale”, “P = Price”. On the Right, “Maturity Date” and “S = Face Value”. in the Middle, “On Date of Sale”, “Yield (r) = ? annually”. [Back to Figure 8.6.Y]
Figure 8.6.3: Timeline showing on the Left, “Issue Date (364 days before maturity)”, “P = $489,027.04”. t = 217 days later to “Date of Sale” and “$496,302.21”. t = 148 days later to “Maturity Date” and “S = $500,000 (Face Value)” on the Right. ra = ? from Issue Date until Date of Sale. rb = ? from Date of Sale to Maturity Date. rc = ? and t = 364 days from Issue Date until Maturity Date. [Back to Figure 8.6.3]

