8.2: Moving Money Involving Simple Interest
Moving Money Involving Simple Interest
Maturity Value (or Future Value)
The maturity value of a transaction is the amount of money resulting at the end of a transaction, an amount that includes both the interest and the principal together. It is called a maturity value because in the financial world the termination of a financial transaction is known as the “maturing” of the transaction. The amount of principal with interest at some point in the future, but not necessarily the end of the transaction, is known as the future value.
For any financial transaction involving simple interest, the following is true:
Applying algebra, you can summarize this expression by the following equation, where the future value or maturity value is commonly denoted by the symbol S.
\[S = P + I\]
Substituting in [latex]I=Prt[/latex], yields the equation
\[S=P + Prt\]
or
\[S=P(1+rt)\]
The Formula
[latex]\colorbox{LightGray}{Formula 8.2A}\; \color{BlueViolet}{\text{Simple Interest Future Value:}\; S=P(1+rt)}[/latex]
where,
I is Interest Amount. The interest amount is the dollar amount of interest that is paid or received.
P is Present Value or Principal. The present value is the amount borrowed or invested at the beginning of a period.
r is Simple Interest Rate. The interest rate is the rate of interest that is charged or earned during a specified time period. It is expressed as a percent.
t is Time Period. The time period or term is the length of the financial transaction for which interest is charged or earned.
From the future value formula S=P(1+rt) you can derive the present value formula (P):
[latex]P=\frac{S}{1+rt}[/latex]
Sometimes you will be required to calculate the simple interest dollar amount (I). the formula is given below.
[latex]\colorbox{LightGray}{Formula 8.2A}\; \color{BlueViolet}{\text{Simple Interest Amount:}\; I=S-P}[/latex]
Example 8.2.1: Calculating Maturity Value and Interest Amount
Assume that today you have $10,000 that you are going to invest at 7% simple interest for 11 months. How much money will you have in total at the end of the 11 months? How much interest do you earn?
Solution:
Step 1: Given variables:
P = $10,000; r = 7%; t = 11 months
Step 2: Express the time in years to match the annual rate; [latex]t=\frac{11}{12}[/latex]
Step 3: Solve for the future value, S.
[latex]\begin{align} S&=P \times(1+rt)\\ &=\$10,\!000 \times \left(0.07 \times \frac{11}{12}\right)\\ &=\$10,\!641.67 \end{align}[/latex]
This is the total amount after 11 months.
Step 4: Solve for the interest amount, I.
I = $10,641.67 – $10,000.00 = $641.67
The $10,000 earns $641.67 in simple interest over the next 11 months, resulting in $10,641.67 altogether.
Concept Check
Example 8.2.2: Saving for a Down Payment on a Home
You just inherited $35,000 from your uncle’s estate and plan to purchase a house four months from today. If you use your inheritance as your down payment on the house, how much will you be able to put down if your money earns 4¼% simple interest? How much interest will you have earned?
Solution:
Calculate the amount of money four months from now including both the principal and interest earned. This is the maturity value (S). Also calculate the interest earned (I).
Step 1: Given variables:
P = $35,000; t = 4months; [latex]r=4\frac{1}{4}\%[/latex] per year
Step 2: Express the time in years to match the annual rate; [latex]t=\frac{4}{12}[/latex]

Step 3: Solve for the future value, S.[latex]\begin{align} S&=P \times(1+rt)\\ &=\$35,\!000 \times \left(1+4\frac{1}{4}\% \times \frac{4}{12}\right)\\ &=\$35,\!000 \times (1+0.0425 \times 0.\overline{3})\\ &=\$35,\!495.83 \end{align}[/latex]
Step 4: Solve for the amount of interest, I.
I = $35,495.83 – $35,000.00 = $495.83
Four months from now you will have $35,495.83 as a down payment toward your house, which includes $35,000 in principal and $495.83 of interest.
Example 8.2.3: Saving for Tuition
Recall the section opener, where you needed $8,000 for tuition in the fall and the best simple interest rate you could find was 4.5%. Assume you have eight months before you need to pay your tuition. How much money do you need to invest today?
Solution:
Calculate the principal amount of money today [latex]P[/latex] that you must invest such that it will earn interest and end up at the $8,000 required for the tuition.
Step 1: Given variables:
S = $8,000; r=4.5% per year; t = 8 months
Step 2: Express the time in years to match the annual rate; [latex]t=\frac{8}{12}[/latex]
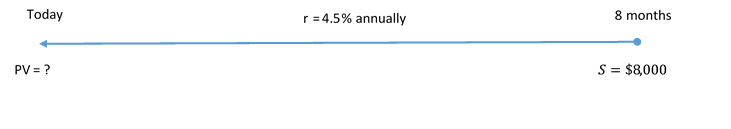
Step 3: Solve for the present value, P.
[latex]\begin{align} P&=\frac{S}{1+rt}\\ &=\frac{\$8,\!000}{(1+4.5\% \times \frac{8}{12})}\\ &=\frac{\$8,\!000}{(1+0.045 \times 0.\overline{6})}\\ &=\$7,\!766.99 \end{align}[/latex]
If you place $7,766.99 into the investment, it will grow to $8,000 in the eight months.
Example 8.2.4: What Exactly Are You being Offered?
You are sitting in an office at your local financial institution on August 4. The bank officer says to you, “We will make you a great deal. If we advance that line of credit and you borrow $20,000 today, when you want to repay that balance on September 1 you will only have to pay us $20,168.77, which is not much more!” Before answering, you decide to evaluate the statement. Calculate the simple interest rate that the bank officer used in her calculations.
Solution:
Determine the rate of interest that you would be charged on your line of credit.
Step 1: Given variables:
P = $20,000; S = $20,168.77; t = August 4 to September 1
Step 2: Calculate the number of days in the transaction.
Calculator Instructions:
Assume the year 2011 and use the DATE function to find the exact number of days:
| DT1 | DT2 | DBD</th | Mode |
|---|---|---|---|
| 8.0411 | 9.0111 | Answer: 28 | ACT |
Step 3: Since interest rates are usually expressed annually, convert the time from days to an annual number; [latex]t=\frac{28}{365}[/latex]
Step 4: Calculate the amount of interest, I.
[latex]I=\$20,\!168.77 - \$20,\!000=\$168.77[/latex]
Step 5: Solve for r.
[latex]\begin{align} r&=\frac{I}{Pt}\\ &=\frac{\$168.77}{\$20,\!000.00\times\frac{28}{365}}\\ &=0.110002\;\text{or}\;11.0002\% \end{align}[/latex]
Equivalent Payments
- Late Payments. If a debt is paid late, then a financial penalty that is fair to both parties involved should be imposed. That penalty should reflect a current rate of interest and be added to the original payment. Assume you owe $100 to your friend and that a fair current rate of simple interest is 10%. If you pay this debt one year late, then a 10% late interest penalty of $10 should be added, making your debt payment $110. This is no different from your friend receiving the $100 today and investing it himself at 10% interest so that it accumulates to $110 in one year.
- Early Payments. If a debt is paid early, there should be some financial incentive (otherwise, why bother?). Therefore, an interest benefit, one reflecting a current rate of interest on the early payment, should be deducted from the original payment. Assume you owe your friend $110 one year from now and that a fair current rate of simple interest is 10%. If you pay this debt today, then a 10% early interest benefit of $10 should be deducted, making your debt payment today $100. If your friend then invests this money at 10% simple interest, one year from now he will have the $110, which is what you were supposed to pay.
Notice in these examples that a simple interest rate of 10% means $100 today is the same thing as having $110 one year from now. This illustrates the concept that two payments are equivalent payments if, once a fair rate of interest is factored in, they have the same value on the same day. Thus, in general you are finding two amounts at different points in time that have the same value, as illustrated in the figure below.

How It Works
The steps required to calculate an equivalent payment are no different from those for single payments. If an early payment is being made, then you know the future value, so you solve for the present value (which removes the interest). If a late payment is being made, then you know the present value, so you solve for the future value (which adds the interest penalty).
Example 8.2.5: Making a Late Payment
Erin owes Charlotte $1,500 today. Unfortunately, Erin had some unexpected expenses and is unable to make her debt payment. After discussing the issue, they agree that Erin can make the payment nine months late and that a fair simple interest rate on the late payment is 5%. Use 9 months from now as your focal date and calculate how much Erin needs to pay. What is the amount of her late penalty?
Solution:
A late payment is a future value amount (S). The late penalty is equal to the interest (I).
Step 1: Given variables:
P = $1,500; r = 5% annually; t = 9 months
Step 2: Express the time in years to match the annual rate; [latex]t=\frac{9}{12}[/latex]

Step 3: Calculate the future value, S.
[latex]\begin{align} S&=P \times(1+rt)\\ S&=\$1,\!500 \times \left(1+5\% \times \frac{9}{12}\right)\\ &=\$1,\!500 \times (1+0.05 \times 0.75)\\ &=\$1,\!556.25 \end{align}[/latex]
Step 4: Solve for the amount of interest, I.
I = $1,556.25 – $1,500.00 = $56.25
Erin’s late payment is for $1,556.25, which includes a $56.25 interest penalty for making the payment nine months late.
Example 8.2.6: Making an Early Payment
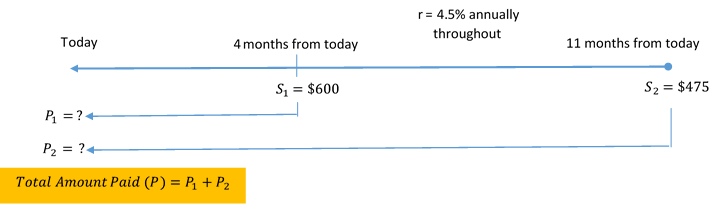
Rupert owes Aminata two debt payments: $600 four months from now and $475 eleven months from now. Rupert came into some money today and would like to pay off both of the debts immediately. Aminata has agreed that a fair interest rate is 7%. Using today as a focal date, what amount should Rupert pay? What is the total amount of his early payment benefit?
Solution:
An early payment is a present value amount ([latex]P[/latex]). Both payments will be moved to today and summed. The early payment benefit will be the total amount of interest removed ([latex]I[/latex]).
Step 1: Given variables:
[latex]r = 7% annually}[/latex]
The two payments and payment due dates are known.
Payment #1: S1 = $600; t = 4 months from now
Payment #2: S2 = $475; t = 11 months from now
Replacement payment is being made today (the focal date).
Payment #1:
Step 2: Express the time in years to match the annual rate; [latex]t=\frac{4}{12}[/latex]
Step 3: Solve for P1.[latex]\begin{align} P_1&= \frac{S_1}{1+rt}\\ &=\frac{\$600}{(1+0.07 \times 0.\overline{3})}\\ &=\$586.32 \end{align}[/latex]
Payment #2:
Step 2: While the rate is annual, the time is in months. Convert the time to an annual number; [latex]t=\frac{11}{12}[/latex]
Step 3: Solve for P2.
[latex]\begin{align} P_2&= \frac{S_2}{1+rt}\\ &=\frac{\$475}{(1+0.07 \times 0.91\overline{6})}\\ &=\$446.36 \end{align}[/latex]
Step 4: Calculate the total amount of interest, I.
Payment #1: I1 = S1 – P1 = $600 – $586.32 = $13.68
Payment #2: I2 = S2 – P2 = $475 – $446.36 = $28.64
I = $13.68 + $28.64 = $42.32
To clear both debts today, Rupert pays $1,032.68, which reflects a $42.32 interest benefit reduction for the early payment.
Exercises
In each of the exercises that follow, try them on your own. Full solutions are available should you get stuck.
- An accountant needs to allocate the principal and simple interest on a loan payment into the appropriate ledgers. If the amount received was $10,267.21 for a loan that spanned April 14 to July 31 at 9.1%, how much was the principal and how much was the interest? (Answers: P = $9,998, I = $269.21)
- Suppose Robin borrowed $3,600 on October 21 and repaid the loan on February 21 of the following year. What simple interest rate was charged if Robin repaid $3,694.63? (Answer: 7.80%)
- Jayne needs to make three payments to Jade requiring $2,000 each 5 months, 10 months, and 15 months from to day. She proposes instead making a single payment eight months from today. If Jade agrees to a simple interest rate of 9.5%, what amount should Jayne pay? (Answer: $5,911.32)
- Merina is scheduled to make two loan payments to Bradford in the amount of $1,000 each, two months and nine months from now. Merina doesn’t think she can make those payments and offers Bradford an alternative plan where she will pay $775 seven months from now and another payment seven months later. Bradford determines that 8.5% is a fair interest rate. What is the amount of the second payment? (Answer: $1,306.99)
Timelines for questions 3 and 4 are included in Solutions to Exercises.
Image Descriptions
Figure 8.2.2: Timeline showing PV =$35,000 at Today (on the Left) with an arrow pointing to the end (on the Right) (4 months) where S = ? and r = 4.25% annually [Back to Figure 8.2.2]
Figure 8.2.3: Timeline showing S = $8,000 the end (on the Right) (8 months) with an arrow pointing back to Today (on the Left) where PV =? and r = 4.5% annually. [Back to Figure 8.2.3]
Figure 8.2.E: General Timeline for Equivalent Payments: On the left, “Earlier Date”, “We are trying to find the amount here…”. In the middle, “…at same fair rate of simple interest…”. At the end, “Later Date”, “that is equivalent to some amount over here.” [Back to Figure 8.2.E]
Figure 8.2.5: Timeline showing: On the Left: “Payment due today”, “P = $1,500”. On the Right: “Payment will be made 9 months later”, “S = ?”. r = 5% annually [Back to Figure 8.2.5]
Figure 8.2.6: Timeline showing S1 = $600 at 4 months from today and S2 = $475 at 11 months from today. S1 = $600 moves back to today as P1 = ? and S2 = $475 moves back to today as P2 = ?. At Today, Total Amount Paid (P) = P1 + P2. r = 4.5% annually throughout. [Back to Figure 8.2.6]

