Exercises: The Real Numbers (1.6)

Exercises: Simplify Expressions with Square Roots

Instructions: For questions 1-12, simplify.

1. [latex]\sqrt{36}[/latex]

Solution

[latex]6[/latex]

2. [latex]\sqrt4[/latex]
3. [latex]\sqrt{64}[/latex]
Solution

[latex]8[/latex]

4. [latex]\sqrt{169}[/latex]

5. [latex]\sqrt9[/latex]
Solution

[latex]3[/latex]

6. [latex]\sqrt{16}[/latex]

7. [latex]\sqrt{100}[/latex]

Solution

[latex]10[/latex]

8. [latex]\sqrt{144}[/latex]

9. [latex]-\sqrt4[/latex]

Solution

[latex]-2[/latex]

10. [latex]-\sqrt{100}[/latex]

11. [latex]-\sqrt1[/latex]
Solution

[latex]-1[/latex]

12. [latex]-\sqrt{121}[/latex]

Exercises: Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

Instructions: For questions 13-16, write as the ratio of two integers.

13.

a. [latex]5[/latex]
b. [latex]3.19[/latex]

Solution

a. [latex]\frac51[/latex]
b. [latex]\frac{319}{100}[/latex]

14.

a. [latex]8[/latex]
b. [latex]1.61[/latex]

15.

a. [latex]-12[/latex]
b. [latex]9.279[/latex]

Solution

a. [latex]\frac{-12}{1}[/latex]
b. [latex]\frac{9297}{1000}[/latex]

16.

a. [latex]-16[/latex]
b. [latex]4.399[/latex]

Exercises: Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

Instructions: For questions 17-20, list the

a. rational numbers
b. irrational numbers

17. [latex]0.75,0.22\overline{3},1.39174...[/latex]
Solution

a. [latex]0.75,0.22\overline{3}[/latex]
b. [latex]1.39174...[/latex]

18. [latex]0.36,0.94729...,2.52\overline{8}[/latex]

19. [latex]0.4\overline{5},1.919293...,3.59[/latex]

Solution

a. [latex]0.4\overline{5},3.59[/latex]
b. [latex]1.919293...[/latex]

20. [latex]0.1\overline{3},0.42982...,1.875[/latex]

Exercises: Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

Instructions: For questions 21-24, identify whether each number is rational or irrational.

21.

a. [latex]\sqrt{25}[/latex]
b. [latex]\sqrt{30}[/latex]

Solution

a. rational
b. irrational

22.

a. [latex]\sqrt{44}[/latex]
b. [latex]\sqrt{49}[/latex]

23.

a. [latex]\sqrt{164}[/latex]
b. [latex]\sqrt{169}[/latex]

Solution

a. irrational
b. rational

24.

a. [latex]\sqrt{225}[/latex]
b. [latex]\sqrt{216}[/latex]

Exercises: Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

Instructions: For questions 25-28, identify whether each number is a real number or not a real number.

25.

a. [latex]-\sqrt{81}[/latex]
b. [latex]\sqrt{-121}[/latex]

Solution

a. real number
b. not a real number

26.

a. [latex]-\sqrt{64}[/latex]
b. [latex]\sqrt{-9}[/latex]

27.

a. [latex]\sqrt{-36}[/latex]
b. [latex]-\sqrt{144}[/latex]

Solution

a. not a real number
b. real number

28.

a. [latex]\sqrt{-49}[/latex]
b. [latex]-\sqrt{144}[/latex]

Exercises: Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

Instructions: For each set of numbers in questions 29-32, list the

a. whole numbers
b. integers
c. rational numbers
d. irrational numbers
e. real numbers

29. [latex]-8,0,1.95286...,\frac{12}{5},\sqrt{36},9[/latex]
Solution

a. [latex]0,\sqrt{36},9[/latex]
b. [latex]-8,0,\sqrt{36},9[/latex]
c. [latex]-8,0,\frac{12}{5},\sqrt{36},9[/latex]
d. [latex]1.95286...[/latex]
e. [latex]-8,0,1.95286...,\frac{12}{5},\sqrt{36},9[/latex]

30. [latex]-9,-3\frac49,-\sqrt9,0.40\overline{9},\frac{11}{6},7[/latex]

31. [latex]-\sqrt{100},-7,-\frac83,-1,0.77,3\frac14[/latex]

Solution

a. none
b. [latex]-\sqrt{100},-7,-1[/latex]
c. [latex]-\sqrt{100},-7,-\frac83,-1,0.77,3\frac14[/latex]
d. none
e. [latex]-\sqrt{100},-7,-\frac83,-1,0.77,3\frac14[/latex]

32. [latex]-6,-\frac52,0,0.\overline{714285},2\frac15,\sqrt{14}[/latex]

Exercises: Locate Fractions on the Number Line

Instructions: For questions 33-40, locate the numbers on a number line.

33. [latex]\frac35,\frac85,\frac{10}{3}[/latex]
Solution
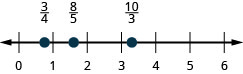
There is a number line shown that runs from 0 to 6. From left to right, the numbers marked are 3/4, 8/5, and 10/3. The number 3/4 is slightly to the left of 1. The number 8/5 is halfway between 1 and 2. The number 10/3 is slightly to the right of 3.
Figure 1P.6.1

34. [latex]\frac14,\frac95,\frac{11}{3}[/latex]

35. [latex]\frac{3}{10},\frac72,\frac{11}{6},4[/latex]

Solution
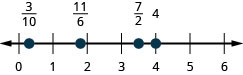
There is a number line shown that runs from 0 to 6. From left to right, the numbers marked are 3/10, 11/6, 7/2, and 4. The number 3/10 is between negative 0 and 1 but slightly closer to 0. The number 11/6 is slightly to the left of 2. The number 4 lies right on the line for 4 on the number line.
Figure 1P.6.2

36. [latex]\frac{7}{10},\frac52,\frac{13}{8},3[/latex]

37. [latex]\frac25,-\frac25[/latex]

Solution
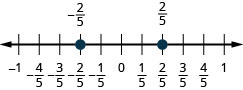
There is a number line shown that runs from negative 1 to 1, with increments of one-fifth. From left to right, the numbers marked are -2/5, and 2/5.
Figure 1P.6.3

38. [latex]\frac34,-\frac34[/latex]

39. [latex]\frac34,-\frac34,1\frac23,-1\frac23,\frac52,-\frac52[/latex]

Solution
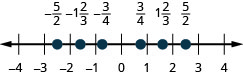
There is a number line shown that runs from negative 4 to positive 4. From left to right, the numbers marked are negative 5/2, negative 1 and 2/3, negative 3/4, positive 3/4, positive 1 and 2/3, and positive 5/2. The number negative 5/2 is halfway between negative 3 and negative 2. The number negative 1 and 2/3 is between negative 2 and negative 1, but slightly closer to negative 2. The number negative 3/4 is slightly to the right of negative 1. The number positive 3/4 is slightly to the left of positive 1. The number positive 1 and 2/3 is between positive 1 and positive 2, but slightly closer to positive 2. The number positive 5/2 is halfway between positive 2 and positive 3.
Figure 1P.6.4

40. [latex]\frac25,-\frac25,1\frac34,-1\frac34,\frac83,-\frac83[/latex]

Exercises: Order Pairs of Numbers

Instructions: For questions 41-48, order each of the pairs of numbers, using < or >.

41. [latex]-1\;\underline{\;\;\;\;}\;-\frac14[/latex]
Solution

[latex]<[/latex]

42. [latex]-1\;\underline{\;\;\;\;}\;-\frac13[/latex]

43. [latex]-2\frac12\;\underline{\;\;\;\;}\;-3[/latex]

Solution

[latex]>[/latex]

44. [latex]-1\frac34\;\underline{\;\;\;\;}\;-2[/latex]

45. [latex]-\frac{5}{12}\;\underline{\;\;\;\;}\;-\frac{7}{12}[/latex]

Solution

[latex]>[/latex]

46. [latex]-\frac{9}{10}\;\underline{\;\;\;\;}\;-\frac{3}{10}[/latex]

47. [latex]-3\;\underline{\;\;\;\;}\;-\frac{13}{5}[/latex]

Solution

[latex]<[/latex]

48. [latex]-4\;\underline{\;\;\;\;}\;-\frac{23}{6}[/latex]

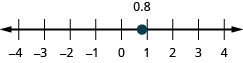
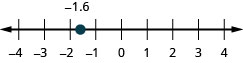
Exercises: Locate Decimals on the Number Line

Instructions: For questions 49-52, locate the number on the number line.

49. [latex]0.8[/latex]
Solution
There is a number line shown that runs from negative 4 to positive 4. The only point given is positive 0.8, which is slightly to the left of positive 1.
Figure 1P.6.5

50. [latex]-0.9[/latex]

51. [latex]-1.6[/latex]

Solution
There is a number line shown that runs from negative 4 to positive 4. The only point given is negative 1.6, which is between negative 2 and negative 1, but slightly closer to negative 2.
Figure 1P.6.6

52. [latex]3.1[/latex]

Exercises: Order Pairs of Numbers

Instructions: For questions 53-60, order each of the pairs of numbers, using < or >.

53. [latex]0.37\;\underline{\;\;\;\;}\;0.63[/latex]
Solution

[latex]<[/latex]

54. [latex]0.86\;\underline{\;\;\;\;}\;0.69[/latex]

55. [latex]0.91\;\underline{\;\;\;\;}\;0.901[/latex]

Solution

[latex]>[/latex]

56. [latex]0.415\;\underline{\;\;\;\;}\;0.41[/latex]

57. [latex]-0.5\;\underline{\;\;\;\;}\;-0.3[/latex]

Solution

[latex]<[/latex]

58. [latex]-0.1\;\underline{\;\;\;\;}\;-0.4[/latex]

59. [latex]-0.62\;\underline{\;\;\;\;}\;-0.619[/latex]

Solution

[latex]<[/latex]

60. [latex]-7.31\;\underline{\;\;\;\;}\;-7.3[/latex]

Exercises: Everyday Math

Instructions: For questions 61-62, answer the given everyday math word problems.

61. Field Trip. All the 5th graders at Lincoln Elementary School will go on a field trip to the science museum. Counting all the children, teachers, and chaperones, there will be [latex]147[/latex] people. Each bus holds [latex]44[/latex] people.

a. How many buses will be needed?
b. Why must the answer be a whole number?
c. Why shouldn’t you round the answer the usual way, by choosing the whole number closest to the exact answer?

Solution

a. [latex]4[/latex] buses
b. Answers may vary
c. Answers may vary

62. Child Care. Serena wants to open a licensed child care center. Her state requires there be no more than [latex]12[/latex] children for each teacher. She would like her child care center to serve [latex]40[/latex] children.

a. How many teachers will be needed?
b. Why must the answer be a whole number?
c. Why shouldn’t you round the answer the usual way, by choosing the whole number closest to the exact answer?

Exercises: Writing Exercises

Instructions: For questions 63-64, answer the given writing exercises.

63. In your own words, explain the difference between a rational number and an irrational number.

Solution

Answers may vary

64. Explain how the sets of numbers (counting, whole, integer, rational, irrationals, reals) are related to each other.

License

Icon for the Creative Commons Attribution-ShareAlike 4.0 International License

Fanshawe Pre-Health Sciences Mathematics 1 Copyright © 2022 by Domenic Spilotro, MSc is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book