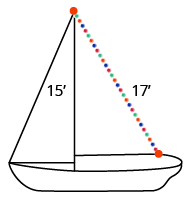
6.1 Use Properties of Angles, Triangles, and the Pythagorean Theorem
Learning Objectives
By the end of this section, you will be able to:
- Use the properties of angles
- Use the properties of triangles
- Use the Pythagorean Theorem
Try It
Before you get started, take this readiness quiz:
1) Solve: [latex]x\;+\;3\;+\;6\;=\;11[/latex]
2) Solve: [latex]\frac a{45}\;=\;\frac43[/latex]
3) Simplify: [latex]\sqrt{36\;+\;64}[/latex]
In this unit, we will study geometry and trigonometry in order to develop our understanding of how some of these tools can help us in a health career. For example, even having a simple understanding of angles will be helpful when performing injections.
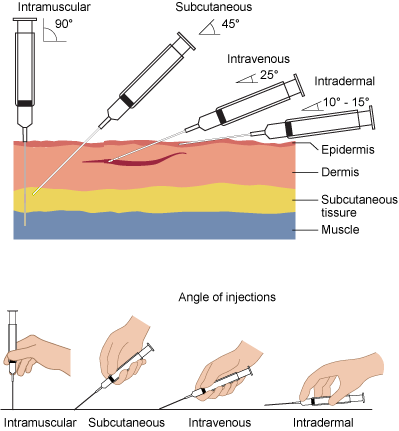
Use the Properties of Angles
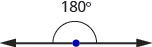
Are you familiar with the phrase ‘do a [latex]180[/latex]‘ It means to make a full turn so that you face the opposite direction. It comes from the fact that the measure of an angle that makes a straight line is [latex]180[/latex] degrees. See Figure 6.1.2.
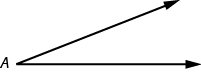
An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle and the common endpoint is called the vertex. An angle is named by its vertex. In Figure 6.1.3, [latex]A[/latex] is the angle with vertex at point [latex]A[/latex]. The measure of [latex]A[/latex] is written [latex]m\angle A[/latex].
[latex]m\angle A[/latex] is the angle with vertex at point [latex]A[/latex].
We measure angles in degrees, and use the symbol [latex]\circ[/latex] to represent degrees. We use the abbreviation [latex]m[/latex] to for the measure of an angle. So if [latex]A[/latex] is [latex]27^\circ[/latex], we would write [latex]m\angle A=27[/latex].
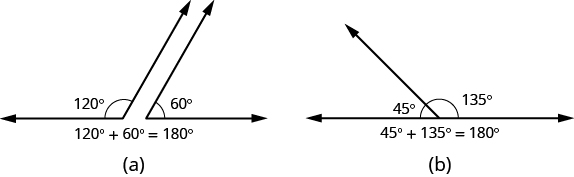
If the sum of the measures of two angles is [latex]180^\circ[/latex] then they are called supplementary angles. In Figure 6.1.4, each pair of angles is supplementary because their measures add to [latex]180^\circ[/latex]. Each angle is the supplement of the other.
The sum of the measures of supplementary angles is [latex]180^\circ[/latex].
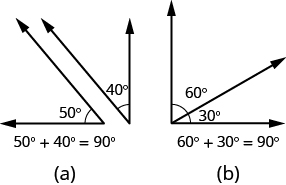
If the sum of the measures of two angles is [latex]90^\circ[/latex], then the angles are complementary angles. In Figure 6.1.5, each pair of angles is complementary, because their measures add to [latex]90^\circ[/latex]. Each angle is the complement of the other.
The sum of the measures of complementary angles is [latex]90^\circ[/latex].
Supplementary and Complementary Angles
If the sum of the measures of two angles is [latex]180^\circ[/latex] then the angles are supplementary.
If [latex]A[/latex] and [latex]B[/latex] are supplementary, then [latex]m\angle A\;+\;m\angle B\;=\;180[/latex].
If the sum of the measures of two angles is [latex]90^\circ[/latex], then the angles are complementary.
If [latex]A[/latex] and [latex]B[/latex] are complementary, then [latex]m\angle A\;+\;m\angle B\;=\;90[/latex].
In this section and the next, you will be introduced to some common geometry formulas. We will adapt our Problem Solving Strategy for Geometry Applications. The geometry formula will name the variables and give us the equation to solve.
In addition, since these applications will all involve geometric shapes, it will be helpful to draw a figure and then label it with the information from the problem. We will include this step in the Problem Solving Strategy for Geometry Applications.
How To
Use a Problem Solving Strategy for Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw a figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for and choose a variable to represent it.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
The next example will show how you can use the Problem Solving Strategy for Geometry Applications to answer questions about supplementary and complementary angles.
Example 6.1.1
An angle measures [latex]40^\circ[/latex]. Find:
a. its supplement
b. its complement
Solution
a. Step 1: Read the problem. Draw the figure and label it with the given information.
Step 2: Identify what you are looking for.
The supplement of a [latex]40^\circ[/latex] angle.
Step 3: Name. Choose a variable to represent it.
Let [latex]s=[/latex] the measure of the supplement
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}m\angle A\;+\;m\angle B\;&=&\;180\\s\;+\;40\;&=&\;180\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}s\;&=&\;140\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}140\;+\;40\;&\overset?=&\;180\\180\;&=&\;180\end{eqnarray*}[/latex]
Step 7: Answer the question.
The supplement of the [latex]40^\circ[/latex] angle is [latex]140^\circ[/latex] .
b. Step 1: Read the problem. Draw the figure and label it with the given information
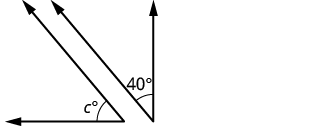
Step 2: Identify what you are looking for.
The compliment of a [latex]40^\circ[/latex] angle.
Step 3: Name. Choose a variable to represent it.
Let [latex]c=[/latex] the measure of the complement.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in given information.
[latex]\begin{eqnarray*}m\angle A\;+\;m\angle B\;&=&\;90\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}c\;+\;40\;&=&\;90\\c\;&=&\;50\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}50\;+\;40\;&\overset?=&\;90\\90\;&=&\;90\end{eqnarray*}[/latex]
Step 7: Answer the question.
The complement of the [latex]40^\circ[/latex] angle is [latex]50^\circ[/latex].
Try it
4) An angle measures [latex]25^\circ[/latex]. Find its:
a. supplement
b. complement
Solution
a. 155°
b. 65°
Try it
5) An angle measures [latex]77^\circ[/latex]. Find its:
a. supplement
b. complement
Solution
a. 103°
b. 13°
Did you notice that the words complementary and supplementary are in alphabetical order just like [latex]90[/latex] and [latex]180[/latex] are in numerical order?
Example 6.1.2
Two angles are supplementary. The larger angle is [latex]30^\circ[/latex] more than the smaller angle. Find the measure of both angles.
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
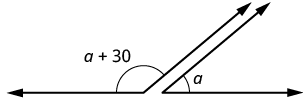
Step 2: Identify what you are looking for.
The measures of both angles.
Step 3: Name. Choose a variable to represent it.
Let [latex]a=[/latex] measure of smaller angle
[latex]a\;+\;3=[/latex] measure of larger angle
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*} m\angle A\;+\;m\angle B\;&=\;180 \end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{align*}(a\;+\;30)\;+\;a\;&=\;180\\2a\;+\;30\;&=\;180\\2a\;&=\;150\\a\;&=\;75\;measure\;of\;smaller\;angle\\a\;&+\;30\;measure\;of\;larger\;angle\\75\;+\;30\;&=\;105\\\end{align*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}m\angle A\;+\;m\angle B\;&=&\;180\\75\;+\;105\;&\overset?=&\;180\\180\;&=&\;180\end{eqnarray*}[/latex]
Step 7: Answer the question.
The measures of the angles are [latex]75^\circ[/latex] and [latex]105^\circ[/latex].
Try it
6) Two angles are supplementary. The larger angle is [latex]100^\circ[/latex] more than the smaller angle. Find the measures of both angles.
Solution
[latex]40^\circ[/latex] and [latex]140^\circ[/latex]
Try it
7) Two angles are complementary. The larger angle is [latex]40^\circ[/latex] more than the smaller angle. Find the measures of both angles.
Solution
[latex]25^\circ[/latex] and [latex]65^\circ[/latex]
Properties of Angles with Parallel Lines
It will be helpful to understand the relationship between parallel lines that are cut by a transversal line and their angles. When two lines are parallel, they will never intersect. We can use this property to find missing angles in various situations.
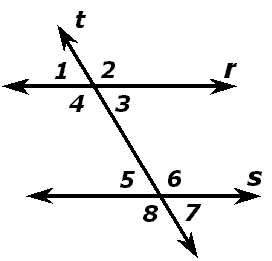
In the image above, the lines [latex]r[/latex] and [latex]s[/latex] are parallel. We can denote this mathematically using the following notation, [latex]r \parallel s[/latex]. The line [latex]t[/latex] is called a transversal line and no matter how it passes through the parallel lines, it will always result in the relationship above.
We notice that angles [latex]\angle 1[/latex], [latex]\angle 3[/latex], [latex]\angle 5[/latex], and [latex]\angle 7[/latex] will all be the same size. Similarly, angles [latex]\angle 2[/latex], [latex]\angle 4[/latex], [latex]\angle 6[/latex], and [latex]\angle 8[/latex] are all the same. Because of these properties, if we are missing certain angles we can find their values if given enough information. Before we do some examples, let’s learn some terminology.
Corresponding angles: angles that occupy the same relative position. The corresponding angles are:
[latex]\angle 1[/latex] and [latex]\angle 5[/latex]
[latex]\angle 2[/latex] and [latex]\angle 6[/latex]
[latex]\angle 3[/latex] and [latex]\angle 7[/latex]
[latex]\angle 4[/latex] and [latex]\angle 8[/latex]
Consecutive angles: angles that are pairs on the same side and inside the two parallel lines. The consecutive angles are:
[latex]\angle 3[/latex] and [latex]\angle 6[/latex]
[latex]\angle 4[/latex] and [latex]\angle 5[/latex]
Alternate interior angles: angles that are pairs on opposite sides of the transversal and inside the two parallel lines. The alternate interior angles are:
[latex]\angle 4[/latex] and [latex]\angle 6[/latex]
[latex]\angle 3[/latex] and [latex]\angle 5[/latex]
Alternate exterior angles: angles that are pairs on opposite sides of the transversal and outside the two parallel lines. The alternate exterior angles are:
[latex]\angle 1[/latex] and [latex]\angle 7[/latex]
[latex]\angle 2[/latex] and [latex]\angle 8[/latex]
Example 6.1.3
If [latex]r \parallel s[/latex], and [latex]\angle 8=110^{\circ}[/latex], find the value of all of the missing angles.
Solution
Since [latex]\angle 8=110^{\circ}[/latex] and [latex]\angle 8[/latex] and [latex]\angle 7[/latex] are supplementary angles, then
[latex]\angle 8+\angle 7=180^{\circ}[/latex] we can solve this equation for the value of [latex]\angle 7[/latex].
[latex]\begin{eqnarray*}110^{\circ}+\angle 7 &=& 180^{\circ}\\\angle 7&=& 180^{\circ}-110^{\circ}\\\angle 7&=&70^{\circ}\end{eqnarray*}[/latex]
Now, since the lines are parallel, and we know the values of [latex]\angle 8[/latex] and [latex]\angle 7[/latex], we can find all the remaining angles. By our properties, [latex]\angle 8=\angle 6=\angle 4=\angle 2=110^{\circ}[/latex] and [latex]\angle 7=\angle 5=\angle 3=\angle 170^{\circ}[/latex]
Use the Properties of Triangles
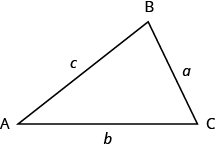
What do you already know about triangles? Triangles have three sides and three angles. Triangles are named by their vertices. The triangle in Figure 6.1.11 is called [latex]\bigtriangleup ABC[/latex], read ‘triangle ABC’. We label each side with a lower case letter to match the upper case letter of the opposite vertex.
[latex]\bigtriangleup ABC[/latex] has vertices [latex]A[/latex], [latex]B[/latex], and [latex]C[/latex] and sides [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex].
The three angles of a triangle are related in a special way. The sum of their measures is [latex]180^\circ[/latex]. [latex]m\angle A\;+\;m\angle B\;+\;m\angle C\;=\;180[/latex].
Sum of the Measures of the Angles of a Triangle
For any [latex]\bigtriangleup ABC[/latex], the sum of the measures of the angles is [latex]180^\circ[/latex]. [latex]m\angle A\;+\;m\angle B\;+\;m\angle C\;=\;180[/latex]
Example 6.1.4
The measures of two angles of a triangle are [latex]55^\circ[/latex] and [latex]82^\circ[/latex]. Find the measure of the third angle.
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
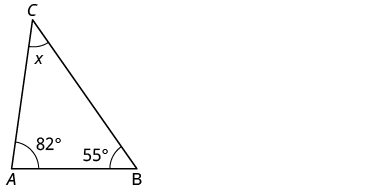
Step 2: Identify what you are looking for.
The measure of the third angle in a triangle.
Step 3: Name. Choose a variable to represent it.
Let [latex]x=[/latex] measure of the angle.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*} m\angle A\;+\;m\angle B\;+\;m\angle C\;&=&\;180 \end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}24\;&=&\;13\;+\;x\\11\;&=&\;x\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*} 55\;+\;82\;+\;43\;&\overset?=&\;180\\180\;&=&\;180\end{eqnarray*}[/latex]
Step 7: Answer the question.
The measures of the third angle is [latex]43[/latex] degrees.
Try it
8) The measures of two angles of a triangle are [latex]31^\circ[/latex] and [latex]128^\circ[/latex]. Find the measure of the third angle.
Solution
[latex]21^\circ[/latex]
Try it
9) A triangle has angles of [latex]49^\circ[/latex] and [latex]75^\circ[/latex]. Find the measure of the third angle.
Solution
[latex]56^\circ[/latex]
Because the perimeter of a figure is the length of its boundary, the perimeter of [latex]\bigtriangleup ABC[/latex] is the sum of the lengths of its three sides.
To find the area of a triangle, we need to know its base and height. The height is a line that connects the base to the opposite vertex and makes a [latex]90^\circ[/latex] angle with the base. We will draw [latex]\bigtriangleup ABC[/latex] again, and now show the height, [latex]h[/latex]. See Figure 6.1.13.
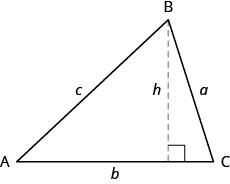
Triangle Properties
For [latex]\bigtriangleup ABC[/latex]:
Angle measures:
- The sum of the measures of the angles of a triangle is [latex]180^\circ[/latex].
Perimeter:
- The perimeter is the sum of the lengths of the sides of the triangle.
Area:
- The area of a triangle is one-half the base times the height.
Example 6.1.5
The perimeter of a triangular garden is [latex]24[/latex] feet. The lengths of two sides are four feet and nine feet. How long is the third side?
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
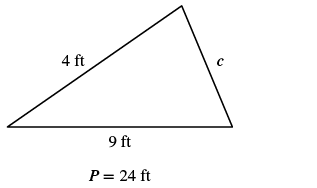
Step 2: Identify what you are looking for.
The length of the third side of a triangle.
Step 3: Name. Choose a variable to represent it.
Let [latex]c=[/latex] the third side.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*} P\;&=&\;a\;+\;b\;+\;c\\P\;&=&\;4\;+\;9\;+\;c \end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}24\;&=&\;13\;+\;c\\11\;&=&\;c\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}P\;&=&\;a\;+\;b\;+\;c\\24\;&\overset?=&\;4\;+\;9\;+\;11\\24\;&=&\;24\end{eqnarray*}[/latex]
Step 7: Answer the question.
The third side is [latex]11[/latex] feet long.
Try it
10) The perimeter of a triangular garden is [latex]48[/latex] feet. The lengths of two sides are [latex]18[/latex] feet and [latex]22[/latex] feet. How long is the third side?
Solution
[latex]8[/latex] feet
Try it
11) The lengths of two sides of a triangular window are seven feet and five feet. The perimeter is [latex]18[/latex] feet. How long is the third side?
Solution
[latex]6[/latex] feet
Example 6.1.6
The area of a triangular church window is [latex]90[/latex] square meters. The base of the window is [latex]15[/latex] meters. What is the window’s height?
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
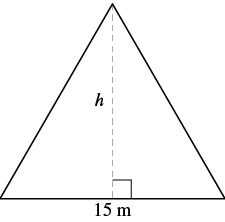
Step 2: Identify what you are looking for.
The height of a triangle.
Step 3: Name. Choose a variable to represent it.
Let [latex]h=[/latex] the height.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*} A\;&=&\;\frac12\;\times\;b\;\times\;h\\90\;&=&\;\frac12\;\times\;15\;\times\;h\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}90\;&=&\;\frac{15}2h\\12\;&=&\;h\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}A\;&=&\;\frac12bh\\90\;&\overset?=&\;\frac12\times15\times12\\90\;&=&\;90\end{eqnarray*}[/latex]
Step 7: Answer the question.
The height of the triangle is [latex]12[/latex] meters.
Try it
12) The area of a triangular painting is [latex]126[/latex] square inches. The base is [latex]18[/latex] inches. What is the height?
Solution
[latex]14[/latex] Inches
Try it
13) A triangular tent door has area [latex]15[/latex] square feet. The height is five feet. What is the base?
Solution
[latex]6[/latex] feet
Right Triangles
The triangle properties we used so far apply to all triangles. Now we will look at one specific type of triangle—a right triangle. A right triangle has one [latex]90^\circ[/latex] angle, which we usually mark with a small square in the corner, Figure 6.1.17.
If we know that a triangle is a right triangle, we know that one angle measures [latex]90^\circ[/latex] so we only need the measure of one of the other angles in order to determine the measure of the third angle.
Example 6.1.7
One angle of a right triangle measures [latex]28^\circ[/latex]. What is the measure of the third angle?
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
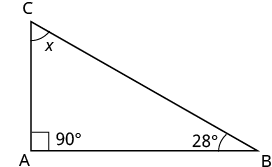
Step 2: Identify what you are looking for.
The measure of an angle.
Step 3: Name. Choose a variable to represent it.
Let [latex]x=[/latex] the measure of an angle.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}m\angle A\;+\;m\angle B\;+\;m\angle C\;&=&\;180\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}x\;+\;90\;+\;28\;&=&\;180\\x\;+\;118\;&=&\;180\\x\;&=&\;62\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}180\;&\overset?=&\;90\;+\;28\;+\;62\\180\;&=&\;180\end{eqnarray*}[/latex]
Step 7: Answer the question.
The measure of the third angle is [latex]62^\circ[/latex].
Try it
14) One angle of a right triangle measures [latex]56^\circ[/latex]. What is the measure of the other angle?
Solution
[latex]34^\circ[/latex]
Try it
15) One angle of a right triangle measures [latex]45^\circ[/latex]. What is the measure of the other angle?
Solution
[latex]45^\circ[/latex]
In the examples so far, we could draw a figure and label it directly after reading the problem. In the next example, we will have to define one angle in terms of another. So we will wait to draw the figure until we write expressions for all the angles we are looking for.
Example 6.1.8
The measure of one angle of a right triangle is [latex]20^\circ[/latex] more than the measure of the smallest angle. Find the measures of all three angles.
Solution
Step 1: Read the problem.
Step 2: Identify what you are looking for.
The measure of all three angles.
Step 3: Name. Choose a variable to represent it.
[latex]\begin{eqnarray*}Let\;a&=&\; the\; 1^{st}\; angle\\a\;+\;20\;&=&\;2^{nd}\;angle\\90\;&=&\;3^{rd}\;angle\;(the\;right\;angle)\end{eqnarray*}[/latex]
Now draw the figure and label it with the given information.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}m\angle A\;+\;m\angle B\;+\;m\angle C\;&=&\;180\\a\;+\;(a\;+\;20)\;+\;90\;&=&\;180\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}2a\;+\;110\;&=&\;180\\2a\;&=&\;70\\a\;&=&\;35\;(first\;angle)\\a\;&+&\;20\;(second\;angle)\\35\;+\;20\;&=&\;55\\90&=&(third\;angle)\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}35\;+\;55\;+\;90\;&\overset?=&180\\180\;&=&\;180\end{eqnarray*}[/latex]
Step 7: Answer the question.
The three angles measure [latex]35^\circ[/latex], [latex]55^\circ[/latex], and [latex]90^\circ[/latex].
Try it
16) The measure of one angle of a right triangle is [latex]50^\circ[/latex] more than the measure of the smallest angle. Find the measures of all three angles.
Solution
[latex]20^\circ[/latex], [latex]70^\circ[/latex], [latex]90^\circ[/latex]
Try it
17) The measure of one angle of a right triangle is [latex]30^\circ[/latex] more than the measure of the smallest angle. Find the measures of all three angles.
Solution
[latex]30^\circ[/latex], [latex]60^\circ[/latex], [latex]90^\circ[/latex]
Similar Triangles
When we use a map to plan a trip, a sketch to build a bookcase, or a pattern to sew a dress, we are working with similar figures. In geometry, if two figures have exactly the same shape but different sizes, we say they are similar figures. One is a scale model of the other. The corresponding sides of the two figures have the same ratio, and all their corresponding angles are have the same measures.
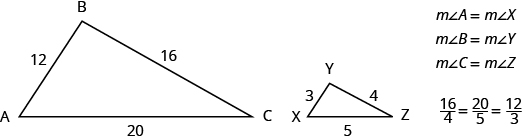
The two triangles in Figure 6.1.20 are similar. Each side of [latex]\bigtriangleup ABC[/latex] is four times the length of the corresponding side of [latex]\bigtriangleup XYZ[/latex] and their corresponding angles have equal measures.
[latex]\bigtriangleup ABC[/latex] and [latex]\bigtriangleup XYZ[/latex] are similar triangles. Their corresponding sides have the same ratio and the corresponding angles have the same measure.
Properties of Similar Triangles
If two triangles are similar, then their corresponding angle measures are equal and their corresponding side lengths are in the same ratio.
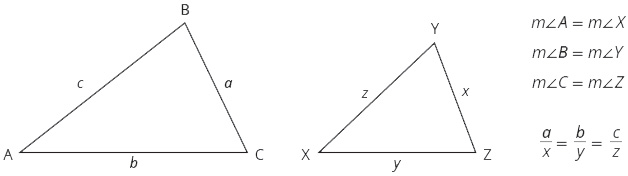
The length of a side of a triangle may be referred to by its endpoints, two vertices of the triangle. For example, in [latex]\bigtriangleup ABC[/latex]:
The length [latex]a[/latex] can also be written [latex]BC[/latex]
The length [latex]b[/latex] can also be written [latex]AC[/latex]
The length [latex]c[/latex] can also be written [latex]AB[/latex]
We will often use this notation when we solve similar triangles because it will help us match up the corresponding side lengths.
Example 6.1.9
[latex]\bigtriangleup ABC[/latex] and [latex]\bigtriangleup XYZ[/latex] are similar triangles. The lengths of two sides of each triangle are shown. Find the lengths of the third side of each triangle.
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
The figure is provided.
Step 2: Identify what you are looking for.
The length of the sides of similar triangles.
Step 3: Name. Choose a variable to represent it.
Let [latex]a=[/latex] the length of the third side of [latex]\bigtriangleup ABC[/latex].
Let [latex]y=[/latex] the length of the third side of [latex]\bigtriangleup XYZ[/latex].
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
The triangles are similar, so the corresponding sides are in the same ratio. So:
[latex]\begin{eqnarray*}\frac{AB}{XY}=\frac{BC}{YZ}=\frac{AC}{XZ}\end{eqnarray*}[/latex]
Since the side [latex]AB\;=\;4[/latex] corresponds to the side [latex]XY\;=\;3[/latex], we will use the ratio [latex]\frac{AB}{XY}=\frac43[/latex] to find the other sides.
Be careful to match up the corresponding sides correctly.
[latex]\begin{align*} &\text{Sides of large triangle (to find }a\text{):}&\frac{AB}{XY}&=\frac{BC}{YZ}\\[2ex] &\text{Sides of large triangle (to find }y\text{):}&\frac{AB}{XY}&=\frac{AC}{XZ}\\[2ex] &\text{Sides of small triangle (to find }a\text{):}&\frac43&=\frac a{4.5}\\[2ex] &\text{Sides of small triangle (to find }y\text{):}&\frac43&=\frac{3.2}y \end{align*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}3a\;&=&\;4(4.5)\\3a\;&=&\;18\\a\;&=&\;6\\\\4y\;&=&\;3(3.2)\\4y\;&=&\;9.6\\y\;&=&\;2.4\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}\frac43\;&\overset?=&\;\frac6{4.5}\\4(4.5)\;&\overset?=&\;6(3)\\18\;&=&\;18\\\\\frac43\;&\overset?=&\;\frac{3.2}{2.4}\\4(2.4)\;&\overset?=&\;3.2(3)\\9.6\;&=&\;9.6\end{eqnarray*}[/latex]
Step 7: Answer the question.
The third side of [latex]\bigtriangleup ABC[/latex] is [latex]6[/latex] and the third side of [latex]\bigtriangleup XYZ[/latex] is [latex]2.4[/latex].
Try it
18) [latex]\bigtriangleup ABC[/latex] is similar to [latex]\bigtriangleup XYZ[/latex]. Find [latex]a[/latex].
Solution
[latex]8[/latex]
Try it
19) [latex]\bigtriangleup ABC[/latex] is similar to [latex]\bigtriangleup XYZ[/latex]. Find [latex]y[/latex].
Solution
[latex]22.5[/latex]
Use the Pythagorean Theorem
The Pythagorean Theorem is a special property of right triangles that has been used since ancient times. It is named after the Greek philosopher and mathematician Pythagoras who lived around 500 BCE.
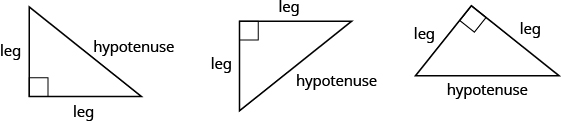
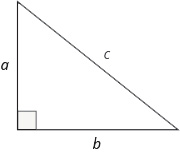
Remember that a right triangle has a [latex]90^\circ[/latex] angle, which we usually mark with a small square in the corner. The side of the triangle opposite the [latex]90^\circ[/latex] angle is called the hypotenuse, and the other two sides are called the legs. See Figure 6.1.25.
In a right triangle, the side opposite the [latex]90^\circ[/latex] angle is called the hypotenuse and each of the other sides is called a leg.
The Pythagorean Theorem tells how the lengths of the three sides of a right triangle relate to each other. It states that in any right triangle, the sum of the squares of the two legs equals the square of the hypotenuse.
The Pythagorean Theorem
In any right triangle [latex]ABC,\;a^2\;+\;b^2\;=\;c^2[/latex].
Where [latex]c[/latex] is the length of the hypotenuse [latex]a[/latex] and [latex]b[/latex] are the lengths of the legs.
To solve problems that use the Pythagorean Theorem, we will need to find square roots. In 1.6 The Real Numbers we introduced the notation [latex]\sqrt m[/latex] and defined it in this way:
If [latex]m\;=\;n^2[/latex], then [latex]\sqrt m\;=\;n[/latex] for [latex]n\;\geq\;0[/latex].
For example, we found that [latex]\sqrt{25}[/latex] is [latex]5[/latex] because [latex]5^2\;=\;25[/latex].
We will use this definition of square roots to solve for the length of a side in a right triangle.
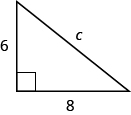
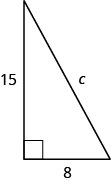
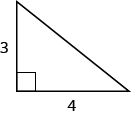
Example 6.1.10
Use the Pythagorean Theorem to find the length of the hypotenuse.
Solution
Step 1: Read the problem.
Step 2: Identify what you are looking for.
The length of the hypotenuse of the triangle.
Step 3: Name. Choose a variable to represent it.
Let [latex]c=[/latex] the length of the hypotenuse.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}a^2\;+\;b^2\;&=&\;c^2\\3^2\;+\;4^2\;&=&\;c^2\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}3a\;&=&\;4(4.5)\\3a\;&=&\;18\\a\;&=&\;6\\\\4y\;&=&\;3(3.2)\\4y\;&=&\;9.6\\y\;&=&\;2.4\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}3^2\;+\;4^2&=&\;5^2\\9\;+\;16&\overset?=&\;25\\25\;&=&\;25\end{eqnarray*}[/latex]
Step 7: Answer the question.
The length of the hypotenuse is [latex]5[/latex].
Try it
20) Use the Pythagorean Theorem to find the length of the hypotenuse.
Solution
[latex]10[/latex]
Try it
21) Use the Pythagorean Theorem to find the length of the hypotenuse.
Solution
[latex]17[/latex]
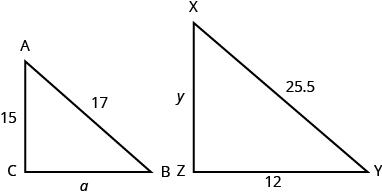
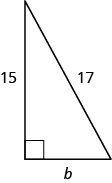
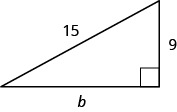
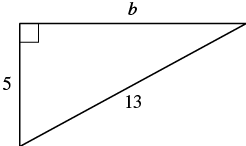
Example 6.1.11
Use the Pythagorean Theorem to find the length of the longer leg.
Solution
Step 1: Read the problem.
Step 2: Identify what you are looking for.
The length of the leg of the triangle.
Step 3: Name. Choose a variable to represent it.
Let [latex]b=[/latex] the leg of the triangle. Label side [latex]b[/latex].
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}a^2\;+\;b^2\;&=&\;c^2\\5^2\;+\;b^2\;&=&\;13^2\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}25\;+\;b^2\;&=&\;169\\b^2\;&=&\;144\\b^2\;&=&\;\sqrt{144}\\b\;&=&\;12\end{eqnarray*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}5^2\;+\;12^2\;&\overset?=&\;13^2\\25\;+\;144\;&\overset?=&\;169\\169\;&=&\;169\end{eqnarray*}[/latex]
Step 7: Answer the question.
The length of the leg is [latex]12[/latex].
Try it
22) Use the Pythagorean Theorem to find the length of the leg.
Solution
[latex]8[/latex]
Try it
23) Use the Pythagorean Theorem to find the length of the leg.
Solution
[latex]12[/latex]
Example 6.1.12
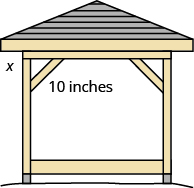
Kelvin is building a gazebo and wants to brace each corner by placing a 10-inch wooden bracket diagonally as shown. How far below the corner should he fasten the bracket if he wants the distances from the corner to each end of the bracket to be equal? Approximate to the nearest tenth of an inch.
Solution
Step 1: Read the problem.
Step 2: Identify what you are looking for.
The distance from the corner that the bracket should be attached.
Step 3: Name. Choose a variable to represent it.
Let [latex]x=[/latex] the distance from the corner.
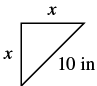
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}a^{2\;}\;+\;b^2\;&=&\;c^2\\x^2\;+\;x^2\;&=&\;10^2\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{align*} &\;&2x^2&=100\\ &\text{Isolate the variable.}&x^2&=50\\ &\text{Use the definition of the square root. Simplify.}&x&=\sqrt{50}\\ &\text{Approximate to the nearest tenth.}&b&\approx7.1 \end{align*}[/latex]
Step 6: Check.
[latex]\begin{eqnarray*}a^2\;+\;b^2\;&=&\;c^2\\7.1^{2\;}+\;7.1^{2\;}&\overset?\approx&10^2\\Yes.\end{eqnarray*}[/latex]
Step 7: Answer the question.
Kelvin should fasten each piece of wood approximately [latex]7.1[/latex]” from the corner.
Try it
24) John puts the base of a [latex]13[/latex]ft ladder [latex]5[/latex] feet from the wall of his house. How far up the wall does the ladder reach?
Solution
[latex]12[/latex] feet
Try it
25) Randy wants to attach a [latex]17[/latex] ft string of lights to the top of the [latex]15[/latex] ft mast of his sailboat. How far from the base of the mast should he attach the end of the light string?
Solution
[latex]8[/latex] feet
Key Concepts
- Supplementary and Complementary Angles
- If the sum of the measures of two angles is 180°, then the angles are supplementary.
- If [latex]\angle A[/latex] and [latex]\angle B[/latex] are supplementary, then [latex]m\angle A\;+\;m\angle B\;=\;180[/latex].
- If the sum of the measures of two angles is [latex]90^\circ[/latex], then the angles are complementary.
- If [latex]\angle A[/latex] and [latex]\angle B[/latex] are complementary, then [latex]m\angle A\;+\;m\angle B\;=\;90[/latex].
- Solve Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw a figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for and choose a variable to represent it.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
- Sum of the Measures of the Angles of a Triangle
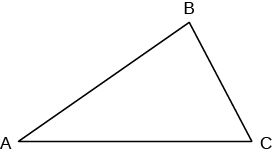
Figure 6.1.38 - For any [latex]\bigtriangleup ABC[/latex], the sum of the measures is [latex]180^\circ[/latex].
- [latex]mA+mB+mC=180[/latex].
- Right Triangle
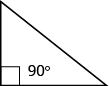
Figure 6.1.39 - A right triangle is a triangle that has one [latex]90^\circ[/latex] angle.
- Properties of Similar Triangles
- If two triangles are similar, then their corresponding angle measures are equal and their corresponding side lengths have the same ratio.
Self Check
After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
What does this checklist tell you about your mastery of this section? What steps will you take to improve?
Glossary
- angle
- An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle.
- complementary angles
- If the sum of the measures of two angles is 90°, then they are called complementary angles.
- hypotenuse
- The side of the triangle opposite the 90° angle is called the hypotenuse.
- legs of a right triangle
- The sides of a right triangle adjacent to the right angle are called the legs.
- right triangle
- A right triangle is a triangle that has one 90° angle.
- similar figures
- In geometry, if two figures have exactly the same shape but different sizes, we say they are similar figures.
- supplementary angles
- If the sum of the measures of two angles is 180°, then they are called supplementary angles.
- triangle
- A triangle is a geometric figure with three sides and three angles.
- vertex of an angle
- When two rays meet to form an angle, the common endpoint is called the vertex of the angle.
An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle.
When two rays meet to form an angle, the common endpoint is called the vertex of the angle.
Two angles are supplementary if the sum of the measures of their angles is 180 degrees.
A triangle is a geometric figure with three sides and three angles
A right triangle is a triangle that has one 90° angle.
A right triangle is a triangle that has one 90° angle.
Two angles are complementary if the sum of the measures of their angles is 90 degrees.
The side of the triangle opposite the 90° angle is called the hypotenuse.
The sides of a right triangle adjacent to the right angle are called the legs.
