Exercises: Slope of a Line (3.10)

Exercises: Use [latex]{\color{White}{m=\frac{\text{rise}}{\text{run}}}}[/latex] to find the Slope of a Line from its Graph

Instructions: For questions 1-16, find the slope of each line shown.

1.

The graph shows the x y coordinate plane. The x and y-axes run from negative 10 to 10. A line passes through the points (negative 10, negative 8), (0, negative 4), and (10, 0).
Figure 3P.10.1
Solution

[latex]\frac{2}{5}[/latex]

2.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 2, negative 8) and (2, negative 2).
Figure 3P.10.2

3.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 4, negative 6) and (4, 4).
Figure 3P.10.3
Solution

[latex]\frac{5}{4}[/latex]

4.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line intercepts the y-axis at (0, negative 2) and passes through the point (3, 3).
Figure 3P.10.4

5.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 3, 3) and (3, 1).
Figure 3P.10.5
Solution

[latex]-\frac{1}{3}[/latex]

6.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 2, 4) and (2, 2).
Figure 3P.10.6

7.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line intercepts the y-axis at (0, 6) and passes through the point (4, 3).
Figure 3P.10.7
Solution

[latex]-\frac{3}{4}[/latex]

8.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the point (negative 3, 1) and intercepts the y-axis at (0, negative 1).
Figure 3P.10.8

9.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 2, 1) and (2, 4).
Figure 3P.10.9
Solution

[latex]\frac{3}{4}[/latex]

10.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 1, 1) and (2, 3).
Figure 3P.10.10

11.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 1, 6) and (1, 1).
Figure 3P.10.11
Solution

[latex]-\frac{5}{2}[/latex]

12.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the point (negative 1, 3) and intercepts the x-axis at (3, 0).
Figure 3P.10.12

13.

The graph shows the x y coordinate plane. The x and y-axes run from negative 7 to 7. A line passes through the points (negative 2, 6) and (1, 4).
Figure 3P.10.13
Solution

[latex]-\frac{2}{3}[/latex]

14.

The graph shows the x y coordinate plane. The x and y-axes run from negative 10 to 10. A line passes through the points (negative 1, 3) and (1, 2).
Figure 3P.10.14

15.

The graph shows the x y coordinate plane. The x and y-axes run from negative 10 to 10. A line intercepts the x-axis at (negative 2, 0) and passes through the point (2, 1).
Figure 3P.10.15
Solution

[latex]\frac{1}{4}[/latex]

16.

The graph shows the x y coordinate plane. The x and y-axes run from negative 10 to 10. A line passes through the points (4, 2) and (7, 3).
Figure 3P.10.16

Exercises: Find the Slope of Horizontal and Vertical Lines

Instructions: For questions 17-24, find the slope of each line.

17. [latex]y=3[/latex]
Solution

[latex]0[/latex]

18. [latex]y=1[/latex]
19. [latex]x=4[/latex]
Solution

undefined

20. [latex]x=2[/latex]
21. [latex]y=-2[/latex]
Solution

[latex]0[/latex]

22. [latex]y=-3[/latex]
23. [latex]x=-5[/latex]
Solution

undefined

24. [latex]x=-4[/latex]

Exercises: Use the Slope Formula to find the Slope of a Line between Two Points

Instructions: For questions 25-36, use the slope formula to find the slope of the line between each pair of points.

25. [latex]\left(1,4\right),\left(3,9\right)[/latex]
Solution

[latex]\frac{5}{2}[/latex]

26. [latex]\left(2,3\right),\left(5,7\right)[/latex]
27. [latex]\left(0,3\right),\left(4,6\right)[/latex]
Solution

[latex]\frac{3}{4}[/latex]

28. [latex]\left(0,1\right),\left(5,4\right)[/latex]
29. [latex]\left(2,5\right),\left(4,0\right)[/latex]
Solution

[latex]-\frac{5}{2}[/latex]

30. [latex]\left(3,6\right),\left(8,0\right)[/latex]
31. [latex]\left(-3,3\right),\left(4,-5\right)[/latex]
Solution

[latex]-\frac{8}{7}[/latex]

32. [latex]\left(-2,4\right),\left(3,-1\right)[/latex]
33. [latex]\left(-1,-2\right),\left(2,5\right)[/latex]
Solution

[latex]\frac{7}{3}[/latex]

34. [latex]\left(-2,-1\right),\left(6,5\right)[/latex]
35. [latex]\left(4,-5\right),\left(1,-2\right)[/latex]
Solution

[latex]-1[/latex]

36. [latex]\left(3,-6\right),\left(2,-2\right)[/latex]

Exercises: Graph a Line Given a Point and the Slope

Instructions: For questions 37-52, graph each line with the given point and slope.

37. [latex]\left(1,-2\right)[/latex]; [latex]m=\frac{3}{4}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (1, negative 2) and (5, 1).
Figure 3P.10.17
38. [latex]\left(1,-1\right)[/latex]; [latex]m=\frac{2}{3}[/latex]
39. [latex]\left(2,5\right)[/latex]; [latex]m=-\frac{1}{3}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (2, 5) and (5, 4).
Figure 3P.10.18
40. [latex]\left(1,4\right)[/latex]; [latex]m=-\frac{1}{2}[/latex]
41. [latex]\left(-3,4\right)[/latex]; [latex]m=-\frac{3}{2}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (negative 3, 4) and (negative 1, 1).
Figure 3P.10.19
42. [latex]\left(-2,5\right)[/latex]; [latex]m=-\frac{5}{4}[/latex]
43. [latex]\left(-1,-4\right)[/latex]; [latex]m=\frac{4}{3}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (negative 1, negative 4) and intercepts the x-axis at (2, 0).
Figure 3P.10.20
44. [latex]\left(-3,-5\right)[/latex]; [latex]m=\frac{3}{2}[/latex]
45. [latex]y[/latex]-intercept 3; [latex]m=-\frac{2}{5}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line intercepts the y-axis at (0, 3) and passes through the point (5, 1).
Figure 3P.10.21

 

46. [latex]y[/latex]-intercept 5; [latex]m=-\frac{4}{3}[/latex]
47. [latex]x[/latex]-intercept [latex]-2[/latex]; [latex]m=\frac{3}{4}[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line intercepts the x-axis at (negative 2, 0) and passes through the point (2, 3).
Figure 3P.10.22
48. [latex]x[/latex]-intercept [latex]-1[/latex]; [latex]m=\frac{1}{5}[/latex]
49. [latex]\left(-3,3\right)[/latex]; [latex]m=2[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (negative 3, 3) and (negative 2, 5).
Figure 3P.10.23

50. [latex]\left(-4,2\right)[/latex]; [latex]m=4[/latex]
51. [latex]\left(1,5\right)[/latex]; [latex]m=-3[/latex]
Solution
The graph shows the x y coordinate plane. The x and y-axes run from negative 12 to 12. A line passes through the points (1, 5) and (2, 2).
Figure 3P.10.24

52. [latex]\left(2,3\right)[/latex]; [latex]m=-1[/latex]

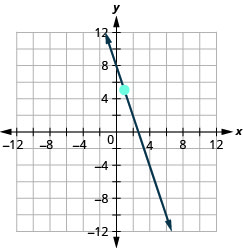
Exercises: Recognize the Relation Between the Graph and the Slope–Intercept Form of an Equation of a Line

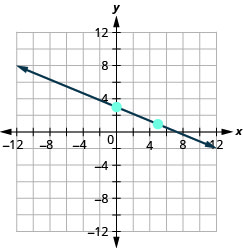
Instructions: For questions 53-58, use the graph to find the slope and y-intercept of each line. Compare the values to the equation [latex]y=mx+b[/latex].

53. [latex]y=4x-2[/latex]
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 2) and (1,2).
Figure 3P.10.25
Solution

slope [latex]m=4[/latex] and [latex]y[/latex]-intercept [latex]\left(0,-2\right)[/latex]

54. [latex]y=3x-5[/latex]

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 5) and (1, negative 2).
Figure 3P.10.26

55. [latex]y=-3x+1[/latex]

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0,1) and (1, negative 2).
Figure 3P.10.27
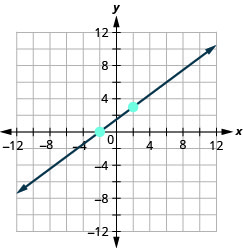
Solution

slope [latex]m=-3[/latex] and [latex]y[/latex]-intercept [latex]\left(0,1\right)[/latex]

56. [latex]y=-x+4[/latex]

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0,4) and (1,3).
Figure 3P.10.28

57. [latex]y=-\frac{2}{5}x+3[/latex]

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0,3) and (1,5).
Figure 3P.10.29
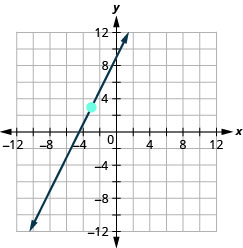
Solution

slope [latex]m=-\frac{2}{5}[/latex] and [latex]y[/latex]-intercept [latex]\left(0,3\right)[/latex]

58. [latex]y=-\frac{4}{3}x+1[/latex]

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0,1) and (3, negative 3).
Figure 3P.10.30

Exercises: Identify the Slope and [latex]{\color{White}{y}}[/latex]-Intercept From an Equation of a Line

Instructions: For questions 59-68, identify the slope and [latex]y[/latex]-intercept of each line.

59. [latex]y=-9x+7[/latex]
Solution

[latex]-9;(0,7)[/latex]

60. [latex]y=-7x+3[/latex]
61. [latex]y=4x-10[/latex]
Solution

[latex]4;(0,-10)[/latex]

62. [latex]y=6x-8[/latex]
63. [latex]4x+y=8[/latex]
Solution

[latex]-4;(0,8)[/latex]

64. [latex]3x+y=5[/latex]
65. [latex]8x+3y=12[/latex]
Solution

[latex]-\frac{8}{3};(0,4)[/latex]

66. [latex]6x+4y=12[/latex]
67. [latex]7x-3y=9[/latex]
Solution

[latex]\frac{7}{3};(0,-3)[/latex]

68. [latex]5x-2y=6[/latex]

Exercises: Graph a Line Using Its Slope and Intercept

Instructions: For questions 69-84, graph the line of each equation using its slope and y-intercept.

69. [latex]y=x+4[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, 4) and (1, 5).
Figure 3P.10.31
70. [latex]y=x+3[/latex]
71. [latex]y=2x-3[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 3) and (1, negative 1).
Figure 3P.10.32
72. [latex]y=3x-1[/latex]
73. [latex]y=-x+3[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, 3) and (1, 2).
Figure 3P.10.33
74. [latex]y=-x+2[/latex]
75. [latex]y=-x-2[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 2) and (1, negative 3).
Figure 3P.10.34
76. [latex]y=-x-4[/latex]
77. [latex]y=-\frac{2}{5}x-3[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 3) and (5, negative 5).
Figure 3P.10.35

78. [latex]y=-\frac{3}{4}x-1[/latex]
79. [latex]y=-\frac{2}{3}x+1[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0,1) and (3, negative 1).
Figure 3P.10.36
80. [latex]y=-\frac{3}{5}x+2[/latex]
81. [latex]4x-3y=6[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, negative 2) and (3,2).
Figure 3P.10.37
82. [latex]3x-4y=8[/latex]
83. [latex]y=0.3x+25[/latex]
Solution
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane runs from negative 10 to 10. The y-axis of the plane runs from negative 10 to 10. The line goes through the points (0, 25) and (negative 50, 10).
Figure 3P.10.38
84. [latex]y=0.1x+15[/latex]

Exercises: Choose the Most Convenient Method to Graph a Line

Instructions: For questions 85-100, determine the most convenient method to graph each line.

85. [latex]y=4[/latex]
Solution

horizontal line

86. [latex]x=2[/latex]
87. [latex]x=-3[/latex]
Solution

vertical line

88. [latex]y=5[/latex]
89. [latex]y=-5x+2[/latex]
Solution

slope–intercept

90. [latex]y=-3x+4[/latex]
91. [latex]x-y=1[/latex]
Solution

intercepts

92. [latex]x-y=5[/latex]
93. [latex]y=\frac{4}{5}x-3[/latex]
Solution

slope–intercept

94. [latex]y=\frac{2}{3}x-1[/latex]
95. [latex]y=-1[/latex]
Solution

horizontal line

96. [latex]y=-3[/latex]
97. [latex]2x-5y=-10[/latex]
Solution

intercepts

98. [latex]3x-2y=-12[/latex]
99. [latex]y=-\frac{1}{3}x+5[/latex]
Solution

slope–intercept

100. [latex]y=-\frac{1}{4}x+3[/latex]

Exercises: Graph and Interpret Applications of Slope–Intercept

Instructions: For questions 101-108, graph and interpret each application of slope-intercept.

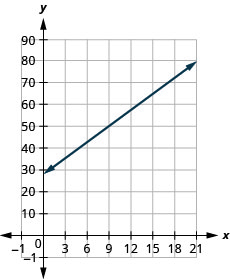
101. The equation [latex]P=28+2.54w[/latex] models the relation between the amount of Randy’s monthly water bill payment, [latex]P[/latex], in dollars, and the number of units of water, [latex]w[/latex], used.

a. Find the payment for a month when Randy used [latex]0[/latex] units of water.
b. Find the payment for a month when Randy used [latex]15[/latex] units of water.
c. Interpret the slope and [latex]P[/latex]-intercept of the equation.
d. Graph the equation.

Solution

a. [latex]$28[/latex]
b. [latex]$66.10[/latex]
c. The slope, [latex]2.54[/latex], means that Randy’s payment, [latex]P[/latex], increases by [latex]$2.54[/latex] when the number of units of water he used, [latex]w[/latex], increases by [latex]1[/latex]. The [latex]P[/latex]–intercept means that if the number units of water Randy used was [latex]0[/latex], the payment would be [latex]$28[/latex].
d.

The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane represents the variable w and runs from negative 2 to 20. The y-axis of the plane represents the variable P and runs from negative 1 to 100. The line begins at the point (0, 28) and goes through the point (15, 66.1).
Figure 3P.10.39

102. The equation [latex]P=31+1.75w[/latex] models the relation between the amount of Tuyet’s monthly water bill payment, [latex]P[/latex], in dollars, and the number of units of water, [latex]w[/latex], used.

a. Find Tuyet’s payment for a month when [latex]0[/latex] units of water are used.
b. Find Tuyet’s payment for a month when [latex]12[/latex] units of water are used.
c. Interpret the slope and [latex]P[/latex]-intercept of the equation.
d. Graph the equation.

103. Janelle is planning to rent a car while on vacation. The equation [latex]C=0.32m+15[/latex] models the relation between the cost in dollars, [latex]C[/latex], per day and the number of miles, [latex]m[/latex], she drives in one day.

a. Find the cost if Janelle drives the car [latex]0[/latex] miles one day.
b. Find the cost on a day when Janelle drives the car [latex]400[/latex] miles.
c. Interpret the slope and [latex]C[/latex]–intercept of the equation.
d. Graph the equation.

Solution

a. [latex]$15[/latex]
b. [latex]$143[/latex]
c. The slope, [latex]0.32[/latex], means that the cost, [latex]C[/latex], increases by [latex]$0.32[/latex] when the number of miles driven, [latex]m[/latex], increases by [latex]1[/latex]. The [latex]C[/latex]-intercept means that if Janelle drives [latex]0[/latex]miles one day, the cost would be [latex]$15[/latex].
d.

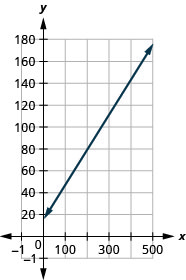
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane represents the variable m and runs from negative 1 to 500. The y-axis of the plane represents the variable C and runs from negative 1 to 200. The line begins at the point (0,15) and goes through the point (400,143).
Figure 3P.10.40

104. Bruce drives his car for his job. The equation [latex]R=0.575m+42[/latex] models the relation between the amount in dollars, [latex]R[/latex], that he is reimbursed and the number of miles, [latex]m[/latex], he drives in one day.

a. Find the amount Bruce is reimbursed on a day when he drives [latex]0[/latex] miles.
b. Find the amount Bruce is reimbursed on a day when he drives [latex]220[/latex] miles.
c. Interpret the slope and [latex]R[/latex]-intercept of the equation.
d. Graph the equation.

105. Patel’s weekly salary includes a base pay plus commission on his sales. The equation [latex]S=750+0.09c[/latex] models the relation between his weekly salary, [latex]S[/latex], in dollars and the amount of his sales, [latex]c[/latex], in dollars.

a. Find Patel’s salary for a week when his sales were [latex]$0[/latex].
b. Find Patel’s salary for a week when his sales were [latex]$18\text{,}540[/latex].
c. Interpret the slope and [latex]S[/latex]-intercept of the equation.
d. Graph the equation.

Solution

a. [latex]$750[/latex]
b. [latex]$2418.60[/latex]
c. The slope, [latex]0.09[/latex], means that Patel’s salary, [latex]S[/latex], increases by [latex]$0.09[/latex] for every [;atex]$1[/latex] increase in his sales. The [latex]S[/latex]-intercept means that when his sales are [latex]$0[/latex], his salary is [latex]$750[/latex].
d.

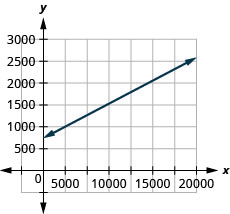
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane represents the variable w and runs from negative 1 to 20000. The y-axis of the plane represents the variable P and runs from negative 1 to 3000. The line begins at the point (0, 750) and goes through the point (18540, 2415).
Figure 3P.10.41

106. Cherie works in retail and her weekly salary includes commission for the amount she sells. The equation [latex]S=400+0.15c[/latex] models the relation between her weekly salary, [latex]S[/latex], in dollars and the amount of her sales, [latex]c[/latex], in dollars.

a. Find Cherie’s salary for a week when her sales were [latex]$0[/latex].
b. Find Cherie’s salary for a week when her sales were [latex]$3600[/latex].
c. Interpret the slope and [latex]S[/latex]–intercept of the equation.
d. Graph the equation.

107. Margie is planning a dinner banquet. The equation [latex]C=750+42g[/latex] models the relation between the cost in dollars, [latex]C[/latex] of the banquet and the number of guests, [latex]g[/latex].

a. Find the cost if the number of guests is [latex]50[/latex].
b. Find the cost if the number of guests is [latex]100[/latex].
c. Interpret the slope and [latex]C[/latex]–intercept of the equation.
d. Graph the equation.

Solution

a. [latex]$2850[/latex]
b. [latex]$4950[/latex]
c. The slope, [latex]42[/latex], means that the cost, [latex]C[/latex], increases by [latex]$42[/latex] for when the number of guests increases by [latex]1[/latex]. The [latex]C[/latex]-intercept means that when the number of guests is [latex]0[/latex], the cost would be [latex]$750[/latex].
d.

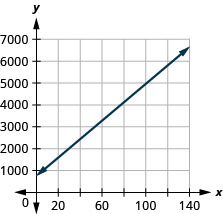
The figure shows a line graphed on the x y-coordinate plane. The x-axis of the plane represents the variable g and runs from negative 1 to 150. The y-axis of the plane represents the variable C and runs from negative 1 to 7000. The line begins at the point (0, 750) and goes through the point (100, 4950).
Figure 3P.10.42

 

108. Costa is planning a lunch banquet. The equation [latex]C=450+28g[/latex] models the relation between the cost in dollars, [latex]C[/latex], of the banquet and the number of guests,[latex]g[/latex].

a. Find the cost if the number of guests is [latex]40[/latex].
b. Find the cost if the number of guests is [latex]80[/latex].
c. Interpret the slope and [latex]C[/latex]-intercept of the equation.
d. Graph the equation.

Exercises: Use Slopes to Identify Parallel Lines

Instructions: For questions 109-134, use slopes and [latex]y[/latex]-intercepts to determine if the lines are parallel.

109. [latex]y=\frac{2}{3}x-1;\;\;2x-3y=-2[/latex]
Solution

parallel

110. [latex]y=\frac{3}{4}x-3;\;\;3x-4y=-2[/latex]
111. [latex]3x-4y=-2;\;\;y=\frac{3}{4}x-3[/latex]
Solution

parallel

112. [latex]2x-5y=-3;\;\;y=\frac{2}{5}x+1[/latex]
113. [latex]6x-3y=9;\;\;2x-y=3[/latex]
Solution

not parallel

114. [latex]2x-4y=6;\;\;x-2y=3[/latex]
115. [latex]8x+6y=6;\;\;12x+9y=12[/latex]
Solution

parallel

116. [latex]4x+2y=6;\;\;6x+3y=3[/latex]
117. [latex]x=7;\;\;x=-8[/latex]
Solution

parallel

118. [latex]x=5;\;\;x=-6[/latex]
119. [latex]x=-3;\;\;x=-2[/latex]
Solution

parallel

120. [latex]x=-4;\;\;x=-1[/latex]
121. [latex]y=5;\;\;y=1[/latex]
Solution

parallel

122. [latex]y=2;\;\;y=6[/latex]
123. [latex]y=-1;\;\;y=2[/latex]
Solution

parallel

124. [latex]y=-4;\;\;y=3[/latex]
125. [latex]4x+4y=8;\;\;x+y=2[/latex]
Solution

not parallel

126. [latex]x-y=2;\;\;2x-2y=4[/latex]
127. [latex]5x-2y=11;\;\;5x-y=7[/latex]
Solution

not parallel

128. [latex]x-3y=6;\;\;2x-6y=12[/latex]
129. [latex]4x-8y=16;\;\;x-2y=4[/latex]
Solution

not parallel

130. [latex]3x-6y=12;\;\;6x-3y=3[/latex]
131. [latex]x-5y=10;\;\;5x-y=-10[/latex]
Solution

not parallel

132. [latex]9x-3y=6;\;\;3x-y=2[/latex]
133. [latex]9x-5y=4;\;\;5x+9y=-1[/latex]
Solution

not parallel

134. [latex]7x-4y=8;\;\;4x+7y=14[/latex]

Exercises: Use Slopes to Identify Perpendicular Lines

Instructions: For questions 135-146, use slopes and [latex]y[/latex]-intercepts to determine if the lines are perpendicular.

135. [latex]x-4y=8;\;\;4x+y=2[/latex]
Solution

perpendicular

136. [latex]3x-2y=8;\;\;2x+3y=6[/latex]
137. [latex]2x+3y=5;\;\;3x-2y=7[/latex]
Solution

perpendicular

138. [latex]2x+5y=3;\;\;5x-2y=6[/latex]
139. [latex]3x-4y=8;\;\;4x-3y=6[/latex]
Solution

not perpendicular

140. [latex]3x-2y=1;\;\;2x-3y=2[/latex]
141. [latex]2x+4y=3;\;\;6x+3y=2[/latex]
Solution

not perpendicular

142. [latex]5x+2y=6;\;\;2x+5y=8[/latex]
143. [latex]2x-6y=4;\;\;12x+4y=9[/latex]
Solution

perpendicular

144. [latex]4x-2y=5;\;\;3x+6y=8[/latex]
145. [latex]8x-2y=7;\;\;3x+12y=9[/latex]
Solution

perpendicular

146. [latex]6x-4y=5;\;\;8x+12y=3[/latex]

Exercises: Everyday Math

Instructions: For questions 147-154, answer the given everyday math word problems.

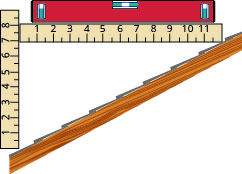
147. Slope of a roof. An easy way to determine the slope of a roof is to set one end of a [latex]12[/latex] inch level on the roof surface and hold it level. Then take a tape measure or ruler and measure from the other end of the level down to the roof surface. This will give you the slope of the roof. Builders, sometimes, refer to this as pitch and state it as an “[latex]x\;12[/latex] pitch” meaning [latex]\frac{x}{12}[/latex], where [latex]x[/latex] is the measurement from the roof to the level—the rise. It is also sometimes stated as an “[latex]x[/latex]-in-[latex]12[/latex] pitch”.

a. What is the slope of the roof in this picture?
b. What is the pitch in construction terms?

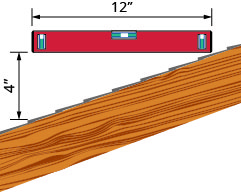
This figure shows one side of a sloped roof of a house. The rise of the roof is labeled “4 inches” and the run of the roof is labeled “12 inches”.
Figure 3P.10.43
Solution

a.[latex]\frac{1}{3}[/latex]
b. [latex]\text{4 12 pitch}[/latex] or [latex]\text{4-in-12 pitch}[/latex]

148. The slope of the roof shown here is measured with a 12” level and a ruler. What is the slope of this roof?

This figure shows one side of a sloped roof of a house. The rise of the roof is measured with a ruler and shown to be 7 inches. The run of the roof is measured with a twelve inch level and shown to be 12 inches.
Figure 3P.10.44
149. Road grade. A local road has a grade of [latex]6\%[/latex]. The grade of a road is its slope expressed as a percent. Find the slope of the road as a fraction and then simplify. What rise and run would reflect this slope or grade?
Solution

[latex]\frac{3}{50}[/latex]; [latex]\text{rise}=3[/latex], [latex]\text{run}=50[/latex]

150. Highway grade. A local road rises [latex]2[/latex] feet for every [latex]50[/latex] feet of highway.

a. What is the slope of the highway?
b. The grade of a highway is its slope expressed as a percent. What is the grade of this highway?

151. Wheelchair ramp. The rules for wheelchair ramps require a maximum [latex]1[/latex]-inch rise for a [latex]12[/latex]-inch run.

a. How long must the ramp be to accommodate a [latex]24[/latex]-inch rise to the door?
b. Create a model of this ramp.

Solution

a. [latex]288[/latex] inches ([latex]24[/latex] feet)
b. Models will vary.

152. Wheelchair ramp. A 1-inch rise for a 16-inch run makes it easier for the wheelchair rider to ascend a ramp.

a. How long must a ramp be to easily accommodate a 24-inch rise to the door?
b. Create a model of this ramp.

153. The equation [latex]n=4T-160[/latex] is used to estimate the number of cricket chirps, [latex]n[/latex], in one minute based on the temperature in degrees Fahrenheit, [latex]T[/latex].

a. Explain what the slope of the equation means.
b. Explain what the [latex]n[/latex]–intercept of the equation means. Is this a realistic situation?

Solution

a. For every increase of one degree Fahrenheit, the number of chirps increases by four.
b. There would be [latex]-160[/latex] chirps when the Fahrenheit temperature is [latex]0^\circ[/latex]. (Notice that this does not make sense; this model cannot be used for all possible temperatures.)

154. The equation [latex]C=\frac{5}{9}F-17.8[/latex] can be used to convert temperatures [latex]F[/latex], on the Fahrenheit scale to temperatures, [latex]C[/latex], on the Celsius scale.

a. Explain what the slope of the equation means.
b. Explain what the [latex]C[/latex]–intercept of the equation means.

Exercises: Writing Exercises

Instructions: For questions 155-158, answer the given writing exercises.
155. What does the sign of the slope tell you about a line?
Solution

When the slope is a positive number the line goes up from left to right. When the slope is a negative number the line goes down from left to right.

156. How does the graph of a line with slope [latex]m=\frac{1}{2}[/latex] differ from the graph of a line with slope [latex]m=2[/latex]?
157. Why is the slope of a vertical line “undefined”?
Solution

A vertical line has [latex]0[/latex] run and since division by [latex]0[/latex] is undefined the slope is undefined.

158. Explain in your own words how to decide which method to use to graph a line.
159. Why are all horizontal lines parallel?
Solution

Answers will vary.

License

Icon for the Creative Commons Attribution-ShareAlike 4.0 International License

Fanshawe Pre-Health Sciences Mathematics 1 Copyright © 2022 by Domenic Spilotro, MSc is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book