6.5 Sine, Cosine and Tangent Ratios and Applications of Trigonometry

Domenic Spilotro, MSc

6.5 Sine, Cosine and Tangent Ratios and Applications of Trigonometry

Learning Objectives

By the end of this section it is expected that you will be able to

Find missing side of a right triangle using sine, cosine, or tangent ratios
Find missing angle of a right triangle using sine, cosine, or tangent ratios
Solve applications using right angle trigonometry

Now, that we know the fundamentals of algebra and geometry associated with a right triangle, we can start exploring trigonometry. Many real life problems can be represented and solved using right angle trigonometry.

Sine, Cosine, and Tangent Ratios

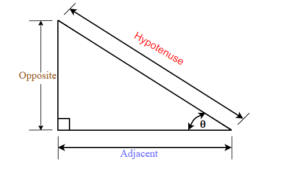
We know that any right triangle has three sides and a right angle. The side opposite to the right angle is called the hypotenuse. The other two angles in a right triangle are acute angles (with a measure less than $90$ degrees). One of those angles we call reference angle and we use $θ$ (theta) to represent it.

The hypotenuse is always the longest side of a right triangle. The other two sides are called opposite side and adjacent side. The names of those sides depends on which of the two acute angles is being used as a reference angle.

This image shows a right triangle. One of the angles is marked. The side that is shared between this angle and the 90 degree angle is called Adjacent. The other side of the angle is called Hypotenuse and the side opposite to the angle is called "opposite". — Figure 6.5.1

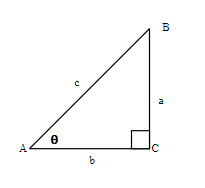
In the right triangle each side is labelled with a lowercase letter to match the uppercase letter of the opposite vertex.

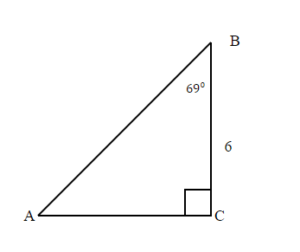
Example 6.5.1

Label the sides of the triangle and find the hypotenuse, opposite, and adjacent.

A right triangle is shown in this image. one on the angles next to the 90degree one is called "theta". — Figure 6.5.2

Solution

We labelled the sides with a lowercase letter to match the uppercase letter of the opposite vertex.

$c$ is hypotenuse
$a$ is opposite
$b$ is adjacent

A right triangle is shown in this image. one on the angles next to the 90degree one is called "theta". the side facing point A, is called lower case a. the same for points C and B — Figure 6.5.3

Try It

1) Label the sides of the triangle and find the hypotenuse, opposite and adjacent.

A right triangle is shown in this image. the 90 degree angle is called Y, the other two angles are called x and z. the angle z is marked. — Figure 6.5.4

Solution

$y$ is hypotenuse
$z$ is opposite
$x$ is adjacent

Trigonometric Ratios

Trigonometric ratios are the ratios of the sides in the right triangle. For any right triangle we can define three basic trigonometric ratios: sine, cosine, and tangent.

Let us refer to Figure 6.5.1 and define the three basic trigonometric ratios as:

Three Basic Trigonometric Ratios

- - $s i n e (θ) = \frac{the length of the opposite side}{the length of the hypotenuse side}$
  - $c o s i n e (θ) = \frac{the length of the adjacent side}{the length of the hypotenuse side}$
  - $t a n g e n t (θ) = \frac{the length of the opposite side}{the length of the adjacent side}$

Where $θ$ is the measure of a reference angle measured in degrees.

Very often we use the abbreviations for sine, cosine, and tangent ratios.

- - $s i n (θ) = \frac{o p p}{h y p}$
  - $c o s (θ) = \frac{a d j}{h y p}$
  - $t a n (θ) = \frac{o p p}{a d j}$

Some people remember the definition of the trigonometric ratios as SOH CAH TOA.

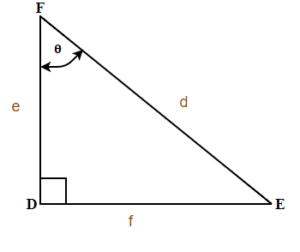
Let’s use the $Δ D E F$ from Example 6.5.2 to find the three ratios.

Example 6.5.2

For the given triangle find the sine, cosine and tangent ratio.

A right triangle is shown in this image. one on the angles next to the 90degree one is called "theta". the side facing point D, is called lower case a. the same for points E and F — Figure 6.5.5

Solution

$\begin{aligned} s i n (θ) & = \frac{f}{d} \\ c o s (θ) & = \frac{e}{d} \\ t a n (θ) & = \frac{f}{e} \end{aligned}$

Try It

2) For the given triangle find the sine cosine and tangent ratio.

Solution

$\begin{aligned} s i n (θ) & = \frac{z}{y} \\ c o s (θ) & = \frac{x}{y} \\ t a n (θ) & = \frac{z}{x} \end{aligned}$

In Example 6.5.2, our reference angles can be angle $E$ or angle $F$ . Using the definition of trigonometric ratios, we can write $s i n (E) = \frac{e}{d}$ , $c o s (E) = \frac{f}{d}$ , and $t a n (E) = \frac{e}{f}$ .

When calculating we will usually round the ratios to four decimal places and at the end our final answer to one decimal place unless stated otherwise.

Example 6.5.3

For the given triangle find the sine, cosine and tangent ratios. If necessary round to four decimal places.

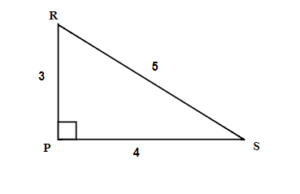
Triangle PRS. p=6, r=4, s=3 — Figure 6.5.7

Solution

We have two possible reference angles: $R$ and $S$ .

Using the definitions, the trigonometric ratios for angle $R$ are:

$s i n (R) = \frac{4}{5} = 0.8$
$c o s (R) = \frac{3}{5} = 0.6$
$t a n (R) = \frac{4}{3} = 1.3333 . . .$

Using the definitions, the trigonometric ratios for angle $S$ :

$s i n (S) = \frac{3}{5} = 0.6$
$c o s (S) = \frac{4}{5} = 0.8$
$t a n (S) = \frac{3}{4} = 0.75$

Try It

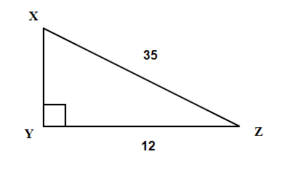
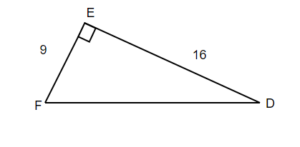
3) For the given triangle find the sine, cosine, and tangent ratios. If necessary round to four decimal places.

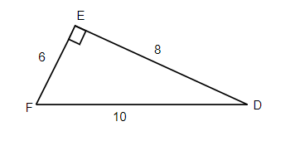
Triangle DEF. d=6, e=10, f=8 — Figure 6.5.8

Solution

$s i n (F) = \frac{8}{10} = 0.8$
$c o s (F) = \frac{6}{10} = 0.6$
$t a n (F) = \frac{8}{6} = 1.3333 . . .$
$s i n (D) = \frac{6}{10} = 0.6$
$c o s (D) = \frac{8}{10} = 0.8$
$t a n (D) = \frac{6}{8} = 0.75$

Now, let us use a scientific calculator to find the trigonometric ratios. Can you find the sin, cos, and tan buttons on your calculator? To find the trigonometric ratios make sure your calculator is in Degree Mode.

Example 6.5.4

Using a calculator find the trigonometric ratios. If necessary, round to 4 decimal places.

a) $s i n (30^{\circ})$
b) $c o s (45^{\circ})$
c) $t a n (60^{\circ})$

Solution

Make sure your calculator is in Degree Mode.

a. Using a calculator find that $s i n (30^{\circ}) = 0.5$
b. Using a calculator find that $c o s (45^{\circ}) = 0.7071$ Rounded to 4 decimal places.
c. Using a calculator find that $t a n (60^{\circ}) = 1.7321$ Rounded to 4 decimal places.

Try It

4) Find the trigonometric ratios. If necessary, round to 4 decimal places.

a. $s i n (60^{\circ})$
b. $c o s (30^{\circ})$
c. $t a n (45^{\circ})$

Solution:

a. $s i n (60^{\circ}) = 0.8660$
b. $c o s (30^{\circ}) = 0.8660$
c. $t a n (45^{\circ}) = 1$

Finding Missing Sides of a Right Triangle

In this section you will be using trigonometric ratios to solve right triangle problems. We will adapt our problem solving strategy for trigonometry applications. In addition, since those problems will involve the right triangle, it is helpful to draw it (if the drawing is not given) and label it with the given information. We will include this in the first step of the problem solving strategy for trigonometry applications.

HOW TO:

Solve Trigonometry Applications

Read the problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.
Identify what we are looking for.
Label what we are looking for by choosing a variable to represent it.
Find the required trigonometric ratio.
Solve the ratio using good algebra techniques.
Check the answer by substituting it back into the ratio in step 4 and by making sure it makes sense in the context of the problem.
Answer the question with a complete sentence

In the next few examples, having given the measure of one acute angle and the length of one side of the right triangle, we will solve the right triangle for the missing sides.

Example 6.5.5

Find the missing sides. Round your final answer to two decimal places

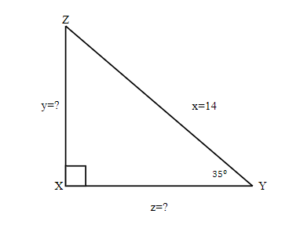
Triangle XYZ. X=90 degrees Y=35 degrees, x=14, z=?, y=? — Figure 6.5.9

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Angle $Y$ is our reference angle, $y$ is opposite side, $z$ is adjacent side, and $x = 14$ is the hypotenuse.

Step 2: Identify what you are looking for.

a. The opposite side.
b. Adjacent side.

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} y & = & ? \\ z & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} a. s i n (35^{\circ}) & = & \frac{y}{14} \\ b. c o s (35^{\circ}) & = & \frac{z}{14} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} a. 14 s i n (35^{\circ}) & = & y \\ 8.03 & = & y \\ b. 14 s i n (35^{\circ}) & = & z \\ 11.47 & = & z \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{aligned} a. 0.57 & \overset{?}{=} 8.03 \div 14 \\ 0.57 & = 0.57 ✓ \\ b. 0.82 & \overset{?}{=} 11.47 \div 14 \\ 0.82 & = 0.82 ✓ \end{aligned}$

Step 7: Answer the question with a complete sentence.

a. The opposite side is $8.03$ .
b. The adjacent side is $11.47$ .

Try It

5) Find the missing sides. Round your final answer to one decimal place.

Triangle ABC. C=90 degrees, A=51 degrees, c=26, b=?, a=? — Figure 6.5.10

Solution

$\begin{aligned} a & = 20.2 \\ b & = 16.4 \end{aligned}$

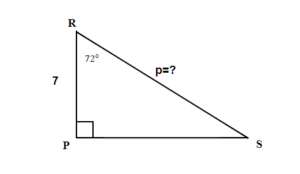
Example 6.5.6

Find the hypotenuse. Round your final answer to one decimal place.

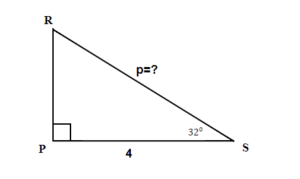
Triangle PRS. P=90 degrees, S=32 degrees, r=4, p=? — Figure 6.5.11

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Angle $S$ is our reference angle, $R$ is opposite side, $r = 4$ is the adjacent side, and $P$ is the hypotenuse.

Step 2: Identify what you are looking for.

The hypotenuse.

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} p & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} c o s (32^{\circ}) & = & \frac{4}{p} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} 0.8480 & = & \frac{4}{p} \\ p & = & 4.7170 Rounding the ratios to 4 decimal places \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{array}{rcl} 0.8480 & \overset{?}{=} & \frac{4}{4.7170} \\ 0.8480 & = & 0.8480 ✓ \end{array}$

Step 7: Answer the question with a complete sentence.

The hypotenuse is $4.7$ . Round final answer to one decimal place.

Try It

6) Find the hypotenuse. Round your final answer to one decimal place.

Triangle PRS. P=90 degrees, R=72 degrees, s=7, p=? — Figure 6.5.12

Solution

$p = 22.7$

Finding Missing Angles of a Right Triangle

Sometimes we have a right triangle with only the sides given. How can we find the missing angles? To find the missing angles, we use the inverse of the trigonometric ratios. The inverse buttons $s i n^{- 1}$ , $c o s^{- 1}$ , and $t a n^{- 1}$ are on your scientific calculator.

Example 6.5.7

Find the angles. Round your final answer to one decimal place.

a. $s i n (A) = 0.5$
b. $c o s (B) = 0.9735$
c. $t a n (C) = 2.89358$

Solution

Use your calculator and press the 2nd FUNCTION key and then press the SIN, COS, or TAN key

a. $\begin{aligned} A & = s i n^{- 1} (0.5) \\ ∠ A & = 30^{\circ} \end{aligned}$

b. $\begin{aligned} B & = c o s^{- 1} (0.9735) \\ ∠ B & = {13.2}^{\circ} \end{aligned}$ Rounded to one decimal place

c. $\begin{aligned} C & = t a n^{- 1} (2.89358) \\ ∠ C & = {70.9}^{\circ} \end{aligned}$ Rounded to one decimal place

Try It

7) Find the angles. Round your final answer to one decimal place.

a. $s i n (X) = 1$
b. $c o s (Y) = 0.375$
c. $t a n (Z) = 1.676767$

Solution

a. $∠ X = 90^{\circ}$
b. $∠ Y = 68^{\circ}$
c. $∠ Z = {59.2}^{\circ}$

In the example below we have a right triangle with two sides given. Our acute angles are missing. Let us see what the steps are to find the missing angles.

Example 6.5.8

Find the missing $∠ T$ . Round your final answer to one decimal place.

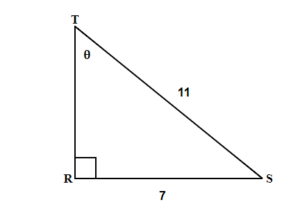
Triangle TRS. R=90 degrees, T=theta, t=7, r=11 — Figure 6.5.13

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Angle $T$ is our reference angle, $t = 7$ is the opposite side, $s$ is adjacent side, and $r = 11$ is the hypotenuse.

Step 2: Identify what you are looking for.

Angle $T$ .

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} ∠ T & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} \sin (T) & = & \frac{7}{11} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} s i n (T) & = & 0.6364 \\ T & = & s i n^{- 1} (0.6364) \\ ∠ T & = & {39.5239}^{\circ} \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{aligned} s i n ({39.523}^{\circ}) & \overset{?}{=} 0.6364 \\ 0.6364 & = 0.6364 ✓ \end{aligned}$

Step 7: Answer the question with a complete sentence.

The missing angle $T$ is ${39.5}^{\circ}$ .

Try It

8) Find the missing angle $X$ . Round your final answer to one decimal place.

Triangle XYZ Y=90 degrees, y=35, x=12 — Figure 6.5.14

Solution

${20.1}^{\circ}$

Example 6.5.9

Find the missing angle $A$ . Round your final answer to one decimal place.

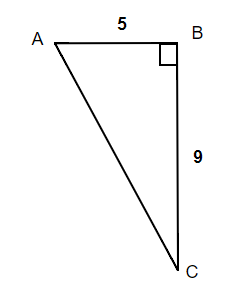
Triangle ABC B=90 degrees c=5, a=9 — Figure 6.5.15

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Angle $A$ is our reference angle, $a = 9$ is the opposite side, $c = 5$ is the adjacent side, and $b$ is the hypotenuse.

Step 2: Identify what you are looking for.

Angle $A$ .

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} ∠ A & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} t a n A & = & \frac{9}{5} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} t a n (A) & = & 1.8 \\ A & = & t a n^{- 1} (1.8) \\ ∠ A & = & {60.9}^{\circ} \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{array}{rcl} t a n {60.9}^{\circ} & \overset{?}{=} & 1.8 \\ 1.8 & = & 1.8 ✓ \end{array}$

Step 7: Answer the question with a complete sentence.

The missing angle $A$ is ${60.9}^{\circ}$ .

Try It

9) Find the missing angle $C$ . Round your final answer to one decimal place.

Solution

${29.1}^{\circ}$

Solving a Right Triangle

From the section before we know that any triangle has three sides and three interior angles. In a right triangle, when all six parts of the triangle are known, we say that the right triangle is solved.

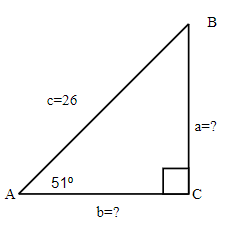
Example 6.5.10

Solve the right triangle. Round your final answer to one decimal place.

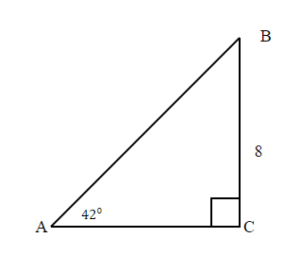
Triangle ABC C=90 degrees, A=42 degrees a=8 — Figure 6.5.17

Solution

Since the sum of angles in any triangle is $180^{\circ}$ , the measure of angle B can be easy calculated.

$\begin{aligned} ∠ B & = 180^{\circ} - 90^{\circ} - 42^{\circ} \\ ∠ B & = 48^{\circ} \end{aligned}$

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Angle $A$ is our reference angle, $a = 8$ is the opposite side, $b$ is the adjacent side, and $c$ is the hypotenuse.

Step 2: Identify what you are looking for.

a. The adjacent side.
b. The hypotenuse.

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} a. b & = & ? \\ b. c & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} a. t a n (42^{\circ}) & = & \frac{8}{b} \\ b. s i n (42^{\circ}) & = & \frac{8}{c} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} a. 0.9004 & = & \frac{8}{b} \\ 0.9004 b & = & 8 \\ b & = & 8.8849 \\ b. 0.6691 & = & \frac{8}{c} \\ 0.6691 c & = & 8 \\ c & = & 11.9563 \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{array}{rcl} a. t a n (42^{\circ}) & \overset{?}{=} & \frac{8}{8.8849} \\ 0.9 & = & 0.9 ✓ \\ b. s i n (42^{\circ}) & \overset{?}{=} & \frac{8}{11.9563} \\ 0.6691 & = & 0.6691 ✓ \end{array}$

Step 7: Answer the question with a complete sentence.

a. The adjacent side is $8.9$ . Rounded to one decimal place.
b. The hypotenuse is $12$ .

We solved the right triangle

$\begin{aligned} ∠ A & = 42^{\circ} \\ ∠ B & = 48^{\circ} \\ ∠ C & = 90^{\circ} \\ a & = 8 \\ b & = 8.9 \\ c & = 12 \end{aligned}$

Try It

10) Solve the right triangle. Round your final answer to one decimal place.

Triangle ABC C=90 degrees B=69 degrees a=6 — Figure 6.5.18

$\begin{aligned} ∠ A & = 21^{\circ} \\ ∠ B & = 69^{\circ} \\ ∠ C & = 90^{\circ} \end{aligned}$

Solution

$\begin{aligned} a & = 6 \\ b & = 15.6 \\ c & = 16.7 \end{aligned}$

Example 6.5.11

Solve the right triangle. Round to two decimal places.

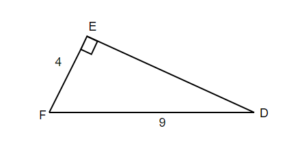
Triangle DEF E=90 degrees, e=9 d=4 — Figure 6.5.19

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

A drawing is given. Let angle $D$ be our reference angle, $d = 4$ is the opposite side, $f$ is the adjacent side, and $e = 9$ is the hypotenuse.

Step 2: Identify what you are looking for.

a. Angle D.
b. The adjacent.

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} a. ∠ D & = & ? \\ b. f & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} a. s i n (D) & = & \frac{4}{9} \\ b. 4^{2} + f^{2} & = & 9^{2} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{aligned} a. & s i n (D) & = 0.4444 \\ D & = s i n - 1 0.4444 \\ ∠ D & = {26.3850}^{\circ} \\ b. & 16 + f^{2} & = 81 \\ f^{2} & = 81 - 16 \\ f^{2} & = 65 \\ f & = \sqrt{65} \\ f & = 8.06 \end{aligned}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{aligned} a. & s i n ({26.3850}^{\circ}) & \overset{?}{=} \frac{4}{9} \\ 0.4444 & = 0.4444 ✓ \\ b. & 4^{2} + {8.06}^{2} & \overset{?}{=} 9^{2} \\ 81 & = 81 ✓ \end{aligned}$

Step 7: Answer the question with a complete sentence.

a. The missing angle $D$ is ${26.39}^{\circ}$ .
b. The adjacent side is $8.06$ . Rounded to two decimal places.

The missing angle $\begin{array}{rcl} F & = & 180^{\circ} - 90^{\circ} - {26.39}^{\circ} \\ F & = & {63.61}^{\circ} \end{array}$ .

We solved the right triangle

$\begin{aligned} ∠ D & = {26.39}^{\circ} \\ ∠ E & = 90^{\circ} \\ ∠ F & = {63.61}^{\circ} \\ d & = 4 \\ e & = 9 \\ f & = 8.06 \end{aligned}$

Try It

11) Solve the right triangle. Round to one decimal place.

Triangle DEF E=90 degrees f=16 d=9 — Figure 6.5.20

$\begin{aligned} ∠ D & = {29.3}^{\circ} \\ ∠ E & = 90^{\circ} \\ ∠ F & = {60.7}^{\circ} \end{aligned}$

Solution

$\begin{aligned} d & = 29.4 \\ e & = 18.4 \\ f & = 60.6 \end{aligned}$

Solve Applications Using Trigonometric Ratios

In the previous examples we were able to find missing sides and missing angles of a right triangle. Now, let’s use the trigonometric ratios to solve real-life problems.

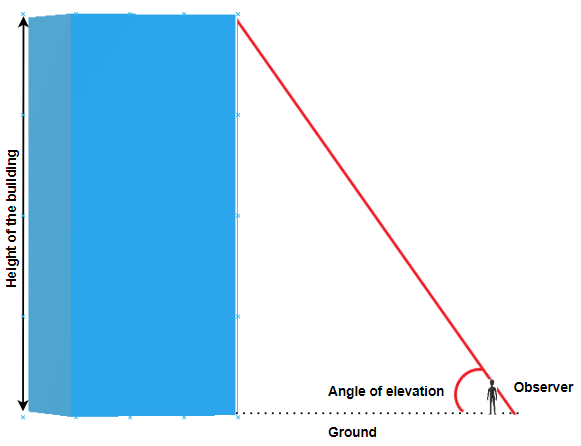
Many applications of trigonometric ratios involve understanding of an angle of elevation or angle of depression.

The angle of elevation is an angle between the horizontal line (ground) and the observer’s line of sight.

Diagram showing an observer looking up at the height of a building (angle of elevation) — Figure 6.5.21

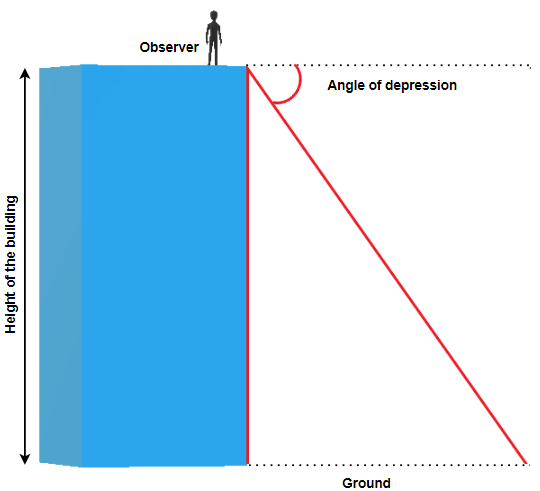
The angle of depression is the angle between horizontal line (that is parallel to the ground) and the observer’s line of sight.

An observer standing on the building looking down at the ground (angle of depression) — Figure 6.5.22

Example 6.5.12

James is standing $31$ metres away from the base of the Harbour Centre in Vancouver. He looks up to the top of the building at a $78^{\circ}$ angle. How tall is the Harbour Centre?

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

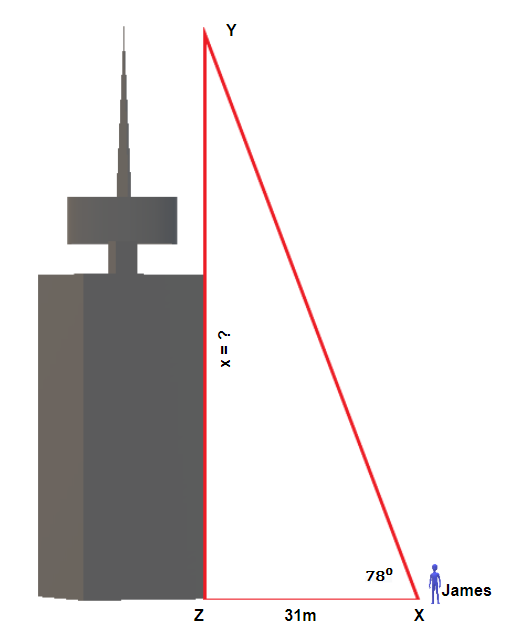
James standing on corner X, building and ground corner Z. Angle of elevation 78 degrees, he is standing 31m away from the building. Height of the building =x — Figure 6.5.23

Angle $X$ is our reference angle, $x$ is opposite side, $y = 31$ m is the adjacent side, and $z$ is the hypotenuse.

Step 2: Identify what you are looking for.

The opposite side.

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} x & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} t a n (78^{\circ}) & = & \frac{x}{31} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} 4.7046 & = & \frac{x}{31} \\ x & = & 145.8426 \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{array}{rcl} 4.7046 & \overset{?}{=} & \frac{145.8426}{31} \\ 4.7046 & = & 4.7046 ✓ \end{array}$

Step 7: Answer the question with a complete sentence.

The Harbour Centre is $145.8426$ metres or rounded to 146 metres.

Try It

12) Marta is standing $23$ metres away from the base of the tallest apartment building in Prince George and looks at the top of the building at a $62^{\circ}$ angle. How tall is the building?

Solution

$43.3$ metres

Example 6.5.13

Thomas is standing at the top of the building that is $45$ metres high and looks at her friend that is standing on the ground, $22$ metres from the base of the building. What is the angle of depression?

Solution

Step 1: Read problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.

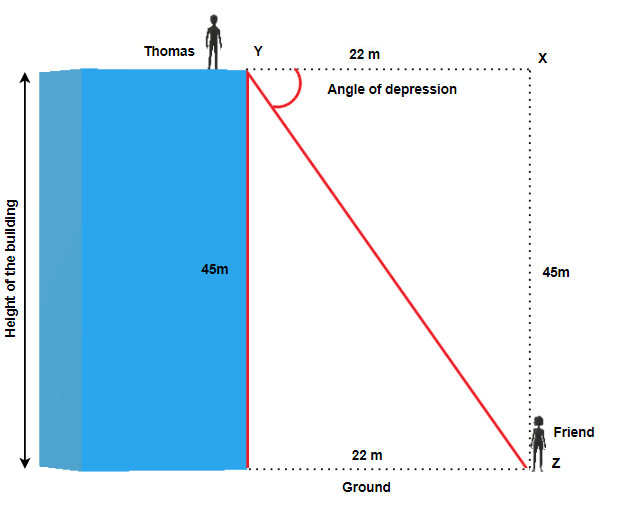
Thomas is standing on a building (45m) looking at Friend who is 22m away from the building. The angle of degressing is the angle he is looking down at — Figure 6.5.24

Angle $Y$ is our reference angle, $y = 45$ m is the opposite side, $z = 22$ m is the adjacent side, and $x$ is the hypotenuse.

Step 2: Identify what you are looking for.

The angle $Y$ .

Step 3: Label what we are looking for by choosing a variable to represent it.

$\begin{array}{rcl} ∠ Y & = & ? \end{array}$

Step 4: Find the required trigonometric ratio.

$\begin{array}{rcl} t a n (Y) & = & \frac{45}{22} \end{array}$

Step 5: Solve the ratio using good algebra techniques.

$\begin{array}{rcl} t a n (Y) & = & 2.0455 \\ Y & = & t a n^{- 1} (2.0455) \\ ∠ Y & = & {63.9470}^{\circ} \end{array}$

Step 6: Check the answer in the problem and by making sure it makes sense.

$\begin{aligned} t a n ({63.9470}^{\circ}) & \overset{?}{=} 2.0455 \\ 2.0455 & = 2.0455 ✓ \end{aligned}$

Step 7: Answer the question with a complete sentence.

The angle of depression is ${63.9470}^{\circ}$ or ${64.0}^{\circ}$ rounded to one decimal place.

Try It

13) Hemanth is standing on the top of a cliff $250$ feet above the ground and looks at his friend that is standing on the ground, $40$ feet from the base of the cliff. What is the angle of depression?

Solution

${80.9}^{\circ}$

Key Concepts

Three Basic Trigonometric Ratios: (Where $θ$ is the measure of a reference angle measured in degrees).
- $s i n e θ = \frac{the length of the opposite side}{the length of the hypotenuse side}$
- $c o s i n e θ = \frac{the length of the adjacent side}{the length of the hypotenuse side}$
- $t a n g e n t θ = \frac{the length of the opposite side}{the length of the adjacent side}$

Problem-Solving Strategy for Trigonometry Applications
1. Read the problem and make sure all the words and ideas are understood. Draw the right triangle and label the given parts.
2. Identify what we are looking for.
3. Label what we are looking for by choosing a variable to represent it.
4. Find the required trigonometric ratio.
5. Solve the ratio using good algebra techniques.
6. Check the answer by substituting it back into the ratio solved in step 5 and by making sure it makes sense in the context of the problem.
7. Answer the question with a complete sentence.

Self Check

a. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

b. Overall, after looking at the checklist, do you think you are well-prepared for the next section? Why or why not?

License

Icon for the Creative Commons Attribution-ShareAlike 4.0 International License

Fanshawe Pre-Health Sciences Mathematics 1 Copyright © 2022 by Domenic Spilotro, MSc is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License, except where otherwise noted.

Sine, Cosine, and Tangent Ratios

Trigonometric Ratios

Three Basic Trigonometric Ratios

Finding Missing Sides of a Right Triangle

HOW TO:

Solve Trigonometry Applications

Finding Missing Angles of a Right Triangle

Solving a Right Triangle

Solve Applications Using Trigonometric Ratios

Self Check

License

Share This Book