6.4 Solve Geometry Applications: Volume and Surface Area
Learning Objectives
By the end of this section, you will be able to:
- Find volume and surface area of rectangular solids
- Find volume and surface area of spheres
- Find volume and surface area of cylinders
- Find volume of cones
Try It
Before you get started, take this readiness quiz:
1) Evaluate [latex]x^3[/latex] when [latex]x\;=\;5[/latex].
2) Evaluate [latex]2^x[/latex] when [latex]x\;=\;5[/latex].
3) Find the area of a circle with radius [latex]\frac72[/latex].
In this section, we will finish our study of geometry applications. We find the volume and surface area of some three-dimensional figures. Since we will be solving applications, we will once again show our Problem-Solving Strategy for Geometry Applications.
HOW TO
Problem Solving Strategy for Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw the figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
Find Volume and Surface Area of Rectangular Solids
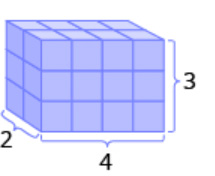
A cheerleading coach is having the squad paint wooden crates with the school colours to stand on at the games. (See Figure 6.4.1.). The amount of paint needed to cover the outside of each box is the surface area, a square measure of the total area of all the sides. The amount of space inside the crate is the volume, a cubic measure.
This wooden crate is in the shape of a rectangular solid. (Figure 6.4.1)
Each crate is in the shape of a rectangular solid. Its dimensions are the length, width, and height. The rectangular solid shown in (Figure 6.4.1) has length [latex]4[/latex] units, width [latex]2[/latex] units, and height [latex]3[/latex] units. Can you tell how many cubic units there are altogether? Let’s look layer by layer.
Breaking a rectangular solid into layers makes it easier to visualize the number of cubic units it contains. This [latex]4[/latex] by [latex]2[/latex] by [latex]3[/latex] rectangular solid has [latex]24[/latex] cubic units.
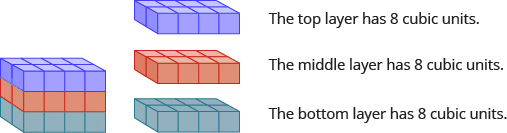
Altogether there are [latex]24[/latex] cubic units. Notice that [latex]24[/latex] is the [latex]length\;\times\;width\;\times\;height[/latex].
[latex]\begin{eqnarray*}Volume\;&=&\;L\;\times\;W\;\times\;H\\24\;&=&\;4\;\times\;2\;\times\;3\end{eqnarray*}[/latex]
The volume, [latex]V[/latex], of any rectangular solid is the product of the length, width, and height.
We could also write the formula for volume of a rectangular solid in terms of the area of the base. The area of the base, [latex]B[/latex], is equal to [latex]length\times width[/latex].
We can substitute [latex]B[/latex] for [latex]LW[/latex] in the volume formula to get another form of the volume formula.
[latex]\begin{eqnarray*}V\;&=&\;L\;\times\;W\;\times\;H\\V\;&=&\;(L\times W)\;\times\;H\\V&=&\;Bh\end{eqnarray*}[/latex]
We now have another version of the volume formula for rectangular solids. Let’s see how this works with the [latex]4\;\times\;2\;\times\;3[/latex] rectangular solid we started with. See Figure 6.4.2.
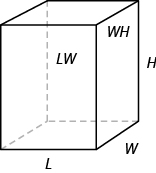
To find the surface area of a rectangular solid, think about finding the area of each of its faces. How many faces does the rectangular solid above have? You can see three of them.
| [latex]A_{front}\;=\;LW[/latex] | [latex]A_{side}\;=\;LW[/latex] | [latex]A_{top}\;=\;LW[/latex] |
| [latex]A_{front}\;=\;4\;\times\;3[/latex] | [latex]A_{side}\;=\;2\;\times\;3[/latex] | [latex]A_{top}\;=\;4\;\times\;2[/latex] |
| [latex]A_{front}\;=\;12[/latex] | [latex]A_{side}\;=\;6[/latex] | [latex]A_{top}\;=\;8[/latex] |
Notice for each of the three faces you see, there is an identical opposite face that does not show.
The surface area [latex]S[/latex] of the rectangular solid shown in Figure 6.4.2 is [latex]52[/latex] square units.
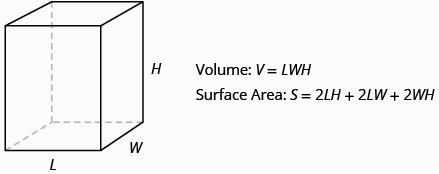
In general, to find the surface area of a rectangular solid, remember that each face is a rectangle, so its area is the product of its length and its width (see Figure 6.4.3). Find the area of each face that you see and then multiply each area by two to account for the face on the opposite side.
Volume and Surface Area of a Rectangular Solid
For a rectangular solid with length [latex]L[/latex], width [latex]W[/latex], and height [latex]H[/latex]:
Example 6.4.1
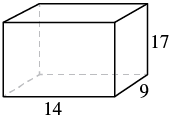
For a rectangular solid with length [latex]14[/latex] cm, height [latex]17[/latex] cm, and width [latex]9[/latex] cm, find:
a. volume
b. surface area
Solution
Step 1 is the same for both a. and b., so we will show it just once.
a. Step 1: Read the problem. Draw the figure and label it with the given information.
Step 2: Identify what you are looking for.
The volume of the rectangular solid.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}V\;&=&\;LWH\\V\;&=&\;14\;\times\;9\;\times17\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}V\;&=&\;2,142\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
We leave it to you to check your calculations.
Step 7: Answer the question.
The volume is [latex]2,142[/latex] cubic centimetres.
b. Step 2: Identify what you are looking for.
The surface area of the solid
Step 3: Name. Choose a variable to represent it.
Let [latex]S[/latex] = surface area.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}S\;&=&\;2LH\;+\;2LW\;+\;2WH\\S\;&=&\;2(14\times17)\;+\;2(14\times9)\;+\;2(9\times17)\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}S\;&=&\;1,034\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
Double-check with a calculator.
Step 7: Answer the question.
The surface area is [latex]1,034[/latex] square centimetres.
Try It
4) Find the a. volume and b. surface area of rectangular solid with the: length [latex]8[/latex] feet, width [latex]9[/latex] feet, and height [latex]11[/latex] feet.
Solution
a. [latex]792[/latex] cu. ft
b. [latex]518[/latex] sq. ft
Try It
5) Find the a. volume and b. surface area of rectangular solid with the: length [latex]15[/latex] feet, width [latex]12[/latex] feet, and height [latex]8[/latex] feet.
Solution
a. [latex]1,440[/latex] cu. ft
b. [latex]792[/latex] sq. ft
Example 6.4.2
A rectangular crate has a length of [latex]30[/latex] inches, width of [latex]25[/latex] inches, and height of [latex]20[/latex] inches. Find:
a. volume
b. surface area
Solution
Step 1 is the same for both a. and b., so we will show it just once.
a. Step 1: Read the problem. Draw the figure and label it with the given information.
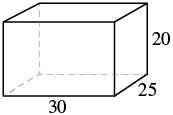
Step 2: Identify what you are looking for.
The volume of the crate.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}V\;&=&\;LWH\\V\;&=&\;30\;\times\;25\;\times\;20\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}V\;&=&\;15,000\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
Double check your math.
Step 7: Answer the question.
The volume is [latex]15,000[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the crate.
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation and substitute in the given information.
[latex]\begin{eqnarray*}S\;&=&\;2LH\;+2LW\;+2WH\\S\;&=&\;2(30\;\times20)\;+2(30\times25)\;+2(25\times20)\end{eqnarray*}[/latex]
Step 5: Solve the equation.
[latex]\begin{eqnarray*}S\;&=&\;3,700\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
Check it yourself!
Step 7: Answer the question.
The surface area is [latex]3,700[/latex] square inches.
Try It
6) A rectangular box has length [latex]9[/latex] feet, width [latex]4[/latex] feet, and height [latex]6[/latex] feet. Find its a. volume and b. surface area.
Solution
a. [latex]216[/latex] cu. ft
b. [latex]228[/latex] sq. ft
Try It
7) A rectangular suitcase has length [latex]22[/latex] inches, width [latex]14[/latex] inches, and height [latex]9[/latex] inches. Find its a. volume and b. surface area.
Solution
a. [latex]2,772[/latex] cu. in.
b. [latex]1,264[/latex] sq. in.
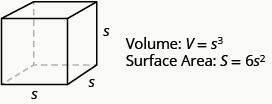
Volume and Surface Area of a Cube
A cube is a rectangular solid whose length, width, and height are equal. See Volume and Surface Area of a Cube, below. Substituting, [latex]s[/latex] for the length, width and height into the formulas for volume and surface area of a rectangular solid, we get:
| [latex]V\;=\;LWH\\\\\\[/latex] | [latex]S\;=\;2LH\;+\;2LW\;+\;2WH\\\\\\[/latex] |
| [latex]V\;=\;sss\\\\\\[/latex] | [latex]S\;=\;2ss\;+\;2ss\;+\;2ss\\\\\\[/latex] |
| [latex]V\;=\;s^3\\\\\\[/latex] | [latex]S\;=\;2s^2\;+\;2s^2\;+\;2s^2\\\\\\[/latex] |
| [latex]S\;=\;6s^2\\\\\\[/latex] |
So for a cube, the formulas for volume and surface area are [latex]V\;=\;s^3[/latex] and [latex]S\;=\;6s^2[/latex].
Example 6.4.3
A cube is [latex]2.5[/latex] inches on each side. Find:
a. volume
b. surface area
Solution
Step 1 is the same for both a. and b., so we will show it just once.
a. Step 1: Read the problem. Draw the figure and label it with the given information.

Step 2: Identify what you are looking for.
The volume of the cube.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}V\;&=&\;s^3\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&=&\;{(2.5)}^3\;\\V\;&=&\;15.625\end{eqnarray*}[/latex]
Step 6: Check — Check your work.
Step 7: Answer the question.
The volume is [latex]15.625[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the cube.
Step 3: Name. Choose a variable to represent it.
Let [latex]S[/latex] = surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}S\;&=&\;6s^2\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&=&\;6\;\times\;{(2.5)}^2\;\\S\;&=&\;37.5\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
Check is left to you.
Step 7: Answer the question.
The surface area is [latex]37.5[/latex] square inches.
Try It
8) For a cube with side [latex]4.5[/latex] meters, find:
a. volume
b. surface area of the cube
Solution
a. [latex]91.125[/latex] cu. m
b. [latex]121.5[/latex] sq. m
Try It
9) For a cube with side [latex]7.3[/latex] yards, find:
a. volume
b. surface area of the cube
Solution
a. [latex]389.017[/latex] cu. yd.
b. [latex]319.74[/latex] sq. yd.
Example 6.4.4
A notepad cube measures [latex]2[/latex] inches on each side. Find:
a. volume
b. surface area
Solution
a. Step 1: Read the problem. Draw the figure and label it with the given information.
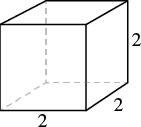
Step 2: Identify what you are looking for.
The volume of the cube.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}V\;&=&\;s^3\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&=&\;2^3\\V\;&=&\;8\end{eqnarray*}[/latex]
Step 6: Check
Check that you did the calculations correctly.
Step 7: Answer the question.
The volume is [latex]8[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the cube
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}S\;&=&\;6s^2\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&=&\;6\;\times\;{(2)}^2\;\\S\;&=&\;24\end{eqnarray*}[/latex]
Step 6: Check. Does this answer make sense?
Check is left to you.
Step 7: Answer the question.
The surface area is [latex]24[/latex] square inches.
Try It
10) A packing box is a cube measuring [latex]4[/latex] feet on each side. Find:
a. volume
b. surface area.
Solution
a. [latex]64[/latex] cu. ft
b. [latex]96[/latex] sq. ft
Try It
11) A wall is made up of cube-shaped bricks. Each cube is [latex]16[/latex] inches on each side. Find the a. volume and b. surface area of each cube.
Solution
a. [latex]4,096[/latex] cu. in.
b. [latex]1536[/latex] sq. in.
Find the Volume and Surface Area of Spheres
A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the centre of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below.
Showing where these formulas come from, like we did for a rectangular solid, is beyond the scope of this course. We will approximate [latex]\mathrm\pi[/latex] with [latex]3.14[/latex].
Volume and Surface Area of a Sphere
For a sphere with radius [latex]r[/latex]:
Example 6.4.5
A sphere has a radius [latex]6[/latex] inches. Find:
a. volume
b. surface area
Solution
Step 1 is the same for both a. and b., so we will show it just once.
a. Step 1: Read the problem. Draw the figure and label it with the given information.
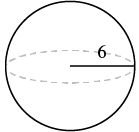
Step 2: Identify what you are looking for.
The volume of the sphere.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}V\;&=&\;\frac43\mathrm{\pi r}^3\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&\approx&\frac43(3.14)6^3\\V\;&\approx&904.32\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume is [latex]904.32[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the sphere.
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}S\;&=&\;4\mathrm{\pi r}^2\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&\approx&\;4(3.14)6^2\\S\;&\approx&\;452.16\end{eqnarray*}[/latex]
Step 6: Check math on a calculator.
Step 7: Answer the question.
The surface area is [latex]452.16[/latex] square inches.
Try It
12) Find the a. volume and b. surface area of a sphere with radius [latex]3[/latex] centimetres.
Solution
a. [latex]113.04[/latex] cu. cm
b. [latex]113.04[/latex] sq. cm
Try It
13) Find the a. volume and b. surface area of each sphere with a radius of [latex]1[/latex] foot.
Solution
a. [latex]4.19[/latex] cu. ft
b. [latex]12.56[/latex] sq. ft
Example 6.4.6
A globe of Earth is in the shape of a sphere with radius [latex]14[/latex] centimetres. Find its a. volume and b. surface area. Round the answer to the nearest hundredth.
Solution
a. Step 1: Read the problem. Draw the figure and label it with the given information.
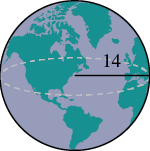
Step 2: Identify what you are looking for.
The volume of the sphere.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}V\;&=&\;\frac43\mathrm{\pi r}^3\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&\approx&\;\frac43(3.14)14^3\\V\;&\approx&\;11,488.21\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume is [latex]11,488.21[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the sphere.
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
[latex]\begin{eqnarray*}S\;&=&\;4\mathrm{\pi r}^2\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&\approx&\;4(3.14)14^2\\S\;&\approx&\;2461.76\end{eqnarray*}[/latex]
Step 6: Check math on a calculator.
Step 7: Answer the question.
The surface area is [latex]2461.76[/latex] square inches.
Try It
14) A beach ball is in the shape of a sphere with radius of [latex]9[/latex] inches. Find:
a. volume
b. surface area
Solution
a. [latex]3052.08[/latex] cu. in.
b. [latex]1017.36[/latex] sq. in.
Try It
15) A Roman statue depicts Atlas holding a globe with radius of [latex]1.5[/latex] feet. Find:
a. volume
b. surface area of the globe
Solution
a. [latex]14.13[/latex] cu. ft
b. [latex]28.26[/latex] sq. ft
Find the Volume and Surface Area of a Cylinder
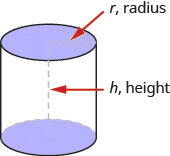
If you have ever seen a can of soda, you know what a cylinder looks like. A cylinder is a solid figure with two parallel circles of the same size at the top and bottom. The top and bottom of a cylinder are called the bases. The height [latex]h[/latex] of a cylinder is the distance between the two bases. For all the cylinders we will work with here, the sides and the height, [latex]h[/latex] , will be perpendicular to the bases.
A cylinder has two circular bases of equal size. The height is the distance between the bases.
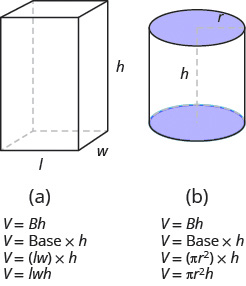
Rectangular solids and cylinders are somewhat similar because they both have two bases and a height. The formula for the volume of a rectangular solid, [latex]V=Bh[/latex], can also be used to find the volume of a cylinder.
For the rectangular solid, the area of the base, [latex]B[/latex], is the area of the rectangular base, [latex]length\times width[/latex]. For a cylinder, the area of the base, [latex]B[/latex], is the area of its circular base, [latex]\mathrm{\pi r}^2[/latex]. Figure 6.4.14 compares how the formula [latex]V=Bh[/latex] is used for rectangular solids and cylinders.
Seeing how a cylinder is similar to a rectangular solid may make it easier to understand the formula for the volume of a cylinder.
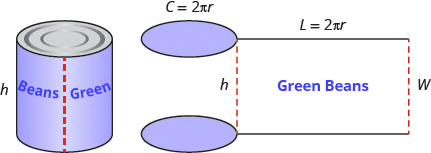
To understand the formula for the surface area of a cylinder, think of a can of vegetables. It has three surfaces: the top, the bottom, and the piece that forms the sides of the can. If you carefully cut the label off the side of the can and unroll it, you will see that it is a rectangle. See Figure 6.4.15.
By cutting and unrolling the label of a can of vegetables, we can see that the surface of a cylinder is a rectangle. The length of the rectangle is the circumference of the cylinder’s base, and the width is the height of the cylinder.
The distance around the edge of the can is the circumference of the cylinder’s base it is also the length [latex]L[/latex] of the rectangular label. The height of the cylinder is the width [latex]W[/latex] of the rectangular label. So the area of the label can be represented as:
[latex]\begin{eqnarray*}A\;&=&\;L\;\times\;W\\A\;&=&\;(2\mathrm{\pi r})\;\times\;(h)\end{eqnarray*}[/latex]
To find the total surface area of the cylinder, we add the areas of the two circles to the area of the rectangle.
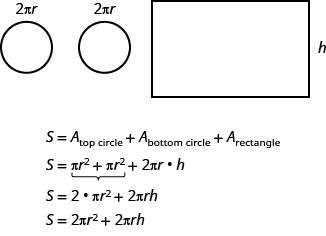
The surface area of a cylinder with radius [latex]r[/latex] and height [latex]h[/latex], is:
[latex]S\;=\;2\mathrm{\pi r}^2\;+\;2\mathrm{\pi rh}[/latex]
Volume and Surface Area of a Cylinder
For a cylinder with radius [latex]r[/latex] and height [latex]h[/latex]:

Example 6.4.7
A cylinder has height [latex]5[/latex] centimetres and radius [latex]3[/latex] centimetres. Find the a. volume and b. surface area.
Solution
a. Step 1: Read the problem. Draw the figure and label it with the given information.
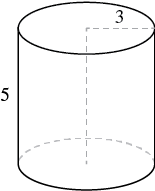
Step 2: Identify what you are looking for.
The volume of the cylinder.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}V\;&=&\;\mathrm{\pi r}^2\mathrm h\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&\approx&\;(3.14)3^2\times\;5\\V\;&\approx&\;141.3\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume is [latex]141.3[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the cylinder.
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}S\;&=&\;2\mathrm{\pi r}^2\;+\;2\mathrm{\pi rh}\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&\approx&\;2(3.14)3^2\;+\;2(3.14)(3)5\\S\;&\approx&\;150.72\end{eqnarray*}[/latex]
Step 6: Check math on a calculator.
Step 7: Answer the question.
The surface area is approximately [latex]150.72[/latex] square inches.
Try It
16) Find the a. volume and b. surface area of the cylinder with radius [latex]4[/latex] cm and height [latex]7[/latex] cm.
Solution
a. [latex]351.68[/latex] cu. cm
b. [latex]276.32[/latex] sq. cm
Try It
17) Find the a. volume and b. surface area of the cylinder with given radius [latex]2[/latex] ft and height [latex]8[/latex] ft.
Solution
a. [latex]100.48[/latex] cu. ft
b. [latex]125.6[/latex] sq. ft
Example 6.4.8
Find the a. volume and b. surface area of a can of soda. The radius of the base is [latex]4[/latex] centimetres and the height is [latex]13[/latex] centimetres. Assume the can is shaped exactly like a cylinder.
Solution
a. Step 1: Read the problem. Draw the figure and label it with the given information.
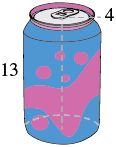
Step 2: Identify what you are looking for.
The volume of the cylinder.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}V\;&=&\;\mathrm{\pi r}^2\mathrm h\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}V\;&\approx&\;(3.14)4^2\times13\\V\;&\approx&\;653.12\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume is approximately [latex]653.12[/latex] cubic inches.
b. Step 2: Identify what you are looking for.
The surface area of the cylinder.
Step 3: Name. Choose a variable to represent it.
Let [latex]S=[/latex] surface area.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}S\;&=&\;2\mathrm{\pi r}^2\;+\;2\mathrm{\pi rh}\end{eqnarray*}[/latex]
Step 5: Solve and substitute into the equation.
[latex]\begin{eqnarray*}S\;&\approx&\;2(3.14)4^2\;+\;2(3.14)(4)13\\S\;&\approx&\;427.04\end{eqnarray*}[/latex]
Step 6: Check math on a calculator.
Step 7: Answer the question.
The surface area is approximately [latex]427.04[/latex] square inches.
Try It
18) Find the a. volume and b. surface area of a can of paint with radius [latex]8[/latex] centimetres and height [latex]19[/latex] centimetres. Assume the can is shaped exactly like a cylinder.
Solution
a. [latex]3,818.24[/latex] cu. cm
b. [latex]1,356.48[/latex] sq. cm
Try It
19) Find the a. volume and b. surface area of a cylindrical drum with radius [latex]2.7[/latex] feet and height [latex]4[/latex] feet. Assume the drum is shaped exactly like a cylinder.
Solution
a. [latex]91.5624[/latex] cu. ft
b. [latex]113.6052[/latex] sq. ft
Find the Volume of Cones
The first image that many of us have when we hear the word ‘cone’ is an ice cream cone. There are many other applications of cones (but most are not as tasty as ice cream cones). In this section, we will see how to find the volume of a cone.
In geometry, a cone is a solid figure with one circular base and a vertex. The height of a cone is the distance between its base and the vertex. The cones that we will look at in this section will always have the height perpendicular to the base. See Figure 6.4.20. The height of a cone is the distance between its base and the vertex.
Earlier in this section, we saw that the volume of a cylinder is [latex]\mathrm V\;=\; \pi \mathrm r^2\mathrm h[/latex]. We can think of a cone as part of a cylinder. Figure 6.4.21 shows a cone placed inside a cylinder with the same height and same base. If we compare the volume of the cone and the cylinder, we can see that the volume of the cone is less than that of the cylinder.
The volume of a cone is less than the volume of a cylinder with the same base and height.
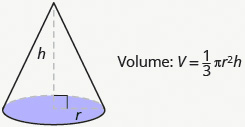
In fact, the volume of a cone is exactly one-third of the volume of a cylinder with the same base and height. The volume of a cone is:
[latex]V\;=\;\frac13Bh[/latex]
Since the base of a cone is a circle, we can substitute the formula of area of a circle, [latex]\mathrm{\pi r}^2[/latex], for [latex]B[/latex] to get the formula for volume of a cone.
[latex]\mathrm V\;=\frac13\mathrm{\pi r}^2\mathrm h[/latex]
In this book, we will only find the volume of a cone, and not its surface area.
Example 6.4.9
Find the volume of a cone with height [latex]6[/latex] inches and radius of its base [latex]2[/latex] inches.
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
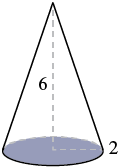
Step 2: Identify what you are looking for.
The volume of the cone.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}\mathrm V\;&=&\frac13\mathrm{\pi r}^2\mathrm h\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}\mathrm V\;&\approx&\frac13\times3.14\times{(2)}^2\times(6)\\\mathrm V\;&\approx&25.12\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume is approximately [latex]25.12[/latex] cubic inches.
Try It
20) Find the volume of a cone with height [latex]7[/latex] inches and radius [latex]3[/latex] inches
Solution
[latex]65.94[/latex] cu. in.
Try It
21) Find the volume of a cone with height [latex]9[/latex] centimetres and radius [latex]5[/latex] centimetres
Solution
[latex]235.5[/latex] cu. cm
Example 6.4.10
Marty’s favourite gastro pub serves french fries in a paper wrap shaped like a cone. What is the volume of a conic wrap that is [latex]8[/latex] inches tall and [latex]5[/latex] inches in diameter? Round the answer to the nearest hundredth.
Solution
Step 1: Read the problem. Draw the figure and label it with the given information.
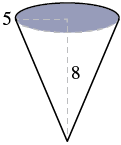
Step 2: Identify what you are looking for.
The volume of the cone.
Step 3: Name. Choose a variable to represent it.
Let [latex]V=[/latex] volume.
Step 4: Translate.
Write the appropriate formula for the situation.
Use [latex]3.14[/latex] for [latex]\mathrm\pi[/latex].
[latex]\begin{eqnarray*}\mathrm V\;&=&\frac13\mathrm{\pi r}^2\mathrm h\end{eqnarray*}[/latex]
Step 5: Solve. Substitute and Solve.
[latex]\begin{eqnarray*}\mathrm V\;&\approx&\frac13\times3.14\times{(2.5)}^2\times(8)\\\mathrm V\;&\approx&52.33\end{eqnarray*}[/latex]
Step 6: Check. Double-check your math on a calculator.
Step 7: Answer the question.
The volume of the wrap is approximately [latex]52.33[/latex] cubic inches.
Try It
22) How many cubic inches of candy will fit in a cone-shaped piñata that is [latex]18[/latex] inches long and [latex]12[/latex] inches across its base? Round the answer to the nearest hundredth.
Solution
[latex]678.24[/latex] cu. in.
Try It
23) What is the volume of a cone-shaped party hat that is [latex]10[/latex] inches tall and [latex]7[/latex] inches across at the base? Round the answer to the nearest hundredth.
Solution
[latex]128.2[/latex] cu. in.
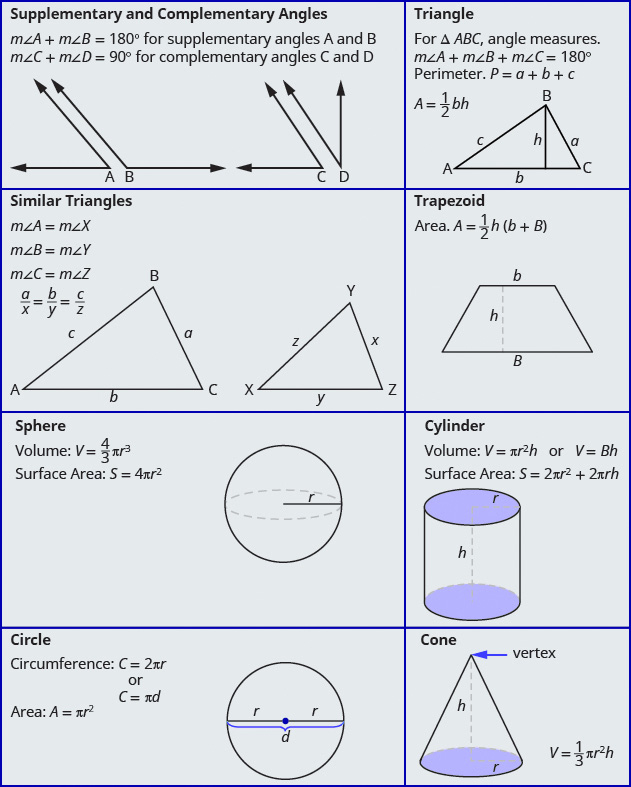
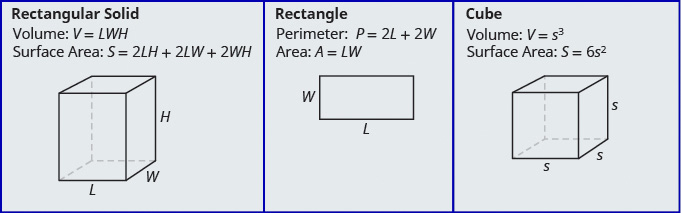
Summary of Geometry Formulas
The following charts summarize all of the formulas covered in this chapter.
Access Additional Online Resources
Key Concepts
- Volume and Surface Area of a Rectangular Solid
- [latex]V=LWH[/latex]
- [latex]S=2LH+2LW+2WH[/latex]
- Volume and Surface Area of a Cube
- [latex]V={s}^{3}[/latex]
- [latex]S=6{s}^{2}[/latex]
- Volume and Surface Area of a Sphere
- [latex]V=\frac{4}{3}\pi {r}^{3}[/latex]
- [latex]S=4\pi {r}^{2}[/latex]
- Volume and Surface Area of a Cylinder
- [latex]V=\pi {r}^{2}h[/latex]
- [latex]S=2\pi {r}^{2} 2\pi rh[/latex]
- Volume of a Cone
- For a cone with radius [latex]r[/latex] and height [latex]h[/latex]:
Volume: [latex]V=\frac{1}{3}\pi {r}^{2}h[/latex]
- For a cone with radius [latex]r[/latex] and height [latex]h[/latex]:
Self Check
After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
After reviewing this checklist, what will you do to become confident for all objectives?
Glossary
A cube is a rectangular solid whose length, width, and height are equal.
A cylinder is a solid figure with two parallel circles of the same size at the top and bottom.
A cone is a solid figure with one circular base and a vertex.