Hyperplanes and half-spaces

L. El Ghaoui

Hyperplanes and half-spaces

Hyperplanes

A hyperplane is a set described by a single scalar product equality. Precisely, an hyperplane in $\mathbb{R}^n$ is a set of the form

${\bf H} = \{x: a^Tx = b\},$

where $a \in \mathbb{R}^n$ , $a \neq 0$ , and $b \in \mathbb{R}$ are given. When $b=0$ , the hyperplane is simply the set of points that are orthogonal to $a$ ; when $b \neq 0$ , the hyperplane is a translation, along direction $a$ , of that set.

If $x_0 \in {\bf H}$ , then for any other element $x \in {\bf H}$ , we have

$b = a^Tx_0 = a^Tx.$

Hence, the hyperplane can be characterized as the set of vectors $x$ such that $x - x_0$ is orthogonal to $a$ :

${\bf H} = \{x: a^T(x-x_0) = 0\}.$

Hyperplanes are affine sets, of dimension $n-1$ (see the proof here). Thus, they generalize the usual notion of a plane in $\mathbb{R}^3$ . Hyperplanes are very useful because they allow to separate the whole space into two regions. The notion of half-space formalizes this.

Example:

A hyperplane in $\mathbb{R}^3$ .

Projection on a hyperplane

Consider the hyperplane ${\bf H} = \{x: a^Tx = b\}$ , and assume without loss of generality that $a$ is normalized ( $||a||_2 =1$ ). We can represent ${\bf H}$ as the set of points $x$ such that $x- x_0$ is orthogonal to $a$ , where $x_0$ is any vector in ${\bf H}$ , that is, such that $a^Tx_0 = b$ . One such vector is $x_{proj}:= ba$ .

By construction, $x_{proj}$ is the projection of $0$ on ${\bf H}$ . That is, it is the point on ${\bf H}$ closest to the origin, as it solves the projection problem

$\min\limits_x ||x||_2: x \in {\bf H}$

Indeed, for any $x \in {\bf H}$ , using the Cauchy-Schwartz inequality:

$||x_0||_2 = |b| = |a^Tx| \leq ||a||_2 \cdot ||x||_2 = ||x||_2,$

and the minimum length $|b|$ is attained with $x_{proj} = ba$ .

Geometry of hyperplanes

Geometrically, a hyperplane ${\bf H} = \{x: a^Tx = b\}$ , with $||a||_2 = 1$ , is a translation of the set of vectors orthogonal to $a$ . The direction of the translation is determined by $a$ , and the amount by $b$ .

Precisely, $|b|$ is the length of the closest point $x_0$ on ${\bf H}$ from the origin, and the sign of $b$ determines if ${\bf H}$ is away from the origin along the direction $a$ or $-a$ . As we increase the magnitude of $b$ , the hyperplane is shifting further away along $\pm a$ , depending on the sign of $b$ . In the image on the left, the scalar $b$ is positive, as $x_0$ and $a$ point to the same direction.

Half-spaces

A half-space is a subset of $\mathbb{R}^n$ defined by a single inequality involving a scalar product. Precisely, a half-space in $\mathbb{R}^n$ is a set of the form

${\bf H} = \{x: a^Tx \ge b\},$

where $a \in \mathbb{R}^n$ , $a \neq 0$ , and $b \in \mathbb{R}$ are given.

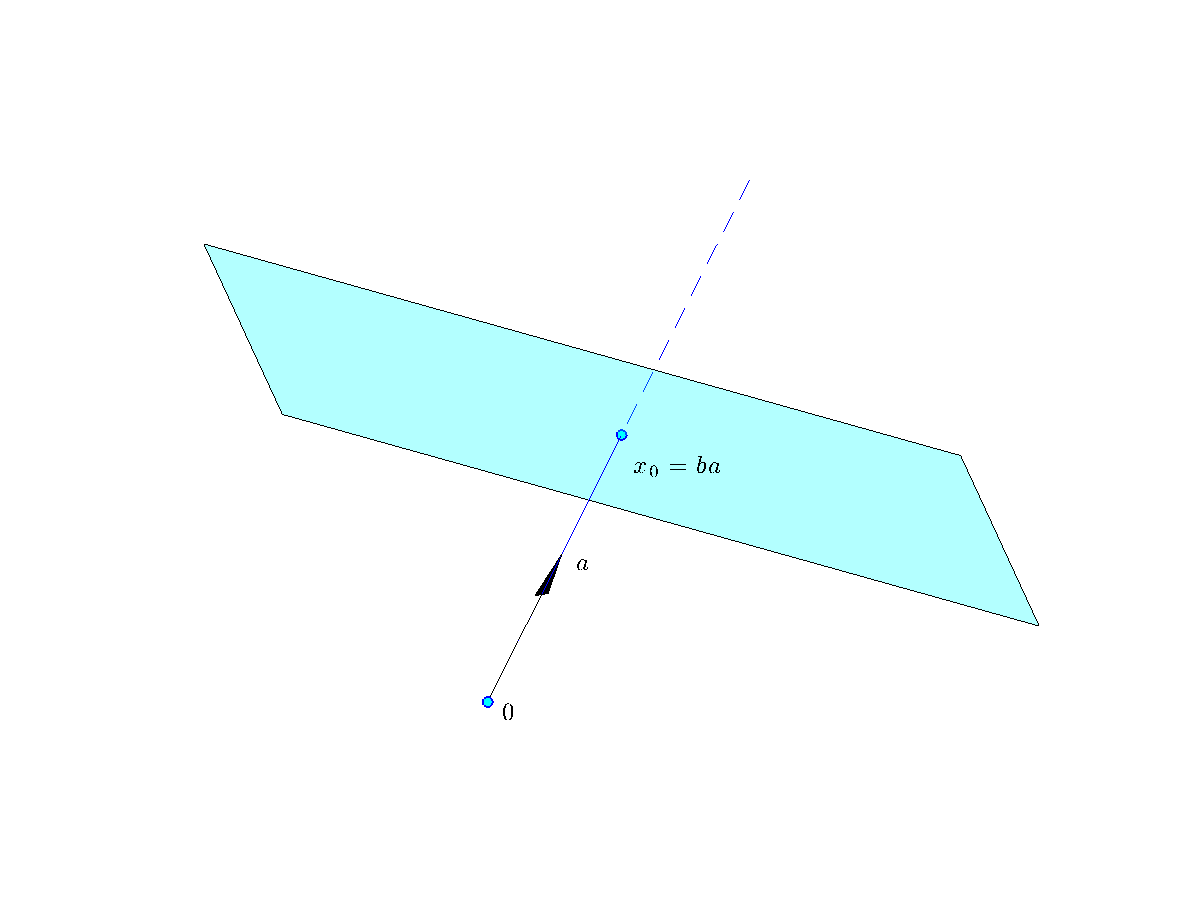
Geometrically, the half-space above is the set of points such that ${a^T(x-x_0) \ge 0$ , that is, the angle between $x - x_0$ and $a$ is acute (in $[-90^{\circ}; +90^{\circ}$ ). Here $x_0$ is the point closest to the origin on the hyperplane defined by the equality $a^Tx = b$ . (When $a$ is normalized, as in the picture, $x_0 = ba$ .)

The half-space $\{x: a^Tx \ge b\},$ is the set of points such that $x-x_0$ forms an acute angle with $a$ , where $x_0$ is the projection of the origin on the boundary of the half-space.

Hyperplanes and half-spaces

Hyperplanes

Projection on a hyperplane

Geometry of hyperplanes

Half-spaces

License

Share This Book