Hessian of a Function
Definition
The Hessian of a twice-differentiable function ![]() at a point
at a point ![]() is the matrix containing the second derivatives of the function at that point. That is, the Hessian is the matrix with elements given by
is the matrix containing the second derivatives of the function at that point. That is, the Hessian is the matrix with elements given by
The Hessian of ![]() at
at ![]() is often denoted
is often denoted ![]() .
.
The second derivative is independent of the order in which derivatives are taken. Hence, ![]() for every pair
for every pair ![]() . Thus, the Hessian is a symmetric matrix.
. Thus, the Hessian is a symmetric matrix.
Examples
Hessian of a quadratic function
Consider the quadratic function
The Hessian of ![]() at
at ![]() is given by
is given by
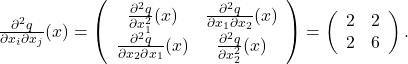
For quadratic functions, the Hessian is a constant matrix, that is, it does not depend on the point at which it is evaluated.
Hessian of the log-sum-exp function
Consider the ‘‘log-sum-exp’’ function ![]() , with values
, with values
The gradient of ![]() at
at ![]() is
is
where ![]() ,
, ![]() . The Hessian is given by
. The Hessian is given by
More generally, the Hessian of the function ![]() with values
with values
is as follows.
- First the gradient at a point
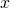 is (see here):
is (see here):
where ![]() , and
, and ![]() .
.
- Now the Hessian at a point
 is obtained by taking derivatives of each component of the gradient. If
is obtained by taking derivatives of each component of the gradient. If 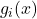 is the
is the 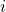 -th component, that is,
-th component, that is,
then
and, for ![]() :
:
More compactly:
