1.6 Determining Whether a Function is One-to-One
Some functions have a given output value that corresponds to two or more input values. For example, in the stock chart shown at the beginning of this chapter, the stock price was $1000 on five different dates, meaning that there were five different input values that all resulted in the same output value of $1000.
However, some functions have only one input value for each output value, as well as having only one output for each input. We call these functions one-to-one functions. As an example, consider a school that uses only letter grades and decimal equivalents, as listed in Table 1-12.
| Letter grade | Grade point average |
|---|---|
| A | 4.0 |
| B | 3.0 |
| C | 2.0 |
| D | 1.0 |
This grading system represents a one-to-one function, because each letter input yields one particular grade point average output and each grade point average corresponds to one input letter.
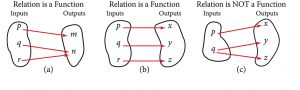
To visualize this concept, let’s look again at the two simple functions sketched in Figure 1-5 (a) and Figure 1 (b). The function in part (a) shows a relationship that is not a one-to-one function because inputs [latex]\text{}q\text{}[/latex] and [latex]\text{}r\text{}[/latex] both give output [latex]\text{}n\text{}[/latex]. The function in part (b) shows a relationship that is a one-to-one function because each input is associated with a single output.
One-to-One Function
A one-to-one function is a function in which each output value corresponds to exactly one input value.
Example 1: Determining Whether a Relationship is a One-to-One Function
Is the area of a circle a function of its radius? If yes, is the function one-to-one?
Example 2: Determining Whether a Relationship is a One-to-One Function
- Is a balance a function of the bank account number?
- Is a bank account number a function of the balance?
- Is a balance a one-to-one function of the bank account number?
Example 3: Determining Whether a Relationship is a One-to-One Function
Evaluate the following:
- If each percent grade earned in a course translates to one letter grade, is the letter grade a function of the percent grade?
- If so, is the function one-to-one?
Using the Vertical Line Test
As we have seen in some examples above, we can represent a function using a graph. Graphs display a great many input-output pairs in a small space. The visual information they provide often makes relationships easier to understand. By convention, graphs are typically constructed with the input values along the horizontal axis and the output values along the vertical axis.
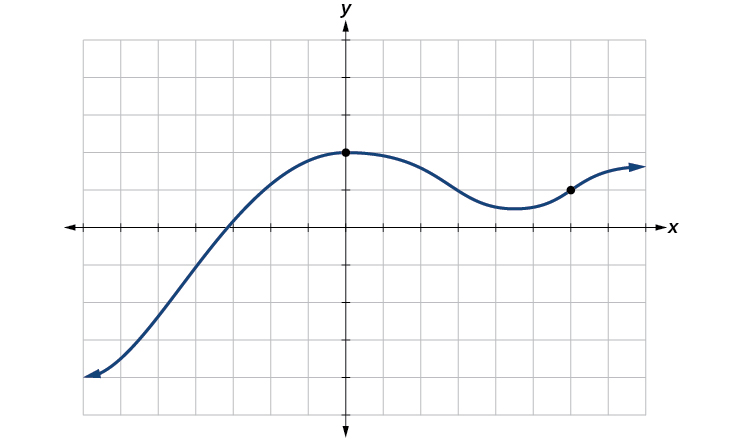
The most common graphs name the input value [latex]\text{}x\text{}[/latex] and the output value [latex]\text{}y\text{}[/latex] and we say [latex]\text{}y\text{}[/latex] is a function of [latex]\text{}x\text{}[/latex], or [latex]\text{}y=f\left(x\right)\text{}[/latex] when the function is named [latex]\text{}f\text{}[/latex]. The graph of the function is the set of all points [latex]\text{}\left(x,y\right)\text{}[/latex] in the plane that satisfies the equation [latex]y=f\left(x\right)\text{}[/latex]. If the function is defined for only a few input values, then the graph of the function is only a few points, where the x-coordinate of each point is an input value and the y-coordinate of each point is the corresponding output value. For example, the black dots on the graph in Figure 1-6 tell us that [latex]\text{}f\left(0\right)=2\text{}[/latex] and [latex]\text{}f\left(6\right)=1\text{}[/latex]. However, the set of all points [latex]\text{}\left(x,y\right)\text{}[/latex] satisfying [latex]\text{}y=f\left(x\right)\text{}[/latex] is a curve. The curve shown includes [latex]\text{}\left(0,2\right)\text{}[/latex] and [latex]\text{}\left(6,1\right)\text{}[/latex] because the curve passes through those points.
The vertical line test can be used to determine whether a graph represents a function. If we can draw any vertical line that intersects a graph more than once, then the graph does not define a function because a function has only one output value for each input value. See Figure 1-7.
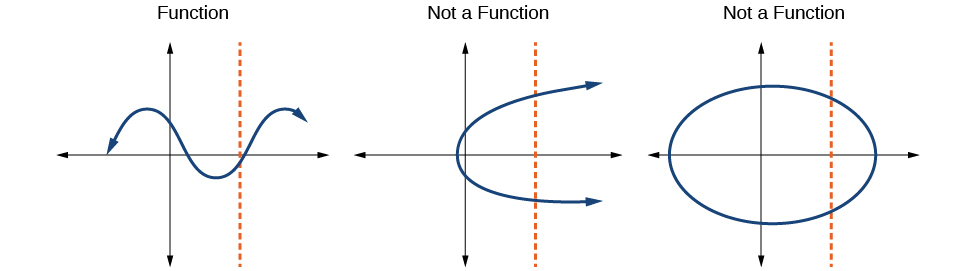
How To
Given a graph, use the vertical line test to determine if the graph represents a function.
- Inspect the graph to see if any vertical line drawn would intersect the curve more than once.
- If there is any such line, determine that the graph does not represent a function.
Example 4: Applying the Vertical Line Test
Using the Horizontal Line Test
Once we have determined that a graph defines a function, an easy way to determine if it is a one-to-one function is to use the horizontal line test. Draw horizontal lines through the graph. If any horizontal line intersects the graph more than once, then the graph does not represent a one-to-one function.
How To
Given a graph of a function, use the horizontal line test to determine if the graph represents a one-to-one function.
- Inspect the graph to see if any horizontal line drawn would intersect the curve more than once.
- If there is any such line, determine that the function is not one-to-one.
Example 5: Applying the Horizontal Line Test
- Consider the functions shown in Figure 1-10 (a) and Figure 1-10 (b). Are either of the functions one-to-one?
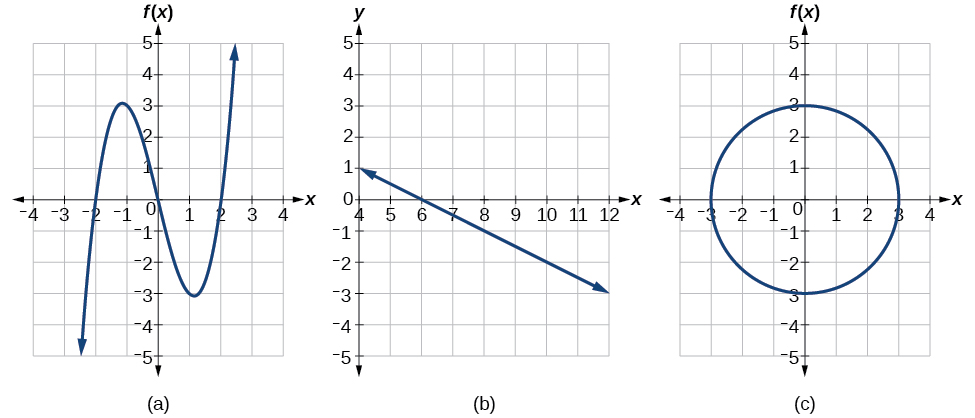
Figure 1-10 - Is the graph shown in Figure 1-10 (c) one-to-one?
Access for free at https://openstax.org/books/precalculus/pages/1-introduction-to-functions