Chapter 4 – Fractions
Map
1.1
1.19
Timeline
1.1
1.19
Introduction
Can you recall a word that sounds like “fraction” and even means the same? It is a word that we use in everyday life. Like when your friend is skiing downhill, falls and … his leg? Right, the word is “to fracture” The word “fraction” comes from the Latin word “fractio,” which means “a breaking.” It reflects the idea of breaking something into smaller parts, which is what a fraction represents.
The fractions that we use today come to us from ancient Egypt. The ancient Egyptians developed the symbols that represented parts of something like halves, quarters, thirds as opposed to wholes like one, three or four. . And one of the reasons that the Egyptians needed fractions was the fact that for thousands of years there was no real money in Egypt. In ancient world before inventing money such as coins or banknotes people used different objects to represent the cost of things. Cacao beans, cowrie shells, whale teeth, and giant stones were among objects used as money in different cultures around the globe. Early civilizations were especially fond of metals, particularly silver, gold which were used to trade and exchange goods and services. But the Egyptians didn’t bother with real objects for money. Instead of money the Egyptians used the system called barter. The barter system is where people trade goods or services for other goods and services without the use of money. For example, someone may trade grain for a fur coat, or someone may trade food in exchange for help with a harvest. Bartering can be tricky because both people must agree that the trade is fair. For most of its history, ancient Egypt’s economy operated on a barter system without cash. Instead of cash they had virtual money, believe it or not, similar to modern bitcoins! A virtual coin was called deben and everything was priced in debens. In every given time in Egypt there were known prices for almost every item imaginable, for instance, 1 cake: 1/5 deben, 1 bundle of vegetables: ½ deben, 1 shirt: 2½ deben, 1 goat: 2½ deben, 1 litre of oil: 1 deben, 1 loaf of bread: 1/10 deben, 1 slave girl: 4 deben, 1 ordinary male slave: 3 deben (yes, slaves, don’t be surprised – every civilization had slaves. Also, do you know that the word “slave” comes from old French word for slavs the people from Eastern Europe? Back in the time they were the majority of enslaved people.)
So the barter worked like that: you compare prices and trade. If a litre of oil costed one deben, and a loaf of bread was 1/10 of deben, 1 litre of oil could be traded fairly for 10 loaves of bread . In this same way, if one jug of beer costed a 1/3 deben and a day’s work was worth one deben then one would fairly be paid three jugs of beer for one’s daily labour. Let’s imagine that you are an ancient Egyptian who hired workers to work in your field or build a shed for you.
You and your workers agreed that you would pay them in beer and bread (that was the usual form of payement in ancient Egypt) and they would work for ten days If one jug of beer costed a 1/3 deben and a day’s work was worth a deben then you would pay your workers 3 jugs of beer. Or 100 loaves of bread since one loaf cost 1/10 deben. And then the workers could exchange their beer and bread for what they need, say sandals or oil or papyrus.
And we can safely say that the Egyptians were very practical in their approach to mathematics and their barter trade required that they could deal in fractions.
Egypt. Eye of Horus
Among all the fractions the Egyptians used there were six fractions considered to be sacred. These sacred fractions, 1/2, 1/4, 1/8, 1/16, 1/32, and 1/64, all with powers of two in their denominators, were used to represent the fractions of hekat, the unit measure of capacity for grains. According to a legend, the Egyptians attributed these fractions to the six parts of the eye of the god Horus the pieces were lost in a battle, and restored by the god Thoth.
Horus, the falcon-headed god, was an important god in ancient Egypt. The symbol representing his eye, known as the Eye of Horus, was a powerful symbol used to protect from evil. According to the old myth, in Egyptian mythology, Horus was the son of Isis and Osiris, king of Egypt.
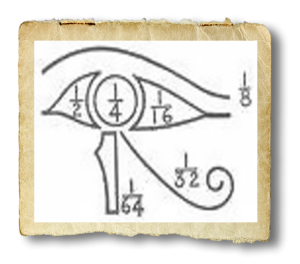
Osiris had a brother named Set, who after years of watching his brother bring prosperity and joy to the people of Egypt became jealous of his brother and killed him to claim his throne. Once he had come of age, Horus sought to reclaim the throne that was rightfully his. In a battle between Horus and Set, Set gouged out Horus’s eye and ripped it into six pieces (the fight wasn’t too one sided – Set lost a testicle).
Seeing the damage of the rightful heir to Egypt, Thoth, the wise moon god and patron of the sciences and the art of writing, volunteered to put Horus’s eye back and heal it. He collected the pieces of Horus’s eye and restored them using magic. To do so, he assigned the value of a fraction to each individual part of the eye. A whole eye represented one. the inner corner of the eye stood for 1/2, the pupil for 1/4, the eyebrow for 1/8, the outer corner for 1/16, the curling line for 1/32, and the cheek mark for 1/64. The total should have added up to a whole, but Thoth made sure that it didn’t.
He named the parts the way that one fraction was missing. Only he would know the value of the missing fraction and this would give him the ultimate power to protect the world from evil! Can you break Thot’s spell and find the missing fraction? The answer is quite simple but requires to know how to add fractions. If you add all the pieces up, you find that the sum comes to 63/64 not 64/64, 1/64 short of 1. So, after all, Thoth’s magic was not that magical!
4.1
1.1
5.1
You have to divide 8 loaves among 10 men. What will be the best way to do it?
4.2
1.1
5.1
A quantity and its [latex]\begin{array}{l}\frac{1}{2}\end{array}[/latex] added together become 16. What is the quantity?
4.3
1.1
5.1
A quantity and its [latex]\begin{array}{l}\frac{2}{3}\end{array}[/latex] are added together and from the sum [latex]\begin{array}{l}\frac{1}{3}\end{array}[/latex] of the sum is subtracted, and 10 remains. What is the quantity?
4.4
1.1
5.1
A quantity, its [latex]\begin{array}{l}\frac{1}{2}\end{array}[/latex] and its [latex]\begin{array}{l}\frac{1}{4}\end{array}[/latex], added together, become 10. What is the quantity?
4.5
1.1
5.1
A quantity together with its two-thirds has one-third of its sum taken away to yield 10. What is the quantity?
4.6
1.1
5.8
The sum of a certain quantity, together with its two-thirds, its half, and its one-seventh, becomes 37. What is the quantity?
4.7
1.1
5.9
[Given] a quantity: its two-thirds, one-half, and one-seventh are added together, giving 33. What is the quantity?
4.8
1.1
5.10
Egyptian only used unit fractions [latex]\begin{array}{l}\frac{1}{n}\end{array}[/latex] with the exception of [latex]\begin{array}{l}\frac{2}{3}\end{array}[/latex]. They would write any fraction whose numerator is not 1 as a sum of unit fractions (and [latex]\begin{array}{l}\frac{2}{3}\end{array}[/latex] if needed), e.g.
[latex]\begin{array}{l}\frac{15}{40}\end{array}[/latex] = [latex]\begin{array}{l}\frac{3}{8}\end{array}[/latex] = [latex]\begin{array}{l}\frac{2}{8}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{8}\end{array}[/latex] = [latex]\begin{array}{l}\frac{1}{4}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{8}\end{array}[/latex]
And a scribe would write in his papyrus not [latex]\begin{array}{l}\frac{15}{40}\end{array}[/latex] but [latex]\begin{array}{l}\frac{1}{4}\end{array}[/latex] [latex]\begin{array}{l}\frac{1}{8}\end{array}[/latex].
Another example:
[latex]\begin{array}{l}\frac{13}{15}\end{array}[/latex] = [latex]\begin{array}{l}\frac{10}{15}\end{array}[/latex] + [latex]\begin{array}{l}\frac{3}{15}\end{array}[/latex] = [latex]\begin{array}{l}\frac{2}{3}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{5}\end{array}[/latex]
And a scribe would write in his papyrus not [latex]\begin{array}{l}\frac{13}{15}\end{array}[/latex] but [latex]\begin{array}{l}\frac{2}{3}\end{array}[/latex] [latex]\begin{array}{l}\frac{1}{5}\end{array}[/latex].
There is another peculiar feature in the way Egyptians decomposed fractions. They never repeat the same fractions in the sums. For instance, [latex]\begin{array}{l}\frac{3}{5}\end{array}[/latex] = [latex]\begin{array}{l}\frac{1}{5}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{6}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{30}\end{array}[/latex]. The trick here is to first bring the fraction to a greater denominator [latex]\begin{array}{l}\frac{3}{5}\end{array}[/latex] = [latex]\begin{array}{l}\frac{12}{30}\end{array}[/latex] And then decompose the resulting fraction [latex]\begin{array}{l}\frac{12}{30}\end{array}[/latex] = [latex]\begin{array}{l}\frac{6}{30}\end{array}[/latex] + [latex]\begin{array}{l}\frac{5}{30}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{30}\end{array}[/latex] = [latex]\begin{array}{l}\frac{1}{5}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{6}\end{array}[/latex] + [latex]\begin{array}{l}\frac{1}{30}\end{array}[/latex].
Can you write the following fractions the way Ahmes-the-scribe wrote in the papyrus in around 1550 BC:
[latex]\begin{array}{l}\frac{2}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{3}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{4}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{5}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{6}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{7}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{8}{10}\end{array}[/latex], [latex]\begin{array}{l}\frac{9}{10}\end{array}[/latex]?
Mesopotamia. The Epic of Gilgamesh
The Epic of Gilgamesh, while not the oldest written piece of literature, is regarded as the oldest written tale in the world. It’s a poem from ancient Mesopotamia (now modern Iraq) and it is over 4,000 years old!
The Epic of Gilgamesh is the work of an anonymous Babylonian poet, about the king of the walled city of Uruk. In the story, King Gilgamesh is claimed to be part god, part human – making him the strongest and most beautiful man in the world, but with the mortality of a human being.
The young Gilgamesh is widely disliked in his kingdom He terrorizes his people, sleeps with the brides of his subjects on their wedding night, and consistently uses force to get his way in all things. The gods decide to humble him by creating the wild man, Enkidu. The gods answer by creating a man – one that is equal to Gilgamesh in strength, yet his opposite. Enkidu was their creation, brought to life from water and clay, and was just as wild as Gilgamesh – but with complete innocence. Enkidu was raised by the animals of the forest, completely ignorant of humans – until a sacred priestess by the name of Shamhat introduces him to the ways of humanity and civilisation.
Once tamed and introduced to civilization, Enkidu is outraged by the stories he hears of Gilgamesh and his arrogance and travels to Uruk to challenge him. Enkidu and Gilgamesh are considered an even match by the people, but after an epic battle, Enkidu is bested. He freely accepts his defeat, and the two become best friends Their friendship tames Gilgamesh a little bit, much to the relief of his people.
In order to make his name immortal, Gilgamesh decided to travel with Enkidu to the Cedar Forest to kill the guardian of the forest monster-demon Humbaba. Humbaba has done nothing wrong and is favored by the gods for his protection of the forest, but this means nothing to Gilgamesh, who is only thinking of himself. Once the two friends have defeated Humbaba, Humbaba cries out for mercy, but Enkidu encourages Gilgamesh to kill him, which he does.
The friends return to Uruk where Gilgamesh prepares to celebrate his victory, putting on his finest clothes. This attracts the attention of the goddess Ishtar. Ishtar is enraged and sends the Bull of Heaven, down to earth to destroy Uruk and Gilgamesh. The two heroes kill the bull and Enkidu flings one of its legs at Ishtar in contempt. For this affront to a deity, as well as his cruelty to Humbaba, the gods decree Enkidu must die.
Enkidu lingers in pain for some time, and when he dies, Gilgamesh falls into deep grief. Recognizing his own mortality through the death of his friend, he questions the meaning of life and the value of human accomplishment in the face of ultimate extinction. He cries:
How can I rest, how can I be at peace? Despair is in my heart. What my brother is now, that shall I be when I am dead. Because I am afraid of death I will go as best I can to find Utnapishtim whom they call the Faraway, for he has entered the assembly of the gods.
Casting away all of his old vanity and pride, Gilgamesh sets out on a quest to find the meaning of life and, finally, some way of defeating death. He travels far, through the mountains and past the Scorpion People, hoping to find Utnapishtim, the man who survived the Great Flood and was rewarded with immortality by the gods. At one point, he meets the goddess of wine-making and brewing Siduri who tells him his quest is in vain and he should accept life as it is and enjoy the pleasures it has to offer. Gilgamesh rejects her advice, however, as he believes life is meaningless if one must eventually lose all that one loves.
Siduri directs him to the ferryman Urshanabi, who takes him across the waters of death to the home of Utnapishtim. Utnapishtim tells him that there is nothing he can do for him. He, Utnapishtim, was granted immortality by the gods, he says, and has no power to do the same for Gilgamesh. Even so, Utnapishtim offers Gilgamesh two chances at eternal life. First, he must show himself worthy by staying awake for six days and nights, which Gilgamesh fails at, and then he is given a magic plant which, in a moment of carelessness, he leaves on the shore while he bathes, and it is eaten by a snake. Having failed in his quest, Gilgamesh has Urshanabi bring him back to Uruk, where he has a dream in which the father of the gods, Enlil tells him: ‘You were given the kingship, such was your destiny, everlasting life was not your destiny. Because of this do not be sad at heart, do not be grieved or oppressed; he has given you power to bind and to loose, to be the darkness and the light of mankind. … But do not abuse this power, deal justly with your servants in the palace, deal justly before the face of the Sun.’
And that is how Gilgamesh, the king of Uruk has lived, until his time came to be laid in the tomb.</p.
4.9
1.1
5.7
A woman weaves a textile that is to be 48 rods long. In one day, she weaves [latex]\begin{array}{l}\frac{1}{3}\end{array}[/latex] rod. In how many days will she cut the textile from the loom [be finished]?
4.10
1.1
5.8
Two-thirds of two-thirds of a certain quantity of barley is taken, and we obtain 100 units. What was the original quantity?
4.11
1.1
5.9
It is known that the digging of a canal becomes more difficult the deeper one goes. In order to compensate for this fact, differential work allotments are computed: a laborer working at the top level is expected to remove [latex]\begin{array}{l}\frac{1}{3}\end{array}[/latex] sar of earth in one day, while a laborer at the middle level removes [latex]\begin{array}{l}\frac{1}{6}\end{array}[/latex] sar, and one at the bottom level, [latex]\begin{array}{l}\frac{1}{9}\end{array}[/latex] sar. If a fixed amount of the earth is to be removed from the canal in one day, how much digging time should be spent at each level?
4.12
1.1
5.10
I have two fields of grain. From the first field, I harvest two-thirds of a bushel of grain per unit area; from the second, one-half a bushel per unit area. The yield of the first exceeds the second by 50 bushels. The total area of the two fields together is 300 units. What is the area of each field?
4.13
1.1
5.8
There are two silver rings; [latex]\begin{array}{l}\frac{1}{7}\end{array}[/latex] of the first and [latex]\begin{array}{l}\frac{1}{11}\end{array}[/latex] of the second ring is broken off, so that what is broken off weighs one shekel. The first that is diminished by [latex]\begin{array}{l}\frac{1}{7}\end{array}[/latex] weighs as much as the second diminished by its [latex]\begin{array}{l}\frac{1}{11}\end{array}[/latex]. What was the weight of the silver rings originally?
Islamic Empire. 1001 Nights or Arabian Nights
We have all heard the story of Aladdin and the Magic Lamp. It has sparked our collective fascination for generations whether through Disney’s famous animated film or the earliest cinematic depiction from all the way back in 1917. The mystique of distant adventures from exotic lands has enticed us into the worlds of Ali Baba and the Forty Thieves, Sinbad the Sailor, and thrust us deep into realms where genies can bring our most impossible dreams to life! But, what do Aladdin, magic lamps, genies, thieves, and sailors all have to do with each other? They all come from one common source! An awe-inspiring collection of folk tales that have roots in Persia, India, Greece, Turkey, Central Asia, Middle East, Egypt. All of them.weaved into one epic book known as 1001 Nights, or Arabian Nights.
The story behind how Arabian Nights came to be is equally as interesting as the folk tales themselves! It begins with an ancient Arabian King by the name of Shahrayar. King Shahrayar was betrayed by his wife who had cheated on him with another man. King Shahrayar was driven mad by his wife’s actions and hatched a sinister plot to enact his revenge. He swore to marry every eligible bride in his kingdom and have them executed the morning after the wedding before they had the opportunity to betray him like his wife did…
Under the influence of his ferocious desire for revenge, King Shahrayar initiated his plan and began marrying and executing all the unwed women in his dominion. Before long, the king had taken the lives of so many brides that there were none left except the daughter of his grand vizier (an advisor to the king). Her name was Shahrazad, and on top of being beautiful, she was also quick-witted, creative, and a brilliant storyteller! With the announcement that she would be the next bride of King Shahrayar, and well aware of her fate, Shahrazad had very little time to devise a strategy to save her own life…
Shahrazad knew that escape was not an option, so she put all of her faith into distracting the king so that the wedding would be delayed for as long as possible. The only question was how to do it. The night before the wedding, Shahrazad took a leap of faith, and began telling the king a long, captivating story. The king became so mesmerized by the story that Shahrazad kept it going on and on until the sun peeked over the horizon and began to rise. King Shahrayar was so interested in how the story would end that he postponed the wedding for the following day!
At that moment, Shahrazad knew she had found her secret weapon. All she had to do was begin a new story every night and leave it unfinished until the following night, at which point she would begin a new story spanning into the next day and repeat the same process. Shahrazad, a masterful storyteller, was able to keep telling stories for one-thousand and one nights, and by that point, through the magical and therapeutic power of storytelling itself, the king was cured of his paranoia and anger. He abandoned his vengeful bloodlust, married Shahrazad, and they lived happily ever after, leaving behind a trail of one-thousand and one fantastic stories!
The power of storytelling managed to literally save Shahrazad’s life, and it has also saved the lives of countless others potential brides. But, listeners beware! The storytelling spell Shahrazad cast on king Shahrayar may have ended happily, but there are some who fall victim to the trance that a well-told story can conjure!
4.14
1.1
5.9
A woman dies, leaving her husband, a son, and three daughters. Calculate the fraction of her estate each will receive.
[Note: The conditions of Islamic law must be followed—that is, the husband must receive one-fourth share, and his son twice as much as a daughter.]
Greece. Archimedes and the Golden Crown
For almost a hundred years, the ancient Greek city of Syracuse had been at war with Carthage, an ancient city in Northern Africa. Finally the Syracusan troops, tired of the inefficiencies of their leaders, elected a commander from amongst themselves. It was a young general called Hiero.
After Hiero led the Syracusans to victory against their enemies, the people of Syracuse chose Hiero to be their king.
Hiero was grateful to the gods for his success and good fortune, and to show his gratitude, he decided to place in a certain temple, a golden crown in their honour. Hiero weighed out a precise amount of gold, and appointing a goldsmith, commanded him to fashion out of the gold a crown worthy of the gods.
The goldsmith did as he had been ordered, and on he appointed day, he delivered to the king an exquisitely wrought crown. The crown seemed to weight exactly as much as the gold that the king had given the goldsmith. Hiero was pleased, and paid the goldsmith handsomely. The goldsmith, receiving his payment, went away.
Hiero made preparations for the ceremony to place the crown in the temple. But a few days before the ceremony, he heard rumours that the goldsmith had cheated him, and given him a crown not of pure gold, but of gold that had silver mixed in it. The goldsmith, said the rumours, had replaced some of the gold that Hiero had given him, with an equal weight of silver.
Hiero was furious to learn that he might have been tricked. But he was a fair-minded man and wished to determine the truth before he punished the goldsmith.
If the goldsmith had indeed cheated him and mixed silver into the gold, then the goldsmith would have to be punished, and the crown could no longer be given as an offering to the gods. But if the goldsmith had been honest, then the crown remained what it had been intended to be, a sacred offering, and it would be placed in the temple as planned. So it was important that Hiero find out the truth quickly, before the day fixed for the ceremony, and without damaging the crown in any way.
Hiero believed there was only one man in Syracuse capable of discovering the truth and solving his problem. This was his cousin, Archimedes, a young man of 22, who was already renowned for his work in mathematics, mechanics and physics.
Deep in thought, pondering how best to solve the king’s problem, Archimedes walked to the public baths for his daily bath. Still thinking about the golden crown, he stepped into a tub of cool water for a dip. As he began to lower himself into the water, the water in the tub began to spill out over the sides. Curious, Archimedes continued to lower himself slowly into the water, and he noticed that the more his body sank into the water, the more water ran out over the sides of the tub. He realised that he had found the solution to Hiero’s problem. He was so excited by his discovery that he jumped out of the tub, and ran all the way home naked without remembering to put his clothes on, and shouting ‘Eureka, Eureka!’ – which in Greek means, ‘I have found it! I have found it!’
What Archimedes had found was a method for measuring the volume of an irregularly-shaped object. He realised that an object, when immersed in water, displaced a volume of water equal to its own volume, and that by measuring the volume of the displaced water, the volume of the object could be determined, regardless of the object’s shape. So, he could measure the volume of the crown by measuring the volume of the water spilled from a container filled with water to the brim when the crown was fully dipped in it.
How then, would this realisation help him to answer Hiero’s question – had the goldsmith mixed silver in the golden crown or not? Let us see how Archimedes used his discovery to solve the king’s problem.
Archimedes knew that gold was denser than silver – so a piece of gold weighing a certain amount would be smaller than a piece of silver weighing the same:Thus, if the goldsmith had stolen some of the gold the king had given him, and replaced it with an equal weight of silver in the crown, then the total volume of the gold and silver crown would be greater than the volume of the original amount of gold.
So now, all that remained for Archimedes to do was to compare the volume of the crown to the volume of the amount of gold that Hiero had given the goldsmith. He filled the bowl with water to the brim. He lowered the crown into the water. He knew that if the crown was pure gold, its volume would be the same as that of the lump of gold (which he had made sure weighed the same as the crown), regardless of shape, and that it would displace the same amount of water as the gold. But, if the goldsmith had replaced some of the gold with silver, then the volume of the gold and silver crown would be greater than the volume of the gold, and so the crown would displace more water than the gold.
Archimedes found that the crown did, in fact displace more water than the lump of gold of equal weight. Thus he came to the conclusion that the crown was not pure gold, and that the goldsmith had indeed mixed some silver (or other, lighter metal) into the gold and cheated the king. That is how Archimedes is said to have helped King Hiero detect the goldsmith’s fraud.
4.15
1.1
5.7
If someone says a workman receives a pay of 10 dirhams per month, how much must he be paid for six days?
1 mina = 100 drachms, the Greek silver coins
4.16
1.1
5.8
The father perished in the shoals of the Syrtis, and this, the eldest of the brothers, came back from that voyage with five talents. To me he gave twice two thirds of his share, on our mother he bestowed two eighths of my share, nor did he sin against divine justice.
1 talent = 6,000 drachmas, the Greek silver coins.
4.17
1.1
5.9
“Best of clocks, how much of the day is past?” There remain twice two thirds of what is gone.
4.18
1.1
5.10
We three Loves stand here pouring out water for the bath, sending streams into the fair flowing tank. I on the right, from my long-winged feet, fill it full in the sixth part of a day; I on the left, from my jar, fill it in four hours; and I in the middle, from my bow, in just half a day. Tell me in what a short time we should fill it, pouring water from wings, bow, and jar all at once.
4.19
1.1
5.8
I am a brazen lion; my spouts are my two eyes, my mouth, and the flat of my right foot. My right eye fills a jar in two days, my left eye in three, and my foot in four. My mouth is capable of filling it in six hours; tell me how long all four together will take to fill it.
4.20
1.1
5.9
Brick makers, I am in a great hurry to erect this house. Today is cloudless, and I do not require many more bricks, but I have all I want but three hundred. Thou alone in one day couldst make as many, but thy son left off working when he had finished two hundred, and thy son in law when he had made two hundred and fifty. Working all together, in how many hours can you make these?
4.21
1.1
5.10
After praying for a just increase in my fortunes of gold, I have nothing. I gave 40 talents of gold under evil auspices to my friends in vain, and I see my enemies in possession of a half, a third, and an eighth of my fortune. How many talents did this man once have?
4.22
1.1
5.9
The inscription on the tomb reads: “This tomb holds Diophantus. Ah, how great a marvel!” The inscription then tells the length of his life as follows: God granted him to be a boy one-sixth of his life, and adding one- twelfth part of this, he clothed his cheeks with down. He lit the light of wedlock after one-seventh part of his life, and after 5 years in his marriage he granted him a son. Alas, late-born child; after reaching one-half the measure of his father’s life, cruel fate took him. After consoling his grief by the science of mathematics for 4 years, Diophantus ended his life. How old was Diophantus when he passed away?
