2.2 – Valence Bond Theory
Chapter 2.1.1. introduced the concept of covalent bonding, which involves the sharing of electrons between two atoms. The electronegativity of these atoms determines where the electron density is more closely held and the polarity of the bond. While the exact location of an electron cannot be known, the probability of finding an electron in a particular area can be estimated. This area is called an orbital.
Electrons reside in orbitals according to the quantum mechanical model. According to valence bond theory, a chemical bond is formed when two half-filled orbitals overlap to share two electrons.
Hybridization
Hybridization is a concept that was developed to explain the experimentally observed electron geometries around atoms in bonds that could not be explained by atomic orbitals alone.
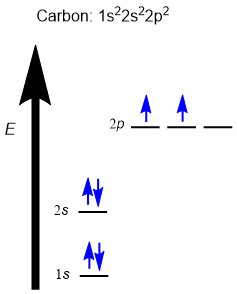
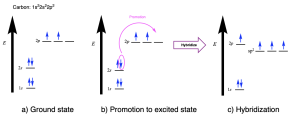
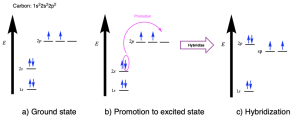
Hybridization explains the bonding in a wide range of molecules, including simple molecules like methane (CH4). The electronic ground state of carbon (1s22s22p2) has two unpaired valence electrons in p orbitals (Figure 2.2.a). This would suggest that:
- Carbon should only make two bonds through orbital overlap, since it only has two unpaired electrons in two half-filled shells; and
- The H–C–H bond angle in methane should be 90º, since the p orbitals are oriented 90º from one another.
However, experimental structure determination of methane shows that:
- There are four hydrogens around the central carbon atom with equal C–H bond lengths, which suggests the same C–H bond energy for all four bonds.
- There is a tetrahedral geometry with 109.5º bond angles.
Taken together, these experimental results imply that electrons must be occupying orbitals of equal energy, called degenerate orbitals, to form bonds with equal bond angles and bond energies.
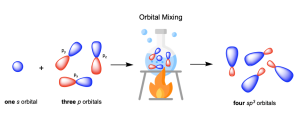
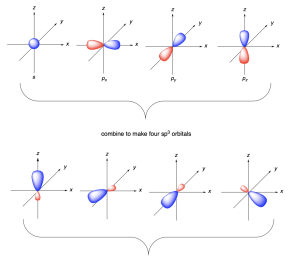
The bonding in methane can instead be described by mixing the valence orbitals (one 2s and three 2p) to form four degenerate sp3 hybrid orbitals (Figure 2.2.b). Being degenerate, Hund’s Rule states that each orbital will first be filled with a single electron before electrons are paired together. Thus, these orbitals are half-filled, with one electron in each. These four degenerate sp3 hybridized orbitals are oriented in 3-dimensaional space to minimize electrostatic repulsion of the occupying electrons, resulting in the tetrahedral geometry. Thus, carbon makes four bonds using four half-filled hybrid orbitals, all of equal bond energy and bond length.
The word hybrid means something of mixed origin or composition. With respect to atomic systems, hybridization is the mixing of two or more standard atomic orbitals (such as s, p, d, f orbitals) to form new hybrid orbitals (such as sp3 orbitals). Hybridization explains how the energy levels and orientations of ground state atomic orbitals (s, p, d, f) could be adjusted in 3 dimensional space to match the experimental observations found in organic molecules.
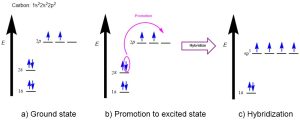
Promotion of an electron from the ground state to facilitate the formation of hybrid orbitals requires energy, making it an endothermic process. However, bond formation releases energy, making it an exothermic process. The hybridized carbon atom can now form four covalent bonds with its four unpaired electrons (instead of two in the unhybridized state), resulting in a net release of energy upon bond formation.
There are two key rules in hybridization:
- The number of standard atomic orbitals mixed together is equal to the number of hybrid orbitals formed. In other words, the total number of orbitals is conserved upon hybridization. For example, mixing one 2s and three 2p orbitals generates four sp3 hybrid orbitals.
- The combination of standard atomic orbitals mixed together determines the shapes of the hybrid orbitals formed. For example, mixing one s and three p orbitals of the same energy level generates four sp3 hybrid orbitals that point towards the corners of a tetrahedron, (Figure 2.2.c).
Sigma and Pi Bonding
Two types of bonds formed by orbital overlap will be considered: sigma (σ) and pi (π) bonds.
σ bonds involve orbitals overlapping end-on, as illustrated in Figure 2.2.d. Exactly one σ bond is possible between two atoms. All single bonds are made through σ bonding.

In contrast, π bonds consist of side-on orbital overlap. π bonds are seen in molecules with double bonds or triple bonds. Double bonds consist of one σ and one π bond, while triple bonds consist of one σ bond and two π bonds. Up to two π bonds in total are possible between two atoms.

VSEPR vs. Hybrid Orbitals
The Valence Shell Electron Pair Repulsion (VSEPR) model and the hybridization model predict the same molecular geometry (Table 2.2.a). This is because the orientation of the hybrid orbitals produces certain bond angles to minimize repulsion, yielding a specific geometry.
Table 2.2.a. Molecular geometries of molecules predicted based on the number of electron groups. The same result is obtained through the VSEPR model and the hybridization model.
| # of electron groups | Electron–group geometry from VSEPR | Hybridization | Ideal Bond Angle | Examples |
| 2 | Linear | sp | 180˚ | CO2, BeCl2 |
| 3 | Trigonal planar | sp2 | 120˚ | BH3, SO2 |
| 4 | Tetrahedral | sp3 | 109.5˚ | CH4, H2O |
NOTE: The hybridization schemes shown in this chart work well for organic compounds, but do not apply to all molecules.
Are You Wondering? A Review of VSEPR Theory
Valence Shell Electron Pair Repulsion (VSEPR) Theory is a model to explain the molecular shapes of molecules based on the idea that electron groups repel one another. An electron group can be a bond (single, double, triple) or a lone pair. A molecule is symbolized a AXnEm, where A is the central atom, X represents atoms that are bonded to the central atom, and E represents lone pairs of electrons. There can be any number of bonded atoms (n) or lone pairs (m). The chart below summarizes some of the important VSEPR classes for 2, 3, or 4 groups of electrons. Other VSEPR classes exist for greater numbers of electron groups, but they are largely unnecessary for organic compounds.
| 2 e– groups | 3 e– groups | 3 e– groups | 4 e– groups | 4 e– groups | 4 e– groups | |
| VSEPR Cass | AX2 | AX3 | AX2E | AX4 | AX3E | AX2E2 |
| Molecular Geometry | Linear | Trigonal planar | Bent | Tetrahedral | Trigonal pyramidal | Bent |
| Angles | 180° | 120° | < 120° | 109.5° | < 109.5° | < 109.5° |
| Examples | BeCl2, CO2 | BF3, CH3+ | BH2– | CH4 | NH3, CH3– | H2O |
sp3 Hybridization
As previously mentioned with methane, sp3 hybridization involves four atomic orbitals (one 2s and three 2p) undergoing hybridization to form four sp3 hybrid orbitals. Since these hybrid orbitals are degenerate in energy, the bond length and bond energy of all four bonds are equal. The sp3 orbitals resemble an asymmetric dumbbell shape, with most electron density residing in the larger lobe.
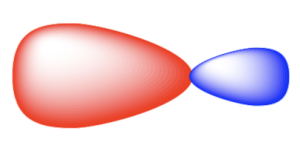
In sp3 hybridization, the large lobes point to the corners of a tetrahedron. The angle between orbitals is 109.5º. The hybrid orbitals overlap with orbitals on other atoms to form σ bonds.
Below, the hybridization and bonding in four molecules are discussed: methane (CH4), methide anion (CH3–), ammonia (NH3), and ethane (C2H6).
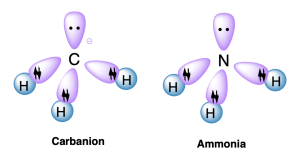
The sp3 hybrid orbitals in carbon overlap with atomic s orbitals on hydrogen to produce C–H σ bonds, as seen in methane (Figure 2.2.h.) and methide anion (Figure 2.2.i, left). For methane, all four sp3 hybrid orbitals form σ bonds to hydrogen; for methide anion, three sp3 hybrid orbitals form σ bonds to hydrogen, with the fourth sp3 hybrid orbital containing a lone pair of electrons. Ammonia is isoelectronic to methide anion, which means that they have the same bonding arrangement (Figure 2.2.i, right): three sp3 hybrid orbitals to form σ bonds to hydrogen, with the fourth sp3 hybrid orbital containing a lone pair of electrons. This example showcases the fact that hybridization can be invoked not just for carbon but for heteroatoms such as nitrogen as well.
The geometry around the central atom is tetrahedral, with ideal bond angles of 109.5°. For methide anion and ammonia, the H–C–H or H–N–H bond angle will be slightly less than 109.5° due to the electrostatic repulsion by the lone pair of electrons.

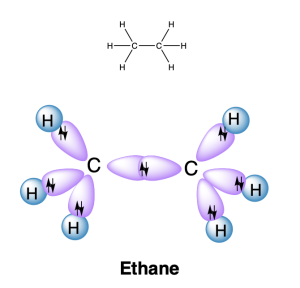
End-on overlap of hybrid orbitals on two atoms can also produce a σ bond. For example, in ethane (C2H6), both carbon atoms are sp3 hybridized, and overlap of these orbitals produces a C–C σ bond (Figure 2.2.j.). There are also six C–H σ bonds, formed from overlap of an sp3 hybrid orbital on carbon with an atomic s orbital on hydrogen. The geometry around the central carbon atom is tetrahedral, with ideal bond angles of 109.5°.

sp2 Hybridization
sp2 hybridization (Figures 2.2.l-n) involves the mixing of three atomic orbitals (one 2s and two 2p orbitals) to form three sp2 hybrid orbitals. One atomic p orbital remains unhybridized.
The three sp2 hybrid orbitals each contain an unpaired electron and can overlap with other orbitals to form three σ bonds. The three hybrid orbitals point to the corners of an equilateral triangle, resulting in a trigonal planar geometry, with bond angles of 120°. The unhybridized p orbital lies in an orthogonal (perpendicular) plane.
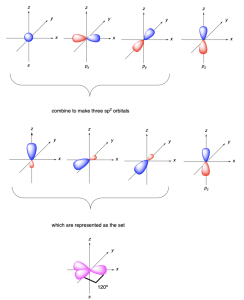

Below, the hybridization and bonding in four molecules will be discussed: borane (BH3), methyl cation (CH3+), and ethene (C2H4).
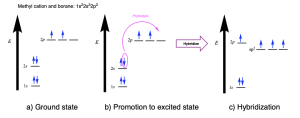
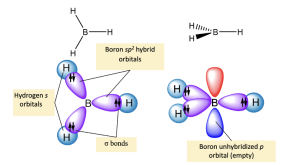
Borane and methyl cation are isoelectronic, with three valence electrons on the central atom (boron or carbon). The orbital energy diagram is shown below (Figure 2.2.o.). Three atomic orbitals (one 2s and only two of the 2p orbitals) undergo hybridization to form three sp2 hybrid orbitals. One 2p orbital remains unhybridized. Because there are only three valence electrons in this case, the unhybridized p orbital is empty.
For borane, the sp2 hybrid orbitals on boron overlap with an atomic 1s orbital on each hydrogen to produce B–H σ bonds (Figure 2.2.p.). The remaining unhybridized p orbital is not involved in bonding. The positively charged methyl cation is isoelectronic with borane, and therefore has the same bonding arrangement (Figure 2.2.q.): three C–H σ bonds are formed from the overlap of the sp2 hybrid orbitals on carbon with an atomic 1s orbital on each hydrogen, while the unhybridized p orbital does not participate in bonding.
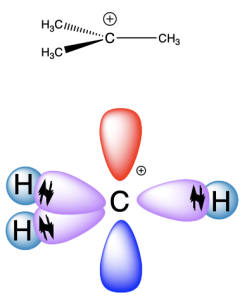
The empty, unhybridized p orbital in borane or methyl cation is not involved in bonding. However, a half-filled p orbital (Figure 2.2.r.) can also overlap side-on with another half-filled p orbital to form a π-bond. Functional groups with a double bond have sp2 hybrid orbitals, along with an unhybridized p orbital.
An example of a molecule with a double bond is ethene (CH2=CH2) (Figure 2.2.r.). The C=C double bond is formed by: (1) end-on overlap of the two carbon atoms’ sp2 orbitals, creating a σ bond, and (2) side-on overlap of the two carbon atoms’ half-filled unhybridized p orbital, creating a π bond. Therefore, a double bond is formed from a σ bond and a π bond.
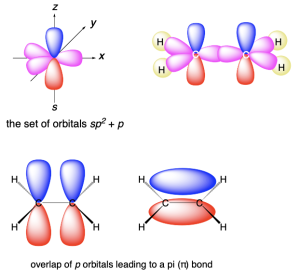
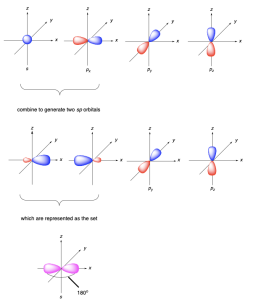
sp Hybridization
sp hybridization (Figure 2.2.s) involves mixing two atomic orbitals (one 2s and one 2p) to form two sp2 hybrid orbitals. Two atomic p orbitals remain unhybridized.
The two sp hybrid orbitals each contain an unpaired electron and can make two σ bonds. The two hybrid orbitals point along a straight line, resulting in a linear geometry, with a bond angle of 180°. The unhybridized p orbitals lie in orthogonal (perpendicular) planes (Figure 2.2.t.).
The half-filled unhybridized p orbitals can overlap side-on with another half-filled p orbital to form a π-bond. Functional groups with a triple bond have sp hybrid orbitals (to form a σ bond), along with two unhybridized p orbitals (to form two π bonds).
An example of a molecule with a triple bond is ethyne (HC≡CH) (Figure 2.2.u.). The C≡C triple bond is formed by: (1) end-on overlap of the two carbon atoms’ sp orbitals, creating a σ bond, and (2) side-on overlap of the two carbon atoms’ half-filled unhybridized p orbitals, creating two π bonds. The two π bonds are oriented 90º to one another, matching the angle between the p orbitals. Therefore, a triple bond is formed from a σ bond and two π bonds.

The following videos below include examples from previous CHEM 1AA3 tests or exams that students struggled with. Try solving the practice questions on your own before looking at the solution.
(The full solution to this problem can be found in Chapter 5.1).
(The full solution to this problem can be found in Chapter 5.1).
Key Takeaways
- According to quantum mechanics, the exact location of an electron cannot be known, thus electrons reside in general areas called orbitals. Orbitals are a probable estimate of where the electrons will be.
- Hybridization in bonding is a better way in which experimentally observed electron geometries around two atoms in a bond can be explained.
- Hybridization involves the existence of hybrids of s and p orbitals.
- sp3 hybridization involves one s orbital and three p orbitals hybridizing to form four new hybrid sp3 orbitals. This allows for four bonds.
- Example: methane
- Geometry: tetrahedral
- sp2 hybridization involves one s orbital and two p orbitals hybridizing to form two new hybrid sp2 orbitals, with one leftover p orbital. This allows for three bonds.
- Example: Ethene
- Geometry: trigonal planar
- sp hybridization involves one s and one p orbital to hybridize, forming two new sp orbitals, and leaving two unhybridized p orbitals.
- Example: ethyne
- Geometry: planar
- In bonding, orbitals of two atoms form bonds from overlapping orbitals.
- When two orbitals overlap directly head on, for example two neighbouring sp3 orbitals, it is called a sigma (σ) bond.
- When two orbitals overlap from side-to-side contact, for example two neighbouring p orbitals, they form a pi (π) bond.
- Double and triple bonds with carbon utilize sp2 and sp hybridized carbons respectively.
- Double bonds involve one σ bond and one π bond between two sp2 hybridized carbons, as there is one available p orbital which can overlap in an sp2 hybridized carbon.
- Triple bonds involve one σ bond and two π bonds between two sp hybridized carbons, as there are two available p orbitals which can overlap in an sp hybridized carbon.
Key terms in this chapter:
| Key Term | Definition |
| Hybridization | The mixing of two or more standard atomic orbitals (such as s, p, d, f orbitals) to form new hybrid orbitals (such as sp3 orbitals) suitable for bonding. |
Diversity in Chemistry: From Fukui to Krylov
Valence bond theory is introduced in this chapter, yet there is another notable theory that is used to explain chemical binding. This theory is called the Molecular Orbital Theory, and it was developed years after the original valence bond theory was introduced. Kenichi Fukui was the first person of East Asian descent to be awarded the Nobel Prize in Chemistry, winning one-half of the award in 1981 for his work involved with molecular orbital theory. His research specifically focused on the discovery of two molecular orbitals involved in the bonding process: the highest occupied molecular orbital, the HOMO, and the lowest unoccupied molecular orbital, the LUMO. These two molecular orbitals are the entire basis of how organic reactions occur. In short, he discovered that it was possible to approximate reactivity by looking at these two orbitals, as the HOMO and LUMO orbitals interact with each other resulting in attraction.
When it was first published, his work was deemed a “sleeping beauty — it generally fell under the radar due to Fukui’s highly mathematical approach and because his works were published in a more Japanese-oriented journal. However, after being cited by another famous computational chemist working on a similar topic, Roald Hoffman, who was awarded the second half of the same Nobel Prize, Fukui gained worldwide recognition. More information on Fukui can be found on his profile at the Michigan State University.

In the present day, the study of chemical structure and reactions has gotten much more advanced, employing new technologies to understand these interactions. Computational chemistry is a branch of chemistry that uses computer programs that use theoretical chemistry to calculate molecular structure & properties, as well as model organic reactions. Anna Krylov is a current professor at the University of Southern California whose main work focuses on modeling open shell (unfilled valence shells) and electronically excited species. She invented the spin-flip approach, which expanded the breadth of a popular quantum chemistry theory to have more applications to biradicals and bond-breaking events. She also uses computational chemistry to investigate the role of radicals and excited state species in events such as combustion, astrochemistry, solar energy and many more diverse fields. Krylov is also very active in the field of science education and outreach, developing labs and tutorials to promote quantum chemistry literacy among chemists. She is also passionate about promoting gender equality in the theoretical chemistry field and is an advocate of freedom of speech and academic freedom.
The mixing of two or more standard atomic orbitals (such as s, p, d, f orbitals) to form new hybrid orbitals (such as sp3 orbitals) suitable for bonding.

