"

20 Nonlinear Inequations

\bullet An inequality for which the side can be written as a product or quotient of linear factors or quadratic factors (that cannot be factored) can be solved through a sign diagram.

\bullet How to solve an inequality ?

– determine the points where each factor is 0

– determine the sign of each factor in each interval

– use laws of multiplication or division to determine the sign of the entire quantity.

\bullet The set of solution of \quad (x-\alpha)(x-\beta)(x-\gamma) \leqslant 0\quad is \quad (-\infty, \alpha]\cup [\beta, \gamma], \quad (\alpha < \beta < \gamma) , since we have

\begin{matrix} x & -\infty\hskip 5.5cm \alpha \hskip 3.5cm \beta \hskip 3cm \gamma \hskip 3cm +\infty \\ \hline x-\alpha &\hskip 2cm - \hskip 3cm + \hskip 4cm + \hskip 3cm +\\ \hline x-\beta & \hskip 2cm -\hskip 3cm-\hskip 4cm + \hskip 3cm + \\ \hline x-\gamma & \hskip 2cm - \hskip 3cm -\hskip 4cm - \hskip 3cm +\\ \hline (x-\alpha)(x-\beta)(x-\gamma) & \hskip 2cm - \hskip 3cm +\hskip 4cm - \hskip 3cm + \\ \hline \end{matrix}

 

Watch a Video


View on YouTube

 

Show Examples

 

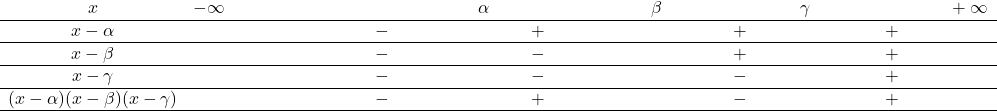
Exercise 1

 

Show/Hide Solution.

The set of possible solutions is : \displaystyle{ (-\infty , -\frac{{1}}{{9}} )\cup (\frac{{9}}{{7}}, +\infty ) }.

 

Exercise 2

 

Show/Hide Solution.

The set of all possible solutions is : \displaystyle{ (-\infty , -3 ]\cup [2,4]}.

 

Exercise 3

 

Show/Hide Solution.

Note that \quad \displaystyle{ \frac{ ( 2x - 1 )^{1/3} ( - 4 x + 1 )^2 }{ ( x + 5)^3 (x^2 + 7 ) } = \frac{ ( 2x - 1 )^{1/3} }{ ( x + 5)^3 } \, . \, \frac{ ( - 4 x + 1 )^2 }{ (x^2 + 7 ) } } .

We have

\qquad \displaystyle{ \frac{ ( - 4 x + 1 )^2 }{ (x^2 + 7 ) } \geq 0 \qquad \forall x \quad \hbox{ and } \quad \frac{ ( - 4 x + 1 )^2 }{ (x^2 + 7 ) } = 0 \quad \hbox{ for } \quad x=1/4.}

Now, we have

\displaystyle{ \frac{ ( 2x - 1 )^{1/3} }{ ( x + 5)^3 } \, \geq 0 \quad \Longleftrightarrow \quad \frac{ 2x - 1 }{ x + 5 } \geq 0 \quad \Longleftrightarrow \quad x \in (-\infty, -5) \cup [1/2, +\infty) }.

Thus, the set of solutions is : (-\infty, -5) \cup \{ 1/4\}\cup [1/2, +\infty).

License

Icon for the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License

Guide to Precalculus Review Copyright © 2025 by Samia CHALLAL is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License, except where otherwise noted.

Share This Book