"

7.3 Confidence Intervals for a Single Population Mean with Unknown Population Standard Deviation

LEARNING OBJECTIVES

  • Calculate and interpret confidence intervals for estimating a population mean where the population standard deviation is unknown.

In practice, we rarely know the population standard deviation. In the past, when the sample size was large, this did not present a problem to statisticians. They used the sample standard deviation [latex]s[/latex] as an estimate for [latex]\sigma[/latex], and proceeded as before to calculate a confidence interval with close enough results. However, statisticians ran into problems when the sample size was small because a small sample size created inaccuracies in the confidence interval.

William S. Goset (1876–1937) of the Guinness Brewery in Dublin, Ireland, ran into this problem. His experiments with hops and barley produced very few samples. Just replacing the population standard deviation [latex]\sigma[/latex] with the sample standard deviation [latex]s[/latex] did not produce accurate results when he tried to calculate a confidence interval. Goset realized that he could not use a normal distribution for the calculation. He found that the actual distribution depends on the sample size. This problem led him to “discover” what is called the Student’s [latex]t[/latex]-distribution. The name comes from the fact that Gosset wrote under the pen name “Student.”

Up until the mid-1970s, some statisticians used the normal distribution approximation for large sample sizes and only used the [latex]t[/latex]-distribution for sample sizes of at most [latex]30[/latex]. With technology, the practice now is to use the [latex]t[/latex]-distribution whenever [latex]s[/latex] is used as an estimate for [latex]\sigma[/latex].

When a simple random sample of size [latex]n[/latex] is taken from a population that has an approximately normal distribution with mean [latex]\mu[/latex], an unknown population standard deviation, and the sample standard deviation [latex]s[/latex] is used as an estimate for the population standard deviation, the distribution of the sample means follows a [latex]t[/latex]-distribution with [latex]n-1[/latex] degrees of freedom. For each sample size [latex]n[/latex], there is a different [latex]t[/latex]-distribution. The [latex]t[/latex]-score is

[latex]\displaystyle{t=\frac{\overline{x}-\mu}{\frac{s}{\sqrt{n}}}}[/latex]

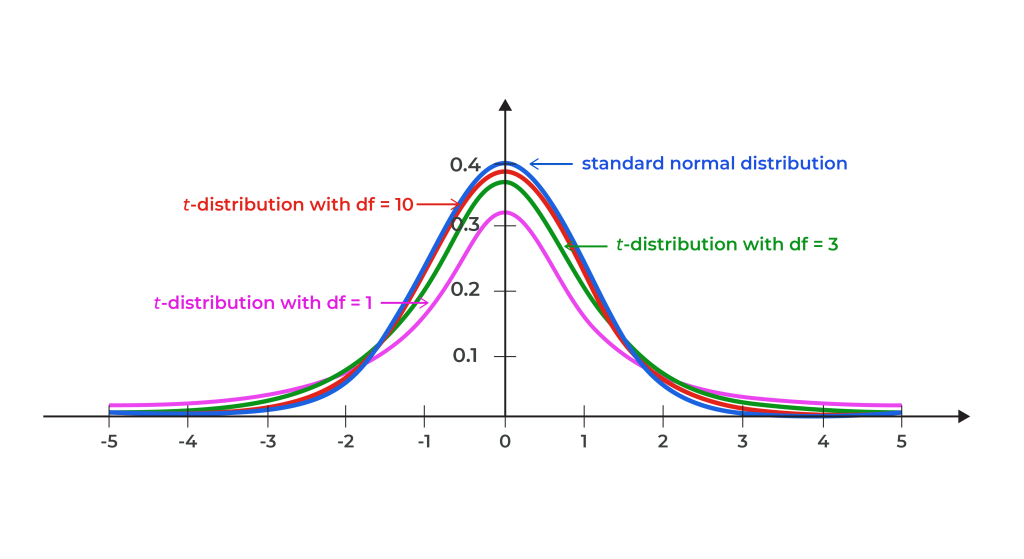
Every [latex]t[/latex]-distribution has a parameter called the degrees of freedom (df). In this case, where the [latex]t[/latex]-distribution is used for the distribution of the sample means, the value of the degrees of freedom is [latex]n-1[/latex] where [latex]n[/latex] is the sample size. Here the value of [latex]n-1[/latex] used as the degrees of freedom comes from the calculation of the sample standard deviation [latex]s[/latex]. Because the sum of the deviations is zero, we can find the last deviation once we know the other [latex]n–1[/latex] deviations. The other [latex]n–1[/latex] deviations can change or vary freely. Note that the value or formula of the degrees of freedom for the [latex]t[/latex]-distribution will vary depending on the situation in which the [latex]t[/latex]-distribution is used.

Properties of the [latex]t[/latex]-Distribution

  • The mean for the [latex]t[/latex]-distribution is [latex]0[/latex].
  • The graph for the [latex]t[/latex]-distribution is a symmetric, bell-shaped curve, similar to the standard normal curve. The graph is symmetric about the mean [latex]0[/latex].
  • The [latex]t[/latex]-distribution has more probability in its tails than the standard normal distribution because the spread of the [latex]t[/latex]-distribution is greater than the spread of the standard normal distribution. So, the graph of the [latex]t[/latex]-distribution will be thicker in the tails and shorter in the centre than the graph of the standard normal distribution.
  • The exact shape of the [latex]t[/latex]-distribution depends on the degrees of freedom. As the degrees of freedom increases, the graph of [latex]t[/latex]-distribution becomes more like the graph of the standard normal distribution. In fact, the [latex]t[/latex]-distribution with an infinite number of degrees of freedom is the standard normal distribution.
  • The underlying population of individual observations is assumed to be normally distributed with an unknown population mean [latex]\mu[/latex] and unknown population standard deviation [latex]\sigma[/latex]. The size of the underlying population is generally not relevant unless it is very small. If it is bell-shaped (normal), then the assumption is met and does not need discussion. Random sampling is assumed, but that is a completely separate assumption from normality.

Graphs of t distribution curves.

Constructing the Confidence Interval

When finding a confidence interval for an unknown population mean when the population standard deviation is unknown, we use the sample standard deviation [latex]s[/latex] as an estimate for the (unknown) population standard deviation, and we use a [latex]t[/latex]-distribution with [latex]n-1[/latex] degrees of freedom to find the required [latex]t[/latex]-score for the confidence interval. In this case, we replace the [latex]z[/latex]-score with a [latex]t[/latex]-score and [latex]\sigma[/latex] with [latex]s[/latex] in the formulas for the limits of the confidence interval for a population mean.

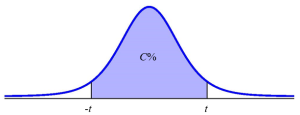
To construct the confidence interval, take a random sample of size [latex]n[/latex] from the population. Calculate the sample mean [latex]\overline{x}[/latex] and the sample standard deviation [latex]s[/latex]. The limits for the confidence interval with confidence level [latex]C[/latex] for an unknown population mean [latex]\mu[/latex] when the population standard deviation [latex]\sigma[/latex] is unknown are

[latex]\begin{eqnarray*}\\\text{Lower Limit}&=&\overline{x}-t\times\frac{s}{\sqrt{n}}\\\\\text{Upper Limit}&=&\overline{x}+t\times\frac{s}{\sqrt{n}}\\\\\end{eqnarray*}[/latex]

where [latex]t[/latex] is the (positive) [latex]t[/latex]-score of the [latex]t[/latex]-distribution with [latex]n-1[/latex] degrees of freedom so the area under the [latex]t[/latex]-distribution in between [latex]-t[/latex] and [latex]t[/latex] is the confidence level [latex]C[/latex].

Graph of how to construct a confidence interval with confidence level C using a t-distribution. Along the horizontal axis the points -t and t are labeled. There is a vertical line from -t to the t-distribution curve. There is a vertical line from t to the t-distribution curve. The area under the curve between -t and t is shaded and labeled C%.

CALCULATING THE [latex]\color{white}{t}[/latex]-SCORE FOR A CONFIDENCE INTERVAL IN EXCEL

To find the [latex]t[/latex]-score to construct a confidence interval with confidence level [latex]C[/latex], use the t.inv.2t(area in the tails, degrees of freedom) function.

  • For area in the tails, enter the sum of the area in the tails of the [latex]t[/latex]-distribution. For a confidence interval, the area in the tails is [latex]1-C[/latex].
  • For degrees of freedom, enter the value of the degrees of freedom for the [latex]t[/latex]-distribution. For a confidence interval for a population mean, the degrees of freedom is [latex]n-1[/latex].

The output from the t.inv.2t function is the value of the [latex]t[/latex]-score needed to construct the confidence interval.

Visit the Microsoft page for more information about the t.inv.2t function.

NOTE

The t.inv.2t function requires that we enter the sum of the area in both tails. The area in the middle of the distribution is the confidence level [latex]C[/latex], so the sum of the area in both tails is the leftover area [latex]1-C[/latex].

Video: “Confidence Interval for a population mean – t distribution” by Joshua Emmanuel [7:40] is licensed under the Standard YouTube License.Transcript and closed captions available on YouTube.

EXAMPLE

Suppose you do a study of acupuncture to determine how effective it is in relieving pain. You measure sensory rates for [latex]15[/latex] subjects with the results given below.

Sensory Rate Results
8.6 7.3 10.3
9.4 9.2 5.4
7.9 9.6 8.1
6.8 8.7 5.5
8.3 11.4 6.9
  1. Construct a [latex]95\%[/latex] confidence interval for the mean sensory rate.
  2. Interpret the confidence interval found in part 1.
  3. Is it reasonable to conclude that the mean sensory rate is [latex]10[/latex]? Explain.

Solution

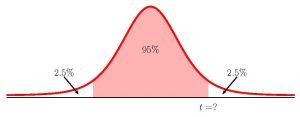
  1. To find the confidence interval, we need to find the [latex]t[/latex]-score for the [latex]95\%[/latex] confidence interval. This means that we need to find the [latex]t[/latex]-score so that the area in the tails is [latex]1-0.95=0.05[/latex]. The degrees of freedom for the [latex]t[/latex]-distribution is [latex]n-1=15-1=14[/latex].

    Graph of a t-distribution curve. Along the horizontal axis the point t is labeled. There is a vertical line from t to the normal distribution curve. The area under the curve in the middle of the distribution is labeled 95%. The area in the left tail is labeled 2.5%. The area in the right tail is labeled 2.5%.

    Function t.inv.2t
    Field 1 0.05
    Field 2 14
    Answer 2.1447…

    So [latex]t=2.1447....[/latex]. From the sample data supplied in the question [latex]\overline{x}=8.226...[/latex], [latex]s=1.672...[/latex] and [latex]n=15[/latex].  The [latex]95\%[/latex] confidence interval is

    [latex]\begin{eqnarray*}\\\text{Lower Limit}&=&\overline{x}-t\times\frac{s}{\sqrt{n}}\\&=&8.226...-2.1447...\times\frac{1.672...}{\sqrt{15}}\\&=&7.30\\\\\text{Upper Limit}&=&\overline{x}+t\times\frac{s}{\sqrt{n}}\\&=&8.226...+2.1447...\times\frac{1.672...}{\sqrt{15}}\\&=&9.15\\\\\end{eqnarray*}[/latex]

  2. We are [latex]95\%[/latex] confident that the mean sensory rate is between [latex]7.30[/latex] and [latex]9.15[/latex].
  3. It is not reasonable to conclude that the mean sensory rate is [latex]10[/latex] because [latex]10[/latex] is outside of the confidence interval.

NOTE

When calculating the limits for the confidence interval, keep all of the decimals in the [latex]t[/latex]-score and other values such as [latex]\overline{x}[/latex] and [latex]s[/latex] throughout the calculation. This will ensure that there is no round-off error in the answers. Use Excel to do the calculation of the limits, clicking on the cells containing the [latex]t[/latex]-score, [latex]\overline{x}[/latex] and [latex]s[/latex], to ensure that all of the decimal places are used in the calculation.

TRY IT

You do a study of hypnotherapy to determine how effective it is in increasing the number of hours of sleep subjects get each night. You measure hours of sleep for [latex]12[/latex] subjects with the following results.

Hours of Sleep
8.2 8.6 8.9 9.2
9.1 6.9 9.9 7.5
7.7 11.2 10.1 10.5
  1. Construct a [latex]97\%[/latex] confidence interval for the mean number of hours slept each night.
  2. Interpret the confidence interval found in part 1.
  3. Is it reasonable to assume that the mean number of hours slept each night is [latex]9[/latex] hours? Explain.

 

Click to see Solution
  1. Function t.inv.2t
    Field 1 0.03
    Field 2 11
    Answer 2.4906…

    [latex]\begin{eqnarray*}\\\text{Lower Limit}&=&\overline{x}-t\times\frac{s}{\sqrt{n}}\\&=&8.9833...-2.4906...\times\frac{1.2904...}{\sqrt{12}}\\&=&8.056\\\\\text{Upper Limit}&=&\overline{x}+t\times\frac{s}{\sqrt{n}}\\&=&8.9833...+2.4906...\times\frac{1.2904...}{\sqrt{12}}\\&=&9.911\\\\\end{eqnarray*}[/latex]

  2. We are [latex]97\%[/latex] confident that the mean number of hours slept each night is between [latex]8.056[/latex] hours and [latex]9.911[/latex] hours.
  3. It is reasonable to assume the mean number of hours slept each night is [latex]9[/latex] hours because [latex]9[/latex] is inside the confidence interval.

EXAMPLE

The Human Toxome Project (HTP) is working to understand the scope of industrial pollution in the human body. Industrial chemicals may enter the body through pollution or as ingredients in consumer products. In October 2008, the scientists at HTP tested cord blood samples for [latex]20[/latex] newborn infants in the United States. The cord blood of the “In utero/newborn” group was tested for [latex]430[/latex] industrial compounds, pollutants, and other chemicals, including chemicals linked to brain and nervous system toxicity, immune system toxicity, reproductive toxicity, and fertility problems. There are health concerns about the effects of some chemicals on the brain and nervous system. This table shows how many of the targeted chemicals were found in each infant’s cord blood.

Targeted Chemicals
79 145 147 160 116 100 159 151 156 126
137 83 156 94 121 144 123 114 139 99
  1. Construct a [latex]90\%[/latex] confidence interval for the mean number of targeted industrial chemicals found in an infant’s blood.
  2. Interpret the confidence interval found in part 1.

Solution

  1. To find the confidence interval, we need to find the [latex]t[/latex]-score for the [latex]90\%[/latex] confidence interval. This means that we need to find the [latex]t[/latex]-score so that the area in the tails is [latex]1-0.90=0.1[/latex]. The degrees of freedom for the [latex]t[/latex]-distribution is [latex]n-1=20-1=19[/latex]
    Function t.inv.2t
    Field 1 0.1
    Field 2 19
    Answer 1.7291…

    So [latex]t=1.7291....[/latex]. From the sample data supplied in the question [latex]\overline{x}=127.45[/latex], [latex]s=25.9645...[/latex] and [latex]n=20[/latex].  The [latex]90\%[/latex] confidence interval is

    [latex]\begin{eqnarray*}\\\text{Lower Limit}&=&\overline{x}-t\times\frac{s}{\sqrt{n}}\\&=&127.45-1.7291...\times\frac{25.9645...}{\sqrt{20}}\\&=&117.41\\\\\text{Upper Limit}&=&\overline{x}+t\times\frac{s}{\sqrt{n}}\\&=&127.45+1.7291...\times\frac{25.9645...}{\sqrt{20}}\\&=&137.49\\\\\end{eqnarray*}[/latex]

  2. We are [latex]90\%[/latex] confident that the mean number of targeted industrial chemicals found in an infant’s blood is between [latex]117.41[/latex] and [latex]137.49[/latex].

TRY IT

A random sample of statistics students were asked to estimate the total number of hours they spend watching television in an average week. The responses are recorded in this table.

Hours Watching Television
0 3 1 20 9
5 10 1 10 4
14 2 4 4 5
  1. Construct a [latex]98\%[/latex] confidence interval for the mean number of hours statistics students will spend watching television in one week.
  2. Interpret the confidence interval found in part 1.
  3. Is it reasonable to conclude that the mean number of hours statistics students spend watching television in one week is [latex]5[/latex]? Explain.
Click to see Solution

 

  1. Function t.inv.2t
    Field 1 0.02
    Field 2 14
    Answer 2.6244…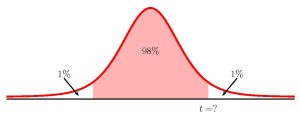

    Graph of a t-distribution curve. Along the horizontal axis the point t is labeled. There is a vertical line from t to the normal distribution curve. The area under the curve in the middle of the distribution is labeled 98%. The area in the left tail is labeled 1%. The area in the right tail is labeled 1%.[latex]\begin{eqnarray*}\\\text{Lower Limit}&=&\overline{x}-t\times\frac{s}{\sqrt{n}}\\&=&6.133...-2.6244...\times\frac{5.514...}{\sqrt{15}}\\&=&2.397\\\\\text{Upper Limit}&=&\overline{x}+t\times\frac{s}{\sqrt{n}}\\&=&6.133...+2.6244...\times\frac{5.514...}{\sqrt{15}}\\&=&9.870\\\\\end{eqnarray*}[/latex]

  2. We are [latex]98\%[/latex] confident that the mean number of hours statistics students will spend watching television in one week is between [latex]2.397[/latex] hours and [latex]9.870[/latex] hours.
  3. It is reasonable to assume the mean number of hours statistics students will spend watching television in one week is [latex]5[/latex] hours because [latex]5[/latex] is inside the confidence interval.

Exercises

  1. A zoo keeper wants to estimate the mean weight of newborn elephants. From a sample of [latex]50[/latex] newborn elephants, the mean weight was [latex]122[/latex] kg with a standard deviation of [latex]5.5[/latex] kg.
    1. Construct a [latex]95\%[/latex] confidence interval for the mean weight of newborn elephants.
    2. Interpret the confidence interval found in part (a).
    3. What will happen to the confidence interval obtained if [latex]500[/latex] newborn elephants are weighed instead of [latex]50[/latex]?
    Click to see Answer
    1. [latex]\text{Lower Limit}=120.33[/latex], [latex]\text{Upper Limit}=123.56[/latex]
    2. There is a [latex]95\%[/latex] probability that the mean weight of newborn elephants is between [latex]120.44[/latex] kg and [latex]123.56[/latex] kg.
    3. The confidence interval will get narrower.

     

  2. A researcher in Sweden wants to estimate the mean height of adult Swedish men. The researcher takes a sample of [latex]45[/latex] adult Swedish men and finds the mean height in the sample is [latex]177.5[/latex] cm with a standard deviation of [latex]7[/latex] cm.
    1. Construct a [latex]98\%[/latex] confidence interval for the mean height of adult Swedish men.
    2. Interpret the confidence interval found in part (a).
    3. The research claims that the mean height of adult Swedish men is [latex]187.5[/latex] cm. Can the research make this claim? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=174.98[/latex], [latex]\text{Upper Limit}=180.02[/latex]
    2. There is a [latex]98\%[/latex] probability that the mean height of adult Swedish men is between [latex]174.98[/latex] cm and [latex]180.02[/latex] cm.
    3. No, because [latex]187.5[/latex] cm is outside the confidence interval.

     

  3. Announcements for [latex]84[/latex] upcoming engineering conferences were randomly picked from a stack of IEEE Spectrum magazines. The mean length of the conferences was [latex]3.94[/latex] days with a standard deviation of [latex]1.28[/latex] days.
    1. Construct a [latex]97\%[/latex] confidence interval for the mean length of time of engineering conferences.
    2. Interpret the confidence interval found in part (a).
    3. Is it reasonable to claim that the mean length of time of engineering conferences is [latex]3[/latex] days? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=3.63[/latex], [latex]\text{Upper Limit}=4.25[/latex]
    2. There is a [latex]97\%[/latex] probability that the mean length of time of engineering conferences is between [latex]3.63[/latex] days and [latex]4.25[/latex] days.
    3. No, because [latex]3[/latex] cm is outside the confidence interval.

     

  4. A hospital is trying to cut down on emergency room wait times. It is interested in the amount of time patients must wait before being called back to be examined. An investigation committee randomly surveyed [latex]70[/latex] patients and found that the sample mean was [latex]1.5[/latex] hours with a sample standard deviation of [latex]0.5[/latex] hours.
    1. Construct a [latex]99\%[/latex] confidence interval for the mean wait time in the emergency room.
    2. Interpret the confidence interval found in part (a).
    3. Is it reasonable to claim that the mean wait time is [latex]2[/latex] hours? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=1.34[/latex], [latex]\text{Upper Limit}=1.66[/latex]
    2. There is a [latex]99\%[/latex] probability that the mean wait time in the emergency room is between [latex]1.34[/latex] days and [latex]1.66[/latex] days.
    3. No, because [latex]2[/latex] cm is outside the confidence interval.

     

  5. A researcher wants to estimate the mean time adults spend watching television per month. In a sample of [latex]108[/latex] adults, the mean time spent watching television per month was [latex]151[/latex] hours with a standard deviation of [latex]32[/latex] hours.
    1. Construct a [latex]93\%[/latex] confidence interval for the mean time adults spend watching television per month.
    2. Interpret the confidence interval found in part (a).
    3. The researcher claims that the mean time adults spend watching television per month is [latex]155[/latex] hours. Can the researcher make this claim? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=145.36[/latex], [latex]\text{Upper Limit}=156.64[/latex]
    2. There is a [latex]93\%[/latex] probability that the mean time adults spend watching television per month is between [latex]145.36[/latex] hours and [latex]156.64[/latex] hours.
    3. No, because [latex]3[/latex] cm is outside the confidence interval.

     

  6. A researcher wants to estimate the mean enrollment at the country’s post-secondary institutions. A random survey of enrollment numbers at [latex]35[/latex] post-secondary institutions across the country yielded the following figures:
    6,414 4,300 5,481 6,357 17,500 13,713 1,263
    1,550 5,944 5,200 27,000 9,200 17,768 7,285
    2,109 5,722 5,853 9,414 7,380 7,493 28,165
    9,350 2,825 2,750 7,681 18,314 2,771 5,080
    21,828 2,044 10,012 3,200 6,557 2,861 11,622
    1. Construct a [latex]95\%[/latex] confidence interval for the mean enrollment at post-secondary institutions across the country.
    2. Interpret the confidence interval found in part (a).
    3. Is it reasonable to conclude that the mean enrollment at post-secondary institutions across the country is [latex]15,000[/latex] students? Explain.
    4. What will happen to the confidence interval if [latex]500[/latex] post-secondary institutions were surveyed instead of [latex]35[/latex]?
    Click to see Answer
    1. [latex]\text{Lower Limit}=6,243.44[/latex], [latex]\text{Upper Limit}=11,014.05[/latex]
    2. There is a [latex]95\%[/latex] probability that the mean enrollment at post-secondary institutions across the country is between [latex]6,243.44[/latex] students and [latex]11,014.05[/latex] students.
    3. No, because [latex]15,000[/latex] students is outside the confidence interval.
    4. The confidence interval would get narrower.

     

  7. Suppose that a committee is studying whether or not there is a waste of time in our judicial system. The committee is interested in the mean amount of time individuals spend at the courthouse waiting to be called for jury duty. The committee randomly surveyed [latex]81[/latex] people who recently served as jurors and found that the sample mean wait time was [latex]8[/latex] hours with a sample standard deviation of [latex]4[/latex] hours.
    1. Construct a [latex]96\%[/latex] confidence interval for the mean time individuals spend waiting to be called for jury duty.
    2. Explain in a complete sentence what the confidence interval means.
    Click to see Answer
    1. [latex]\text{Lower Limit}=7.07[/latex], [latex]\text{Upper Limit}=8.93[/latex]
    2. There is a [latex]96\%[/latex] probability that the mean time individuals spend waiting to be called for jury duty is between [latex]7.07[/latex] hours and [latex]8.93[/latex] hours.

     

  8. A pharmaceutical company makes tranquillizers. Researchers in a hospital used the drug on a random sample of patients and recorded the length of time, in hours, that the tranquillizer lasted. The data is recorded as follows:
    Tranquilizer Duration (hours)
    2.9 2.3 2.4 2.6 3.2 2
    3 2.5 2.5 3.2 3 2.8
    2 2.9 2.6 3.2 2.5 2.6
    2 2.6 2.5 2.5 2.5 2.7
    3.1 2.5 2.8 2.4 2.3 2.9
    1. Construct a [latex]94\%[/latex] confidence interval for the mean length of time the tranquillizers last.
    2. What does it mean to be “[latex]94\%[/latex] confident” in this problem?
    3. Can the researchers claim that the mean length of time the tranquillizer lasts is [latex]2.7[/latex] hours? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=2.51[/latex], [latex]\text{Upper Limit}=2.76[/latex]
    2. There is a [latex]94\%[/latex] probability that the mean length of time the tranquillizer lasts is between [latex]2.51[/latex] hours and [latex]2.76[/latex] hours.
    3. Yes, because [latex]2.7[/latex] hours is inside the confidence interval.

     

  9. Unoccupied seats on flights cause airlines to lose revenue. Suppose a large airline wants to estimate the mean number of unoccupied seats per flight over the past year. To accomplish this, the records of [latex]225[/latex] flights are randomly selected, and the number of unoccupied seats is noted for each of the sampled flights. In the sample, the mean number of unoccupied seats is [latex]11.6[/latex] seats with a standard deviation is [latex]4.1[/latex] seats.
    1. Construct a [latex]92\%[/latex] confidence interval for the mean number of unoccupied seats per flight.
    2. Interpret the confidence interval found in part (a).
    3. Is it reasonable for the airlines to claim that the mean number of unoccupied seats per flight is [latex]15[/latex]? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=11.12[/latex], [latex]\text{Upper Limit}=12.08[/latex]
    2. There is a [latex]92\%[/latex] probability that the mean number of unoccupied seats per flight is between [latex]11.12[/latex] seats and [latex]12.08[/latex] seats.
    3. No, because [latex]15[/latex] seats is outside the confidence interval.

     

  10. A used car dealership wants to estimate the mean cost of a used car. In a recent sample of [latex]84[/latex] used car sales costs, the sample mean was [latex]\$6,425[/latex] with a standard deviation of [latex]\$3,156[/latex].
    1. Construct a [latex]98\%[/latex] confidence interval for the mean cost of a used car.
    2. Explain what a “[latex]98\%[/latex] confidence interval” means for this study.
    3. Can the used car dealership claim that the mean cost of a used car is [latex]\$7,000[/latex]? Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=5,608.17[/latex], [latex]\text{Upper Limit}=7,241.83[/latex]
    2. There is a [latex]98\%[/latex] probability that the mean cost of a used car is between [latex]\$5,608.17[/latex] and [latex]\$7,241.83[/latex] seats.
    3. Yes, because [latex]\$7,000[/latex] is inside the confidence interval.

     

  11. A local bargain hunter wants to estimate the mean dollar amount off that coupons provided by local retailers provide to consumers. The bargain hunter takes a random sample of coupons and records the amount off, in dollars, offered by each coupon:
    Coupon Savings (in dollars)
    3.00 0.70 0.80 2.20 1.90 1.80
    0.50 2.60 1.80 2.40 0.60 2.80
    0.50 2.50 2.60 2.20 2.80 1.30
    2.80 1.60 2.50 2.80 2.20 1.00
    1.70 1.60 1.10 1.20 1.90 2.70
    2.80 1.20 1.40 2.40 2.70 1.70
    1. Construct a [latex]99\%[/latex] confidence interval for the mean dollar amount off of coupons.
    2. Interpret the confidence interval found in part (a).
    3. Can the bargain hunter claim that the mean dollar amount off provided by coupons is [latex]\$2.00[/latex]. Explain.
    Click to see Answer
    1. [latex]\text{Lower Limit}=1.55[/latex], [latex]\text{Upper Limit}=2.25[/latex]
    2. There is a [latex]99\%[/latex] probability that the mean amount off of coupons is between [latex]\$1.55[/latex] and [latex]\$2.25[/latex] seats.
    3. Yes, because [latex]\$2.00[/latex] is inside the confidence interval.

     

“7.3 Confidence Intervals for a Single Population Mean with Unknown Population Standard Deviation” and “7.6 Exercises” from Introduction to Statistics by Valerie Watts is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Introduction to Statistics - Second Edition Copyright © 2025 by Valerie Watts is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book