1.5 Decimals

Learning Objectives

By the end of this section, you will be able to:

  • Name and write decimals
  • Round decimals
  • Add and subtract decimals
  • Multiply and divide decimals
  • Convert decimals, fractions, and percents

Name and Write Decimals

Decimals are another way of writing fractions whose denominators are powers of 10.

[latex]0.1=\frac1{10}[/latex] [latex]0.1[/latex] is “one tenth”
[latex]0.01=\frac1{100}[/latex] [latex]0.01[/latex] is “one hundredth”
[latex]0.001=\frac1{1000}[/latex] [latex]0.001[/latex] is “one thousandth”
[latex]0.0001=\frac1{10000}[/latex] [latex]0.0001[/latex] is “one ten-thousandth”

Notice that “ten thousand” is a number larger than one, but “one ten-thousandth” is a number smaller than one. The “th” at the end of the name tells you that the number is smaller than one.

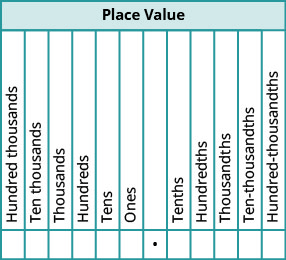
When we name a whole number, the name corresponds to the place value based on the powers of ten. We read 10,000 as “ten thousand” and 10,000,000 as “ten million.” Likewise, the names of the decimal places correspond to their fraction values. (Figure 1.5.1) shows the names of the place values to the left and right of the decimal point.

Place value of decimal numbers are shown to the left and right of the decimal point.

A table is shown with the title Place Value. From left to right the row reads “Hundred thousands,” “Ten thousands,” “Thousands,” “Hundreds,” “Tens,” and “Ones.” Then there is a blank cell and below it is a decimal point. To the right of this, the cells read “Tenths,” “Hundredths,” “Thousandths,” “Ten-thousandths,” and “Hundred-thousandths.”
Figure 1.5.1

Example 1

Name the decimal 4.3.

Solution

Step 1: Name the number to the left of the decimal point.

4 is to the left of the decimal point.

[latex]\begin{eqnarray*}&4.3&\\&\text{four ___________}&\\\end{eqnarray*}[/latex]

Step 2: Write ‘and’ for the decimal point.

[latex]\begin{eqnarray*}&\text{four and ________}&\end{eqnarray*}[/latex]

Step 3: Name the ‘number’ part to the right of the decimal point as if it were a whole number.

3 is to the right of the decimal point.

[latex]\begin{eqnarray*}&\text{four and three _________}&\end{eqnarray*}[/latex]

Step 4: Name the decimal place.

Four and three tenths.

Try It

1) Name the decimal: 6.7

Solution

Six and seven tenths.

2) Name the decimal: 5.8

Solution

Five and eight tenths.

We summarize the steps needed to name a decimal below.

How to

Name a Decimal.

  1. Name the number to the left of the decimal point.
  2. Write “and” for the decimal point.
  3. Name the “number” part to the right of the decimal point as if it were a whole number.
  4. Name the decimal place of the last digit.

 

Example 2

Name the decimal: -15.571

Solution

Step 1: Name the number to the left of the decimal point.

[latex]\begin{eqnarray*}&\text{negative fifteen _________}&\end{eqnarray*}[/latex]

Step 2: Write “and” for the decimal point.

[latex]\begin{eqnarray*}&\text{negative fifteen and _________}&\end{eqnarray*}[/latex]

Step 3: Name the number to the right of the decimal point.

[latex]\begin{eqnarray*}&\text{negative fifteen and five hundred seventy one _________}&\end{eqnarray*}[/latex]

Step 4: The 1 is in the thousandths place.

Negative fifteen and five hundred seventy-one thousandths.

Try It

3) Name the decimal: -13.461

Solution

Negative thirteen and four hundred sixty-one thousandths.

4) Name the decimal: -2.053.

Solution

Negative two and fifty-three thousandths.

When we write a check we write both the numerals and the name of the number. Let’s see how to write the decimal from the name.

Example 3

Write “fourteen and twenty-four thousandths” as a decimal.

Solution

Step 1: Look for the word ‘and’; it locates the decimal point. Place a decimal point under the word ‘and’.

Translate the words before ‘and’ into the whole number and place to the left of the decimal point.

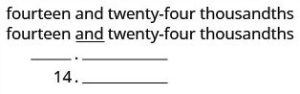
A table is given with four steps. The first step reads “Step 1: Look for the work ‘and’ – it locates the decimal point. Place a decimal point under the word ‘and’. Translate the words before ‘and’ into the whole number and place it to the left of the decimal point.” To the right of this, we have the words “fourteen and twenty-four thousandths.” Below this word, we have “fourteen and twenty-four thousandths” with the word “and” underlined. Below this word, we have a small blank space separated from a larger blank space by a decimal point. Under this, we have 14 in the small blank space followed by the decimal point and the larger blank space.
Figure 1.5.2

Step 2: Mark the number of decimal places needed to the right of the decimal point by noting the place value indicated by the last word.

The second step reads “Step 2: Mark the number of decimal places needed to the right of the decimal point by noting the place value indicated by the last word.” To the right of this it reads “The last word is thousandths.” To the right of this there is the number 14 followed by a decimal point and three small blank spaces. Under the blank spaces, the words “tenths,” “hundredths,” and “thousandths” are written.
Figure 1.5.3

Step 3: Translate the words after ‘and’ into the number to the right of the decimal point. 

Write the number in the spaces — putting the final digit in the last place.

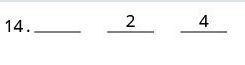
Step 3: Translate the words after ‘and’ into the number to the right of the decimal point. Write the number in the spaces – putting the final digit in the last place.” To the right of this, we have 14 followed by a decimal followed by a blank space followed by 2 and 4 on the other two previously blank spaces.
Figure 1.5.4

Step 4: Fill in zeros for empty place holders as needed. 

Zeros are needed in the tenths place.

Step 4: Fill in zeros for empty place holders as needed.” To the right of this, it reads “Zeros are needed in the tenths place.” To the right of this, we have 14 followed by a decimal point followed by 0, 2, and 4, respectively, on the blank spaces. Below this, we have “fourteen and twenty-four thousandths is written 14.024.
Figure 1.5.5

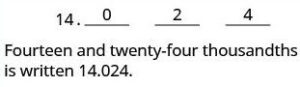
Try It

5) Write as a decimal: thirteen and sixty-eight thousandths.

Solution

13.068

6) Write as a decimal: five and ninety-four thousandths.

Solution

5.094

We summarize the steps to writing a decimal.

How to

Write a decimal.

  1. Look for the word “and”—it locates the decimal point.
    • Place a decimal point under the word “and.” Translate the words before “and” into the whole number and place it to the left of the decimal point.
    • If there is no “and,” write a “0” with a decimal point to its right.
  2. Mark the number of decimal places needed to the right of the decimal point by noting the place value indicated by the last word.
  3. Translate the words after “and” into the number to the right of the decimal point. Write the number in the spaces—putting the final digit in the last place.
  4. Fill in zeros for placeholders as needed.

 

Round Decimals

Rounding decimals is very much like rounding whole numbers. We will round decimals with a method based on the one we used to round whole numbers.

Example 4

Round 18.379 to the nearest hundredth.

Solution

Step 1: Locate the given place value and mark it with an arrow. 

A table is given with four steps. The first step reads “Step 1: Locate the given place value and mark it with an arrow.” To the right of this, we have the number 18.379; above it, are the words hundreds place, which has an arrow pointing to the 7.
Figure 1.5.6

Step 2: Underline the digit to the right of the given place value.

Step 2: Underline the digit to the right of the given place value.” To the right of this, we have 18.379 with the 9 underlined
Figure 1.5.7

Step 3: Is this digit greater than or equal to 5?

Yes: Add 1 to the digit in the given place value.
No: Do not change the digit in the given place value.

Step 3. Is this digit greater than or equal to 5? Below this reads, “Yes: add 1 to the digit in the given place value.” Below this reads, “No: do not change the digit in the given place value.” To the right of this, it says “Because 9 is greater than or equal to ” To the right of this, we have the number 18.379 with the 9 marked “delete” and the 7 marked “add 1.”
Figure 1.5.8

Step 4: Rewrite the number, removing all digits to the right of the rounding digit. 

Finally, the last step reads “Step 4. Rewrite the number, removing all digits to the right of the rounding digit.” To the right of this, we have 18.38 followed by “18.38 is 18.379 rounded to the nearest hundredth."
Figure 1.5.9

Try It

7) Round to the nearest hundredth: 1.047

Solution

1.05

8) Round to the nearest hundredth: 9.173

Solution

9.17

We summarize the steps for rounding a decimal here.

How to

Round Decimals.

  1. Locate the given place value and mark it with an arrow.
  2. Underline the digit to the right of the place value.
  3. Is this digit greater than or equal to 5?
    • Yes—add 1 to the digit in the given place value.
    • No—do not change the digit in the given place value.
  4. Rewrite the number, deleting all digits to the right of the rounding digit.

 

Example 5

Round 18.379 to the nearest

a. tenth
b. whole number.

 
Solution

Round 18.379

a. to the nearest tenth

Step 1: Locate the tenths place with an arrow.

Figure 1.5.10

Step 2: Underline the digit to the right of the given place value.

.
Figure 1.5.11

Step 3: Because 7 is greater than or equal to 5, add 1 to the 3.

.
Figure 1.5.12

Step 4: Rewrite the number, deleting all digits to the right of the rounding digit.

[latex]18.4[/latex]

Step 5: Notice that the deleted digits were NOT replaced with zeros.

So, [latex]18.379[/latex] rounded to the nearest tenth is [latex]18.4[/latex].

b. to the nearest whole number

Step 1: Locate the ones place with an arrow.

.
Figure 1.5.13

Step 2: Underline the digit to the right of the given place value.

.
Figure 1.5.14

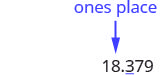
Step 3: Since 3 is not greater than or equal to 5, do not add 1 to the 8.

.
Figure 1.5.15

Step 4: Rewrite the number, deleting all digits to the right of the rounding digit.

So, 18.379 rounded to the nearest whole number is 18.

Try It

9) Round 6.582 to the nearest

a. hundredth
b. tenth
c. whole number.

Solution

a. 6.58
b. 6.6
c. 7

10) Round 15.2175 to the nearest

a. thousandth
b. hundredth
c. tenth.

Solution

a. 15.218
b. 15.22
c. 15.2

Add and Subtract Decimals

To add or subtract decimals, we line up the decimal points. By lining up the decimal points this way, we can add or subtract the corresponding place values. We then add or subtract the numbers as if they were whole numbers and then place the decimal point in the sum.

How to

Add or Subtract Decimals.

  1. Write the numbers so the decimal points line up vertically.
  2. Use zeros as placeholders, as needed.
  3. Add or subtract the numbers as if they were whole numbers. Then place the decimal point in the answer under the decimal points in the given numbers.

 

Example 6

Add: [latex]23.5+41.38[/latex]

Solution

Step 1: Write the numbers so the decimal points line up vertically.

[latex]\begin{array}{lllll}\;\;\;23.50\\ \underline{+41.38}\\ \end{array}[/latex]

Step 2: Put 0 as a placeholder after the 5 in 23.5. Remember, [latex]\frac5{10}=\frac{50}{100}[/latex] so, [latex]0.5 = 0.50[/latex].

[latex]\begin{array}{lllll}\;\;\;23.50\\ \underline{+41.38}\\ \end{array}[/latex]

Step 3: Add the numbers as if they were whole numbers. Then place the decimal point in the sum.

[latex]\begin{array}{lllll}\;\;\;23.50\\ \underline{+41.38}\\ \;\;\;64.88 \end{array}[/latex]

Try It

11) Add: [latex]4.8+11.69[/latex]

Solution

16.49

12) Add: [latex]5.123+18.47[/latex]

Solution

23.593

Example 7

Subtract: [latex]20-14.65[/latex]

Solution

Step 1: Write the numbers so the decimal points line up vertically.

[latex]\begin{array}{lllll}\;\;\;20.\\ \underline{-14.65}\\ \end{array}[/latex]

Step 2: Put in zeros to the right as placeholders.

Remember, 20 is a whole number, so place the decimal point after the 0.

[latex]\begin{array}{lllll}\;\;\;20.00\\ \underline{-14.65}\end{array}[/latex]

Step 3: Subtract and place the decimal point in the answer.

[latex]\begin{array}{lllll}\;\;\;20.00\\ \underline{-14.65}\\\;\;\;\;5.35\end{array}[/latex]

Try It

13) Subtract [latex]10-9.58[/latex].

Solution

0.42

14) Subtract: [latex]50-37.42[/latex].

Solution

12.58

Multiply and Divide Decimals

Multiplying decimals is very much like multiplying whole numbers—we just have to determine where to place the decimal point. The procedure for multiplying decimals will make sense if we first convert them to fractions and then multiply.

So let’s see what we would get as the product of decimals by converting them to fractions first. We will do two examples side-by-side. Look for a pattern!

[latex](0.\underset{1\;place}{\underbrace3)}\;\;\;\;\;\;\;\;(0.\underset{1\;place}{\underbrace7)}[/latex]
 [latex](0.\underset{1\;place}{\underbrace2)}\;\;\;\;\;\;\;\;(0.\underset{2\;places}{\underbrace{46})}[/latex]
Convert to fractions. [latex]\frac3{10}\cdot\frac7{10}[/latex] [latex]\frac2{10}\cdot\frac{46}{100}[/latex]
Multiply. [latex]\frac{21}{100}[/latex] [latex]\frac{92}{1000}[/latex]
Convert to decimals. [latex](0.\underset{2\;places}{\underbrace{21})}[/latex] [latex](0.\underset{3\;places}{\underbrace{096})}[/latex]

Notice, in the first example, we multiplied two numbers that each had one digit after the decimal point and the product had two decimal places. In the second example, we multiplied a number with one decimal place by a number with two decimal places and the product had three decimal places.

We multiply the numbers just as we do whole numbers, temporarily ignoring the decimal point. We then count the number of decimal points in the factors and that sum tells us the number of decimal places in the product.

The rules for multiplying positive and negative numbers apply to decimals, too, of course!

When multiplying two numbers,

  • if their signs are the same the product is positive.
  • if their signs are different the product is negative.

When we multiply signed decimals, first we determine the sign of the product and then multiply as if the numbers were both positive. Finally, we write the product with the appropriate sign.

How to

Multiply Decimals

  1. Determine the sign of the product.
  2. Write in vertical format, lining up the numbers on the right. Multiply the numbers as if they were whole numbers, temporarily ignoring the decimal points.
  3. Place the decimal point. The number of decimal places in the product is the sum of the number of decimal places in the factors.
  4. Write the product with the appropriate sign.

 

Example 8

Multiply: [latex](-3.9)(4.075)[/latex]

Solution

Step 1: The signs are different. The product will be negative.
Step 2: Write in vertical format, lining up the numbers on the right.

[latex]\begin{align*} 4.075&\\ \underline{\times\;\;3.9}&\\ \end{align*}[/latex]

Step 3: Multiply.

[latex]\begin{align*}4.075&\\ \underline{\times\;\;3.9}&\\158925&\end{align*}[/latex]

Step 4: Add the number of decimal places in the factors [latex](1 + 3)[/latex].

[latex](-3.\underbrace{9}_{1\;place})[/latex]   [latex](4.\underbrace{075}_{3\;places})[/latex]

Step 5: Place the decimal point 4 places from the right.

[latex]\begin{align*}4.075\\\underline{\times\;\;3.9}\\15.\underbrace{8925}&\\ \;\;\;\;\text{4 places}\end{align*}[/latex]

Step 6: The signs are different, so the product is negative.

[latex](−3.9)(4.075) = −15.8925[/latex]

Try It

15) Multiply: [latex]-4.5(6.107)[/latex]

Solution

-27.4815

16) Multiply: [latex]-10.79(8.12)[/latex]

Solution

-87.6148

In many of your other classes, especially in the sciences, you will multiply decimals by powers of 10 (10, 100, 1000, etc.). If you multiply a few products on paper, you may notice a pattern relating the number of zeros in the power of 10 to number of decimal places we move the decimal point to the right to get the product.

How to

Multiply a Decimal by a Power of Ten

  1. Move the decimal point to the right in the same number of places as the number of zeros in the power of 10.
  2. Add zeros at the end of the number as needed.

 

Example 9

Multiply 5.63

a. by 10
b. by 100
c. by 1,000

Solution

By looking at the number of zeros in the multiple of ten, we see the number of places we need to move the decimal to the right.

a.

Step 1: There is 1 zero in 10, so move the decimal point 1 place to the right.

[latex]5.63(10)[/latex]

Fig. 1.5.16

b.

Step 1: There are 2 zeros in 100, so move the decimal point 2 places to the right.

[latex]5.63(100)[/latex]

.
Figure 1.5.17

c.

Step 1: There are 3 zeros in 1,000, so move the decimal point 3 places to the right.

[latex]5.63(1000)[/latex]

.
Figure 1.5.18

Step 2: A zero must be added at the end.

[latex]5,630[/latex]

Try It

17) Multiply 2.58

a. by 10
b. by 100
c. by 1,000

Solution

a. 25.8
b. 258
c. 2,580

18) Multiply 14.2
a. by 10
b. by 100
c. by 1,000

Solution

a. 142
b. 1,420
c. 14,200

Just as with multiplication, dividing decimals is like dividing whole numbers. We just have to figure out where the decimal point must be placed.

To divide decimals, determine what power of 10 to multiply the denominator by to make it a whole number. Then multiply the numerator by that same power of [latex]10[/latex]. Because of the equivalent fractions property, we haven’t changed the value of the fraction! The effect is to move the decimal points in the numerator and denominator the same number of places to the right. For example:

[latex]\frac{0.8}{0.4}[/latex]

[latex]\frac{0.8(10)}{0.4(10)}[/latex]

[latex]\frac{8}{4}[/latex]

We use the rules for dividing positive and negative numbers with decimals, too. When dividing signed decimals, first determine the sign of the quotient and then divide as if the numbers were both positive. Finally, write the quotient with the appropriate sign.

We review the notation and vocabulary for division:

a divided by b equals c. Then "a" is dividend and "b" is divisor and "c" is quotient.
Figure 1.5.19

We’ll write the steps to take when dividing decimals, for easy reference.

HOW TO

Divide Decimals.

  1. Determine the sign of the quotient.
  2. Make the divisor a whole number by “moving” the decimal point all the way to the right. “Move” the decimal point in the dividend to the same number of places—adding zeros as needed.
  3. Divide. Place the decimal point in the quotient above the decimal point in the dividend.
  4. Write the quotient with the appropriate sign.

Example 10

Divide: [latex]-25.65\div (-0.06)[/latex]

Solution

Remember, you can “move” the decimals in the divisor and dividend because of the Equivalent Fractions Property.

Step 1: The signs are the same.

The quotient is positive.

Step 2: Make the divisor a whole number by “moving” the decimal point all the way to the right.
Step 3: “Move” the decimal point in the dividend the same number of places.

.
Figure 1.5.20

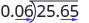
Step 4: Divide.

Place the decimal point in the quotient above the decimal point in the dividend.

.
Figure 1.5.21

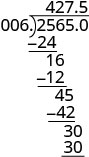
Step 5: Write the quotient with the appropriate sign.

[latex]-25.65\div (-0.06)=427.5[/latex]

Try It

19) Divide: [latex]-23.492\div (-0.04)[/latex]

Solution

587.3

20) Divide: [latex]-4.11\div (-0.12)[/latex]

Solution

34.25

A common application of dividing whole numbers into decimals is when we want to find the price of one item that is sold as part of a multi-pack. For example, suppose a case of 24 water bottles costs $3.99. To find the price of one water bottle, we would divide $3.99 by 24. We show this division in Example 1.5.13. In calculations with money, we will round the answer to the nearest cent (hundredth).

Example 11

Divide: [latex]\$3.99\div 24[/latex].

Solution

Step 1: Place the decimal point in the quotient above the decimal point in the dividend.
Step 2: Divide as usual.

When do we stop? Since this division involves money, we round it to the nearest cent (hundredth.) To do this, we must carry the division to the thousandths place.

.
Figure 1.5.22

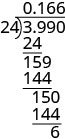
Step 3: Round to the nearest cent.

[latex]\begin{eqnarray*}0.166&\approx&0.17\\\$3.99\div24&\approx&\$0.17\end{eqnarray*}[/latex]

Try It

21) Divide: [latex]\$6.99\div 36[/latex]

Solution

0.19

22) Divide: [latex]\$4.99\div 12[/latex]

Solution

0.42

Convert Decimals, Fractions, and Percents

We convert decimals into fractions by identifying the place value of the last (farthest right) digit. In the decimal 0.03, the 3 is in the hundredth place, so 100 is the denominator of the fraction equivalent to 0.03.

[latex]0.03=\frac{3}{100}[/latex]

Notice, that when the number to the left of the decimal is zero, we get a fraction whose numerator is less than its denominator. Fractions like this are called proper fractions.

The steps to take to convert a decimal to a fraction are summarized in the procedure box.

How to

Convert a Decimal to a Proper Fraction.

  1. Determine the place value of the final digit.
  2. Write the fraction.
    • numerator—the “numbers” to the right of the decimal point
    • denominator—the place value corresponding to the final digit

 

Example 12

Write [latex]0.374[/latex] as a fraction.

Solution

Step 1: Determine the place value of the final digit.

.
Figure 1.5.23

Step 2: Write the fraction for 0.374:

  • The numerator is 374.
  • The denominator is 1,000.

[latex]\frac{374}{1000}[/latex]

Step 3: Simplify the fraction.

[latex]\frac{2\;\times\;187}{2\;\times\;500}[/latex]

Step 4: Divide out the common factors.

[latex]\frac{187}{500}[/latex]

So, [latex]0.374=\frac{187}{500}[/latex].

Did you notice that the number of zeros in the denominator of [latex]\frac{374}{1,000}[/latex] is the same as the number of decimal places in [latex]0.374[/latex]?

Try It

23) Write [latex]0.234[/latex] as a fraction.

Solution

[latex]\frac{117}{500}[/latex]

24)Write [latex]0.024[/latex] as a fraction.

Solution

[latex]\frac{3}{125}[/latex]

We’ve learned to convert decimals to fractions. Now we will do the reverse—convert fractions to decimals. Remember that the fraction bar means division. So [latex]\frac{4}{5}[/latex] can be written [latex]4\div5[/latex] or 5[latex]\overline)4[/latex] . This leads to the following method for converting a fraction to a decimal.

How to

Convert a Fraction to a Decimal.

To convert a fraction to a decimal, divide the numerator of the fraction by the denominator of the fraction.

 

Example 13

Write [latex]-\frac{5}{8}[/latex] as a decimal.

Solution

Step 1: Since a fraction bar means division, we begin by writing [latex]-\frac{5}{8}[/latex] as [latex]-8)\overline{5}[/latex]

Step 2: Now divide.

This is a long division problem with 8 dividing 5.000 and 0.625 as the quotient. Below 5.000 we have 48, a solid horizontal line, 20, 16, a solid horizontal line, 40, 40, and a final horizontal line. So five eighths equals 0.625.
Figure 1.5.24

Try It

25) Write [latex]\frac{-7}{8}[/latex] as a decimal.

Solution

[latex]-0.875[/latex]

26) Write [latex]\frac{-3}{8}[/latex] as a decimal.

Solution

[latex]-0.375[/latex]

When we divide, we will not always get a zero remainder. Sometimes the quotient ends up with a decimal that repeats. A repeating decimal is a decimal in which the last digit or group of digits repeats endlessly. A bar is placed over the repeating block of digits to indicate it repeats.

Repeating Decimal

A repeating decimal is a decimal in which the last digit or group of digits repeats endlessly.

A bar is placed over the repeating block of digits to indicate it repeats.

Example 14

Write [latex]\frac{43}{22}[/latex] as a decimal.

Solution
The number 43/22 is given. The direction is given to “Divide 43 by 22.” A long division problem is given with 22 dividing 43.00000 with 1.95454 as the quotient. Below 43.00000 we have 22, a solid horizontal line, 210, 198, a solid horizontal line, 120, 110, a horizontal line, 100, 88, a solid horizontal line, 120, 110, a solid horizontal line, 100, 88, a solid horizontal line, and then three dots. It is noted that the 120 repeats and that the 100 repeats. This is further explicated as “The pattern repeats, so the numbers in the quotient will repeat as well. At the end, we are given the statement that 43/22 equals 1.954 with a small horizontal line over the 54.
Figure 1.5.25

Try It

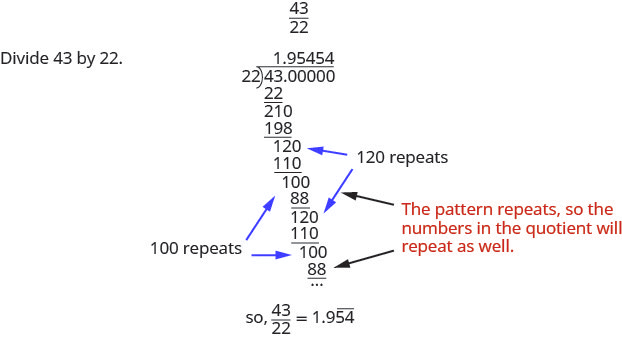
27) Write [latex]\frac{27}{11}[/latex] as a decimal.

Solution

[latex]2.\bar{45}[/latex]

28) Write [latex]\frac{51}{22}[/latex] as a decimal.

Solution

[latex]2.3\bar{18}[/latex]

Sometimes we may have to simplify expressions with fractions and decimals together.

Example 15

Simplify: [latex]\frac{7}{8}+6.4[/latex]

Solution

First we must change one number so both numbers are in the same form. We can change the fraction to a decimal, or change the decimal to a fraction. Usually it is easier to change the fraction to a decimal.

Step 1: Change [latex]\frac{7}{8}[/latex] to a decimal.

.
Figure 1.5.26

Step 2: Add.

[latex]0.875+6.4 = 7.275[/latex]

So, [latex]\frac{7}{8}+6.4=7.275[/latex]

Try It

29) Simplify: [latex]\frac{3}{8}+4.9[/latex]

Solution

[latex]5.275[/latex]

30) Simplify: [latex]5.7+\frac{13}{20}[/latex]

Solution

[latex]6.35[/latex]

A percent is a ratio whose denominator is 100. Percent means per hundred. We use the percent symbol, %, to show percent.

Percent

A percent is a ratio whose denominator is 100.

Since a percent is a ratio, it can easily be expressed as a fraction. Percent means per 100, so the denominator of the fraction is 100. We then change the fraction to a decimal by dividing the numerator by the denominator.

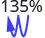
[latex]6\%[/latex] [latex]78\%[/latex] [latex]135\%[/latex]
Write as a ratio with a denominator of 100. [latex]\frac{6}{100}[/latex] [latex]\frac{78}{100}[/latex] [latex]\frac{135}{100}[/latex]
Change the fraction to a decimal by dividing the numerator by the denominator. [latex]0.06[/latex] [latex]0.78[/latex] [latex]1.35[/latex]

Do you see the pattern? To convert a percent number to a decimal number, we move the decimal point two places to the left.

The first part of this figure shows 6% with an arrow drawn from between the 6 and the percentage sign to the space to the left of 6 and then to the space further to the left of that space. Below this, the number 0.06 is given. The second part of this figure shows 78% with an arrow drawn from between the 8 and the percentage sign to the space between the 7 and the 8 and then to the space to the left of the 7. Below this, the number 0.78 is given. The third part of this figure shows 2.7% with an arrow drawn from the decimal point to the space to the left of the 2 and then to the space further to the left of that space. Below this, the number 0.027 is given. The fourth part of this figure shows 135% with an arrow drawn from between the 5 and the percentage sign to the space between 3 and 5 and then to the space between 1 and 3. Below this, the number 1.35 is given.
Figure 1.5.27

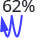
Example 16

Convert each percent to a decimal:

a. [latex]62\%[/latex]
b. [latex]135\%[/latex]
c. [latex]5.7\%[/latex]

Solution

a.

Step 1: Move the decimal point two places to the left.

.
Figure 1.5.28

[latex]0.62[/latex]

b.

Step 1: Move the decimal point two places to the left.

.
Figure 1.5.29

[latex]1.35[/latex]

c.

Step 1: Move the decimal point two places to the left.

.
Figure 1.5.30

[latex]0.057[/latex]

Try It

31) Convert each percent to a decimal:
a. [latex]9\%[/latex]
b. [latex]87\%[/latex]
c. [latex]3.9\%[/latex]

Solution

a. [latex]0.09[/latex]
b. [latex]0.87[/latex]
c. [latex]0.039[/latex]

32) Convert each percent to a decimal:
a. [latex]3\%[/latex]
b. [latex]91\%[/latex]
c. [latex]8.3\%[/latex]

Solution

a. [latex]0.03[/latex]
b. [latex]0.91[/latex]
c. [latex]0.083[/latex]

Converting a decimal to a percent makes sense if we remember the definition of percent and keep place value in mind.

To convert a decimal to a percent, remember that percent means per hundred. If we change the decimal to a fraction whose denominator is 100, it is easy to change that fraction to a percent.

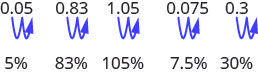
[latex]0.83[/latex] [latex]1.05[/latex] [latex]0.075[/latex]
Write as a fraction. [latex]\frac{83}{100}[/latex] [latex]\frac{5}{100}[/latex] [latex]\frac{75}{1000}[/latex]
The denominator is 100. [latex]\frac{105}{100}[/latex] [latex]\frac{7.5}{100}[/latex]
Write the ratio as a percent. [latex]83\%[/latex] [latex]105\%[/latex] [latex]7.5\%[/latex]

Recognize the pattern? To convert a decimal to a percent, we move the decimal point two places to the right and then add the percent sign.

The first part of this figure shows 0.05 with an arrow drawn from the decimal point to the space between 0 and 5 and then to the space after 5. Below this, the number 5% is given. The second part of this figure shows 0.83 with an arrow drawn from the decimal point to the space between 8 and 3 and then to the space after 3. Below this, the number 83% is given. The third part of this figure shows 1.05 with an arrow drawn from the decimal point to the space between 0 and 5 and then to the space after 5. Below this, the number 105% is given. The fourth part of this figure shows 0.075 with an arrow drawn from the decimal point to the space between 0 and 7 and then to the space between 7 and 5. Below this, the number 7.5% is given. The fifth part of this figure shows 0.3 with an arrow drawn from the decimal point to the space after 3 and then to space further to the right of that 3. Below this, the number 30% is given.
Figure 1.5.31

Example 17

Convert each decimal to a percent:

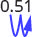
a. [latex]0.51[/latex]
b. [latex]1.25[/latex]
c. [latex]0.093[/latex]

Solution

a.

Step 1: Move the decimal point two places to the right.

.
Figure 1.5.32

[latex]51\%[/latex]

b.

Step 1: Move the decimal point two places to the right.

.
Figure 1.5.33

[latex]125\%[/latex]

c.

Step 1: Move the decimal point two places to the right.

.
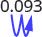
Figure 1.5.34

[latex]9.3\%[/latex]

Try It

33) Convert each decimal to a percent:
a. [latex]0.17[/latex]
b. [latex]1.75 [/latex]
c. [latex]0.0825[/latex]

Solution

a. [latex]17\%[/latex]
b. [latex]175\%[/latex]
c. [latex]8.25\%[/latex]

34) Convert each decimal to a percent:
a. [latex]0.41 [/latex]
b. [latex]2.25[/latex]
c. [latex]0.0925[/latex]

Solution

a. [latex]41\%[/latex]
b. [latex]225\%[/latex]
c. [latex]9.25\%[/latex]

Key Concepts

  • Name a Decimal
    1. Name the number to the left of the decimal point.
    2. Write ”and” for the decimal point.
    3. Name the “number” part to the right of the decimal point as if it were a whole number.
    4. Name the decimal place of the last digit.
  • Write a Decimal
    1. Look for the word ‘and’—it locates the decimal point. Place a decimal point under the word ‘and.’ Translate the words before ‘and’ into the whole number and place it to the left of the decimal point. If there is no “and,” write a “0” with a decimal point to its right.
    2. Mark the number of decimal places needed to the right of the decimal point by noting the place value indicated by the last word.
    3. Translate the words after ‘and’ into the number to the right of the decimal point. Write the number in the spaces—putting the final digit in the last place.
    4. Fill in zeros for placeholders as needed.
  • Round a Decimal
    1. Locate the given place value and mark it with an arrow.
    2. Underline the digit to the right of the place value.
    3. Is this digit greater than or equal to 5? Yes—add 1 to the digit in the given place value. No—do not change the digit in the given place value.
    4. Rewrite the number, deleting all digits to the right of the rounding digit.
  • Add or Subtract Decimals
    1. Write the numbers so the decimal points line up vertically.
    2. Use zeros as place holders, as needed.
    3. Add or subtract the numbers as if they were whole numbers. Then place the decimal in the answer under the decimal points in the given numbers.
  • Multiply Decimals
    1. Determine the sign of the product.
    2. Write in vertical format, lining up the numbers on the right. Multiply the numbers as if they were whole numbers, temporarily ignoring the decimal points.
    3. Place the decimal point. The number of decimal places in the product is the sum of the decimal places in the factors.
    4. Write the product with the appropriate sign.
  • Multiply a Decimal by a Power of Ten
    1. Move the decimal point to the right the same number of places as the number of zeros in the power of 10.
    2. Add zeros at the end of the number as needed.
  • Divide Decimals
    1. Determine the sign of the quotient.
    2. Make the divisor a whole number by “moving” the decimal point all the way to the right. “Move” the decimal point in the dividend the same number of places – adding zeros as needed.
    3. Divide. Place the decimal point in the quotient above the decimal point in the dividend.
    4. Write the quotient with the appropriate sign.
  • Convert a Decimal to a Proper Fraction
    1. Determine the place value of the final digit.
    2. Write the fraction: numerator—the ‘numbers’ to the right of the decimal point; denominator—the place value corresponding to the final digit.
  • Convert a Fraction to a Decimal Divide the numerator of the fraction by the denominator

Glossary

decimal
A decimal is another way of writing a fraction whose denominator is a power of ten.
percent
A percent is a ratio whose denominator is 100.
repeating decimal
A repeating decimal is a decimal in which the last digit or group of digits repeats endlessly.

Exercises: Name and Write Decimals

Instructions: For questions 1–8, write as a decimal.

1)  Twenty-nine and eighty-one hundredths

2)  Sixty-one and seventy-four hundredths

3)  Seven tenths

4)  Six tenths

5)  Twenty-nine thousandths

6)  Thirty-five thousandths

7)  Negative eleven and nine ten-thousandths

8)  Negative fifty-nine and two ten-thousandths

Odd Answers

1)  [latex]29.81[/latex]

3)  [latex]0.7[/latex]

5)  [latex]0.029[/latex]

7)  [latex]-11.0009[/latex]

Exercises: Name and Write Decimals

Instructions: For questions 9–16, name each decimal.

9)  [latex]5.5[/latex]

10)  [latex]14.02[/latex]

11)  [latex]8.71[/latex]

12)  [latex]2.64[/latex]

13)  0.002

14)  0.479

15)  [latex]−17.9[/latex]

16)  [latex]-31.4[/latex]

Odd Answers

9)  five and five tenths

11)  eight and seventy-one hundredths

13)  two thousandths

15)  negative seventeen and nine tenths

Exercises: Round Decimals

Instructions: For questions 17–20, round each number to the nearest tenth.

17)  [latex]0.67[/latex]

18)  [latex]0.49[/latex]

19)  [latex]2.84[/latex]

20)  [latex]4.63[/latex]

Odd Answers

17)  [latex]0.7[/latex]

19)  [latex]2.8[/latex]

Exercises: Round Decimals

Instructions: For questions 21–26, round each number to the nearest hundredth.

21)  [latex]0.845[/latex]

22)  [latex]0.761[/latex]

23)  [latex]0.299[/latex]

24)  [latex]0.697[/latex]

25)  [latex]4.098[/latex]

26)  [latex]7.096[/latex]

Odd Answers

21)  [latex]0.85[/latex]

23)  [latex]0.30[/latex]

25)  [latex]4.10[/latex]

Exercises: Round Decimals

Instructions: For questions 27–30, round each number to the nearest

a)  hundredth
b)  tenth
c)  whole number

27)  [latex]5.781[/latex]

28)  [latex]1.6381[/latex]

29)  [latex]63.479[/latex]

30)  [latex]84.281[/latex]

Odd Answers

27a)  [latex]5.78[/latex]
27b)  [latex]5.8[/latex]
27c) [latex]6[/latex]

29a)  [latex]63.48[/latex]
29b)  [latex]63.5[/latex]
29c)  [latex]63[/latex] 

Exercises: Add and Subtract Decimals

Instructions: For questions 31–48, add or subtract.

31)  [latex]16.92+7.56[/latex]

32)  [latex]248.25-91.29[/latex]

33)  [latex]21.76-30.99[/latex]

34)  [latex]38.6+13.67[/latex]

35)  [latex]-16.53-24.38[/latex]

36)  [latex]-19.47-32.58[/latex]

37)  [latex]-38.69+31.47[/latex]

38)  [latex]29.83+19.76[/latex]

39)  [latex]72.5-100[/latex]

40)  [latex]86.2-100[/latex]

41)  [latex]15+0.73[/latex]

42)  [latex]27+0.87[/latex]

43)  [latex]91.95-(-10.462)[/latex]

44)  [latex]94.69-(-12.678)[/latex]

45)  [latex]55.01-3.7[/latex]

46)  [latex]59.08-4.6[/latex]

47)  [latex]2.51-7.4[/latex]

48)  [latex]3.84-6.1[/latex]

Odd Answers

31)  [latex]24.48[/latex]

33)  [latex]-9.23[/latex]

35)  [latex]-40.91[/latex]

37)  [latex]-7.22[/latex]

39)  [latex]-27.5[/latex]

41)  [latex]15.73[/latex]

43)  [latex]102.412[/latex]

45)  [latex]51.31[/latex]

47)  [latex]-4.89[/latex]

Exercises: Multiply Decimals

Instructions: For questions 49–62, multiply.

49)  [latex](0.24)(0.6)[/latex]

50)  [latex](0.81)(0.3)[/latex]

51)  [latex](5.9)(7.12)[/latex]

52)  [latex](2.3)(9.41)[/latex]

53)  [latex](-4.3)(2.71)[/latex]

54)  [latex](-8.5)(1.69)[/latex]

55)  [latex](-5.18)(-65.23)[/latex]

56)  [latex](-9.16)(-68.34)[/latex]

57)  [latex](0.06)(21.75)[/latex]

58)  [latex](0.08)(52.45)[/latex]

59)  [latex](9.24)(10)[/latex]

60)  [latex](6.531)(10)[/latex]

61)  [latex](55.2)(1000)[/latex]

62)  [latex](99.4)(1000)[/latex]

Odd Answers

49)  [latex]0.144[/latex]

51)  [latex]42.008[/latex]

53)  [latex]-11.653[/latex]

55)  [latex]337.8914[/latex]

57)  [latex]1.305[/latex]

59)  [latex]92.4[/latex]

61)   [latex]55\text{,}200[/latex]

Exercises: Divide Decimals

Instructions: For questions 63–76, divide.

63)  [latex]4.75\div25[/latex]

64)  [latex]12.04\div43[/latex]

65)  [latex]$117.25\div48[/latex]

66)  [latex]$109.24\div36[/latex]

67)  [latex]0.6\div0.2[/latex]

58)  [latex]0.8\div0.4[/latex]

69)  [latex]1.44\div(-0.3)[/latex]

70)  [latex]1.25\div(-0.5)[/latex]

71)  [latex]-1.75\div(-0.05)[/latex]

72)  [latex]-1.15\div(-0.05)[/latex]

73)  [latex]5.2\div2.5[/latex]

74)  [latex]6.5\div3.25[/latex]

75)  [latex]11\div0.55[/latex]

76)  [latex]14\div0.35[/latex]

Odd Answers

63)  [latex]0.19[/latex]

65)  [latex]$2.44[/latex]

67)  [latex]3[/latex]

69)  [latex]-4.8[/latex]

71)  [latex]35[/latex]

73)  [latex]2.08[/latex]

75)  [latex]20[/latex]

Exercises: Convert Decimals and Fractions

Instructions: For questions 77–98, write each decimal as a fraction.

77)  [latex]0.04[/latex]

78)  [latex]0.19[/latex]

79)  [latex]0.52[/latex]

80)  [latex]0.78[/latex]

81)  [latex]1.25[/latex]

82)  [latex]1.35[/latex]

83)  [latex]0.375[/latex]

84)  [latex]0.464[/latex]

85)  [latex]0.095[/latex]

86)  [latex]0.085[/latex]

Instructions: For questions 87-98, convert each fraction to a decimal.

87)  [latex]\frac{17}{20}[/latex]

88)  [latex]\frac{13}{20}[/latex]

89)  [latex]\frac{11}{4}[/latex]

90)  [latex]\frac{17}{4}[/latex]

91)  [latex]-\frac{310}{25}[/latex]

92)  [latex]-\frac{284}{25}[/latex]

93)  [latex]\frac{15}{11}[/latex]

94)  [latex]\frac{18}{11}[/latex]

95)  [latex]\frac{15}{111}[/latex]

96)  [latex]\frac{25}{111}[/latex]

97)  [latex]2.4+\frac{5}{8}[/latex]

98)  [latex]3.9+\frac{9}{20}[/latex]

Odd Answers

77)  [latex]\frac{1}{25}[/latex]

79)  [latex]\frac{13}{25}[/latex]

81)  [latex]\frac{5}{4}[/latex]

83) [latex]\frac{3}{8}[/latex]

85) [latex]\frac{19}{200}[/latex]

87) [latex]0.85[/latex]

89) [latex]2.75[/latex]

91) [latex]-12.4[/latex]

93) [latex]1.\overline{36}[/latex]

95) [latex]0.\overline{135}[/latex]

97)  [latex]3.025[/latex]

Exercises: Convert Decimals and Percents

Instructions: For questions 99–108, convert each percent to a decimal.

99)  [latex]1\%[/latex]

100)  [latex]2\%[/latex]

101)  [latex]63\%[/latex]

102)  [latex]71\%[/latex]

103)  [latex]150\%[/latex]

104)  [latex]250\%[/latex]

105)  [latex]21.4\%[/latex]

106)  [latex]39.3\%[/latex]

107)  [latex]7.8\%[/latex]

108)  [latex]6.4\%[/latex]

Instructions: For questions 109–118, convert each decimal to a percent.

109)  [latex]0.01[/latex]

110)  [latex]0.03[/latex]

111)  [latex]1.35[/latex]

112)  [latex]1.56[/latex]

113)  [latex]3[/latex]

114)  [latex]4[/latex]

115)  [latex]0.0875[/latex]

116)  [latex]0.0625[/latex]

117)  [latex]2.254[/latex]

118)  [latex]2.317[/latex]

Odd Answers

99) [latex]0.01[/latex]

101) [latex]0.63[/latex]

103) [latex]1.5[/latex]

105) [latex]0.214[/latex]

107) [latex]0.078[/latex]

109) [latex]1\%[/latex]

111) [latex]135\%[/latex]

113) [latex]300\%[/latex]

115) [latex]8.75\%[/latex]

117) [latex]225.4\%[/latex] 

Exercises: Everyday Math

Instructions: For questions 119–124, answer the given everyday math word problems.

119)  Salary Increase: Danny got a raise and now makes [latex]$58\text{,}965.95[/latex] a year. Round this number to the nearest

a)  dollar
b)  thousand dollars
c)  ten thousand dollars

120)  New Car Purchase: Selena’s new car cost [latex]$23\text{,}795.95[/latex]. Round this number to the nearest

a)  dollar
b)  thousand dollars
c)  ten thousand dollars

121)  Sales Tax: Hyo Jin lives in San Diego. She bought a refrigerator for [latex]$1\text{,}624.99[/latex] and when the clerk calculated the sales tax it came out to exactly [latex]$142.186625[/latex]. Round the sales tax to the nearest

a)  penny
b)  dollar

122)  Sales Tax: Jennifer bought a [latex]$1\text{,}038.99[/latex] dining room set for her home in Cincinnati. She calculated the sales tax to be exactly [latex]$67.53435[/latex]. Round the sales tax to the nearest

a)  penny
b)  dollar

123)  Paycheck. Annie has two jobs. She gets paid [latex]$14.04[/latex] per hour for tutoring at City College and [latex]$8.75[/latex] per
hour at a coffee shop. Last week she tutored for 8 hours and worked at the coffee shop for 15 hours.
a)  How much did she earn?
b)  If she had worked all 23 hours as a tutor instead of working both jobs, how much more would she have earned?
124)  Paycheck. Jake has two jobs. He gets paid [latex]$7.95[/latex] per hour at the college cafeteria and [latex]$20.25[/latex] at the art gallery. Last week he worked 12 hours at the cafeteria and 5 hours at the art gallery.
a)  How much did he earn?
b)  If he had worked all 17 hours at the art gallery instead of working both jobs, how much more would he have earned?

Odd Answers 

119a)  [latex]$58\text{,}966[/latex]
119b)  [latex]$59\text{,}000[/latex]
119c)  [latex]$60\text{,}000[/latex]

121a)  [latex]$142.19[/latex]
121b)  [latex]$142[/latex]

123a)  [latex]$243.57[/latex] 
123b)  [latex]$79.35[/latex] 

Exercises: Writing Exercises

Instructions: For questions 125–128, answer the given writing exercises.

125)  How does knowing about US money help you learn about decimals?

126)  Explain how you write “three and nine hundredths” as a decimal.

127)  Without solving the problem “[latex]44[/latex] is [latex]80\%[/latex] of what number” think about what the solution might be. Should it be a number that is greater than [latex]44[/latex] or less than [latex]44[/latex]? Explain your reasoning.

128)  When the Szetos sold their home, the selling price was [latex]500\%[/latex] of what they had paid for the house 30 years ago. Explain what [latex]500\%[/latex] means in this context.

Odd Answers

125)  Answers may vary

127)  Answers may vary

 

definition

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Medical Mathematics Copyright © 2024 by Mike LePine is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book