Chapter 3 Review Exercises
True or False. In the following exercises, justify your answer with a proof or a counterexample.
1. A function has to be continuous at ![]() if the
if the ![]() exists.
exists.
2. You can use the quotient rule to evaluate ![]() .
.
Solution
False
3. If there is a vertical asymptote at ![]() for the function
for the function ![]() , then
, then ![]() is undefined at the point
is undefined at the point ![]() .
.
4. If ![]() does not exist, then
does not exist, then ![]() is undefined at the point
is undefined at the point ![]() .
.
Solution
False. A removable discontinuity is possible.
5. Using the graph, find each limit or explain why the limit does not exist.

In the following exercises, evaluate the limit algebraically or explain why the limit does not exist.
6. ![]()
Solution
5
7. ![]()
8. ![]()
Solution
![]()
9. ![]()
10. ![]()
Solution
DNE
11. ![]()
12. ![]()
Solution
![]()
13. ![]()
14. ![]()
Solution
−4
15. ![]()
In the following exercises, use the squeeze theorem to prove the limit.
16. ![]()
Solution
Since ![]() , then
, then ![]() . Since
. Since ![]() , it follows that
, it follows that ![]() .
.
17. ![]()
18. Determine the domain such that the function ![]() is continuous over its domain.
is continuous over its domain.
Solution
![]()
In the following exercises, determine the value of ![]() such that the function remains continuous. Draw your resulting function to ensure it is continuous.
such that the function remains continuous. Draw your resulting function to ensure it is continuous.
19. 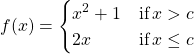
20. 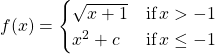
Solution
![]()
In the following exercises, use the precise definition of limit to prove the limit.
21. ![]()
22. ![]()
Solution
![]()
23. A ball is thrown into the air and the vertical position is given by ![]() . Use the Intermediate Value Theorem to show that the ball must land on the ground sometime between 5 sec and 6 sec after the throw.
. Use the Intermediate Value Theorem to show that the ball must land on the ground sometime between 5 sec and 6 sec after the throw.
24. A particle moving along a line has a displacement according to the function ![]() , where
, where ![]() is measured in meters and
is measured in meters and ![]() is measured in seconds. Find the average velocity over the time period
is measured in seconds. Find the average velocity over the time period ![]() .
.
Solution
![]() m/sec
m/sec
25. From the previous exercises, estimate the instantaneous velocity at ![]() by checking the average velocity within
by checking the average velocity within ![]() sec.
sec.

