8.1. Concepts: Tension and Compression
Introduction
Tension is the force exerted by a rope, string, or cable on one or more objects. Force of tension appears in flexible elements when an object is being suspended, pulled, swung, etc.
Compression is a force that appears in rigid elements, such as bars, beams, etc., when they are pushed or pressed against.
Examples of situations when force of tension is involved:
- A load hanging from a crane’s system of cables
- A wagon being pulled by a rope
Figure 8.1. Examples of force of tension
Concepts
Tension questions with one cable (rope, string) involved
When an object hangs from a cable, string or rope, a tension will appear in the rope that balances the force of gravity acting on the object. The object will be in equilibrium.To draw a FBD for this situation we can imagine that we cut the rope and replace its effect by a force – force of tension.
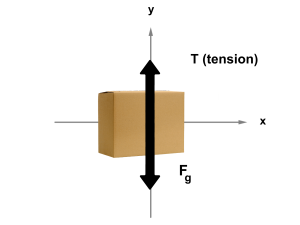
In this situation, the object is in equilibrium on both x and y axes. Therefore:
[latex]\large \vec T = F_g[/latex]
If the object hung by the rope is now given an upward acceleration, [latex]a[/latex], then the object will not be in equilibrium on the y axis anymore.
In this case we write the net force using Newton’s second law and using the net force definition:
[latex]\large F_{net} = m[/latex] X [latex]\large a[/latex]
[latex]\large \vec F_{net} = \sum \vec F[/latex]
On the y axis:
[latex]\large F_{net} = m[/latex] X [latex]\large a[/latex], and
[latex]\large F_{net} = T - F_g[/latex]
So:
[latex]\large T - F_g = m[/latex] X [latex]\large a , \hspace{.2cm}[/latex]or [latex]\large \hspace{.2cm} T = F_g \hspace{.2cm}\text + \hspace{.2cm} m[/latex] X [latex]\large a[/latex]
Image Attributions
- Figure 8.1 adapted from: A crane designed by Freepik.
- Figure 8.2 adapted from: A boy pulling a wagon designed by Freepik.
-
Figure 8.3 adapted from:
- Rope designed by Freepik
- Wooden beam designed by Freepik
- Cardboard box designed by Freepik
- Geometric figure adapted from Geogebra and licensed under CC-BY-NC-SA 3.0.
