3.1. Concepts: Vector Addition

Introduction

There are many situations arising in daily life or encountered in the sciences where vector addition is used to solve a problem. This is why adding vectors is a fundamental concept in physics and engineering, and one often seen in various real-life scenarios.

For instance, if a boat’s engine gives a velocity to the boat directed east and the water current flows with a velocity directed south, then we would need to add the two velocity vectors to find the real magnitude and direction of the resultant velocity of the boat.

Another example would be an object subjected to two or more forces directed in different directions (see image below). To find the net force acting on the object and the direction the object is moving, we would need to add all those force vectors and find a resultant.

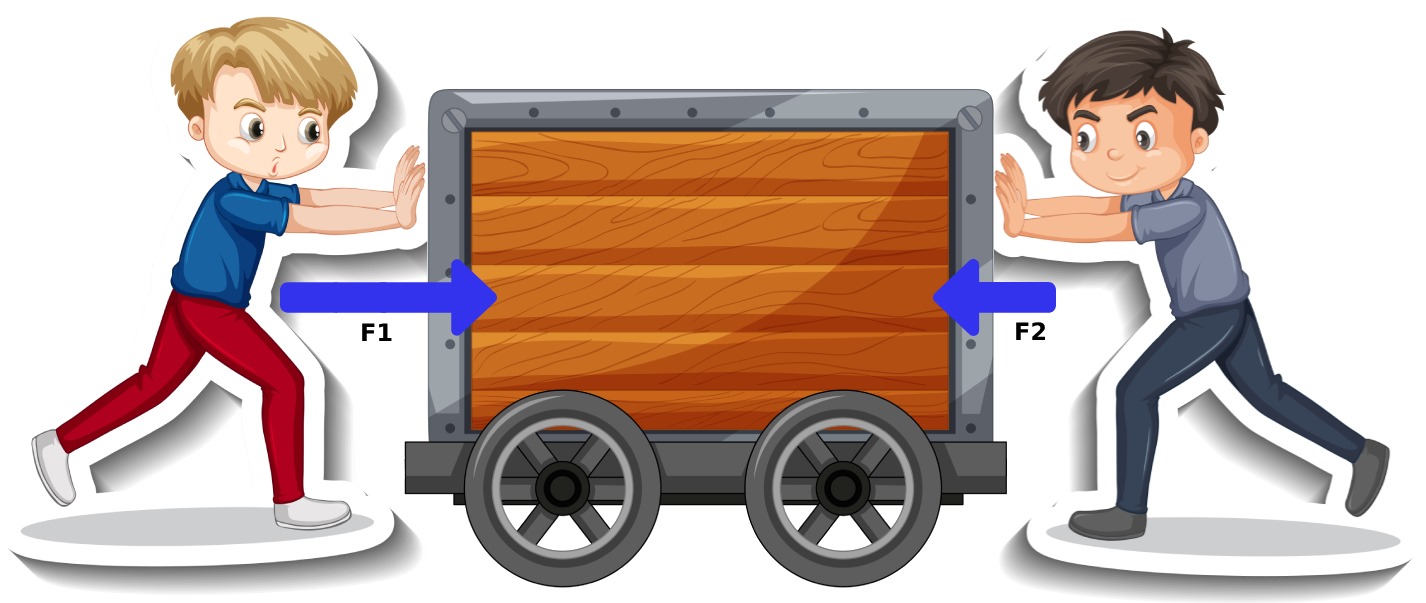
Concepts

Adding vectors can be done graphically or algebraically.

3.1.1 Graphical methods

Graphical methods are based on measuring and drawing. The equipment needed includes a ruler, a protractor and squared paper.

We will present two graphical methods: the polygon method and the parallelogram method.

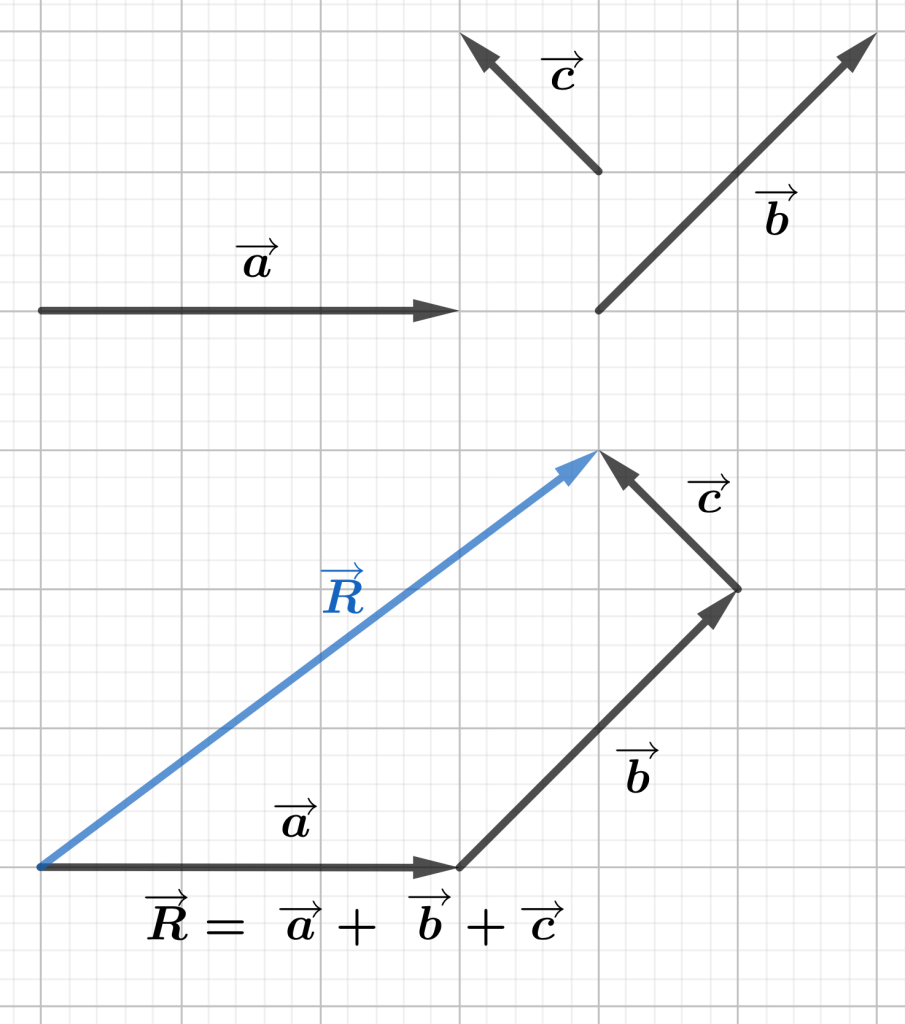
3.1.2 The polygon method

Adding two vectors in the polygon method involves the following steps:

• Choose a convenient scale. Measure and draw the first vector.
• Place the second vector with the tail to the tip of the first one.
• Continue to measure and draw the remaining vectors, each with the tail to the tip of the previous vector.
• The resultant will be the vector that connects the tail of the first vector to the tip of the last vector.

Example

Given vectors a, b, and c below, find the resultant by adding the vectors using a polygon method.

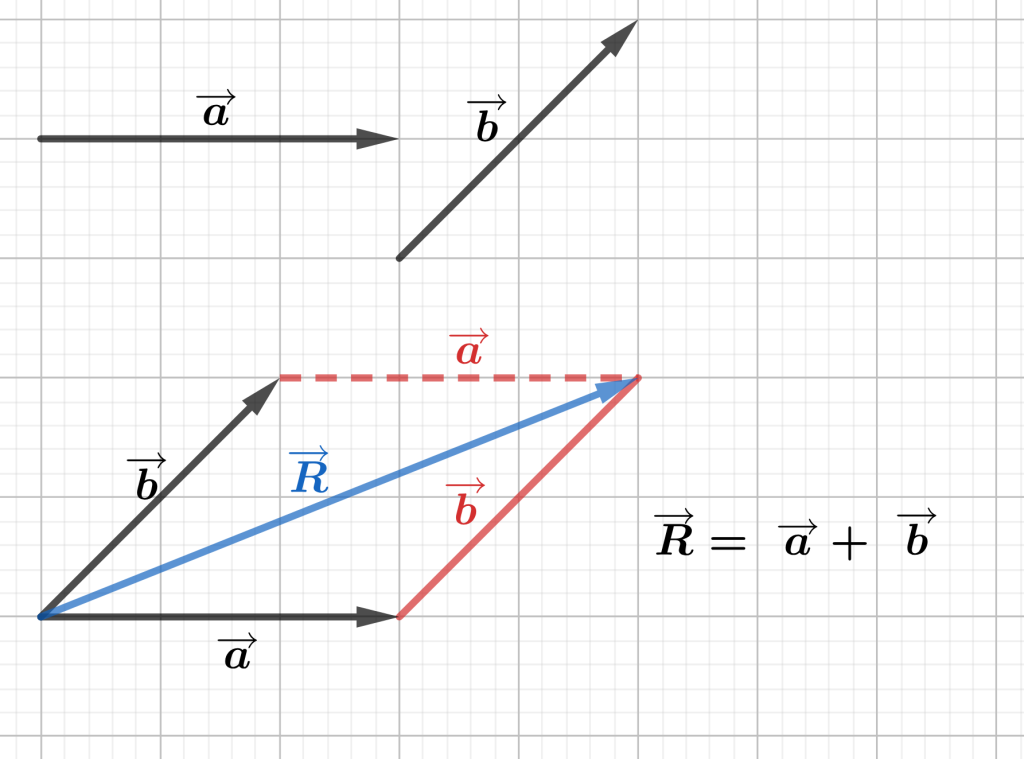
3.1.3 The parallelogram method

To add two vectors using the parallelogram method follow these steps:

1. Choose a convenient scale and draw the vectors with the tails in the same point.
2. Draw parallel lines to both vectors to create a parallelogram.
3. Connect the common tail of the vectors to the opposite end of the parallelogram (the diagonal). This will be the resultant (the sum of the two vectors).

Example

Given a and b below, find the resultant by adding the vectors using a parallelogram method.

3.1.4 The algebraic method

Adding vectors by using the algebraic method involves using the components of all vectors to be added. This is a precise method compared to the graphical method, since the latter relies on the precision of the instruments used for measuring and drawing, the thickness of the pencils, human measuring and drawing skills, etc.

To add vectors using the algebraic method, follow these steps:

• Sketch the vectors on a Cartesian plane.
• Make a table of the vectors and their components so that the x- and y-components can be added separately to find Rx and Ry (representing the x and y components of the resultant).
• Determine the quadrant in which the resultant lies.
• Calculate the magnitude of the resultant by using the Pythagorean theorem.
• Calculate the reference angle using the trigonometric function tangent.
• Calculate the angle in standard position by using the formula relating the reference angle to the standard position angle (according to the respective quadrant); see the equations in Chapter 2.

Image Attributions

• • Figure 3.1 adapted from:
    • A Wooden Cart on White Background designed by Freepik.
    • Boy pushing something cartoon character designed by Freepik.
    • Boy pushing something cartoon character designed by Freepik.
  • Figure 3.2 – 3.3: Created with Geogebra and licensed under CC-BY-NC-SA 3.0.