2.3. Practice Sets: Vectors and Scalars; Vector Components

Chapter Equations

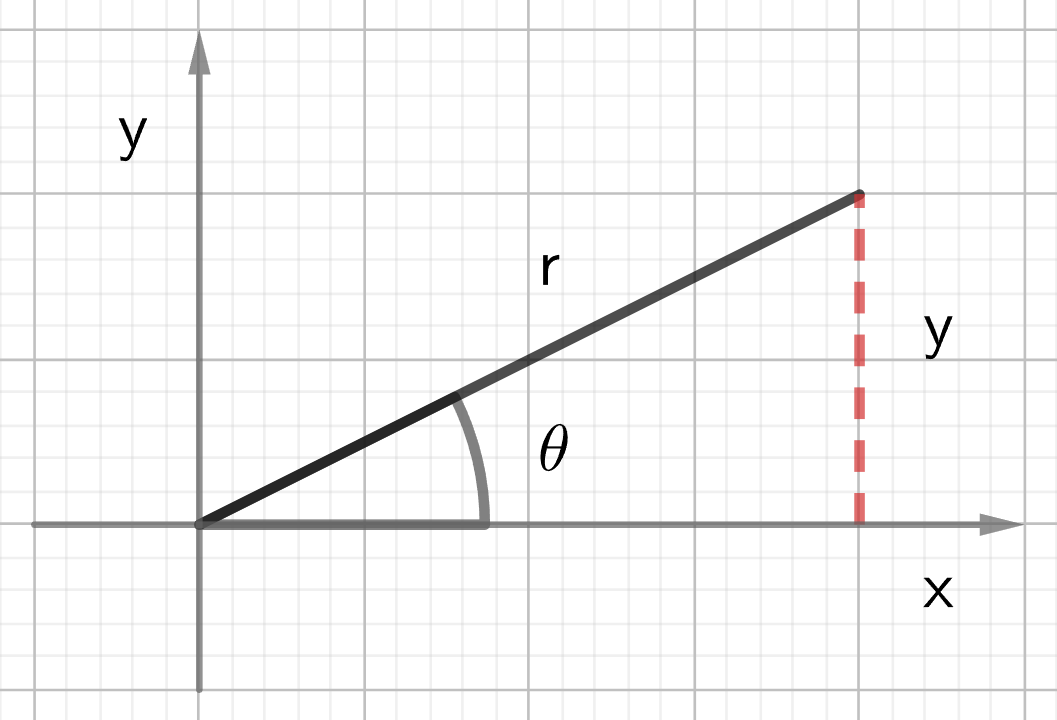
Based on the right-angle triangle in the diagram above:

[latex]x = r \cos \theta[/latex]

[latex]y = r \sin \theta[/latex]

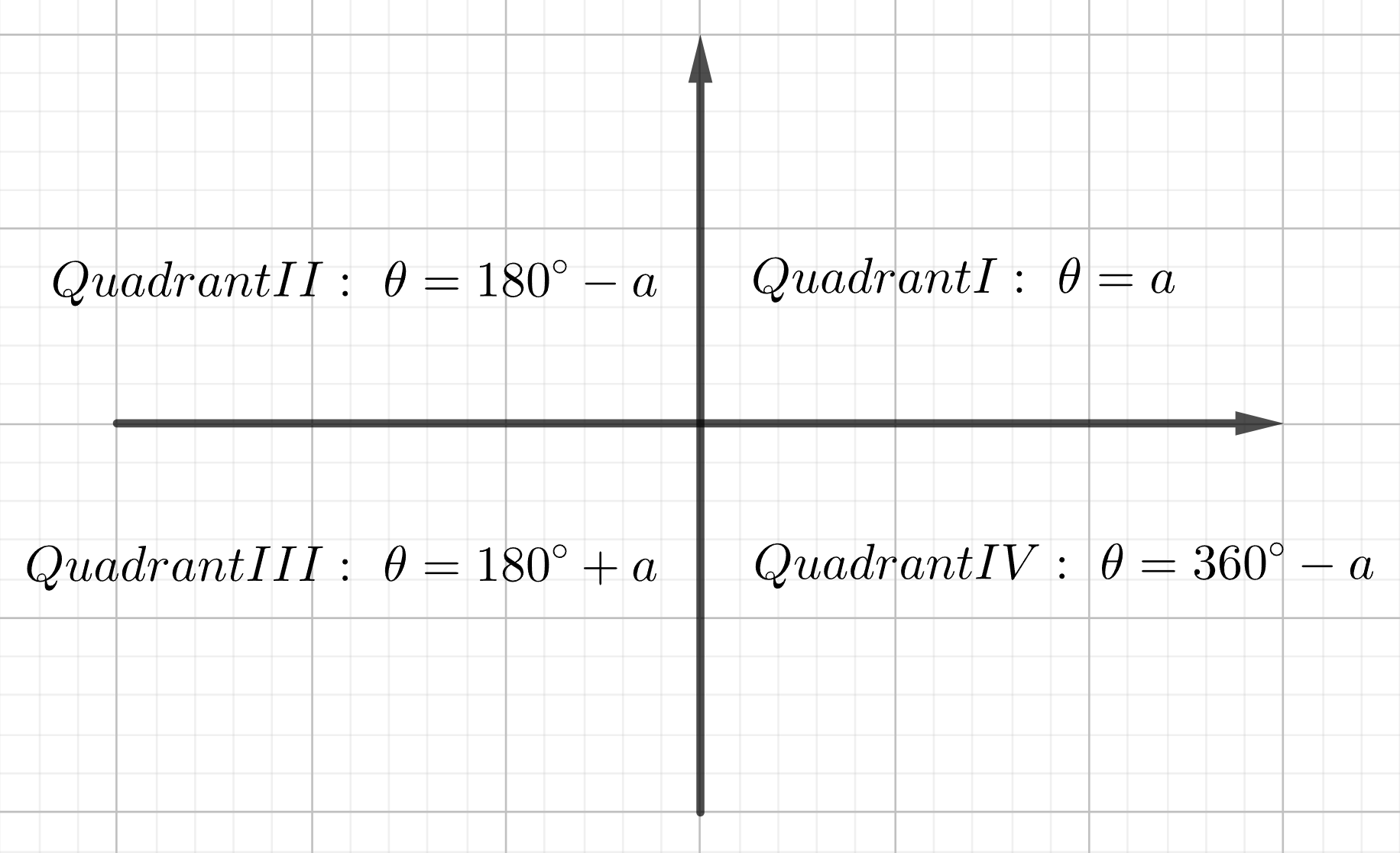
[latex]\alpha[/latex] – the reference angle is, by definition, the shortest angular distance from the terminal side of the angle to the x-axis.

[latex]\theta[/latex] – the angle in standard position is, by definition, the angle positioned with the vertex in the origin of axes and the initial side on the positive part of the x-axis. [latex]null[/latex]

If the Cartesian coordinates are known, the reference angle [latex]\alpha[/latex] can be calculated by using the inverse of tangent:

[latex]\alpha = \tan^{-1} \left(\frac{|y|}{|x|}\right)[/latex]

The Pythagorean theorem states that in a right-angle triangle:

[latex]r^2 = x^2 + y^2[/latex]

The relationship between the angle in standard position and the reference angle is:

Practice

1. Find the x and y components of a force vector [latex]\text{F = }35 \text{ N}[/latex], positioned [latex]56 \text{°}[/latex] north of west.
   Answer:
   [latex]-19.6^\circ N,\quad 29.0^\circ N[/latex]
2. Find the x and y components of a velocity vector [latex]\text{v = }20 \text{ m/s}[/latex], positioned [latex]25 \text{°}[/latex] south of east.
   Answer:
   [latex]18.1 \text{ m/s}, \quad -8.5 \text{ m/s}[/latex]
3. Sketch and find the displacement vector in standard position given the x-component is [latex]+32.2 \text{ cm}[/latex] and the y-component is [latex]–22.2 \text{ cm}[/latex].
   Answer:
   [latex]39.1 \text{ cm}, \quad 325.4^\circ[/latex]
4. Sketch and find the displacement vector in standard position given the x-component is [latex]–45 \text{ cm}[/latex] and the y-component is [latex]–75 \text{ cm}[/latex].
   Answer:
   [latex]87.5 \text{ cm}, \quad 239^\circ[/latex]

Image Attributions

• Figure 2.4 – 2.5: Created with Geogebra and licensed under CC-BY-NC-SA 3.0.