2.1. Concepts: Vectors and Scalars; Vector Components
Introduction
Vectors are physical quantities that can be completely described by a number (their magnitude), direction, and a unit.
Examples of vectors:
- Velocity
- Force
- Acceleration
- Displacement
For instance, we express velocity as [latex]60 \, \text{km/h}[/latex] due north. So, velocity is a vector because it has magnitude and direction (north). Another example of a vector is displacement. For instance, we can express a displacement as 30 km due south. Again, we see that displacement has both magnitude and direction.
Scalars are physical quantities that have a magnitude, but no direction. For instance, we can express the kinetic energy of a car travelling 30 m/s north and that of a car travelling 30 m/s south. We see that the kinetic energy will be the same regardless of the direction of travel. That means that energy is a scalar quantity.
Other examples of scalar quantities are:
- Speed
- Distance
- Work
- Power
Concepts
To find the components of a vector, we use the following diagram:
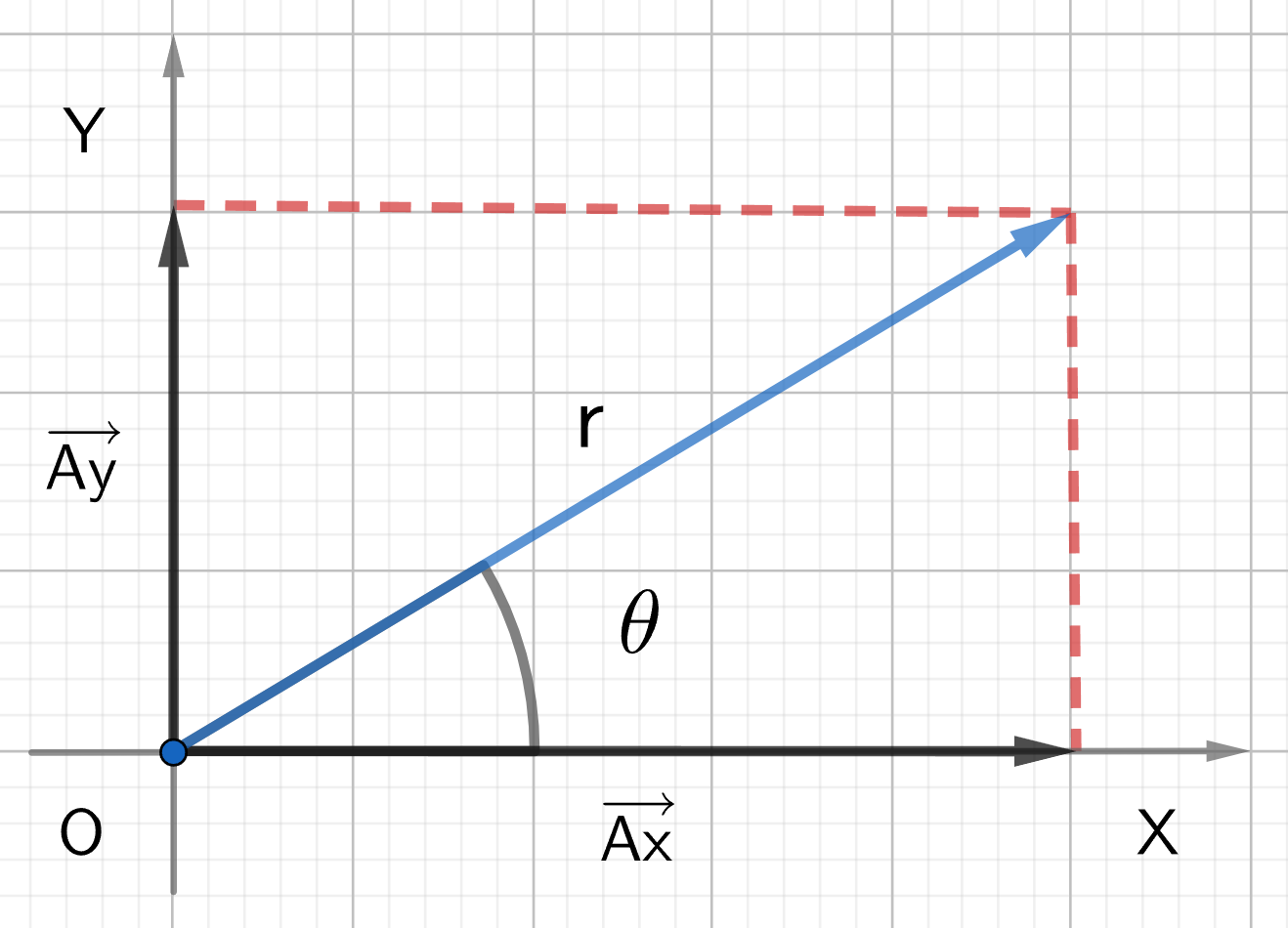
To find the x-component:
- Draw a perpendicular line from the tip of the vector to the x-axis.
- Connect the origin of the axes to the drop point.
To find the y-component:
- Draw a perpendicular to the y-axis.
- Connect the origin of the axes to the drop point.
The components can be calculated by using the trigonometric functions in the right-angle triangle from the diagram above:
Image Attributions
- Figure 2.1: Created with Geogebra and licensed under CC-BY-NC-SA 3.0.
