Speed of Sound
Introduction
2.1 Heat Capacity Ratio
The velocity of sound in gases is a particularly important measurement as it is one of the few ways to accurately measure ![]() , the ratio of the heat capacity at constant pressure (
, the ratio of the heat capacity at constant pressure (![]() ), to the heat capacity at constant volume (
), to the heat capacity at constant volume (![]() ). This heat capacity ratio, sometimes called the adiabatic index, is important to thermodynamically reversible processes involving ideal gases. The principle of the Equipartition of Energy can be used to calculate
). This heat capacity ratio, sometimes called the adiabatic index, is important to thermodynamically reversible processes involving ideal gases. The principle of the Equipartition of Energy can be used to calculate ![]() , the relevant variable being the number of degrees of freedom (translational, rotational and vibrational) that contribute to the heat capacities:
, the relevant variable being the number of degrees of freedom (translational, rotational and vibrational) that contribute to the heat capacities:
(1) ![]()
where ![]() is the number of degrees of freedom. Air is mostly diatomic molecules (
is the number of degrees of freedom. Air is mostly diatomic molecules (![]() and
and ![]() ), which have three translational degrees of freedom, and two rotational degrees of freedom. Therefore, one might expect air to have a heat capacity ratio of 1.4.
), which have three translational degrees of freedom, and two rotational degrees of freedom. Therefore, one might expect air to have a heat capacity ratio of 1.4.
2.2 Standing Waves
The essence of the experiment is to measure the velocity of sound by a resonance technique in a cylindrical tube of fixed length. At one end of the tube, a small speaker excites the sound waves. At the other end, there is a microphone that measures the amplitude of the sound waves. A standing wave is set up in the column when the frequency of the applied sound wave meets the resonance condition of the tube:
(2) ![]()
where ![]() is the length of the cylinder,
is the length of the cylinder, ![]() is the wavelength of the sound wave, and
is the wavelength of the sound wave, and ![]() is a positive integer. When a resonance condition is met, the sound wave and its reflection interfere constructively, and the amplitude of the wave increase drastically.
is a positive integer. When a resonance condition is met, the sound wave and its reflection interfere constructively, and the amplitude of the wave increase drastically.
Cylinders are not special in terms of reaching resonance conditions. Spherical cavities have resonant conditions, too, though the resonant peaks are not evenly spaced. The boundary condition for sound waves in a spherical cavity require that particle displacement and velocity be zero at the surface of the sphere. Resonant frequencies are then those frequencies that satisfy
(3) ![]()
where ![]() is the radius of the sphere,
is the radius of the sphere, ![]() is a positive integer, and
is a positive integer, and ![]() are Bessel functions of the first kind. DO NOT WORRY ABOUT BESSEL FUNCTIONS! You’ll have plenty of time to sweat Bessel functions when you are studying spherical harmonics in the hydrogen atom in some quantum mechanics course. The values of
are Bessel functions of the first kind. DO NOT WORRY ABOUT BESSEL FUNCTIONS! You’ll have plenty of time to sweat Bessel functions when you are studying spherical harmonics in the hydrogen atom in some quantum mechanics course. The values of ![]() that satisfy this boundary condition are
that satisfy this boundary condition are
(4) ![]()
where ![]() is the
is the ![]() th zero of the derivative of the spherical Bessel function of order
th zero of the derivative of the spherical Bessel function of order ![]() . Now the important result: The resonant frequencies in a spherical cavity are related to the velocity of the wave by
. Now the important result: The resonant frequencies in a spherical cavity are related to the velocity of the wave by
(5) ![]()
Please take my word for it that the resonant frequency near 3670 Hz in air is the second zero of the derivative of the Bessel function (![]() ), of order
), of order ![]() . The value of
. The value of ![]() is 3.342094. You may or may not need all of those digits.
is 3.342094. You may or may not need all of those digits.
2.3 Determining γ
Remind yourself that you are measuring these resonant conditions not just to determine the speed of sound, but to determine the heat capacity ratio, ![]() , which is also the ratio of the adiabatic bulk modulus (
, which is also the ratio of the adiabatic bulk modulus (![]() ) and the absolute pressure
) and the absolute pressure ![]() The speed of sound is related to the bulk modulus via
The speed of sound is related to the bulk modulus via
(6) 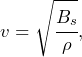
where ![]() is the density of the medium the wave is moving through. Therefore, if the speed of sound is measured, and the density of the medium is known, one can determine the bulk modulus, then determine
is the density of the medium the wave is moving through. Therefore, if the speed of sound is measured, and the density of the medium is known, one can determine the bulk modulus, then determine ![]() .
.
