Graphs

Samia CHALLAL

29 Graphs

Plane curve: is the graph of an equation in two variables; that is the set of all points $(x,y)$ that satisfy the equation.

In particular, the graph of a function $f$ is the set

$G_f=\{ (x,y): \,\, y= f(x) \,\, \hbox{ and } \,\,x\in D_f\}$

$x$ -Intercepts = $x$ -coordinates of a point where the graph crosses the $x$ -axis.

$y$ -Intercepts = $y$ -coordinates of a point where the graph crosses the $y$ -axis.

Symmetry : a graph $G$ is

$-$ symmetric with respect to the $y$ -axis if : $\qquad \,\, (a,b) \in G \quad \Longleftrightarrow\quad (-a, b)\in G$

$-$ symmetric with respect to the $x$ -axis if : $\qquad \,\, (a,b) \in G \quad \Longleftrightarrow\quad (a, -b)\in G$

$-$ symmetric with respect to the origin if : $\qquad \,\, (a,b) \in G \quad \Longleftrightarrow\quad (-a, -b)\in G$

$-$ symmetric with respect to the line $y= x$ if : $\qquad \,\, (a,b) \in G \quad \Longleftrightarrow\quad (b, a)\in G$

The vertical line test: A plane curve is the graph of a function if and only if no vertical line intersects the curve more than once.

Watch a Video

View on YouTube

Show Examples

Exercise 1

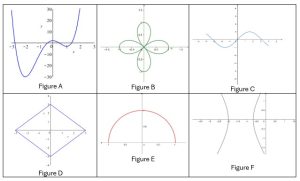
Show/Hide Solution.

Figure A is the graph of a function because the vertical line rule is satisfied.
Figure B is not the graph of a function because the vertical line rule is not satisfied.
Figure C is the graph of a function because the vertical line rule is satisfied.
Figure D cannot be the graph of a function because the vertical line 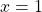$x = 1$ intersects the curve into two different points 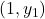$(1, y_1)$ and $(1, y_2)$ .
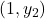Figure E is the graph of a function because the vertical line rule is satisfied.
Figure F is not the graph of a function because the vertical line rule is not satisfied.

Exercise 2

Show/Hide Solution.

Set $x=0$ , then $y^2={25}$ . Hence $y=\pm {5}$ are the $y$ -intercepts.