Simplifying Rational Expressions

Samia CHALLAL

14 Simplifying Rational Expressions

* A rational expression
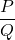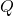is an expression that can be simplified in the form $\displaystyle{ \frac{P}{Q} }$ where $P$ and $Q$ are polynomials.

* To simplify a rational expression, we can:

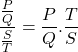
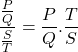
– combine numerator and denominator into single quotients, then divide : $\displaystyle{ \frac{\frac{P}{Q}}{\frac{S}{T}} = \frac{P}{Q} . \frac{T}{S}}$

– reduce to the same denominator : 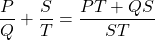$\displaystyle{ \frac{P}{Q} + \frac{S}{T} = \frac{P T + Q S }{ST} }$

– reduce to lower terms : 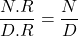$\displaystyle{ \frac{N.R}{D.R} = \frac{N}{D} }$

Watch a Video

View on YouTube

Show Examples

Exercise 1

Show/Hide Solution.

We have

$x^2 -{6}x + {8} = (x-{2})(x-{4})$

$x^2 +{6}x -{16} = (x-{2})(x+{8})$ .

Thus, by simplifying the common factor $(x-{2})$ , we obtain

$\displaystyle{\frac{x^2 -{6}x + {8} }{ x^2 +{6}x -{16}} = \frac{(x-{2})(x-{4})}{(x-{2})(x+{8})} =\frac{x-{4}}{x+{8}}}$ .

Exercise 2

Show/Hide Solution.

We have

$\displaystyle{\frac{ (x-{4})( {3}x+ {2} ) }{ x^3-{64} } }$ = $\displaystyle{\frac{ (x-{4})( {3}x+ {2} ) }{(x-{4}) (x^2 +{4}x + {16}) } }$ = $\displaystyle{\frac{ {3}x+ {2} }{x^2 +{4}x + {16} } }$ .