Annuities
3.5 Number of Payments and Time: Calculator Approach
A. Introduction
Suppose you are setting aside funds for a renovation project with a target of $50,000. You plan to save $100 every week and deposit it into your savings account. The key question is how long will it take to reach your $50,000 savings goal. To find this out, you need to first calculate the number of weekly payments (N) you must make to accumulate the desired amount. Once you have determined N, you will know the exact duration required to achieve your savings target for the renovation project.
In this section, we turn our attention to computing the total number of payments in annuities (N) and understanding how long it takes for an annuity to achieve its financial objectives, whether it’s accumulating a desired sum for retirement, paying off a loan, or reaching a specific investment goal.
When the time of the annuity term (t) is unknown, the initial step is to calculate N using either a formula or a financial calculator. Once N is known, it can then be used to work out the time. Depending on the scenario, whether it involves retirement plans, loans, or savings schemes, the calculation of N may be based on the Periodic Payment (PMT) and either the Future Value (FV), the Present Value (PV) of the annuity, or a combination of both.
For the calculator approach, we use the time-value-of-money (TVM) worksheet in financial calculators. It’s also important to follow the cash flow sign convention (see Table 2.2.1 in Section 2.2) when inputting monetary values into the calculator. This ensures consistency and accuracy in our calculations.
It is important to note that in annuities calculations, unlike compound interest calculation where N is the number of compounding periods in the term, N represents the number of payments in the term. This value is always rounded up to the next whole number of payments, which may result in the last payment being smaller than the others.
Once N is computed, the annuity’s term (t) can be determined by rearranging Formula 3.1a.
[asciimath]t=N/(P//Y)[/asciimath]Formula 3.1b
B. Payment of Ordinary Annuity
Mahsa wants to save $80,000 for her house down payment. Suppose she plans to make deposits of $1,290 at the end of every three months into an account earning 4.7% compounded quarterly. a) How many deposits will she have to make to achieve her goal? b) How long will it take? Give the time in terms of years and months.
Show/Hide Solution
Given Information
- Interest is compounded quarterly so [asciimath]C//Y = 4[/asciimath]
- Payments are made at the end of every three months so [asciimath]P//Y = 4[/asciimath]
[asciimath]C//Y = P//Y[/asciimath] [asciimath]=>[/asciimath] Ordinary Simple Annuity
- Nominal interest rate: [asciimath]I//Y = 4.7%[/asciimath]
- No initial investment: [asciimath]PV=0[/asciimath]
- The payments are made to a bank, so they are cash outflow: [asciimath]PMT = -$1,290[/asciimath]
- Future value is received, so it is a cash inflow: [asciimath]FV = $80,000[/asciimath]
a) [asciimath]N=?[/asciimath]

The number of payments in the term, N, is rounded up to 47 deposits.
b) [asciimath]t=?[/asciimath]
To find the time period of the term, we substitute N and P/Y into Formula 3.1b:
[asciimath]t=N/(P//Y)[/asciimath]
[asciimath]t=47/4[/asciimath]
[asciimath]=11.75[/asciimath] years
[asciimath]=11[/asciimath] years [asciimath]+ 0.75(12)[/asciimath] months
[asciimath]=11[/asciimath] years and [asciimath]9[/asciimath] months
Note: In annuities, N represents the number of payments in the term and its value is always rounded up to the next whole number of payments regardless of the decimal part of the calculated N.
Try an Example
Mahsa already has $25,000 in her savings account, and she wants this to accumulate to $80,000 for a house down payment. Suppose she plans to make deposits of $1,290 at the end of every three months into the account earning 4.7% compounded quarterly. a) How many deposits will she have to make to achieve her goal? b) How long will it take? Give the time in terms of years and months.
Show/Hide Solution
Given Information
- Interest is compounded quarterly so [asciimath]C//Y = 4[/asciimath]
- Payments are made at the end of every three months so [asciimath]P//Y = 4[/asciimath]
[asciimath]C//Y = P//Y[/asciimath] [asciimath]=>[/asciimath] Ordinary Simple Annuity
- Nominal interest rate: I/Y = 4.7%
- The payments are made to a bank, so they are cash outflow: [asciimath]PMT = -$1,290[/asciimath]
- Present value is a lumpsum invested, so it is a cash outflow: [asciimath]PV = -$25,000[/asciimath]
- Future value is received, so it is a cash inflow: [asciimath]FV = $80,000[/asciimath]
a) [asciimath]N=?[/asciimath]

The number of payments in the term, N, is rounded up to 30 deposits.
b) [asciimath]t=?[/asciimath]
To find the time period of the term, we substitute N and P/Y into Formula 3.1b:
[asciimath]t=N/(P//Y)[/asciimath]
[asciimath]t=30/4[/asciimath]
[asciimath]=7.5[/asciimath] years
[asciimath]=7[/asciimath] years [asciimath]+ 0.5(12)[/asciimath] months
[asciimath]=7[/asciimath] years and [asciimath]6[/asciimath] months
Try an Example
Emanuel won a lottery prize of $1,000,000 and invested it in a fund that earns 4% interest compounded monthly. He plans to withdraw $1,000 at the end of each week. a) Determine the total number of withdrawals Emanuel will make. b) How long will the money last with these weekly withdrawals? Give the time in terms of years and weeks.
Show/Hide Solution
Given Information
- Interest is compounded annually so [asciimath]C//Y = 12[/asciimath]
- Withdrawals are at the end of every week so [asciimath]P//Y = 52[/asciimath]
[asciimath]C//Y != P//Y[/asciimath] [asciimath]=>[/asciimath] Ordinary General Annuity
- Nominal interest rate: [asciimath]I//Y = 4%[/asciimath]
- Payments are received, so they are cash inflow: [asciimath]PMT = $1000[/asciimath]
- Present value is invested in a bank, so it is a cash outflow: [asciimath]PV = -$1,000,000[/asciimath]
- The account will be depleted at the end of the term: [asciimath]FV=0[/asciimath]
a) [asciimath]N=?[/asciimath]

The number of payments in the term, N, is rounded up to 1904 withdrawals.
b) [asciimath]t=?[/asciimath]
To find the time period of the term, we substitute N and P/Y into Formula 3.1b:
[asciimath]t=N/(P//Y)[/asciimath]
[asciimath]t=1904/52[/asciimath]
[asciimath]=36.6153...[/asciimath] years
[asciimath]=36[/asciimath] years [asciimath]+ 0.6153... (52)[/asciimath] weeks
[asciimath]=36[/asciimath] years and [asciimath]32[/asciimath] weeks
C. Payment of Annuity Due
In the following examples, we will focus on calculating N and the time for annuities due. In these annuities, since payments are made at the start of each payment period, it is important to change the payment timing setting on your calculator to ‘BGN’ (beginning of the payment period).
Esmeralda wants to accumulate $71,000 for her condominium down payment. She plans to make deposits of $3,890 at the beginning of every three months into her savings account, which earns interest at 5.29% compounded quarterly. a) How many deposits will she have to make to achieve her goal? b) How long will it take? Give the time in terms of years and months.
Show/Hide Solution
Given Information
- Annuity Due: Set your calculator to BGN mode
- Interest is compounded quarterly so [asciimath]C//Y = 4[/asciimath]
- Payments are made at the beginning of every three months so [asciimath]P//Y = 4[/asciimath]
[asciimath]C//Y = P//Y[/asciimath] [asciimath]=>[/asciimath] Simple Annuity Due
- Nominal interest rate: [asciimath]I//Y = 5.29%[/asciimath]
- No initial balance: [asciimath]PV=0[/asciimath]
- Payments are made, so they are cash outflow: [asciimath]PMT = -$3,890[/asciimath]
- Future value is received, so it is a cash inflow: [asciimath]FV = $71,000[/asciimath]
a) [asciimath]N=?[/asciimath]

The number of payments in the term, N, is rounded up to 17 deposits.
b) [asciimath]t=?[/asciimath]
To find the time period of the term, we substitute N and P/Y into Formula 3.1b:
[asciimath]t=N/(P//Y)[/asciimath]
[asciimath]t=17/4[/asciimath]
[asciimath]=4.25[/asciimath] years
[asciimath]=4[/asciimath] years [asciimath]+ 0.25(12)[/asciimath] months
[asciimath]=4[/asciimath] years and [asciimath]3[/asciimath] months
Try an Example
Dario recently retired and has $500,000 in his retirement fund, which earns an annual interest rate of 8% compounded annually. He intends to withdraw $4,000 at the beginning of every month. a) Calculate the total number of withdrawals Dario will make. b) How long will it take for the fund to be depleted?
Show/Hide Solution
Given Information
- Annuity Due: Set your calculator to BGN mode
- Interest is compounded annually so [asciimath]C//Y = 1[/asciimath]
- Payments are withdrawn at the beginning of every month so [asciimath]P//Y = 12[/asciimath]
[asciimath]C//Y != P//Y[/asciimath] [asciimath]=>[/asciimath] General Annuity Due
- Nominal interest rate: [asciimath]I//Y = 8%[/asciimath]
- Payments are received, so they are cash inflow: [asciimath]PMT = $4,000[/asciimath]
- Present value is invested in a bank, so it is a cash outflow: [asciimath]PV = -$500,000[/asciimath]
- The fund balance at the end of the term will be zero, so [asciimath]FV=0[/asciimath]
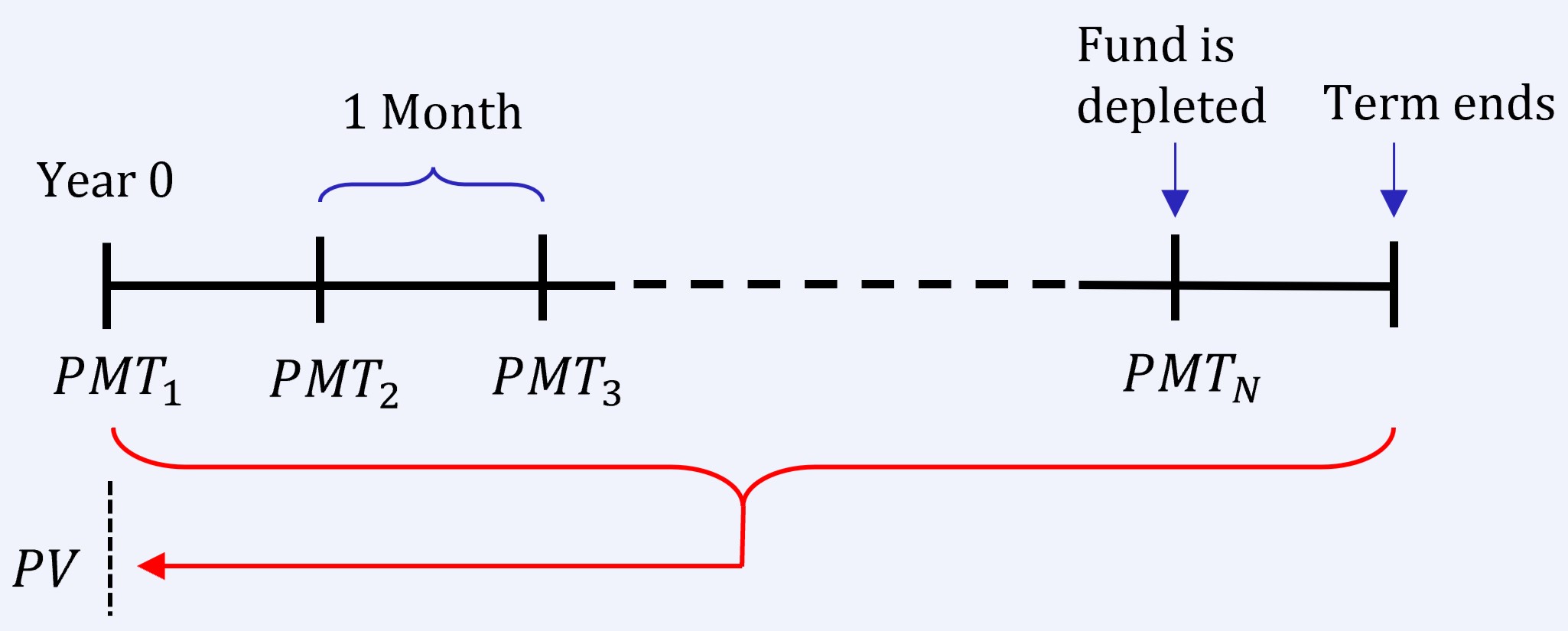
a) [asciimath]N=?[/asciimath]

The number of payments in the term, N, is rounded up to 251 withdrawals.
b) [asciimath]t=?[/asciimath]
To find the duration of the annuity term, we substitute N and P/Y into Formula 3.1b:
[asciimath]t=N/(P//Y)[/asciimath]
[asciimath]t=251/12[/asciimath]
[asciimath]=20.91bar(6)[/asciimath] years
[asciimath]=20[/asciimath] years [asciimath]+ 0.91bar6(12)[/asciimath] months
[asciimath]=20[/asciimath] years and [asciimath]11[/asciimath] months
As shown in the timeline, in an annuity due, the term extends beyond the last withdrawal by one period (in this case, one month). Because our focus is on the timeframe up to the final withdrawal (when the fund is depleted), we must deduct one period (one month) from the annuity due term.
Thus, the fund will be depleted in 20 years and 10 months.
Section 3.5 Exercises
- Karina wants to accumulate $52,500 for her condominium down payment. She plans to make deposits of $1,330 at the beginning of every month into her savings account, which earns interest at 6.61% compounded monthly. a) How many deposits will Karina have to make to achieve her goal? b) How long will it take? Give the time in terms of years and months.
Show/Hide Answer
a) N = 36 deposits
b) t = 3 years and 0 months
- BoardWalk Corporation aims to save a total of $641,000 over the coming years. They plan to start with an initial deposit of $176,800 and then make deposits of $5,200 at the end of each month. (a) Determine the number of additional monthly deposits and (b) the total time required for BoardWalk Corporation to accumulate $641,000, considering an interest rate of 6.37% compounded semi-annually. Give the time in years and months.
Show/Hide Answer
a) N = 64 payments
b) t = 5 years and 4 months
- Alo has saved $2,500,000 in his retirement fund, which earns an annual interest rate of 1.5% compounded daily. He intends to withdraw $10,000 at the beginning of every month. a) Calculate the total number of withdrawals Alo will make. b) Determine the duration for which Alo’s retirement fund will sustain these monthly withdrawals. Give the time in years and months.
Show/Hide Answer
a) N = 300 withdrawals
b) t = 25 years

